AP Calculus AB Exam Practice Strategies and Tips

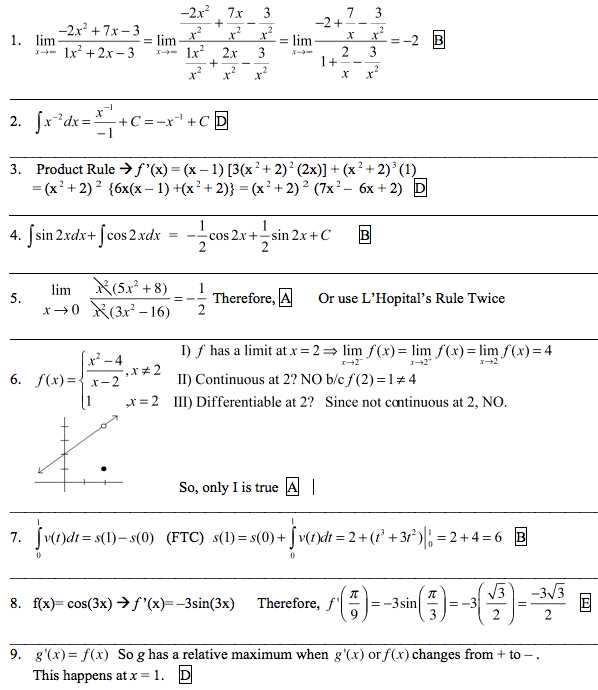
Preparing for advanced mathematics assessments requires a clear and structured approach. Mastery of key concepts is essential, and consistent effort can significantly improve performance. To succeed in such evaluations, it’s crucial to engage in targeted exercises that challenge your understanding and strengthen your problem-solving skills.

Developing a solid foundation in the core principles of calculus is necessary to approach the questions with confidence. By reviewing topics in a logical sequence and testing your knowledge regularly, you can refine your skills and identify areas that need more attention. This process will help you gain the proficiency needed to excel in high-level mathematics challenges.

Strategic preparation involves more than simply completing exercises. It’s about focusing on the areas that require the most improvement and ensuring that you have a comprehensive understanding of both theoretical concepts and practical applications. The right approach will not only help you perform well but also enhance your overall understanding of the subject.

AP Calculus AB Exam Practice

Preparing for high-level mathematics assessments requires an in-depth approach to reinforce key concepts and hone problem-solving abilities. It is essential to tackle a wide variety of questions, focusing on both theoretical knowledge and practical application. This approach ensures a thorough understanding and boosts confidence when it comes time to face the actual challenge.

Strengthening Fundamental Skills

Success in advanced mathematics depends on a deep grasp of the foundational concepts. Working through a diverse set of problems allows you to test your knowledge and discover areas that need additional focus. Whether reviewing derivatives, integrals, or more complex topics, strengthening these basics ensures that you can confidently address even the most challenging questions.

Effective Time Management

Time is one of the most valuable resources when preparing for such assessments. It is important to simulate test conditions by timing yourself during exercises. This helps improve both your speed and accuracy, ensuring that you can work through problems efficiently under pressure. Regular timed sessions will also help you develop strategies for managing complex tasks within the limited time frame.

Understanding the AP Calculus AB Format

Familiarity with the structure of advanced mathematics assessments is crucial for effective preparation. Knowing what to expect in terms of question types and overall organization allows you to approach the challenge with confidence and a clear strategy. Understanding the test format enables you to tailor your preparation efforts for maximum impact.

Components of the Assessment

The assessment consists of two main sections: multiple-choice questions and free-response questions. Each section evaluates different aspects of your understanding and problem-solving abilities.

• Multiple-Choice Section: This part consists of several questions where you select the correct answer from a list of options. It tests your ability to apply concepts and solve problems quickly and accurately.
• Free-Response Section: This section requires you to solve problems and provide detailed explanations for your solutions. It tests your ability to communicate mathematical reasoning and apply concepts to more complex scenarios.

Time Management and Strategies

Both sections have specific time limits, which means managing your time efficiently is essential. Here are some tips:

1. For the multiple-choice section, work quickly but carefully. Skip difficult questions and return to them if time permits.
2. In the free-response section, read each problem thoroughly before beginning. Plan your solutions, write clear steps, and check your work if time allows.
3. Simulate test conditions during your preparation to improve your time management and comfort with the format.

Essential Topics to Study for the Exam

To succeed in advanced mathematics assessments, it’s important to focus on key areas that are frequently tested. Mastering these fundamental topics will not only improve your problem-solving abilities but also help you build a strong foundation for more complex questions. Thorough preparation in these areas is essential for achieving a high score.

Functions and Their Properties are one of the core concepts you need to understand deeply. This includes exploring various types of functions, such as polynomial, rational, and trigonometric, and understanding their behavior, limits, and transformations. You will need to apply this knowledge to solve problems involving graphing, asymptotes, and continuity.

Derivatives and Their Applications are central to many problems. Understanding how to calculate derivatives and apply the rules of differentiation is crucial. Focus on the application of derivatives, including rates of change, optimization, and motion problems. Recognizing the significance of the first and second derivatives will help you approach a wide range of questions with confidence.

Integrals and Area Calculations also play a major role in the assessment. You will need to understand how to calculate definite and indefinite integrals and interpret their geometric meanings, such as finding areas under curves. Be sure to practice integration techniques such as substitution and integration by parts, as these are key to solving more complex problems.

Series and Sequences represent another area of focus. You should be comfortable with convergence and divergence, and be able to apply tests to determine whether a series converges. Understanding the behavior of sequences and series will enable you to solve a variety of challenging problems efficiently.

Effective Time Management for Practice

One of the most important skills to develop when preparing for a challenging mathematical assessment is effective time management. Learning how to allocate your time wisely allows you to maximize your preparation efforts, ensuring you cover all necessary topics without feeling rushed. By practicing good time management, you can approach the test with greater confidence and clarity.

Setting Realistic Goals

Before diving into practice sessions, it’s essential to establish clear, achievable goals. This will help you stay focused and measure progress. Break down large topics into smaller, manageable tasks to avoid feeling overwhelmed. Prioritize areas that require more attention based on your strengths and weaknesses.

• Daily Goals: Set specific goals for each study session. Focus on one or two topics, and try to master them before moving on.
• Weekly Goals: Review all the topics you’ve studied during the week. Take a practice test to evaluate your understanding and adjust your goals accordingly.
• Long-Term Goals: Keep track of your overall progress. Set milestones to ensure that you are on track to cover all material before the test.

Timing Your Sessions

Allocating the right amount of time to each practice session is critical. By timing yourself, you can simulate real test conditions and learn how to work under pressure. This also helps you develop a sense of how long you need to spend on various types of questions.

1. Set Time Limits: During each practice session, set a timer for each task or question to avoid spending too much time on any one thing.
2. Break Sessions into Blocks: Study in focused intervals, such as 25 to 30 minutes, followed by short breaks. This method helps maintain concentration and prevents burnout.
3. Adjust Time as Needed: If you’re struggling with a particular topic, allocate extra time to it. Flexibility allows you to target areas that require more attention.

How to Solve Free Response Questions

When facing open-ended questions in advanced mathematics assessments, it is essential to approach each problem systematically. Unlike multiple-choice questions, these require not only the correct answer but also a detailed explanation of the process. By following a clear strategy, you can effectively tackle these challenges and demonstrate your reasoning skills.

Read the Question Carefully before you begin solving. Pay close attention to what is being asked and identify the key elements in the problem. Break the question into smaller parts if necessary, and make sure you understand the specific concepts involved.

Organize Your Work logically and step by step. Start with the information provided in the problem and apply the appropriate formulas or methods. Showing your work clearly is crucial, as partial credit can be awarded for correct steps even if the final answer is incorrect.

• Step 1: Write down any known values or equations that relate to the problem.
• Step 2: Apply relevant techniques, such as differentiation or integration, to solve the problem.
• Step 3: Check each step for accuracy and ensure that your solution is logical and complete.

Show Your Reasoning throughout the solution. In many cases, it is not enough to simply provide the final answer. Be sure to explain the steps you took to reach your conclusion. This demonstrates your understanding of the concepts and helps you earn more points.

Key Strategies for Multiple-Choice Questions

Multiple-choice questions are designed to test your ability to apply mathematical concepts quickly and accurately. Unlike open-ended questions, they require a strategic approach to efficiently navigate through the options and identify the correct answer. Developing effective techniques for handling these types of questions is crucial for success in any advanced mathematics assessment.

Eliminate Incorrect Options first. Often, some answers can be quickly ruled out because they are clearly wrong. Focus on identifying these options to narrow down your choices. By reducing the number of possibilities, you increase your chances of selecting the correct answer even if you’re unsure at first.

Read Carefully and pay attention to the details in both the question and the answer choices. Sometimes, small differences in wording or notation can lead to a significantly different outcome. Make sure you understand the question fully before selecting an answer.

Use Estimation Techniques when possible. In many cases, you can use estimation to eliminate options that are clearly too large or too small. For example, if the answer involves calculating an area or a rate, estimate the result based on your understanding of the problem, and rule out any options that don’t make sense.

Double-Check Your Work if time permits. After selecting your answer, take a moment to review your calculations and reasoning. This helps ensure you didn’t make a simple mistake in the process that could cost you valuable points.

Using Past Exams for Preparation

Reviewing previous assessments is one of the most effective strategies for preparing for a challenging mathematics test. By analyzing past questions, you can familiarize yourself with the types of problems likely to appear and gain insights into the format and structure of the test. This helps reduce uncertainty and boosts confidence on the day of the actual assessment.

Benefits of Reviewing Previous Assessments

Past assessments offer several advantages in your preparation process:

• Familiarity with Question Types: Understanding the variety of questions that may appear allows you to focus on the most relevant concepts.
• Time Management Practice: Working through past problems under time constraints helps improve your speed and efficiency.
• Identification of Weak Areas: By reviewing your performance on past assessments, you can identify areas that require more attention.

How to Maximize Your Use of Past Assessments

When using past tests, it’s essential to approach them strategically. Here are a few key steps to follow:

1. Start with Untimed Practice: Begin by working through the problems without a time limit to get a feel for the material.
2. Move to Timed Practice: Once you’re comfortable, simulate actual testing conditions by timing yourself.
3. Analyze Mistakes: Review any errors carefully and understand why you made them to avoid repeating them in the future.

Sample Breakdown of Past Assessments

Here is an example of how past assessments can be structured and analyzed:

Topic	Frequency in Past Assessments	Average Difficulty Level
Derivatives	30%	Medium
Integrals	40%	High
Limits	15%	Low
Series	15%	High

By reviewing this breakdown, you can see which topics appear most frequently and where to focus your efforts for optimal preparation.

Practice Tools and Resources Available

Access to the right tools and resources is essential for effective preparation. With a wide range of materials available, you can enhance your understanding of complex concepts and refine your problem-solving skills. These resources not only provide practice questions but also offer explanations and solutions to help you learn from your mistakes and improve your performance.

Online Platforms are one of the most convenient ways to access practice materials. Many websites offer interactive problems, step-by-step solutions, and practice tests designed to simulate real assessment conditions. These platforms often track your progress, allowing you to focus on areas where you need the most improvement.

• Math Websites: Websites like Khan Academy, Brilliant, and Coursera provide structured lessons and exercises that align with the topics covered in advanced mathematics.
• Interactive Problem Solvers: Tools like WolframAlpha can help you understand complex solutions and verify your answers.

Books and Study Guides are also valuable resources. Many publishers offer comprehensive guides filled with practice questions, detailed explanations, and tips for solving problems efficiently. These resources are ideal for those who prefer working through physical materials rather than digital ones.

• Textbooks: Standard textbooks often have chapter reviews and practice problems that can help reinforce key concepts.
• Study Guides: Dedicated review books typically provide targeted practice sets along with explanations tailored to specific topics and question types.

Mobile Apps offer on-the-go practice, allowing you to study anywhere. These apps often feature timed quizzes, flashcards, and video explanations, making it easy to fit in short study sessions throughout the day.

• Flashcard Apps: Tools like Quizlet help you review key concepts and definitions quickly and efficiently.
• Quiz Apps: Apps designed for timed quizzes simulate real test conditions, helping you practice under pressure.

By utilizing these diverse tools and resources, you can tailor your preparation to your own learning style, ensuring you are well-equipped for the challenge ahead.

Common Mistakes to Avoid During Practice

While preparing for advanced mathematics assessments, it’s easy to fall into certain habits that can hinder your progress. These mistakes often stem from a lack of attention to detail or improper study techniques. Recognizing and addressing these common errors early on will help ensure a more efficient and effective study routine.

Rushing Through Problems is one of the most common mistakes students make. While it’s important to practice under time constraints, taking shortcuts or failing to fully understand each step can lead to careless errors. Make sure to focus on quality over speed, especially when learning new concepts.

Neglecting to Show Work is another frequent issue. Many students try to skip the process of showing each step in their solution, assuming they can just remember the correct method. However, showing your work is critical not only for clarity but also for reinforcing your understanding of the concepts.

Other Mistakes to Avoid

Mistake	Why It Happens	How to Avoid It
Skipping Review	Students often move on to new material without reviewing past lessons.	Regularly revisit older topics to reinforce your understanding and prevent knowledge gaps.
Not Asking for Help	Students hesitate to ask for clarification when they don’t understand a concept.	Seek assistance from teachers, tutors, or peers when struggling with difficult material.
Focusing Too Much on One Topic	Focusing too heavily on one area and neglecting others can create an unbalanced understanding.	Make sure to allocate study time across all topics to ensure balanced preparation.
Overloading with Practice	Trying to cram too much material in one session leads to burnout and ineffective learning.	Break study sessions into manageable chunks, focusing on quality rather than quantity.

Avoiding these mistakes will help you stay on track and make the most of your study time, leading to better results in your assessments. Remember, effective preparation is about building solid habits and understanding, not just memorizing answers.

Setting Realistic Study Goals

Establishing clear and attainable goals is essential for effective preparation. By setting realistic expectations for yourself, you can stay focused and motivated throughout your study sessions. Goals should be specific, measurable, and achievable, allowing you to track your progress and adjust as needed. A well-defined plan will guide you toward success without feeling overwhelmed.

Steps for Setting Achievable Goals

To create meaningful study goals, follow these steps:

• Break Down Large Tasks: Instead of focusing on broad topics, break them into smaller, manageable sections. This makes the study process less intimidating and allows for more frequent accomplishments.
• Set Time-Based Milestones: Assign deadlines to each goal. For instance, set a specific date to complete a chapter or master a particular concept.
• Balance Your Study Sessions: Allocate time for both review and new material. Avoid overwhelming yourself by focusing solely on difficult areas–include lighter topics to maintain balance.

Maintaining Motivation and Adjusting Goals

Staying motivated is crucial when working towards long-term study goals. It’s important to be flexible and adjust your objectives if needed. Here are some tips to maintain momentum:

1. Track Your Progress: Keep a record of your achievements. Regularly review what you’ve completed to stay motivated and proud of your progress.
2. Celebrate Small Wins: Acknowledge every milestone, no matter how small. This helps you stay positive and engaged throughout your study journey.
3. Be Flexible: If you find a certain goal too challenging or unrealistic, don’t be afraid to revise it. Adaptability is key to long-term success.

By setting realistic and well-defined goals, you create a structured approach to your preparation that will guide you toward success. Keep your objectives clear, monitor your progress, and stay motivated to achieve your desired results.

How to Review Your Practice Tests

Reviewing your completed assessments is a crucial step in the learning process. It allows you to identify strengths and weaknesses, improve problem-solving techniques, and reinforce the material you have studied. Effective review goes beyond just looking at the correct answers; it involves understanding why you made certain mistakes and how to avoid them in the future.

Steps for an Effective Review

Analyze Mistakes: Start by focusing on the questions you got wrong. Don’t just move on–take the time to carefully go over each mistake. Ask yourself what went wrong and why. Was it a misunderstanding of the concept, a simple calculation error, or a misinterpretation of the question?

Understand the Correct Solution: Once you’ve identified the errors, work through the correct solution step by step. Ensure that you understand each part of the problem and why the correct approach works. This will reinforce your knowledge and prevent similar mistakes in the future.

Additional Tips for Reviewing

• Review Solutions from Different Sources: If you’re struggling with a particular question, look at different explanations or approaches. Different perspectives can help clarify complex concepts.
• Re-do the Problems: After thoroughly understanding the correct solution, try solving the same problems again without looking at the answers. This will help reinforce your learning and improve retention.
• Track Patterns: Keep track of recurring mistakes or areas where you consistently struggle. This will allow you to prioritize these topics in future study sessions.

By carefully reviewing your completed tests, you turn mistakes into valuable learning opportunities. This reflective process helps you fine-tune your understanding and approach, ultimately leading to better performance in future assessments.

Importance of Consistent Study Habits

Maintaining a steady and reliable study routine is crucial for mastering complex subjects. Consistency in your study habits helps you build long-term retention, reinforces key concepts, and minimizes the stress of last-minute cramming. By dedicating regular time for focused study, you can steadily improve your understanding and increase your confidence over time.

Benefits of a Consistent Routine

• Improved Retention: Regular study sessions help to embed information in your long-term memory, making it easier to recall when needed.
• Better Time Management: Establishing a routine allows you to allocate specific time slots for each topic, ensuring comprehensive coverage without feeling rushed.
• Reduced Anxiety: Spacing out your study time reduces the pressure of needing to learn everything at once, leading to lower stress levels.

How to Develop Consistent Study Habits

Creating and sticking to a study schedule is essential. Here are a few tips for building consistent habits:

1. Set Specific Goals: Break down your study objectives into manageable tasks, such as mastering a specific topic or completing a set of problems. This keeps you focused and organized.
2. Create a Study Schedule: Plan your study sessions in advance, ensuring you allocate enough time to review all necessary materials. Consistency is key–try to study at the same time each day.
3. Stay Organized: Keep track of your progress with a study journal or digital planner. This helps you stay on track and provides motivation when you see how much you’ve accomplished.
4. Limit Distractions: During study sessions, minimize distractions by turning off notifications, finding a quiet place, and focusing entirely on the task at hand.

Consistency in your study routine not only improves your understanding but also fosters discipline and time management skills. By committing to regular, focused sessions, you set yourself up for success in the long run.

How to Build Conceptual Understanding

Developing a strong grasp of the underlying concepts is crucial for success in any challenging subject. Understanding the “why” behind mathematical principles, rather than just memorizing formulas or procedures, allows you to apply knowledge flexibly and solve complex problems more effectively. This deeper understanding makes it easier to connect different ideas and improve overall problem-solving skills.

Approach to Building Strong Concepts

Start with Fundamentals: Begin by mastering the basic principles before moving on to more complex topics. A solid foundation allows you to approach advanced problems with confidence and clarity.

Visualize the Concepts: Visual tools, such as graphs, diagrams, and charts, can help you see the relationships between different ideas. Visualizing a problem often provides insights that are harder to grasp through text alone.

Effective Techniques for Conceptual Mastery

• Ask Questions: Whenever you encounter a new concept, ask yourself how it fits into the larger picture. What is its purpose? How does it relate to what you already know?
• Work Through Examples: Apply the concept to a variety of problems to see how it works in different contexts. Real-world applications can help you understand its relevance and deepen your understanding.
• Teach What You Learn: Explaining concepts to others is a powerful way to reinforce your own understanding. When you teach, you’re forced to clarify your thoughts and identify any gaps in your knowledge.

By focusing on conceptual understanding rather than rote memorization, you gain the flexibility to solve problems in a variety of ways and adapt to new challenges. Developing this skill allows you to approach future topics with greater ease and confidence.

Mastering Derivatives and Integrals

Understanding the core concepts of differentiation and integration is essential for mastering advanced mathematics. These two fundamental operations are the building blocks for solving many complex problems, whether in real-world applications or theoretical exercises. By fully grasping the processes involved, you can tackle a wide variety of problems with confidence and precision.

Understanding Derivatives

Derivatives represent the rate of change of a function and are crucial for analyzing how quantities change over time or under varying conditions. To master differentiation, it’s essential to understand the rules and methods that govern how functions behave. The power rule, product rule, quotient rule, and chain rule are key techniques that make differentiation manageable and intuitive.

• Power Rule: Use this rule to differentiate functions of the form x^n.
• Product and Quotient Rules: These rules are used when differentiating products or quotients of functions, allowing for more complex expressions.
• Chain Rule: This rule is essential for differentiating composite functions, or functions within functions.

Understanding Integrals

Integration, the reverse of differentiation, allows you to compute the total accumulation of a quantity, such as area under a curve. By mastering integration techniques, you can solve problems involving areas, volumes, and other cumulative quantities. Key integration techniques include substitution, integration by parts, and partial fractions.

• Substitution: This method simplifies the integral by substituting a part of the function with a simpler variable.
• Integration by Parts: Use this technique when the integrand is a product of two functions.
• Partial Fractions: Decompose rational functions into simpler fractions to make integration more straightforward.

Mastering both differentiation and integration techniques enables you to solve a wide range of problems, from finding instantaneous rates of change to calculating total quantities. With practice and understanding, these concepts become indispensable tools for advanced problem-solving.

Practice with Online Platforms

In the digital age, many online platforms offer a wealth of resources to help students strengthen their understanding of advanced mathematical concepts. These platforms provide a variety of interactive tools, exercises, and tutorials that can be accessed at any time. Leveraging these online resources can significantly enhance your learning experience, allowing for targeted practice and immediate feedback.

Interactive Exercises and Tutorials

Many websites and apps feature interactive exercises that simulate real-world problems. These platforms often offer step-by-step solutions and allow users to work through problems at their own pace. Some of the key features you can expect from these platforms include:

• Instant Feedback: Immediate explanations help identify mistakes and offer corrective strategies.
• Varied Problem Sets: A wide range of exercises, from simple to complex, ensures comprehensive coverage of essential topics.
• Interactive Graphs: Visual tools allow students to manipulate equations and functions, improving conceptual understanding.

Personalized Learning Paths

Some platforms tailor their content to meet individual learning needs by offering personalized practice schedules based on performance. These adaptive systems monitor progress and suggest areas for improvement. Benefits include:

• Targeted Review: Focus on specific areas where you need the most improvement.
• Progress Tracking: Track your improvements over time with detailed performance analytics.
• Flexible Learning: Access lessons and exercises that fit your schedule and learning style.

Using online platforms can complement your studying by providing both structure and flexibility. These tools are invaluable for reinforcing concepts and improving problem-solving skills, ensuring you’re well-prepared for any challenges ahead.

Test-Day Tips and Mental Preparation

The day of an important assessment can be stressful, but with the right mindset and preparation, you can perform at your best. Proper mental preparation, along with a few key strategies, can help you manage anxiety and stay focused during the test. By following a few simple steps, you can maximize your performance and boost your confidence as you approach each section of the evaluation.

Stay Calm and Focused

Maintaining a calm and focused mindset is crucial when facing any challenging assessment. Try the following tips to manage stress and keep your attention sharp:

• Practice Deep Breathing: Take deep, slow breaths to relax and clear your mind before entering the testing environment.
• Visualize Success: Imagine yourself successfully solving problems, which can help boost your confidence and reduce anxiety.
• Stay Positive: A positive mindset can improve your concentration and problem-solving abilities during the test.

Time Management and Test Strategy

Effective time management is essential for completing each section efficiently. Consider the following strategies for managing your time and prioritizing tasks:

• Read Instructions Carefully: Before starting each section, take a few moments to read the instructions thoroughly to avoid mistakes.
• Start with Easier Questions: Begin with the questions you feel most confident about to build momentum and save time for more challenging ones.
• Keep Track of Time: Periodically check the clock to ensure you are staying on track and not spending too much time on any one problem.

By preparing mentally and managing your time effectively, you’ll be in a strong position to tackle the assessment with confidence and achieve your best results.