Algebra 2 1.1 Answers and Step by Step Solutions

Understanding the foundational principles of advanced mathematics is crucial for solving complex problems. This section focuses on enhancing problem-solving abilities by guiding you through essential techniques and methods that will sharpen your skills. Whether you’re tackling equations or interpreting functions, the goal is to simplify the process and ensure a deeper grasp of the material.

By breaking down each step carefully, you’ll learn how to approach various mathematical challenges with confidence. The focus is on building a strong base of knowledge that can be applied to more advanced topics, ensuring steady progress. In this guide, we’ll emphasize logical reasoning, critical thinking, and practical strategies for handling various types of problems effectively.

Algebra 2 1.1 Answers

This section is dedicated to providing comprehensive solutions and detailed steps for tackling mathematical challenges commonly encountered in early-stage courses. It offers clear, concise explanations to help you navigate through various problem types with confidence. The focus is on helping you understand how to approach and solve different problems systematically.

By following the outlined steps, you will be able to break down complex problems into smaller, more manageable parts. The methodical approach ensures that key concepts are fully understood, allowing you to apply them effectively in future exercises.

Here’s a breakdown of common problem types you will encounter:

• Solving linear equations and systems
• Identifying and graphing functions
• Working with inequalities and absolute values
• Simplifying algebraic expressions
• Factoring and expanding binomials

Each of these topics requires specific strategies to achieve the correct solution. By practicing regularly, you will become more adept at recognizing patterns and applying the right techniques in various scenarios.

To ensure a strong grasp of the material, it is important to work through examples, review steps, and understand the reasoning behind each solution. With continued effort and focus, solving increasingly challenging problems will become second nature.

Understanding the Key Concepts

Grasping the fundamental principles of mathematics is essential for solving problems effectively. In this section, we will explore the core ideas that serve as the foundation for more advanced topics. By mastering these concepts, you’ll gain the confidence to approach any problem with a systematic mindset.

It’s important to focus on the basic building blocks, such as understanding variables, equations, and functions. Each concept builds upon the previous one, creating a structured framework that will guide you through more complex scenarios.

Below is a table summarizing some of the key concepts you’ll encounter:

Concept	Description	Example
Variables	Symbols that represent unknown values in equations.	x + 2 = 5
Equations	Mathematical statements that assert the equality of two expressions.	3x – 4 = 8
Functions	Relationships between two sets where each input has one output.	f(x) = 2x + 3
Factoring	Breaking down expressions into simpler components.	x² – 9 = (x – 3)(x + 3)

Each of these concepts plays a crucial role in solving more advanced problems. By understanding their underlying principles, you will be able to apply them in a variety of mathematical situations, ensuring a solid foundation for future learning.

Step by Step Problem Solving

Breaking down complex problems into manageable steps is a key strategy for mastering any mathematical challenge. By following a structured approach, you can tackle even the most difficult problems with ease. This method not only makes the process more understandable but also helps ensure accuracy at every stage.

Each problem can be dissected into smaller tasks. Start by identifying the variables and the relationship between them. From there, you can apply appropriate methods, whether it’s simplifying expressions, isolating variables, or solving equations. The goal is to stay organized and focused on one step at a time.

For example, when solving an equation, begin by eliminating any constants or terms that are not part of the variable expression. Next, apply the necessary operations to isolate the variable. As you proceed, check your work to confirm that each step brings you closer to the solution.

Steps to Follow:

1. Identify the key elements – Understand the given information and what is being asked.
2. Simplify the equation – Eliminate unnecessary terms and combine like terms where possible.
3. Isolate the variable – Use inverse operations to get the variable on one side of the equation.
4. Check your work – After finding a solution, plug it back into the original equation to ensure it’s correct.

By practicing this step-by-step approach, you will develop a methodical way to solve problems and improve your problem-solving efficiency in various mathematical scenarios.

Common Mistakes in Algebra 2

Even experienced learners can fall into common traps when solving mathematical problems. Recognizing these frequent errors is crucial for improving accuracy and efficiency. By understanding where mistakes typically occur, you can avoid them and solve problems more confidently.

Many mistakes arise from overlooking the order of operations, misinterpreting expressions, or incorrectly applying formulas. These errors can lead to incorrect solutions, and understanding their causes can help in correcting them quickly. Below are some of the most common mistakes students make.

Misunderstanding the Order of Operations

One of the most frequent issues is failing to follow the proper order of operations, which is essential for solving equations correctly. This involves working with parentheses, exponents, multiplication, division, addition, and subtraction in the correct sequence. Ignoring this order can lead to incorrect results.

Incorrectly Simplifying Expressions

Simplifying expressions improperly is another common mistake. This includes adding or subtracting terms that shouldn’t be combined, or forgetting to distribute terms correctly. Such errors often happen when working with polynomials or rational expressions, leading to faulty solutions.

By being mindful of these common pitfalls and double-checking your work, you can significantly improve your problem-solving skills and avoid mistakes in future problems.

Tips for Mastering Algebra 2

Achieving proficiency in advanced mathematics requires practice, patience, and the right strategies. Mastery comes from consistently applying techniques, understanding underlying principles, and refining problem-solving methods. In this section, we’ll explore effective strategies to help you gain confidence and excel in more complex topics.

Practice Regularly and Consistently

The key to mastering any mathematical subject is consistent practice. Regularly working through problems helps reinforce concepts and improves your ability to recognize patterns. The more problems you solve, the more comfortable you become with applying various methods. Set aside time each day to practice, even if it’s just for a short period, to maintain steady progress.

Focus on Conceptual Understanding

While memorization of formulas and methods is important, understanding the reasoning behind them is crucial. Take time to grasp why certain techniques work and how they are connected to one another. This deeper understanding will help you apply the right strategy in different scenarios and make it easier to tackle more challenging problems.

By integrating these tips into your study routine, you’ll build a solid foundation and approach advanced topics with greater ease and confidence.

How to Approach Word Problems

Word problems can often feel overwhelming, but breaking them down into smaller steps makes them more manageable. The key is to carefully analyze the information provided and translate it into mathematical terms. By following a structured approach, you can approach any word problem with confidence and clarity.

Step-by-Step Strategy for Solving Word Problems

Here’s a clear method for solving word problems effectively:

1. Read the problem carefully – Understand what is being asked and identify the key information.
2. Highlight important details – Look for numbers, units, and relationships between variables.
3. Define variables – Assign letters to unknown quantities for easier manipulation.
4. Translate the problem – Convert the words into an equation or expression based on the relationships described.
5. Solve the equation – Use appropriate mathematical methods to find the solution.
6. Check the result – Ensure the solution makes sense in the context of the problem.

Common Pitfalls to Avoid

• Misinterpreting the question – Always ensure you understand what is being asked before solving.
• Forgetting units – Pay attention to units of measurement and convert them if necessary.
• Skipping steps – Don’t rush the process. Take time to organize and check your work.

By following these steps and avoiding common mistakes, you can confidently approach and solve word problems, transforming challenges into opportunities for learning.

Using Graphs in Algebra 2

Graphs are a powerful tool for visualizing relationships between variables and understanding complex mathematical concepts. By plotting equations and functions, you can see how changes in one variable affect another, making it easier to analyze and interpret data. In this section, we will explore how to effectively use graphs to solve problems and gain insights into mathematical situations.

Graphing provides a visual representation of an equation, making abstract concepts more tangible. It allows you to quickly identify key features such as intercepts, slopes, and symmetry. This approach is particularly helpful when working with linear and quadratic relationships, as well as more advanced functions.

Key Steps to Using Graphs Effectively:

• Plot key points: Start by plotting the intercepts and any other known points on the graph.
• Draw the curve: Connect the points in a smooth curve, paying attention to the shape of the graph.
• Analyze the graph: Look for important features such as the slope, concavity, and behavior at the edges.
• Use graphing tools: Utilize graphing calculators or software to check your work and explore more complex functions.

By regularly practicing graphing techniques, you will strengthen your ability to visualize and solve problems, making complex topics more accessible and easier to understand.

Breaking Down Complex Equations

Solving intricate equations can seem intimidating at first, but by breaking them down into smaller, more manageable parts, you can simplify the process significantly. Understanding the structure of an equation and methodically isolating variables is key to solving it efficiently. This approach allows you to work through each step logically and reach the correct solution.

Step-by-Step Approach to Solving Complex Equations

The first step in solving a complex equation is to identify the different components, such as constants, variables, and operators. Once you’ve broken the equation into its basic parts, you can apply systematic operations to simplify it.

• Distribute terms: If necessary, apply the distributive property to expand any parentheses.
• Combine like terms: Group terms with the same variables or constants to reduce the complexity of the equation.
• Isolate the variable: Use inverse operations to move terms around and isolate the variable you are solving for.
• Solve for the unknown: After isolating the variable, perform the final steps to find its value.

Common Strategies for Tackling Difficult Equations

When facing particularly challenging equations, it’s helpful to apply strategies such as factoring, using the quadratic formula, or substituting known values to reduce the equation’s complexity. These methods can make even the most complicated equations easier to handle and lead to more straightforward solutions.

By consistently practicing these strategies, you will become more confident in tackling complex equations and solving them effectively, step by step.

Important Formulas to Remember

In mathematics, certain formulas serve as essential tools for solving problems across various topics. These formulas not only provide shortcuts for calculations but also help establish connections between different concepts. Mastering these key formulas is critical for efficiently solving equations and understanding more complex principles.

Essential Formulas to Keep in Mind:

• Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a – Used for solving quadratic equations of the form ax² + bx + c = 0.
• Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) – Helps find the slope of a line given two points (x₁, y₁) and (x₂, y₂).
• Pythagorean Theorem: a² + b² = c² – Applies to right-angled triangles, where a and b are the legs, and c is the hypotenuse.
• Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²) – Used to find the distance between two points in a coordinate plane.
• Exponent Laws: a^m × a^n = a^(m+n), (a^m)^n = a^(m×n) – Help simplify expressions with exponents.

Familiarizing yourself with these key formulas and understanding when to apply them will significantly enhance your ability to solve problems quickly and accurately. Regular practice is the best way to ensure these formulas become second nature.

Exploring the Quadratic Formula

The quadratic formula is a powerful tool used to solve quadratic equations, which are equations involving a variable raised to the second power. This formula provides a straightforward way to find the solutions, or roots, of any quadratic equation, even when factoring is difficult or impossible. Understanding the formula’s structure and how to apply it is essential for solving these types of problems efficiently.

Understanding the Formula

The general form of a quadratic equation is ax² + bx + c = 0, where a, b, and c are constants. The quadratic formula is given by:

x = (-b ± √(b² – 4ac)) / 2a

By substituting the values of a, b, and c from the equation into this formula, you can quickly calculate the solutions for x. The symbol ± indicates that there may be two possible solutions, depending on whether you add or subtract the value inside the square root.

Key Considerations When Using the Formula

• Discriminant: The expression under the square root, b² – 4ac, is known as the discriminant. It determines the nature of the solutions:
• If the discriminant is positive, there are two real and distinct solutions.
• If the discriminant is zero, there is one real solution.
• If the discriminant is negative, there are no real solutions, only complex ones.
• Simplification: Always simplify the expression under the square root and the final result, if possible, to make the answer clearer.
• Multiple Solutions: Remember, the ± sign means there are two possible solutions, so be sure to calculate both.

By mastering the quadratic formula, you can easily solve any quadratic equation, even those that seem complicated at first glance.

Factoring Techniques for Beginners

Factoring is a key skill in solving various types of equations, especially when dealing with polynomials. It involves rewriting an expression as a product of its simpler components, making it easier to solve or simplify. For beginners, mastering the basic techniques of factoring is essential to building a strong foundation in mathematics. Understanding these methods allows for more efficient problem-solving and prepares you for more complex concepts.

The most common factoring techniques include identifying greatest common factors, factoring trinomials, and recognizing special patterns such as difference of squares and perfect square trinomials. Each of these methods can be applied to specific types of expressions, and with practice, you’ll be able to recognize when and how to use them effectively.

As you practice these techniques, remember that factoring often requires patience and persistence. The more problems you solve, the more intuitive the process will become, and you’ll soon develop a better understanding of how to approach more complicated expressions with confidence.

Solving Linear Systems Efficiently

When solving systems of equations, the goal is to find values for the variables that satisfy all the equations in the system simultaneously. These systems can range from simple to complex, but mastering efficient methods is key to quickly finding solutions. Whether you are working with two or more equations, using the right technique can make the process much smoother and more manageable.

Common Methods for Solving

There are several standard methods for solving linear systems, each with its strengths. The most commonly used are:

• Graphical Method: Plotting both equations on a graph and identifying the point of intersection. While visual, this method can be imprecise without accurate graphing tools.
• Substitution Method: Solving one equation for one variable and substituting that expression into the other equation. This method is effective when one of the equations is easily solvable for a specific variable.
• Elimination Method: Adding or subtracting equations to eliminate one variable. This method is particularly useful when both equations are in a form that allows easy cancellation of a variable.

Tips for Efficiency

• Choose the Right Method: Based on the given system, pick the most straightforward method. For example, if one equation is easily solved for a variable, substitution is quick. If the coefficients are easily manipulated, elimination may be faster.
• Check for Special Cases: Be on the lookout for systems with no solution (inconsistent) or infinite solutions (dependent). Recognizing these cases early can save time and prevent unnecessary steps.
• Practice Mental Math: Being comfortable with basic arithmetic can speed up the process, especially when working with large systems or coefficients.

By using these techniques and strategies, you can efficiently solve linear systems with accuracy and speed, ensuring you can tackle even the most challenging problems with confidence.

Identifying Functions and Their Graphs

Understanding how to identify functions and interpret their graphs is an essential skill in mathematics. A function is a relationship between two sets of numbers, where each input is associated with exactly one output. Recognizing functions and their behaviors on a graph allows you to analyze and solve problems more effectively. Graphs visually represent functions and provide insight into how the variables interact, making it easier to understand complex relationships.

To identify a function, there are several key characteristics to consider. First, check if every input (usually represented on the x-axis) corresponds to exactly one output (usually on the y-axis). A common way to test this is through the “vertical line test,” which states that if a vertical line intersects the graph at more than one point, the graph does not represent a function.

Types of Functions and Their Graphs

Different types of functions have distinct graph shapes. Below is a table summarizing common functions and their graph characteristics:

Function Type	Graph Shape	Example
Linear	Straight line	y = 2x + 3
Quadratic	Parabola	y = x² – 4
Cubic	Curved line with a single inflection point	y = x³
Absolute Value	V-shaped graph	y = |x|
Exponential	Rapidly increasing or decreasing curve	y = 2^x

By understanding these basic functions and their corresponding graphs, you can begin to recognize patterns in more complex problems and develop a deeper understanding of mathematical relationships. Identifying functions and their graphs is a fundamental skill that forms the foundation for more advanced topics in mathematics.

Strategies for Simplifying Expressions

Simplifying mathematical expressions is an essential skill that helps reduce complexity and makes problems easier to solve. By using a variety of techniques, you can rewrite complex expressions in a more manageable form. These strategies can help identify common patterns, eliminate unnecessary terms, and make calculations more efficient. Below are several key approaches to simplifying expressions effectively.

Key Techniques for Simplification

There are several strategies that you can use when simplifying expressions. These techniques are foundational in making calculations more straightforward:

• Combining Like Terms: Group terms that have the same variable and exponent. This will reduce the expression to fewer terms, making it simpler to work with.
• Distributive Property: Apply the distributive property to eliminate parentheses and multiply terms inside them.
• Factoring: Factor expressions whenever possible to break them down into simpler components. This technique can often reveal hidden patterns and simplify the overall expression.
• Rationalizing Denominators: If an expression contains a fraction with a square root in the denominator, multiply both the numerator and denominator by the square root to simplify.

Example of Simplifying an Expression

Consider the expression 3x + 5x – 4 + 2. By combining like terms, we can simplify it as follows:

• Combine the x terms: 3x + 5x = 8x
• Combine the constants: -4 + 2 = -2

The simplified expression is: 8x – 2.

By applying these strategies, you can efficiently simplify a wide range of mathematical expressions and approach problems with more clarity and confidence. Mastering these techniques will also help with more advanced topics in mathematics.

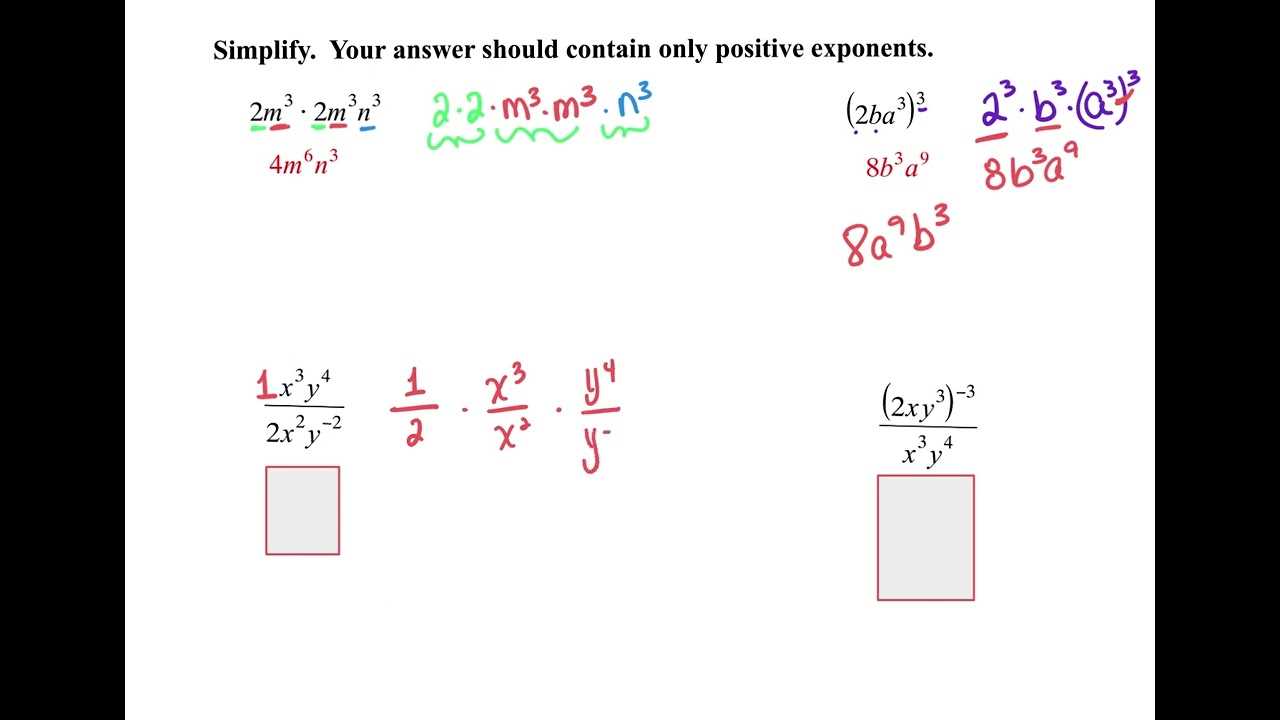
How to Handle Exponents and Roots

Understanding how to work with powers and roots is a fundamental aspect of simplifying and solving mathematical problems. Exponents represent repeated multiplication, while roots are essentially the opposite operation, helping to undo the effect of an exponent. By mastering the rules and properties of exponents and roots, you can solve a wide range of problems with ease. Below, we’ll explore key principles for handling these operations effectively.

Rules for Working with Exponents

When working with exponents, there are several important rules to keep in mind:

• Product of Powers Rule: When multiplying two expressions with the same base, add their exponents. For example, a^m × aⁿ = a^m+n.
• Quotient of Powers Rule: When dividing expressions with the same base, subtract the exponents. For example, a^m ÷ aⁿ = a^m-n.
• Power of a Power Rule: When raising an exponent to another exponent, multiply the exponents. For example, (a^m)ⁿ = a^m×n.
• Negative Exponent Rule: A negative exponent means the reciprocal of the base raised to the opposite positive exponent. For example, a^-n = 1/aⁿ.
• Zero Exponent Rule: Any nonzero number raised to the power of zero equals 1. For example, a⁰ = 1.

Understanding and Simplifying Roots

Roots, such as square roots and cube roots, can also be simplified using certain rules. Here’s a quick guide on how to handle them:

• Square Roots: The square root of a number is the value that, when multiplied by itself, equals the original number. For example, √16 = 4.
• Cube Roots: The cube root of a number is the value that, when multiplied by itself three times, equals the original number. For example, ∛27 = 3.
• Rationalizing the Denominator: If a fraction has a square root or cube root in the denominator, multiply both the numerator and denominator by the necessary factor to remove the root from the denominator.

By mastering these rules, you can simplify complex expressions and equations involving exponents and roots, making them more manageable and easier to solve.

Applications of Mathematical Concepts in Real Life

Mathematical concepts extend far beyond the classroom, providing valuable tools for solving everyday problems. These principles are essential for understanding patterns, making decisions, and predicting outcomes in various fields. From budgeting to engineering, the ability to apply mathematical reasoning in real-life situations can lead to more informed and effective solutions. Below are some practical ways in which mathematical techniques are used in daily life.

Budgeting and Personal Finance

Managing personal finances requires understanding how to handle different types of calculations, such as interest rates, savings growth, and expenses. Mathematical models help individuals track their spending, set financial goals, and make wise decisions about their investments. Some examples include:

• Compound Interest: Calculating how money grows over time when interest is earned on both the principal and the accumulated interest.
• Loan Repayments: Determining how long it will take to pay off a loan or the impact of different interest rates on total repayment amounts.
• Budget Planning: Creating a monthly budget by categorizing income and expenses and adjusting amounts to meet financial goals.

Engineering and Construction

In fields like construction, architecture, and civil engineering, mathematical models and calculations are used to design structures and systems that are safe, efficient, and sustainable. Here are a few key areas where mathematics is applied:

• Structural Design: Engineers use equations to determine the strength and stability of materials and how they will behave under various loads.
• Project Scheduling: Mathematical techniques help estimate the time required to complete projects, taking into account factors like resources and deadlines.
• Cost Estimation: Calculations help predict the cost of construction materials, labor, and equipment needed for large projects.

By applying mathematical reasoning, professionals in these fields can ensure that projects are both cost-effective and technically sound. From everyday budgeting to complex engineering tasks, mathematical thinking is an indispensable tool in making informed decisions.

Reviewing Practice Problems for Mastery

Consistent practice is essential for mastering complex concepts. To truly internalize the material, it’s important to regularly revisit and work through a variety of problems. This approach not only strengthens understanding but also builds confidence when tackling new challenges. By reviewing and solving problems systematically, individuals can identify areas that need improvement and reinforce their knowledge effectively. Below are some strategies for approaching practice exercises to ensure mastery.

Strategies for Effective Practice

Approaching practice problems with a focused mindset is crucial. Here are some methods to maximize the effectiveness of your review sessions:

• Break Down Problems: Start by carefully analyzing each problem, identifying key information and what is being asked.
• Work Through Step-by-Step: Break the problem into manageable steps, and don’t skip any parts of the process. Each step builds towards the solution.
• Review Solutions: After solving a problem, check the solution and understand any mistakes made. This step helps prevent repeating errors in the future.

Tracking Progress with Problem Sets

Tracking your progress over time is an important aspect of mastering any skill. Using a variety of problem sets allows for practice in different contexts, helping to reinforce concepts and uncover any persistent challenges. The following table illustrates a sample problem set that covers different areas of focus:

Topic	Problem Type	Difficulty Level
Equations	Solve linear equations	Easy
Systems of Equations	Solve using substitution	Medium
Exponents	Simplify expressions with exponents	Hard
Quadratic Functions	Factor quadratic expressions	Hard

By regularly reviewing and solving problems across different levels of difficulty, you can gradually master more complex topics and improve problem-solving skills. Each successful solution builds on previous understanding, leading to greater confidence and a stronger foundation in the subject.