Honors Algebra 2 Final Exam Answers and Study Guide

As you prepare for the culmination of your mathematical studies, it’s crucial to solidify your understanding of key concepts and problem-solving techniques. The journey through this challenging subject requires not only practice but also a strategic approach to mastering complex topics. Whether it’s tackling equations, working with functions, or interpreting graphs, the final phase demands a thorough grasp of the material.

Throughout this guide, we’ll explore various methods to approach the test, offering valuable insights into common pitfalls and effective strategies. By practicing a wide range of problems and familiarizing yourself with the structure of questions, you’ll be well-equipped to perform at your best. Focused revision, combined with a solid understanding of foundational principles, will help you tackle even the most difficult problems with confidence.

Prepare yourself to confidently approach each challenge by developing both your skills and your test-taking mindset. This approach will help ensure that you’re ready for success when it matters most.

Mathematics Assessment Overview

This section provides an overview of the comprehensive evaluation designed to test your mastery of advanced mathematical concepts. The assessment covers a wide range of topics, evaluating both theoretical knowledge and practical application skills. It is structured to challenge your understanding of key mathematical principles while testing your ability to apply them in real-world scenarios.

Key Topics and Areas of Focus

The evaluation will include questions on various areas, such as complex equations, functions, graphing, and systems of equations. You will be expected to demonstrate proficiency in solving quadratic and polynomial expressions, as well as applying trigonometric identities and exponential functions. Expect to encounter both theoretical problems that test your understanding and practical problems that require logical reasoning to solve.

Preparing for the Challenge

Success in this evaluation depends on thorough preparation. Familiarizing yourself with the types of problems and practicing a variety of question formats will help ensure you’re ready. A strong understanding of both the theory and practical applications of these topics is essential. Focus on areas that may be more challenging and make sure to review key concepts that are often tested. With the right approach, you can tackle the assessment with confidence and clarity.

Key Topics Covered in the Assessment

To succeed in this assessment, it’s essential to focus on the core mathematical principles that are regularly tested. A strong foundation in the major topics ensures you’re prepared for any challenge that arises. This section highlights the key areas that will be assessed, providing insight into the material you need to master before tackling the test.

Equations and Expressions: Expect a variety of problems involving solving and manipulating different types of equations. These may include linear, quadratic, and polynomial expressions, as well as systems of equations that require precise solutions.

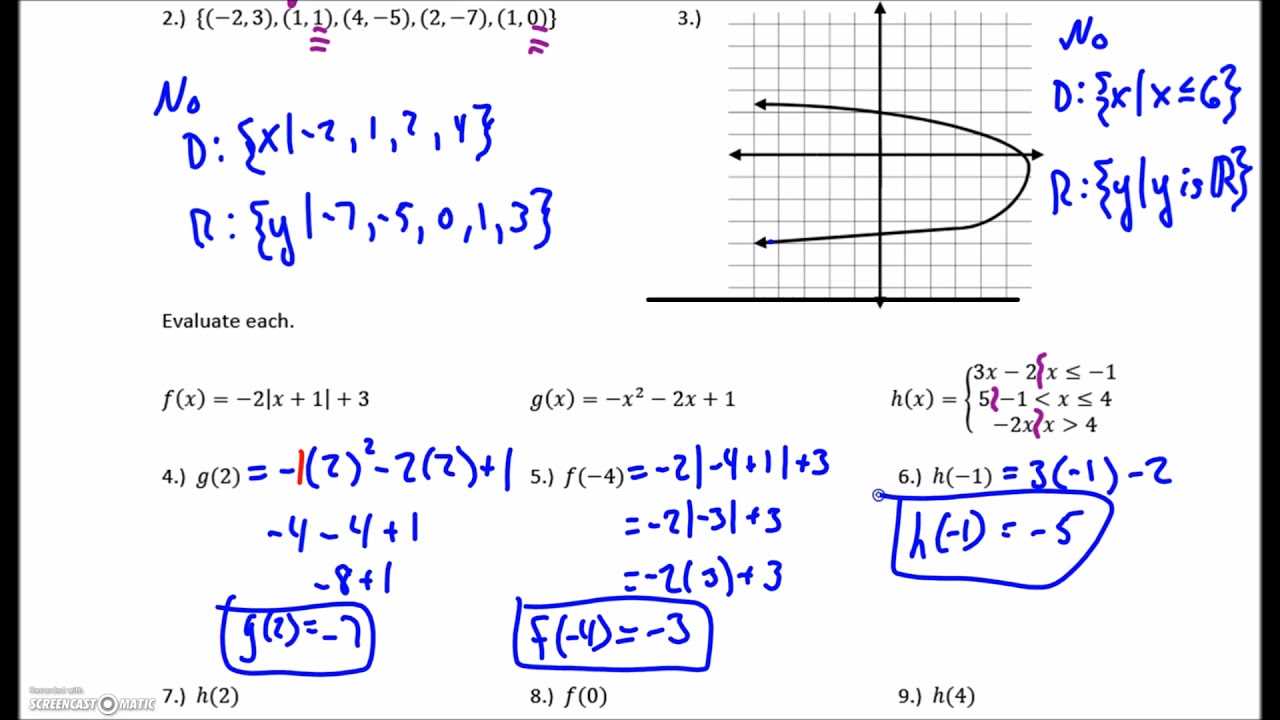
Functions and Graphing: Understanding the behavior of various functions, including exponential, logarithmic, and trigonometric, is crucial. You will also need to demonstrate your ability to graph these functions and interpret their meaning in both theoretical and applied contexts.

Complex Numbers and Systems: Proficiency in working with complex numbers, as well as solving systems of equations, is another critical area. Being able to manipulate both real and imaginary numbers will be essential for certain problem sets.

Probability and Statistics: Knowledge of statistical concepts, such as probability, mean, median, and standard deviation, will be important for solving data-related problems. You may also encounter questions on interpreting data sets and making predictions.

Trigonometry: A strong understanding of trigonometric functions, identities, and their applications will be necessary. Expect problems that involve the unit circle, solving for unknown angles, and applying trigonometric properties in real-world scenarios.

Understanding the Assessment Format

Familiarity with the structure of the evaluation is crucial for effective preparation. Knowing how questions are organized and the types of tasks you will face can significantly improve your approach. This section explains the layout and format of the assessment, helping you feel more confident when the time comes.

Types of Questions

The assessment will consist of various types of questions, each designed to test your knowledge in different ways. You can expect multiple-choice questions, where you select the correct answer from a list of options, as well as open-ended problems that require you to show your work and explain the steps leading to the solution. Additionally, there may be word problems that challenge your ability to apply mathematical concepts to real-world situations.

Time Management and Pacing

Effective time management is key to completing the assessment successfully. While each section has a set time limit, it’s important to pace yourself to ensure you can address all questions thoroughly. Prioritize questions based on their difficulty and the time needed to solve them. Some questions may require deeper thought, while others can be answered more quickly. Planning your approach ahead of time will help you avoid rushing through important tasks.

How to Approach Mathematical Questions

When facing challenging mathematical problems, having a structured approach can make a significant difference in your ability to solve them efficiently. The key is to break down each question into manageable steps and apply the appropriate strategies. This section outlines how to tackle different types of problems methodically to maximize your success.

Read the Problem Carefully: Begin by thoroughly understanding the question. Pay attention to key terms and values provided in the problem. Identify what is being asked before you start solving. Misinterpreting a question can lead to unnecessary errors.

Organize Your Work: Whether it’s solving equations, graphing functions, or working with data, always write down your steps clearly. Show your work in an organized manner, which not only helps avoid mistakes but also ensures that you can track your progress. It’s essential to keep equations and calculations in a logical order for clarity.

Use a Step-by-Step Process: When dealing with complex problems, break them down into smaller, simpler tasks. For instance, if you are solving for a variable, isolate it step by step rather than trying to jump to the solution. This method helps prevent confusion and increases accuracy.

Double-Check Your Work: After reaching an answer, always review your solution. Verify that each step logically follows the previous one and that no mistakes were made in the calculations. If possible, plug your solution back into the original problem to confirm it works as expected.

Effective Study Strategies for Success

Achieving success in any mathematical assessment requires more than just understanding concepts; it involves adopting the right study techniques. By organizing your preparation and staying consistent, you can improve retention, reduce anxiety, and ensure you’re fully prepared. This section provides valuable strategies to help you make the most of your study time.

• Create a Study Schedule: Organize your study time by breaking it into manageable blocks. Allocate specific time slots for each topic and stick to this plan consistently. This will help you avoid cramming and allow for in-depth review.
• Focus on Weak Areas: Identify the topics where you feel least confident and prioritize them. Spend extra time reviewing these concepts until they become second nature. This targeted approach will improve your overall performance.
• Practice Regularly: Consistent practice is essential for reinforcing your understanding. Work through problems daily, starting with basic examples and gradually progressing to more complex ones. The more you practice, the more familiar you’ll become with various question formats.
• Use Practice Tests: Simulate the test environment by taking timed practice tests. This will help you familiarize yourself with the pacing and structure of the problems. It also allows you to identify areas that need further review.
• Study in Groups: Collaborating with peers can enhance your learning experience. Discussing problems and explaining concepts to others strengthens your own understanding and provides new perspectives on challenging topics.

By adopting these strategies, you can develop a structured and focused study routine that will maximize your chances of success. Consistency, practice, and review are key to mastering the material and feeling confident when it matters most.

Common Mistakes to Avoid During the Assessment

Even with thorough preparation, it’s easy to make mistakes during a challenging test. Often, these errors stem from rushing through questions or overlooking key details. Being aware of the most common pitfalls can help you avoid them and improve your overall performance. This section highlights the mistakes you should watch out for while tackling problems.

• Skipping Important Steps: One of the most frequent errors is skipping intermediate steps in problem-solving. While it may seem quicker, omitting steps can lead to careless mistakes. Always show your work clearly and follow a logical process from start to finish.
• Misinterpreting the Question: Carefully read each problem before jumping to a solution. Many mistakes occur when students misinterpret what is being asked. Look for keywords and pay attention to units or conditions that affect the answer.
• Overcomplicating Simple Problems: It’s easy to overthink a question, especially when it’s simple on the surface. Take a moment to breathe and assess if the solution requires more steps than necessary. Sometimes the simplest approach is the most effective.
• Forgetting to Check Your Work: Leaving a problem without double-checking your calculations can lead to avoidable errors. Always take a few minutes at the end of the test to review your answers, especially for complex problems.
• Not Managing Time Wisely: Spending too much time on difficult questions and neglecting easier ones can be detrimental. Be mindful of the time limit and pace yourself to ensure you answer all questions.

By avoiding these common mistakes, you can approach the assessment with more confidence and accuracy. Staying calm, focused, and methodical will ensure you complete the test to the best of your ability.

Solving Quadratic Equations Efficiently

Quadratic equations are a fundamental part of many mathematical assessments. Solving them efficiently requires understanding the different methods available and knowing when to apply each one. This section explores the most effective techniques for solving quadratic problems quickly and accurately, helping you tackle them with confidence.

Methods for Solving Quadratic Equations

There are several methods for solving quadratic equations, each suitable for different situations. The three most commonly used approaches are factoring, using the quadratic formula, and completing the square. Understanding when to use each method can save you valuable time during an assessment.

Method	When to Use	Steps
Factoring	When the equation can be easily factored into two binomials.	1. Write the equation in standard form. 2. Factor the quadratic expression. 3. Set each factor equal to zero and solve.
Quadratic Formula	When the equation is difficult to factor or factoring is not possible.	1. Write the equation in standard form. 2. Apply the quadratic formula: x = (-b ± √(b² – 4ac)) / 2a. 3. Simplify the expression and solve for x.
Completing the Square	When the equation is not easily factorable, but a perfect square trinomial can be created.	1. Rewrite the equation in a form that allows you to complete the square. 2. Add the necessary constant to both sides. 3. Solve for x.

Choosing the Best Method

When deciding which method to use, consider the complexity of the equation and the specific requirements of the problem. Factoring is the quickest method when applicable, but if the equation cannot be factored easily, the quadratic formula is the best choice. Completing the square, while effective, can be more time-consuming and is typically used when other methods are not feasible.

By mastering these techniques and knowing when to apply each one, you can solve quadratic equations efficiently and accurately, ensuring that you complete your assessment on time and with confidence.

Tips for Working with Functions

Functions are a core concept in many areas of mathematics, and understanding how to work with them effectively is crucial for solving a wide range of problems. Whether you’re analyzing their behavior, graphing them, or solving related equations, having a solid grasp of function properties and strategies can make your work much more efficient. This section provides useful tips for mastering functions and ensuring accuracy when solving problems.

Understand Domain and Range: Before diving into problems, always identify the domain (the set of possible input values) and the range (the set of corresponding output values) for the function. This will help you avoid mistakes and ensure you’re working within the correct parameters.

Master Function Notation: Get comfortable with the notation used to represent functions. For example, f(x) is commonly used to represent a function, where “x” is the input variable. Understanding this language will make interpreting problems and solutions much easier.

Practice Graphing Functions: One of the most powerful ways to understand a function is to visualize it. Practice plotting different types of functions on a graph, paying attention to key features like intercepts, asymptotes, and overall shape. This will deepen your understanding of how the function behaves and help you identify important characteristics quickly during problem-solving.

Work with Function Operations: Functions can be combined using addition, subtraction, multiplication, and division. When performing these operations, remember to follow the rules of algebra while considering the domain of the resulting function. For example, division by zero is undefined, so you must account for any restrictions in the domain.

Practice Inverse Functions: The inverse of a function essentially reverses the input-output relationship. Practice finding the inverse of a function by swapping x and y and solving for y. This skill is particularly helpful when solving equations involving functions.

By focusing on these tips, you can approach function-related problems with greater confidence and precision. Understanding the core principles behind functions and practicing their application will lead to more successful problem-solving strategies in any assessment or real-world application.

Mastering Polynomials for the Test

Polynomials are a fundamental topic in mathematics and mastering them is essential for success in any assessment. Understanding how to manipulate and solve polynomial expressions will not only help you solve complex problems but will also save valuable time during the test. This section provides key strategies and tips to ensure you’re fully prepared to tackle polynomial-related questions with confidence.

Key Concepts to Understand

Before diving into solving problems, it is important to grasp the basic concepts related to polynomials. These include identifying terms, degrees, and leading coefficients, as well as understanding how polynomials behave in various operations.

• Recognizing Polynomial Terms: Each term in a polynomial is made up of a coefficient and a variable raised to a power. Practice identifying the highest degree term and the constant term, as these will often be key to solving problems.
• Adding and Subtracting Polynomials: When adding or subtracting polynomials, simply combine like terms. Always ensure that you group terms with the same degree and variable.
• Multiplying Polynomials: Use the distributive property (also known as the FOIL method for binomials) to multiply polynomials. Practice simplifying expressions by multiplying each term in one polynomial by each term in the other.

Strategies for Solving Polynomial Equations

When it comes to solving polynomial equations, different methods can be used depending on the problem. Here are some common strategies to master:

• Factoring Polynomials: If the polynomial is factorable, find its factors and set them equal to zero. This method is useful for solving quadratic or higher-degree equations.
• Using the Rational Root Theorem: If factoring is difficult, try using the Rational Root Theorem to test possible rational roots for the polynomial equation.
• Synthetic Division: Synthetic division is a fast method for dividing polynomials, especially when dividing by binomials like (x – a). It’s essential for simplifying expressions or finding roots.

By practicing these concepts and strategies, you will be well-prepared to handle any polynomial problems efficiently during the test. The more you practice working with different types of polynomial expressions, the more confident and accurate you will become when solving them.

Trigonometry Concepts You Should Know

Trigonometry plays a crucial role in solving problems related to angles, triangles, and periodic functions. Mastering its fundamental concepts will help you tackle a wide range of mathematical problems more efficiently. This section highlights key trigonometric ideas you should understand thoroughly before facing any related questions.

Understanding the Trigonometric Ratios: The basic trigonometric functions – sine, cosine, and tangent – are essential for solving problems involving right triangles. These ratios compare the lengths of specific sides of a right triangle to the angle in question.

• Sine (sin): The ratio of the length of the opposite side to the hypotenuse.
• Cosine (cos): The ratio of the length of the adjacent side to the hypotenuse.
• Tangent (tan): The ratio of the length of the opposite side to the adjacent side.

Unit Circle: The unit circle is a powerful tool for understanding trigonometric functions in all quadrants. It represents the relationship between angles and the coordinates on the circle, allowing you to easily find the values of trigonometric functions for any angle.

Understanding Angles in Radians and Degrees: Angles can be measured in both degrees and radians. It’s important to know how to convert between them and understand when to use each measure in different problems. A full circle is 360 degrees or 2π radians.

Identifying Key Identities: Trigonometric identities such as the Pythagorean identity, reciprocal identities, and co-function identities are essential for simplifying expressions and solving equations. Being familiar with these identities will save you time and effort during problem-solving.

• Pythagorean Identity: sin²θ + cos²θ = 1
• Reciprocal Identities: secθ = 1/cosθ, cscθ = 1/sinθ, cotθ = 1/tanθ

Graphing Trigonometric Functions: Understanding how to graph sine, cosine, and tangent functions is essential. Pay attention to their amplitude, period, phase shift, and vertical shift. This will help you interpret periodic behavior and solve equations more effectively.

By mastering these essential trigonometric concepts, you’ll be better equipped to solve problems and apply trigonometry in various mathematical contexts.

Handling Exponential and Logarithmic Problems

Exponential and logarithmic functions are critical in solving various types of problems, especially those involving growth, decay, and complex equations. To approach these types of questions effectively, it’s essential to understand the core principles behind each concept. This section will guide you through key strategies and helpful tips for solving exponential and logarithmic problems efficiently.

Understanding Exponential Functions

Exponential functions involve a constant base raised to a variable exponent. These functions are often used to model growth and decay, such as population growth or radioactive decay. The general form of an exponential function is f(x) = a * b^x, where “a” is a constant, “b” is the base, and “x” is the exponent.

• Identify the Base: Pay attention to the base value. It determines the behavior of the function. If the base is greater than 1, the function grows exponentially. If the base is between 0 and 1, the function decays.
• Rewrite Exponents: Often, you will need to rewrite exponents to simplify expressions. For example, b^x * b^y = b^(x + y).
• Solving Exponential Equations: To solve for x in an exponential equation, you can either take the logarithm of both sides or rewrite the equation to have a common base.

Mastering Logarithmic Functions

Logarithms are the inverse of exponential functions and are used to solve equations where the unknown appears as the exponent. The logarithmic form of an equation is log_b(x) = y, which is equivalent to b^y = x. Understanding logarithmic properties is key to solving problems effectively.

• Recognize Key Properties: Logarithms have several important properties that can simplify solving equations, such as the product, quotient, and power rules:
  • Product Rule: log_b(xy) = log_b(x) + log_b(y)
  • Quotient Rule: log_b(x/y) = log_b(x) – log_b(y)
  • Power Rule: log_b(x^n) = n * log_b(x)
• Change of Base Formula: When the base of the logarithm is not the same as the base of your calculator, use the change of base formula: log_b(x) = log(x) / log(b).
• Solving Logarithmic Equations: To solve logarithmic equations, you can either apply properties to combine logs or rewrite the logarithmic equation into an exponential form.

With practice, you’ll be able to apply these techniques to handle both exponential and logarithmic problems with ease. These types of functions are valuable tools for understanding complex systems, so mastering them will not only help you perform well in tests but also enhance your overall mathematical proficiency.

Preparing for Word Problems in Algebra 2

Word problems often seem daunting, but they are an excellent opportunity to apply mathematical concepts to real-world situations. These problems can test your ability to interpret information, set up equations, and solve for unknowns. The key to mastering word problems is understanding how to break them down into manageable steps and approach them systematically.

Steps for Solving Word Problems

Before diving into solving the problem, it is essential to understand the given information and what is being asked. Follow these steps to effectively handle any word problem:

• Read the Problem Carefully: Make sure you fully understand the context and what the problem is asking. Highlight or underline key phrases and numbers that will help you form the equation.
• Define Variables: Assign variables to unknown quantities. This will help you translate the word problem into a mathematical equation.
• Translate the Words: Convert the verbal statements into mathematical expressions. Look for keywords like “total,” “difference,” “product,” or “quotient” that indicate operations.
• Formulate the Equation: Once you’ve translated the problem, write down the equation that represents the situation.
• Solve the Equation: Use appropriate methods to solve for the unknowns, such as substitution, elimination, or factoring.
• Check the Solution: After solving, substitute the solution back into the original context to ensure it makes sense in the given scenario.

Common Types of Word Problems

Word problems can cover a wide range of topics, from basic arithmetic to more complex concepts like functions and systems of equations. Some common types you may encounter include:

• Distance, Rate, and Time Problems: These problems typically involve the relationship between speed, distance, and time. The formula to remember is Distance = Rate × Time.
• Mixture Problems: These involve combining two or more substances to achieve a desired concentration or value.
• Age Problems: These problems involve finding the ages of individuals based on relationships between their ages at different times.
• Work Problems: These require you to calculate the time it takes for one or more individuals to complete a task together, often using rates of work.

By practicing these techniques and familiarizing yourself with the types of word problems you may face, you’ll build confidence and improve your problem-solving skills. Remember, the more you practice, the better you’ll become at recognizing patterns and efficiently translating word problems into solvable equations.

Reviewing Systems of Equations

Systems of equations involve solving for multiple unknowns at the same time, and they are a crucial concept in mathematics. These systems can arise in various contexts, such as determining the point where two lines intersect or solving real-world problems involving relationships between multiple variables. Mastering the methods for solving these systems is essential for success in advanced mathematical topics.

Methods for Solving Systems of Equations

There are several approaches to solving systems of equations, and choosing the right method often depends on the form of the system and the context of the problem. Here are the most common methods:

• Substitution Method: In this method, one of the equations is solved for one variable, and this expression is substituted into the other equation. This reduces the system to a single equation with one variable, which can then be solved.
• Elimination Method: This method involves adding or subtracting the equations to eliminate one variable, allowing you to solve for the other. It’s particularly useful when the coefficients of one variable are the same or opposites in both equations.
• Graphical Method: Here, each equation is graphed on the coordinate plane, and the solution is the point where the two lines (or curves) intersect. This method is helpful for visualizing the solutions but can be less precise than algebraic methods.

Tips for Efficiently Solving Systems

To efficiently solve systems of equations, it’s essential to be organized and methodical. Here are some tips to help you avoid mistakes and solve systems effectively:

• Check for Simplifications: Before solving, look for opportunities to simplify the equations, such as factoring terms or canceling out common factors, which can make calculations easier.
• Choose the Best Method: Depending on the structure of the system, pick the method that will be easiest to implement. If one variable has a coefficient of 1 or -1, substitution may be quicker. If the coefficients are opposites, elimination could be more efficient.
• Double-Check Solutions: Once you find a solution, always substitute the values of the variables back into the original equations to verify that they satisfy both equations.

By practicing these methods and tips, you will be able to confidently solve systems of equations, whether they are linear, non-linear, or involve more than two variables. A strong understanding of these techniques will not only help you perform well on tests but will also be valuable in solving real-life problems where multiple factors are involved.

Critical Strategies for Time Management

Effective time management is essential when preparing for any challenging assessment. With limited time available, it’s important to plan your approach carefully to ensure you can cover all necessary material and perform well under pressure. By implementing proven strategies, you can maximize your efficiency and reduce stress during preparation and while taking the test itself.

Planning Your Study Sessions

One of the key strategies for managing time effectively is to plan your study sessions in advance. This involves breaking down the material into smaller, manageable sections and allocating specific times to each topic. Here are some tips for structuring your study sessions:

• Create a Study Schedule: Organize your study time into blocks, ensuring you cover all topics before the test. Be realistic about how much time you need for each section based on your level of comfort with the material.
• Prioritize Difficult Topics: Identify areas where you need more practice or are less confident, and allocate extra time for these subjects. This ensures that you address the more challenging parts first while leaving easier topics for later.
• Incorporate Breaks: Avoid long, uninterrupted study sessions. Schedule short breaks to keep your mind fresh and focused. The Pomodoro technique, which involves 25 minutes of work followed by a 5-minute break, is a popular method for maintaining concentration.

Maximizing Test Performance with Time Efficiency

When it comes to the test itself, effective time management is crucial for completing all questions within the allotted time. Here are some strategies to help you manage your time efficiently while working through the assessment:

• Skim Through the Entire Test: Before you begin, quickly skim through the entire test to get a sense of the question types and how much time each section might take. This will help you allocate time effectively for each part.
• Start with Easier Questions: Begin by answering the questions you feel most confident about. This will give you momentum and ensure you score points quickly before tackling the more difficult problems.
• Watch the Clock: Keep an eye on the clock to ensure you don’t spend too much time on any one question. If you’re stuck, move on to the next question and return to the challenging one later if you have time.
• Leave Time for Review: Always leave the last few minutes to review your answers. This gives you a chance to catch any mistakes and make adjustments before submitting your work.

By applying these time management strategies, you can improve your focus, reduce anxiety, and ensure that you’re fully prepared for the assessment. The ability to allocate your time effectively will not only help you achieve better results but will also enhance your overall test-taking experience.

Understanding Graphing and Its Importance

Graphing is a crucial skill for visualizing mathematical relationships and solving complex problems. It allows you to represent equations and functions in a way that makes their properties more understandable. By plotting data points and drawing curves, you can quickly see trends, intercepts, and other key features of mathematical models. This visual approach is particularly valuable when working with functions, as it helps clarify abstract concepts and makes analysis more intuitive.

The ability to graph accurately is essential for solving many types of problems, particularly when it comes to interpreting and analyzing data. Whether you are dealing with linear equations, quadratic functions, or more advanced topics, graphing provides a clear, visual representation of how different variables interact. It also serves as a powerful tool for checking the reasonableness of solutions, helping to identify mistakes or confirm correct answers.

Furthermore, understanding how to manipulate graphs–by shifting, stretching, or reflecting them–allows you to explore the effects of various transformations on functions. This knowledge is foundational for many topics in mathematics and is often required for successfully completing assessments and applying mathematical concepts in real-world scenarios.

Utilizing Practice Exams Effectively

Practice tests are an essential tool for reinforcing your understanding and assessing your readiness for any mathematical challenge. By simulating the test-taking environment, they help you become familiar with the types of questions you may encounter and provide an opportunity to refine your problem-solving skills. More importantly, they allow you to identify areas of weakness, so you can focus your study efforts on the topics that need the most attention.

Effective use of practice tests goes beyond simply completing the questions. To truly benefit from this method, it is important to approach these exercises strategically. Below are key tips for utilizing practice exams to their fullest potential:

1. Time Yourself

When practicing, always time yourself to mimic the real testing conditions. This will help you get used to managing your time effectively, ensuring that you don’t rush through questions or spend too much time on any one problem.

2. Review Your Mistakes

After completing a practice test, thoroughly review your mistakes. Understanding why you got a question wrong is just as important as knowing the correct answer. This will help you recognize patterns in your errors and avoid them in the future.

Strategy	Benefit
Timed Practice	Improves time management skills
Post-Test Review	Identifies areas of improvement
Multiple Practice Sets	Reinforces understanding of key concepts

By strategically integrating practice tests into your study routine, you can significantly improve your ability to handle the material confidently and efficiently. Ultimately, the goal is not just to complete the tests, but to learn from them and continuously improve your performance.

Final Exam Day Tips for Success

The day of an important assessment can be a source of stress and anxiety, but approaching it with the right mindset and preparation can significantly boost your performance. By ensuring that you’re physically, mentally, and emotionally ready, you can maximize your chances of success. This section highlights effective strategies that will help you stay focused, calm, and confident when it’s time to showcase what you’ve learned.

1. Get Adequate Rest the Night Before

A good night’s sleep is crucial for cognitive function. Rest helps improve memory retention, concentration, and overall problem-solving ability. Avoid cramming the night before, as this can lead to unnecessary stress and fatigue. Instead, focus on relaxation and ensuring that you wake up refreshed and ready to tackle the task ahead.

2. Eat a Healthy Breakfast

Your brain needs fuel to perform at its best. Eat a nutritious breakfast that includes protein, complex carbs, and healthy fats to provide sustained energy throughout the duration of the test. Avoid sugary or overly processed foods that may lead to an energy crash.

Tip	Benefit
Sleep Well	Improves cognitive function and memory
Healthy Breakfast	Provides sustained energy and focus
Arrive Early	Reduces stress and allows time to settle in

In addition to sleep and nutrition, it is important to arrive early to the testing location. This gives you time to calm your nerves, gather your materials, and mentally prepare for the task at hand. Being well-prepared will allow you to perform to the best of your ability, so take the time to organize your materials and approach the assessment with confidence.

Where to Find Reliable Answer Keys

Accessing trustworthy solutions to practice problems and assessments is crucial for effective learning. Having accurate keys can help verify your methods, correct mistakes, and solidify your understanding. However, it’s important to ensure the sources you use are dependable and authoritative to avoid confusion or misinformation.

1. Textbooks and Official Resources

Most textbooks come with a set of problems and their corresponding solutions either at the end of the book or in a separate companion guide. These solutions are usually reliable, as they are published by the authors or academic publishers.

• Check the back of the textbook for problem solutions.
• Look for companion solution manuals or guides published by the textbook authors.
• Use online platforms associated with the publisher for additional materials and support.

2. Educational Websites and Online Tools

There are numerous websites that offer detailed, step-by-step solutions for various subjects. Websites like Khan Academy, Wolfram Alpha, and other math-focused resources provide quality explanations for a wide range of problems.

• Websites like Wolfram Alpha can help with solving and explaining complex problems.
• Khan Academy provides video tutorials and practice problems that explain key concepts thoroughly.
• Other specialized academic websites often offer answer keys along with explanations and strategies.

3. Teachers and Tutors

In many cases, educators are a valuable resource for verifying your answers. Reaching out to your teacher or a tutor can provide personalized assistance in understanding the solutions, and they may even offer additional practice materials.

• Consult your teacher for additional resources and feedback.
• Work with a tutor to ensure you’re on the right track and that you’re understanding the material correctly.