Go Math Grade 5 Chapter 6 Answers and Solutions

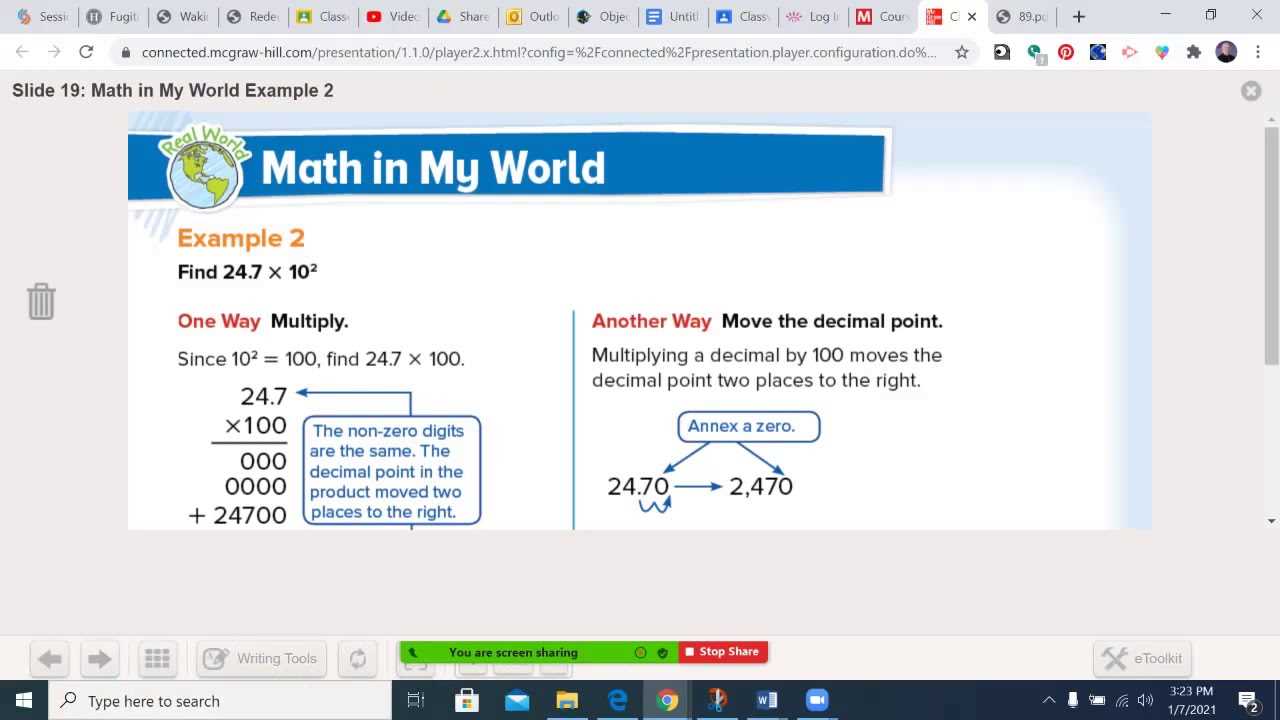
In this section, students will explore a variety of essential skills that form the foundation of their mathematical knowledge. These skills are crucial for building confidence and competence as learners progress through more complex topics. The focus is on understanding basic principles, practicing problem-solving techniques, and applying them in real-world situations.

Throughout the unit, students will encounter a range of exercises designed to reinforce important concepts and improve their ability to work with numbers and patterns. From basic arithmetic to more advanced topics, each lesson provides opportunities for learners to develop critical thinking and sharpen their reasoning skills.

By mastering these topics, students will be better equipped to tackle future challenges and excel in their academic journey. The practice problems included offer step-by-step guidance, ensuring that learners gain a solid understanding of the material while boosting their problem-solving abilities.

Go Math Grade 5 Chapter 6 Overview

This section focuses on key mathematical concepts that serve as building blocks for more advanced problem-solving techniques. The main goal is to guide students through various types of numerical operations, helping them to grasp the relationships between numbers and develop a deeper understanding of how to apply these skills in different contexts.

Throughout this unit, learners will engage with a series of practical exercises aimed at strengthening their computational abilities. Topics covered include the manipulation of fractions, multi-step calculations, and the application of these principles to solve real-life challenges. By working through these exercises, students will enhance their capacity to think critically and solve problems with confidence.

In addition to practice problems, visual aids and step-by-step explanations are provided to help clarify complex ideas. These resources ensure that learners can progress at their own pace while reinforcing the foundational knowledge required for future topics in their academic path.

Understanding the Key Concepts

In this section, students will delve into fundamental ideas that form the foundation for more advanced learning. These core principles are crucial for developing problem-solving skills and are applicable across various real-world situations. The goal is to build a strong understanding, allowing students to approach complex tasks with confidence.

Some of the key areas covered include:

• Identifying and comparing numerical values
• Understanding the structure of fractions and decimals
• Mastering basic operations such as addition, subtraction, multiplication, and division
• Solving word problems using critical thinking strategies

By thoroughly exploring these essential topics, students will improve their ability to tackle more challenging problems as they continue to progress in their academic journey. The focus is on building a solid base of knowledge that can be applied to a wide range of mathematical tasks.

Step-by-Step Solutions for Practice Problems

This section provides a detailed guide to solving practice exercises. By breaking down each problem into manageable steps, students can gain a clearer understanding of how to approach similar challenges in the future. The goal is to reinforce key concepts and offer a methodical approach to solving problems accurately and efficiently.

Breaking Down Complex Problems

Each solution begins by identifying the core elements of the problem. Understanding the question and determining the necessary operations are essential first steps. Once the problem is broken down, students can proceed with the calculations, applying the correct methods to find the solution.

Stepwise Approach for Accuracy

By following a structured, step-by-step approach, students will learn how to avoid common mistakes and build confidence in their ability to solve problems. This method ensures that each solution is reached logically and systematically, reinforcing the concepts taught in the previous sections.

Common Mistakes in Chapter 6

As students work through this section, there are several common errors that can occur. These mistakes often arise from misunderstandings of key concepts or rushing through problems without careful thought. Identifying these issues is essential for improving accuracy and ensuring a solid grasp of the material.

Some frequent errors include:

• Misunderstanding the relationship between fractions and decimals
• Forgetting to follow the correct order of operations
• Making calculation errors due to lack of attention to detail
• Overlooking key information in word problems

By recognizing and addressing these common mistakes, students can refine their skills and approach problems with greater confidence and precision.

How to Solve Word Problems Effectively

Word problems often present challenges because they require students to translate a written scenario into a mathematical operation. The key to solving these types of problems is breaking them down step-by-step and carefully identifying the important information. With a clear approach, students can solve even the most complex situations with confidence.

Identify the Important Information

Begin by reading the problem carefully and highlighting the key details. Pay attention to numbers, units, and any specific conditions mentioned in the text. By extracting this information, you’ll have a clearer idea of what the problem is asking and what needs to be calculated.

Set Up the Problem and Solve

Once you have identified the necessary information, set up the appropriate calculation or equation. It may help to write down the steps and work through the problem logically. Take your time to ensure that each step is followed accurately, and double-check your work before finalizing the answer.

Tips for Mastering Fractions

Understanding fractions is a crucial skill that can be challenging for many students. By mastering the basic principles and applying effective strategies, learners can gain confidence in working with fractions and apply these skills to solve various problems. Here are some practical tips to improve your fraction skills.

Key Strategies for Success

• Start by understanding the concept of a numerator and denominator
• Practice converting between fractions, decimals, and percentages
• Use visual aids like fraction bars or pie charts to better visualize the parts of a whole
• Focus on simplifying fractions by finding the greatest common divisor (GCD)

Common Mistakes to Avoid

• Adding or subtracting fractions with different denominators without finding a common denominator first
• Forgetting to reduce fractions to their simplest form after performing operations
• Misinterpreting word problems that involve fractions

By following these strategies and avoiding common pitfalls, students can significantly improve their understanding and proficiency with fractions.

Visual Aids for Better Understanding

Visual tools can significantly enhance a student’s comprehension and retention of complex concepts. By using diagrams, charts, and other graphical representations, learners can better understand relationships between numbers, fractions, and operations. These aids simplify abstract ideas and make them more tangible and relatable.

Effective Visual Tools

• Fraction Bars: A great way to visualize parts of a whole and compare different fractions.
• Number Lines: Help students understand the order of numbers and the concept of intervals.
• Pie Charts: Useful for showing percentages and visualizing how portions relate to a whole.
• Tables: Ideal for organizing data and making comparisons easier.

How to Use Visual Aids

• Start by breaking down problems into smaller, more manageable parts, and then represent these parts visually.
• Use color-coding to differentiate between different values or operations in a diagram.
• Practice drawing and interpreting these visual aids to strengthen your understanding of concepts.

Incorporating visual aids into problem-solving can help students grasp difficult concepts more easily and improve their overall learning experience.

Interactive Learning with Chapter 6

Engaging with content through interactive activities is a powerful way to reinforce learning. By actively participating in problem-solving and applying concepts in real-time, students can solidify their understanding. Interactive tools allow learners to experiment, make mistakes, and receive instant feedback, all of which contribute to a deeper grasp of the material.

Benefits of Interactive Exercises

• Encourages active participation and critical thinking
• Provides immediate feedback to help correct misunderstandings
• Enhances engagement through fun and dynamic activities
• Helps develop problem-solving skills in a practical setting

Interactive Learning Tools

Here is an example of how interactive tools can be used to practice various skills:

Activity	Concept Reinforced	Tools/Resources
Drag and Drop Fractions	Comparing fractions	Interactive fraction models
Quiz on Operations	Order of operations	Online quizzes
Word Problem Solver	Solving real-life scenarios	Interactive word problem generators

Using these interactive activities, students can actively explore and apply the concepts learned, which helps reinforce their knowledge and improves retention.

Help with Tough Problems in Chapter 6

Some problems can feel overwhelming, especially when they involve complex calculations or tricky concepts. However, with the right approach and problem-solving strategies, these challenges become easier to tackle. This section provides guidance for overcoming difficult problems and finding effective solutions.

Step-by-Step Problem Solving

When faced with a tough problem, break it down into smaller, manageable steps. First, identify what the problem is asking and determine the key information provided. Then, organize the data in a way that makes it easier to understand. For example, you can:

• Highlight the important numbers or values
• Identify the operations required (addition, subtraction, multiplication, etc.)
• Use diagrams or charts to visualize the relationships between numbers

Common Strategies for Success

• Check Your Work: Double-check each step to avoid simple mistakes.
• Ask for Help: Don’t hesitate to seek guidance from a teacher, peer, or tutor if needed.
• Practice Similar Problems: Repetition helps reinforce the techniques and methods used to solve these types of problems.

By following these steps and strategies, students can build confidence and develop the skills needed to overcome even the toughest problems.

Quick Review of Essential Formulas

Understanding key formulas is fundamental to solving problems efficiently and accurately. These formulas provide the tools needed to simplify calculations and help students apply concepts in a structured way. Here’s a quick review of some of the most important formulas that can be used in various types of problems.

Basic Arithmetic Operations

• Addition: a + b = c
• Subtraction: a – b = c
• Multiplication: a × b = c
• Division: a ÷ b = c

Fraction and Decimal Operations

• Adding Fractions: a/b + c/d = (ad + bc) / bd
• Converting Fractions to Decimals: a/b = a ÷ b
• Converting Decimals to Percentages: a × 100 = b%

By becoming familiar with these fundamental formulas, students can quickly navigate problems and develop a deeper understanding of the subject.

Importance of Repetition in Learning

Repetition plays a crucial role in mastering new skills and reinforcing concepts. The more often students engage with a particular task or problem, the more familiar it becomes, allowing them to internalize the information and perform better. Repeated practice helps strengthen neural connections, making it easier to recall and apply knowledge in future situations.

How Repetition Enhances Understanding

• Improves Retention: Frequent exposure to concepts helps move information from short-term to long-term memory.
• Builds Confidence: Repeated practice leads to increased competence and self-assurance when solving problems.
• Refines Skills: The more often a concept is practiced, the more precise and efficient the application becomes.

Effective Ways to Implement Repetition

• Work on similar problems regularly to reinforce techniques.
• Review previously learned concepts to strengthen understanding.
• Use a variety of practice methods, such as worksheets, interactive tools, or games, to maintain engagement.

Through consistent practice and repetition, learners can achieve mastery and confidence in their abilities, leading to long-term success in their studies.

How to Prepare for Chapter 6 Test

Proper preparation is key to performing well on any test. To achieve success, it’s essential to review concepts thoroughly, practice problem-solving skills, and manage your time effectively. This section provides a guide to help you prepare efficiently for the upcoming evaluation.

Review Key Concepts

• Understand Core Ideas: Revisit the main principles covered throughout the unit. Focus on the essential ideas and how they are applied to different types of problems.
• Study Practice Problems: Go through practice questions and review your answers. Identify areas where you may need additional practice.
• Clarify Doubts: If you have any lingering questions or uncertainties, ask a teacher or tutor for clarification.

Time Management Tips

• Set a Study Schedule: Break your study sessions into smaller, manageable time slots. This will help you stay focused and retain more information.
• Practice Under Test Conditions: Simulate the test environment by timing yourself while solving problems to improve your efficiency.
• Stay Organized: Keep track of the topics you’ve covered and make sure to allocate enough time for revision.

With consistent review, practice, and time management, you will be well-prepared to tackle any questions that come your way during the test.

Best Study Resources for Chapter 6

To effectively prepare for any unit, utilizing a variety of study materials can make a significant difference. From online platforms to printed resources, there are several tools available to help reinforce the concepts learned. This section explores some of the best resources that can aid in mastering the material covered in this unit.

Top Online Resources

• Interactive Learning Platforms: Websites such as Khan Academy and IXL provide interactive lessons and exercises to reinforce key concepts.
• Educational YouTube Channels: Channels dedicated to explaining various topics in a clear and engaging way can offer visual demonstrations of difficult concepts.
• Online Practice Tests: Platforms like Quizlet and Study.com offer practice quizzes to test your knowledge and track progress.

Printed and Offline Resources

• Workbooks and Practice Sheets: Workbooks that focus on specific topics provide ample practice and examples to work through.
• Teacher-Provided Materials: Teachers often provide study guides, worksheets, and practice problems tailored to the content.
• Study Groups: Collaborative learning with peers can help clarify doubts and provide different perspectives on the same material.

Resource Comparison Table

Resource	Format	Benefits
Interactive Websites (Khan Academy, IXL)	Online	Engaging exercises, instant feedback, tracking progress
YouTube Educational Channels	Online Videos	Visual demonstrations, easy-to-understand explanations
Workbooks & Practice Sheets	Printed	Hands-on practice, self-paced

Using a combination of these resources will help solidify your understanding and ensure you are well-prepared for the test. Whether you prefer interactive learning, watching videos, or doing hands-on practice, these tools provide a wide range of options to suit different learning styles.

Online Tools for Extra Practice

To reinforce learning and gain a deeper understanding of the concepts covered, online platforms offer a wide range of resources for extra practice. These tools allow learners to work at their own pace, revisit challenging topics, and track progress in real-time. From interactive exercises to comprehensive quizzes, these websites provide the support needed to solidify knowledge and improve problem-solving skills.

Interactive Learning Platforms

• Khan Academy: Offers free lessons, exercises, and instructional videos on a variety of topics. The interactive format helps students practice concepts and assess their understanding.
• IXL: Provides personalized practice in various subjects, offering immediate feedback and targeted learning paths based on performance.
• Prodigy: A game-based learning platform where students can practice skills in a fun, engaging way while progressing through levels.

Quiz and Practice Websites

• Quizlet: An excellent resource for creating custom flashcards and taking practice quizzes to test understanding of different topics.
• Study.com: Offers quizzes, video lessons, and practice exams to reinforce learning in a structured, guided way.
• Brilliant.org: Focuses on problem-solving and critical thinking through interactive problems and puzzles in a variety of subjects.

By integrating these online tools into your study routine, learners can gain additional practice, develop stronger problem-solving techniques, and build confidence in their ability to tackle complex topics. These platforms offer flexibility and tailored resources to suit every learner’s needs and pace.

Real-Life Applications of Math Concepts

The skills learned in academic exercises have practical applications in the real world. Whether you’re managing your personal finances, designing a building, or planning a trip, the ability to work with numbers, measurements, and problem-solving techniques is essential. In this section, we will explore how various principles are used in everyday scenarios and professions.

Everyday Uses

• Budgeting: Understanding how to calculate expenses, savings, and income allows individuals to make informed decisions about their finances.
• Shopping: Whether it’s calculating discounts or determining the total cost, knowledge of percentages and multiplication helps in making smart purchases.
• Cooking: Recipes often require measurements and conversions. Being able to adjust ingredient amounts based on serving size involves fractions and ratios.

Professional Applications

• Architecture: Architects use geometry and measurement concepts to design structures, ensuring they are safe and functional.
• Engineering: Engineers apply various principles of calculations, estimations, and measurements to develop machines, systems, and infrastructures.
• Science and Technology: Data analysis, statistical predictions, and algorithm development all rely on an understanding of numbers and patterns to solve problems.

By seeing how these concepts are used in the world around us, it becomes clear that the knowledge gained in academic studies has tangible value, shaping everyday life and contributing to many industries.

Parent and Teacher Guide for Support

Supporting a child’s learning journey requires collaboration between educators and caregivers. The key to success lies in clear communication, understanding the concepts being taught, and providing encouragement and reinforcement. In this section, we will explore effective strategies for parents and teachers to assist students in mastering the necessary skills and building confidence in their abilities.

Effective Strategies for Parents

• Create a Routine: Establish a consistent study schedule that allows time for both practice and rest. A quiet, distraction-free environment helps maintain focus.
• Ask Guiding Questions: Instead of giving answers directly, encourage critical thinking by asking questions like, “What do you think will happen next?” or “Can you explain how you solved that?”
• Positive Reinforcement: Celebrate small victories and progress. Recognize effort, not just results, to boost motivation and self-esteem.
• Be Patient: Every student learns at their own pace. Be understanding of frustrations and provide reassurance when needed.

How Teachers Can Assist

• Individualized Attention: Tailor instruction to meet the unique needs of each student. Differentiating learning techniques can help address various challenges.
• Provide Additional Resources: Offer extra practice materials, interactive tools, or visual aids to reinforce key concepts.
• Regular Check-ins: Consistently assess students’ understanding through informal quizzes or discussions to identify areas where extra support is needed.
• Encourage Collaboration: Facilitate group work or peer discussions, which can help students learn from each other and reinforce their understanding of concepts.

By working together, both parents and teachers can create a strong support system that fosters a positive and productive learning environment for students. Open communication and consistent encouragement can make a significant difference in a student’s academic growth and success.