Business Math Final Exam Answers and Solutions

Mastering the skills required to navigate complex calculations and logical problems is essential for anyone aiming to excel in assessment tasks. Gaining clarity and confidence in solving diverse scenarios ensures better outcomes and a solid grasp of critical concepts.
This guide provides practical insights, helpful strategies, and effective techniques to address various question types commonly encountered in tests. By focusing on clear examples and efficient approaches, you can enhance your preparation and achieve the results you desire.
Whether tackling numerical equations or interpreting situational problems, understanding the foundational principles and applying them systematically can make all the difference. With the right guidance and preparation, you’ll be ready to face any challenge with ease.
Business Math Final Exam Answers
Achieving success in any evaluation requires a clear understanding of essential concepts and the ability to apply them under pressure. It’s important to approach each task methodically, ensuring that all steps are followed precisely, from interpreting the question to delivering the correct solution.
Preparation plays a key role in building the confidence necessary to tackle complex scenarios. A strong foundation in core principles, combined with ample practice, allows for the development of problem-solving strategies that can be adapted to any situation presented during an assessment.
To excel, one must not only memorize key formulas but also understand the underlying logic that connects them. Recognizing patterns, simplifying equations, and maintaining focus on the bigger picture can help overcome even the most challenging questions.
Understanding Key Exam Concepts
In any assessment, understanding the core principles is essential for successful problem-solving. These fundamental ideas form the foundation for tackling a wide range of questions effectively and confidently. A solid grasp of these concepts enables you to navigate through various challenges without getting stuck or overwhelmed.
Familiarity with the underlying theories and methodologies allows you to break down complex problems into manageable steps. By mastering key principles, you can apply logical reasoning and strategic thinking, ensuring accuracy in your calculations and interpretations.
Each concept often builds upon others, creating a web of interconnected knowledge. Strengthening your understanding of these core areas provides the flexibility to adapt to different question formats and ensures that you’re prepared for any situation that may arise during the test.
Preparing for Business Math Exams
Proper preparation is key to tackling any assessment with confidence. A structured approach to reviewing material, practicing problems, and refining skills can significantly improve performance. By dedicating time to understanding the essential topics and techniques, you set yourself up for success.
Effective Study Strategies
Start by organizing the topics you need to review. Break them into manageable sections and allocate time each day for focused study. Utilizing practice problems and past tests helps reinforce your understanding and familiarizes you with the format of the questions.
Mastering Core Techniques
Concentrate on mastering the key methods and formulas that are commonly tested. Understanding the theory behind each technique enables you to apply it accurately, even when faced with unfamiliar situations. Regular practice is essential to gaining fluency in these core areas.
Common Topics in Business Math
In any assessment involving numerical reasoning, there are several key areas that are frequently tested. These subjects encompass a broad range of techniques used in solving problems related to finance, calculations, and analysis. Mastery of these topics is crucial for achieving success in any assessment or real-world application.
- Interest calculations: Simple and compound interest are fundamental concepts for understanding growth over time.
- Percentage-based problems: These are commonly used in budgeting, financial analysis, and discount calculations.
- Profit and loss: Knowing how to calculate gains and losses is essential for evaluating business performance.
- Cost analysis: Understanding fixed and variable costs is key to making informed financial decisions.
- Break-even analysis: A crucial tool for determining when a business will start to generate profit.
These topics are essential for anyone looking to work in a number of fields, including accounting, finance, and entrepreneurship. Being able to solve problems in these areas provides a solid foundation for more advanced concepts and applications.
Formula Mastery for Final Exams
Understanding and mastering key formulas is essential for solving a wide range of problems efficiently. These mathematical tools provide the foundation for addressing various scenarios, helping you to calculate values quickly and accurately. Mastery of these formulas ensures you can tackle questions with confidence and speed during assessments.
It’s important to not only memorize formulas but to also understand their applications and the logic behind them. This way, you’ll be able to adapt to different problem types and choose the correct formula based on the context.
| Formula | Description | Example |
|---|---|---|
| Simple Interest = P × R × T | Calculates the interest earned or paid on a principal amount over time. | For a loan of $1000 at 5% for 2 years, the interest would be $1000 × 0.05 × 2 = $100. |
| Compound Interest = P × (1 + R/n)^(nt) | Used for calculating interest when it compounds periodically. | If $1000 is invested at 5% annually for 2 years, with yearly compounding, the result is $1000 × (1 + 0.05/1)^(1×2) = $1102.50. |
| Break-even Point = Fixed Costs / (Price – Variable Costs) | Helps determine the level of sales needed to cover total costs. | If fixed costs are $5000, price per unit is $50, and variable costs are $30, the break-even point is $5000 / (50 – 30) = 250 units. |
By practicing these formulas regularly and understanding their practical applications, you’ll be well-prepared for any scenario that arises during assessments.
Time Management During Exams
Effective time management is crucial when tackling any type of assessment. Properly allocating time to each section ensures that you can address all questions thoughtfully and thoroughly, without feeling rushed or overwhelmed. By planning your approach and sticking to a clear timeline, you can optimize your performance and avoid unnecessary stress.
Strategic Planning
Before you begin, take a moment to review the entire test. Identify the easier questions that can be answered quickly and the more complex ones that require additional time. Allocate time for each section based on its difficulty and the number of marks assigned.
Staying Focused
During the assessment, stay focused on one question at a time. Avoid spending too much time on any single item. If you’re stuck, move on to the next question and come back to the difficult ones later. Prioritizing tasks effectively will help ensure that you complete everything within the allotted time.
| Task | Time Allocation | Notes |
|---|---|---|
| Quick Questions | 15-20 minutes | Answer straightforward questions first to build confidence and save time for complex ones. |
| Moderate Difficulty Questions | 30-40 minutes | Give these questions a balanced amount of time, ensuring thorough answers. |
| Complex Questions | Remaining Time | Spend the last portion of your time solving the hardest questions, ensuring you have enough time to check your work. |
By following a structured approach to time management, you can maintain control throughout the assessment and maximize your chances of success.
Practice Problems for Success
Regular practice is one of the most effective ways to prepare for any assessment. Solving a variety of problems enhances your ability to apply concepts, improves your problem-solving skills, and boosts your confidence. By engaging with a wide range of examples, you can identify patterns, reinforce your understanding, and tackle unfamiliar questions with ease.
Consistency is key. The more you practice, the more familiar you become with different question types and approaches. This repetition allows you to refine your methods, so you’re able to solve problems more quickly and accurately when it counts.
Challenge yourself by working on problems of increasing difficulty. Starting with simpler tasks and gradually progressing to more complex ones ensures steady improvement. It also prepares you to handle tricky situations that may arise during the assessment.
Additionally, practicing under timed conditions helps simulate the pressure of a real test. This practice will allow you to manage your time effectively and build mental stamina, so you’re fully prepared for the actual challenge.
Strategies for Solving Complex Problems
Tackling difficult problems requires a structured approach that allows you to break down the task into manageable steps. By using effective strategies, you can simplify complex scenarios and arrive at the correct solution more efficiently. These strategies can help you stay focused and organized when faced with challenging questions.
- Understand the Problem – Carefully read the question and identify the key elements. Take note of the given information and what is being asked. Understanding the full context is crucial before proceeding.
- Break It Down – Divide the problem into smaller, easier-to-manage parts. Focus on solving one section at a time rather than trying to tackle the entire problem all at once.
- Choose the Right Approach – Determine which method or formula best applies to each part of the problem. Whether it’s a formula-based solution, a logical step-by-step approach, or using estimation, selecting the right technique is essential.
- Work Backwards – If you’re stuck, consider working backwards from the desired solution. This technique can help you identify the steps needed to arrive at the correct result.
- Check Your Work – After completing the problem, review your steps and calculations. Ensure that all the data is applied correctly and that your solution aligns with the question’s requirements.
By employing these strategies, you’ll be able to approach complex problems with confidence, ensuring that you can work through difficult scenarios methodically and with accuracy.
Interpreting Word Problems Efficiently
Word problems often present challenges because they require translating written information into mathematical expressions. To solve these problems effectively, it’s essential to read carefully, extract relevant details, and translate them into a form that can be worked through logically. Mastering this skill can significantly improve your ability to tackle complex scenarios during assessments.
The key to success in word problems lies in identifying the critical elements and ignoring unnecessary details. Breaking down the problem into smaller, manageable parts helps simplify the task and ensures no important information is overlooked.
| Step | Action | Example |
|---|---|---|
| Read the Problem Carefully | Identify what is being asked and what information is provided. | For example, “A store sells pencils at $2 each. If a customer buys 5 pencils, how much does the customer pay?” |
| Highlight Key Information | Underline or note down important figures and terms. | Price per pencil: $2, Quantity: 5 |
| Formulate the Equation | Convert the word problem into a mathematical expression. | Total cost = 2 × 5 = $10 |
| Double-Check Your Work | Review the steps to ensure all information was used correctly. | Verify that you multiplied the correct numbers and interpreted the problem accurately. |
By practicing these techniques and using systematic steps, you can improve your ability to interpret and solve word problems with confidence and efficiency.
Using Calculators Effectively
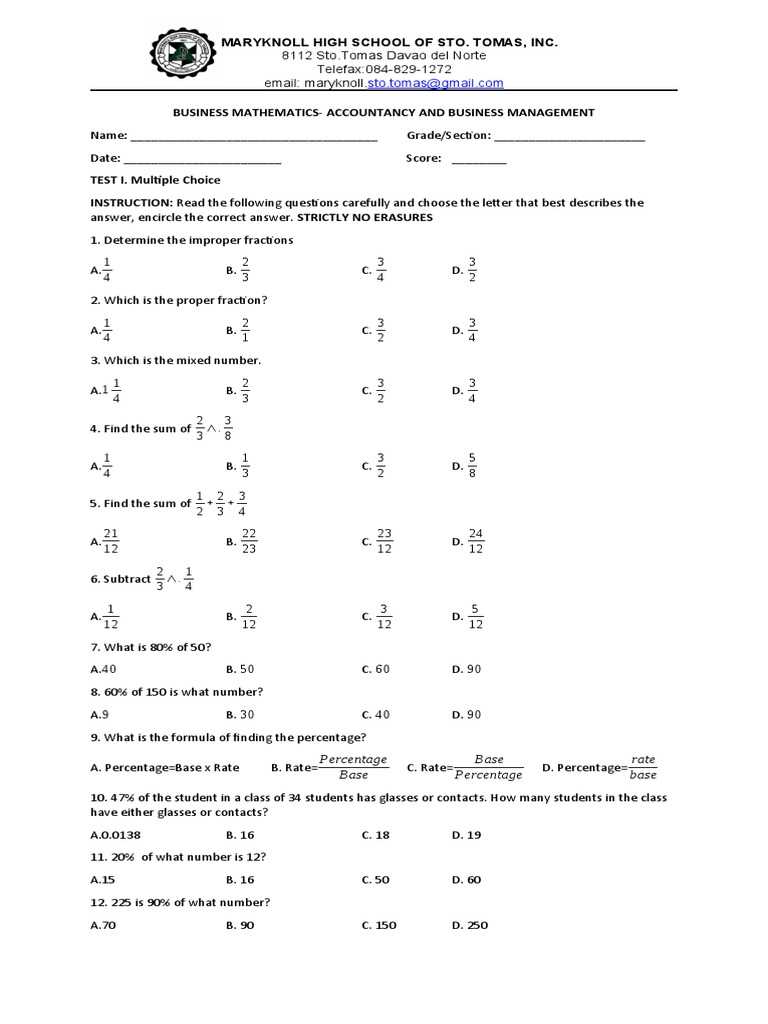
Calculators are powerful tools that can save time and reduce errors when solving numerical problems. However, using them effectively requires understanding their functions, practicing key operations, and knowing when to rely on them for accuracy. Mastering these skills will help you maximize the calculator’s potential and improve your overall performance.
One of the most important aspects of using a calculator is knowing the proper sequence of operations. This includes correctly inputting formulas, utilizing the memory functions, and checking for potential mistakes in calculations. With consistent practice, you can become proficient in using your calculator for both basic and complex tasks.
| Action | Explanation | Example |
|---|---|---|
| Understand Key Functions | Familiarize yourself with the functions your calculator offers, such as memory recall, square roots, or percentage calculations. | Use the square root function to find √16, which gives 4. |
| Use Parentheses for Order of Operations | Always input parentheses to ensure the correct order of calculations is followed. | For the expression (5 + 3) × 2, enter it as (5 + 3) × 2 to avoid incorrect results. |
| Double-Check Your Entries | Ensure the numbers and operators entered are correct before pressing the equals button. | If calculating 150 ÷ 3, verify that the numbers are accurate before pressing equals. |
| Take Advantage of Memory Features | Use memory functions to store and recall intermediate results, reducing the need to re-enter long numbers. | Store 200 in memory, then subtract 50 later by recalling the stored value. |
By practicing and getting familiar with your calculator’s features, you can speed up your problem-solving process, reduce errors, and feel more confident during any assessment or task.
Focus Areas for Business Tests
Preparing for assessments in this field requires focusing on key concepts and techniques that are frequently tested. Identifying and mastering these core areas will enhance your ability to solve problems efficiently and accurately. By honing in on the most relevant topics, you can maximize your preparation and perform at your best.
Essential Concepts to Review
- Percentages and Proportions: Understanding how to calculate percentages, proportions, and ratios is fundamental to many problems.
- Financial Statements: Be familiar with interpreting income statements, balance sheets, and cash flow statements to make accurate financial calculations.
- Interest Calculations: Master both simple and compound interest formulas to tackle time-based financial problems.
- Cost Analysis: Learn how to analyze cost structures, profit margins, and break-even points for different business scenarios.
- Investment Appraisal: Understand methods like net present value (NPV) and internal rate of return (IRR) for evaluating investment opportunities.
Important Problem-Solving Skills
- Problem Deconstruction: Break complex problems into smaller, more manageable steps to avoid feeling overwhelmed.
- Data Interpretation: Be proficient at interpreting graphs, tables, and charts to extract relevant information for calculations.
- Logical Reasoning: Practice developing logical arguments and sequences to ensure you’re solving problems efficiently.
- Time Management: Practice managing time effectively by focusing on high-yield areas and not spending too long on any single question.
By focusing on these key areas, you will be well-equipped to tackle the most common problems that arise in assessments. Targeted practice in these areas will not only help you understand the material but also boost your confidence when approaching complex tasks.
Tips for Mental Math Speed
Enhancing your ability to solve numerical problems quickly in your head is a valuable skill. Speed and accuracy are key when time is limited, and developing efficient mental strategies can help you become more proficient. By practicing specific techniques, you can solve problems faster and with greater confidence.
Start with Simple Calculations: Focus on mastering basic addition, subtraction, multiplication, and division. Familiarity with these operations will form the foundation for tackling more complex problems quickly.
Use Estimation Techniques: When an exact answer isn’t necessary, use estimation to arrive at a close approximation. Round numbers to simpler values and adjust accordingly.
Break Down Complex Problems: Divide larger problems into smaller parts. For example, when multiplying large numbers, break them into more manageable chunks and add the partial products together.
Memorize Key Multiplication Tables: The faster you can recall multiplication facts, the quicker you can handle division and multiplication problems. Familiarity with these basic facts will save you time during more complex calculations.
Practice Mental Shortcuts: Learn shortcuts for common calculations. For example, doubling a number and then halving it, or recognizing patterns in powers of 10, can help you solve problems much faster.
Visualize the Problem: Imagine numbers or visualize simple diagrams to make it easier to perform operations in your head. Visualization aids in organizing the problem in a way that simplifies your approach.
By incorporating these tips into your practice, you will significantly improve your ability to solve problems efficiently without relying on a calculator or paper. With consistent practice, your mental agility will increase, and you’ll be able to tackle challenges with speed and accuracy.
What to Expect in Business Assessments
When preparing for assessments in this field, it’s important to understand the types of questions and topics you will likely encounter. These assessments are designed to test your understanding of key concepts, as well as your ability to apply them in various scenarios. Knowing what to expect can help you focus your study efforts on the most critical areas.
Types of Questions
- Multiple Choice: These questions will test your ability to recognize correct solutions quickly. Often, they require you to apply a concept or formula in a short amount of time.
- Problem-Solving: Expect to encounter word problems where you must apply your knowledge to real-world situations. These questions require a more detailed approach and often involve multiple steps to find the correct solution.
- Calculation-Based Questions: These questions will require you to solve numerical problems, such as calculating interest, profit margins, or cost structures.
- Conceptual Questions: Some questions will test your understanding of core concepts and their applications. These are typically short answer questions that assess your theoretical knowledge.
Key Topics to Prepare For
- Financial Analysis: Be prepared to work with financial statements, profit margins, and other related concepts to assess business performance.
- Investment Calculations: Understand methods like ROI, NPV, and other metrics used to assess the viability of investments.
- Cost and Revenue Analysis: Know how to calculate break-even points, pricing strategies, and analyze cost structures in a business context.
- Basic Algebra and Formulas: Many questions will require you to manipulate basic equations and solve for unknowns, especially in business scenarios.
By focusing on these areas, you will be well-equipped to handle the types of questions that typically appear in these assessments. The key is to stay organized, understand the concepts thoroughly, and practice applying them in various situations.
Key Formulas to Memorize
When preparing for assessments in this area, it’s crucial to have a strong grasp of essential formulas that will help you solve problems quickly and accurately. These formulas serve as tools that you can apply to a wide range of scenarios, making calculations easier and faster. Memorizing these key formulas will save you time and allow you to focus on applying your knowledge effectively.
Here are some of the most important formulas you should commit to memory:
- Simple Interest Formula: I = P × r × t
This formula is used to calculate interest earned or paid on a principal amount, where P is the principal, r is the rate, and t is the time period.
- Compound Interest Formula: A = P(1 + r/n)^(nt)
This formula calculates the amount of money accumulated after interest is applied to the principal over time, with interest compounded at regular intervals.
- Profit Margin Formula: Profit Margin = (Net Income / Revenue) × 100
This formula helps calculate how much profit a company generates from its total revenue, expressed as a percentage.
- Break-even Point Formula: Break-even Point = Fixed Costs / (Selling Price – Variable Cost)
This formula is used to determine the number of units a company must sell to cover its fixed costs and start making a profit.
- Return on Investment (ROI) Formula: ROI = (Net Profit / Investment Cost) × 100
This formula helps assess the profitability of an investment by calculating the return as a percentage of the initial investment cost.
By mastering these formulas, you will be well-prepared to tackle a variety of problems efficiently. Regular practice with these formulas will help you build confidence and speed, allowing you to focus on solving complex questions with ease.
Handling Difficult Business Questions

When faced with challenging questions, it’s important to remain calm and organized. Complex problems may initially seem overwhelming, but breaking them down into smaller, manageable parts can make them much easier to approach. With the right strategies, you can navigate even the toughest problems efficiently and accurately.
Steps to Approach Complex Problems
Start by carefully reading the question to identify what is being asked. Pay attention to keywords and any data provided that will be essential to solving the problem. Once you have a clear understanding, consider the formulas or concepts that are relevant to the task. If the problem involves calculations, determine the best approach to solve it step-by-step.
Effective Strategies for Tough Questions
- Break it down: Break the problem into smaller sections. Tackle each part individually, ensuring you address each step carefully.
- Check your assumptions: Sometimes problems contain hidden information. Double-check all assumptions to ensure you are working with the right values.
- Revisit concepts: If a question seems unclear, revisit related concepts or review any relevant notes. Often, a deeper understanding of underlying principles will reveal the answer.
- Use estimation: If exact values are not immediately clear, use estimation to get a rough idea of what the answer should be. This can guide you toward the correct solution.
By following these strategies, you will be better equipped to handle even the most challenging questions. The key is to stay organized, think critically, and remain patient as you work through the problem. Practice regularly to build your confidence and refine your approach to complex scenarios.
Common Mistakes to Avoid
During assessments, it’s easy to make simple errors that can impact the accuracy of your work. By recognizing common mistakes ahead of time, you can take proactive steps to avoid them. Awareness of these pitfalls is crucial to improving performance and ensuring you achieve the best results possible.
Common Pitfalls to Watch Out For
- Rushing Through Questions: Taking your time to fully read each question and understand its requirements can prevent careless mistakes. Rushing often leads to overlooked details or misinterpretation of the problem.
- Skipping Steps: It can be tempting to skip steps when you feel confident, but this often leads to errors. Always document each part of your solution to ensure you don’t miss critical calculations or logic.
- Misapplying Formulas: Applying the wrong formula or incorrectly using variables can lead to incorrect results. Double-check which formula is appropriate for each situation and ensure you’re using the correct values.
- Overlooking Units: Forgetting to convert or properly account for units can lead to incorrect answers. Make sure to consistently check and manage units throughout your problem-solving process.
How to Prevent These Mistakes
- Double-check your work: Before submitting any solution, review your calculations and logic to catch errors.
- Practice regularly: Repetition helps reinforce key concepts and reduces the likelihood of mistakes during assessments.
- Work systematically: Follow a clear, step-by-step process to solve each problem. This structured approach reduces the risk of skipping essential steps.
- Stay organized: Keep your work neat and organized, as it helps avoid confusion and errors, especially during complex tasks.
By recognizing and preventing these common mistakes, you can improve your accuracy and approach each problem with confidence. Consistent practice, careful attention to detail, and a methodical approach will help you avoid errors and perform at your best.
How to Review Before the Exam
Preparing for an important test requires a structured and focused approach. Reviewing the material effectively can help reinforce key concepts and boost your confidence. By organizing your time and reviewing strategically, you can ensure you are well-prepared for the assessment.
Effective Review Strategies
- Start Early: Begin your review sessions well in advance. This gives you enough time to identify and address any areas where you need improvement.
- Focus on Weak Areas: Spend extra time on topics or problems that you find challenging. Don’t just skim through familiar material, as this can lead to overconfidence.
- Use Practice Problems: Solving practice problems is one of the best ways to reinforce your knowledge and test your understanding of key concepts.
- Summarize Key Concepts: Create a summary or cheat sheet of important formulas, rules, and strategies. This can serve as a quick reference during your review and help you recall essential information more easily.
Maximizing Your Review Time
- Review in Short Sessions: Break your study sessions into manageable chunks. Studying for longer periods can lead to burnout and reduce retention.
- Teach What You’ve Learned: Explaining complex concepts to someone else can help you solidify your own understanding and reveal any gaps in your knowledge.
- Stay Organized: Keep your notes and study materials in order, so you can easily find and review specific topics when needed.
- Take Breaks: Give yourself regular breaks to avoid mental fatigue. A short rest can improve focus and productivity during your next study session.
By following a focused and well-planned review strategy, you can maximize your preparation and approach the assessment with confidence and clarity.