Geometry Quarter 3 Exam Review Answers

Preparing for a challenging assessment in the field of shapes, measurements, and logical problem solving requires a clear understanding of the key concepts. By focusing on the core principles, you can approach each question with confidence and efficiency. This section will guide you through the most important topics you need to grasp before the test day.

From solving complex equations to mastering the relationships between different figures, it’s crucial to familiarize yourself with essential techniques. Whether it’s applying the Pythagorean theorem or analyzing the properties of angles and polygons, this guide will offer valuable insights into tackling each type of problem effectively. Mastery of these fundamentals is your key to success.

Geometry Quarter 3 Exam Review Answers

In this section, we’ll cover the key concepts that will help you navigate through the test. Whether you are dealing with complex shapes or solving measurement problems, understanding the fundamental principles will make a significant difference in your performance. The following tips and strategies will guide you in mastering the material you need for success.

• Familiarize yourself with basic formulas and theorems.
• Understand how to apply algebraic methods to solve geometric problems.
• Review the key properties of different shapes, including triangles, circles, and polygons.
• Practice transforming figures through translation, rotation, and reflection.
• Focus on real-world applications of the concepts you’ve learned.

By focusing on these core elements, you can approach each question with a clearer mindset. Remember to pay special attention to the questions that involve calculations and proofs, as they tend to require the most detailed understanding.

In the following sections, we will break down the essential strategies and solutions to commonly asked questions. Being well-prepared will allow you to manage your time more effectively and handle even the trickiest problems with ease.

Key Topics Covered in Quarter 3

In this section, we’ll highlight the essential subjects you need to master in preparation for the upcoming test. The focus is on understanding the core principles that apply to a wide range of problems, from calculating measurements to proving relationships between different shapes. Mastering these areas will give you the confidence to approach each question effectively.

Transformations and Symmetry

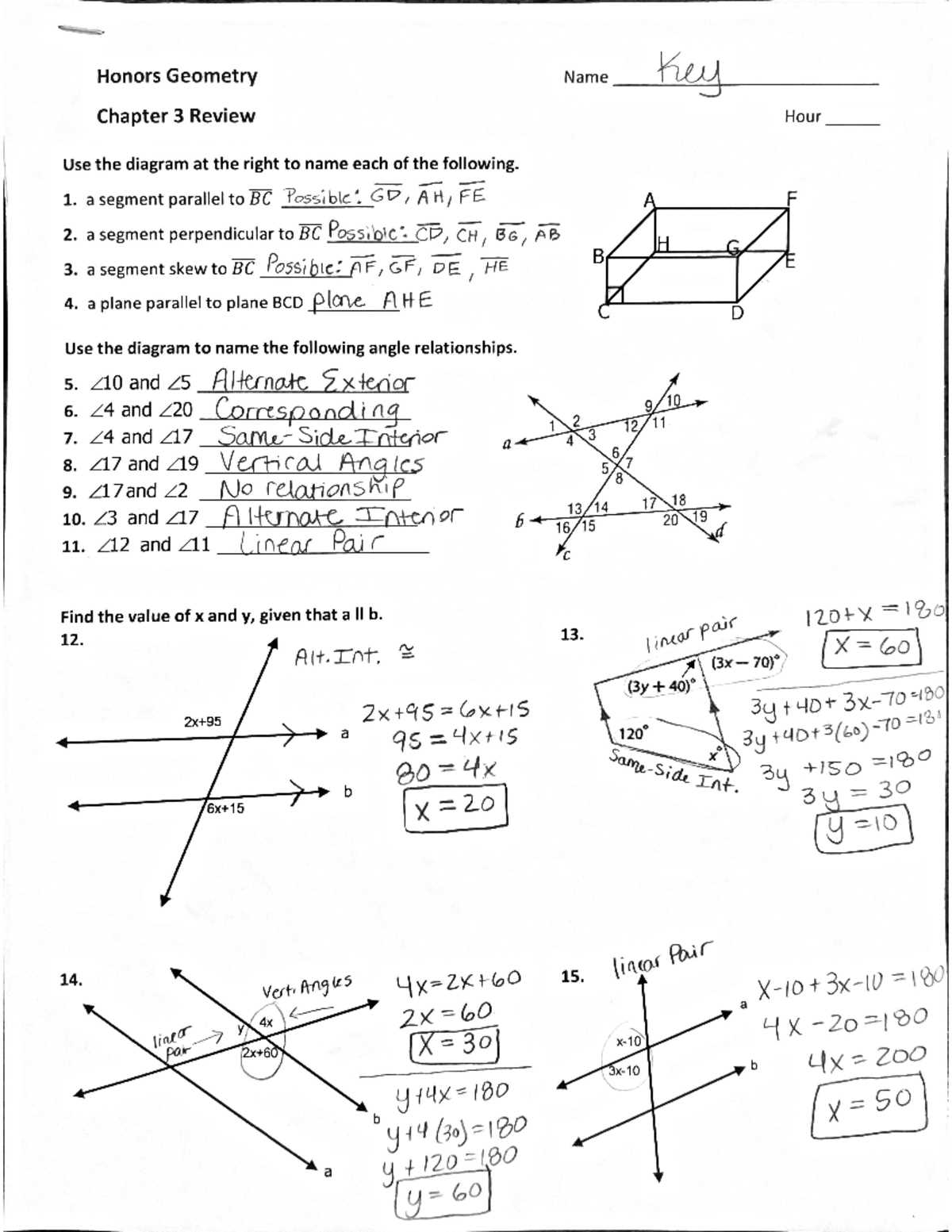
A significant portion of the material involves understanding how figures can be moved and altered. You will need to know how to apply translations, rotations, and reflections to different shapes and determine their properties under these transformations. Symmetry plays an important role, helping you understand the geometric structure of various objects.

Properties of Figures and Solving Problems

Another key area focuses on the properties of various shapes and how to use these characteristics to solve problems. This includes understanding the relationships between the angles, sides, and diagonals of triangles, quadrilaterals, and circles. Additionally, you will need to apply algebraic methods to solve complex problems that involve these figures.

Important Formulas for Geometry Exams

Mastering key formulas is essential for solving problems efficiently and accurately. Understanding how to apply these equations in various scenarios will enable you to approach each question with confidence. Below, we’ll outline some of the most important formulas you’ll need for solving different types of problems.

One of the most frequently used formulas is for calculating the area of basic shapes such as rectangles, triangles, and circles. Additionally, knowing how to calculate the perimeter and volume of three-dimensional objects is crucial. Understanding the relationships between angles and sides in various polygons will also help simplify complex problems.

Make sure to familiarize yourself with these essential equations and practice applying them to various examples. This will allow you to navigate through the test more effectively and save time on complicated questions.

How to Solve Coordinate Geometry Problems

Solving problems involving points on a plane requires understanding the relationship between coordinates and the geometric figures they represent. By mastering key techniques and formulas, you can quickly determine distances, midpoints, slopes, and other important properties. This section will guide you through the necessary steps to tackle these types of problems effectively.

Finding Distance Between Two Points

To calculate the distance between two points on a plane, use the distance formula: d = √((x2 – x1)² + (y2 – y1)²). This formula helps determine how far apart two points are by using their x and y coordinates. It’s essential for solving problems related to lengths of segments and determining relative positions of objects.

Determining the Slope of a Line

The slope of a line is a measure of how steep it is and can be found using the formula: m = (y2 – y1) / (x2 – x1). This ratio of vertical change to horizontal change is fundamental for solving problems that involve the relationship between two points or finding the equation of a line. Understanding the slope will help you analyze parallel and perpendicular lines as well.

Understanding Geometric Proofs and Theorems

Solving logical problems that require justification of statements involves using established principles and methods. A proof is a step-by-step explanation that demonstrates why a particular statement or equation holds true. Theorems are important conclusions that have been proven based on earlier propositions. Mastering these proofs and understanding the reasoning behind each theorem is key to succeeding in related problems.

Types of Proofs

There are various methods for proving geometric statements, with the most common being two-column proofs and paragraph proofs. Each method involves providing logical steps that lead to the desired conclusion, using previously established facts, definitions, and postulates. A clear understanding of the approach you use will help in organizing your reasoning effectively.

Commonly Used Theorems

Below is a table of some important theorems and their applications that you should be familiar with:

Theorem	Application
Pythagorean Theorem	Used to calculate the length of the sides in a right triangle.
Angle Sum Theorem	States that the sum of the interior angles of a triangle is always 180°.
Parallel Postulate	Used to prove the relationships between parallel lines and transversals.
Congruent Triangles Theorem	Helps prove that two triangles are congruent based on side-angle-side (SAS) or angle-side-angle (ASA) conditions.

By practicing these proofs and theorems, you will improve your ability to construct clear and logical solutions, which are essential skills for solving complex problems.

Mastering Transformations in Geometry

Understanding how figures can be moved, rotated, reflected, or resized is essential for solving problems that involve shapes and their positions. Transformations describe the different ways in which a shape can be altered on a plane while preserving certain properties. Mastery of these operations enables you to analyze geometric relationships more effectively and solve a wide range of questions.

There are three primary types of transformations that you need to know: translations, rotations, and reflections. Each type involves shifting the position of a figure in a specific way, but all transformations preserve the figure’s size and shape. Understanding the rules and methods for applying these transformations is key to solving problems that involve symmetry, congruence, or similarity.

Practice applying these techniques by working through problems that ask you to manipulate shapes using one or more of these transformations. The more you familiarize yourself with their properties, the easier it will be to recognize how and when to apply each one in different contexts.

Tips for Solving Word Problems Effectively

Word problems can often seem intimidating, but with the right approach, they become much more manageable. The key to solving them is breaking down the information step by step and translating it into mathematical operations. By staying organized and methodical, you can simplify even the most complex scenarios and find the solution more efficiently.

• Read the problem carefully: Make sure you understand all the details and the question being asked before proceeding.
• Identify key information: Highlight or underline the numbers, terms, and relationships that are important for solving the problem.
• Draw a diagram: Whenever possible, sketch a visual representation of the problem to help you visualize the situation.
• Define variables: Assign variables to unknown quantities to create an equation or system of equations.
• Set up an equation: Use the information provided to form an equation that you can solve for the unknowns.
• Check your work: After solving the problem, review your steps and make sure your answer makes sense in the context of the question.

By following these tips and practicing regularly, you’ll develop the skills needed to tackle word problems with confidence and accuracy. Remember, clarity and organization are key to finding the solution efficiently.

Identifying Types of Triangles and Their Properties

Understanding the different types of triangles and their unique characteristics is fundamental in solving problems involving shapes and angles. Triangles can be classified based on their sides and angles, and each type has specific properties that make it useful for various geometric tasks. Knowing these properties allows you to quickly identify and analyze any triangle in a problem.

Classification by Sides

Triangles can be categorized based on the lengths of their sides. Each classification has distinct characteristics that help in solving related problems:

Type of Triangle	Properties
Equilateral	All three sides are equal, and all interior angles measure 60°.
Isosceles	Two sides are equal, and the angles opposite those sides are also equal.
Scalene	All three sides have different lengths, and all angles are different.

Classification by Angles

In addition to classification by sides, triangles can also be categorized based on their internal angles:

Type of Triangle	Properties
Acute	All angles are less than 90°.
Right	One angle is exactly 90°.
Obtuse	One angle is greater than 90°.

By recognizing these different types of triangles and understanding their properties, you can efficiently solve problems that involve angle relationships, side lengths, and other geometric concepts.

Understanding Circles and Their Equations

Circles are one of the most fundamental shapes in mathematics, and understanding their properties is key to solving various problems involving distances, areas, and coordinates. A circle is defined by its center and radius, and its equation can be used to describe its position on a coordinate plane. Mastering the equation of a circle allows you to determine important properties such as its radius, center, and points that lie on it.

The standard equation of a circle is (x – h)² + (y – k)² = r², where (h, k) represents the center of the circle, and r is the radius. This formula enables you to graph a circle and find the relationship between its geometric properties and its algebraic representation. The ability to manipulate and interpret this equation is crucial for solving problems involving circles in both algebraic and geometric contexts.

Understanding the different forms of the circle’s equation and how to apply them to real-world scenarios will help you solve problems related to distance, tangents, and intersections with other shapes. By practicing the conversion between general and standard forms and exploring how to identify key features from the equation, you’ll gain a deeper insight into how circles function in various geometric situations.

Key Strategies for Tackling Angles

Angles play a central role in many problems involving shapes and measurements. Whether you’re working with straight lines, polygons, or more complex figures, understanding how to identify, calculate, and manipulate angles is essential for solving these types of problems. By applying certain strategies, you can efficiently approach angle-related questions and find solutions more easily.

Using Angle Relationships

One of the most effective strategies is to use known angle relationships to simplify problems. These relationships can help you find missing angles when dealing with parallel lines, intersecting lines, or polygons. Some of the most important relationships include:

Angle Type	Relationship
Complementary Angles	Two angles that add up to 90°.
Supplementary Angles	Two angles that add up to 180°.
Vertical Angles	Angles that are opposite each other when two lines intersect, and are always equal.

Using Trigonometric Ratios

For right-angled triangles, trigonometry provides a powerful set of tools for finding missing angles and sides. The most commonly used trigonometric ratios are:

Ratio	Definition
Sine (sin)	Opposite side / Hypotenuse
Cosine (cos)	Adjacent side / Hypotenuse
Tangent (tan)	Opposite side / Adjacent side

By applying these strategies, you can approach problems involving angles with confidence. Whether you’re calculating unknown angles, applying angle sum rules, or using trigonometry, these methods will help you efficiently find solutions and enhance your problem-solving skills.

Important Geometric Figures You Should Know

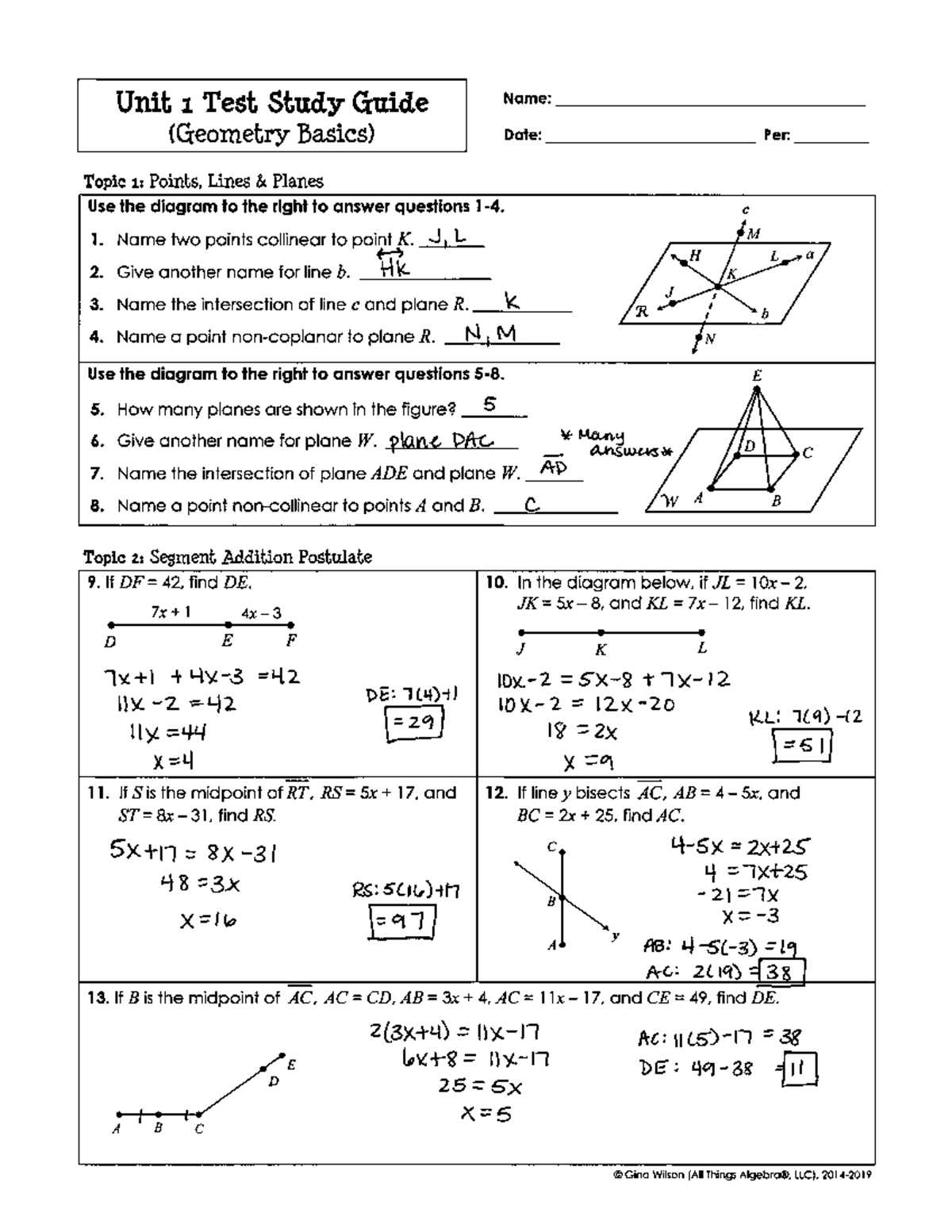
Understanding the basic shapes and their properties is essential for solving various mathematical problems. These figures are foundational to many concepts and are used in a wide range of problem-solving scenarios. Knowing their characteristics, formulas, and how to apply them can make tackling complex problems much easier.

Here are some of the most important shapes and figures you should be familiar with:

• Circle: A round shape where all points are equidistant from the center. The formula for the area is πr² and the circumference is 2πr.
• Triangle: A three-sided polygon that can be classified by its angles (acute, obtuse, right) and sides (equilateral, isosceles, scalene). The area is calculated as ½ × base × height.
• Rectangle: A quadrilateral with opposite sides equal and four right angles. The area is length × width and the perimeter is 2(length + width).
• Square: A special type of rectangle where all sides are equal. The area is side² and the perimeter is 4 × side.
• Parallelogram: A four-sided shape with opposite sides parallel. The area is base × height.
• Trapezoid: A four-sided figure with one pair of parallel sides. The area is ½ × (base₁ + base₂) × height.

Each of these shapes has its own unique properties and formulas. Mastering them will give you a strong foundation for solving a variety of problems, from basic area and perimeter calculations to more advanced applications in coordinate systems and transformations.

How to Approach Measurement Questions

When solving problems involving dimensions, it’s important to have a systematic approach. Measurement questions often require calculating lengths, areas, volumes, or angles. Having a clear strategy and knowing which formulas to use can make these problems much easier to solve.

Here are some essential steps to follow when tackling measurement-related questions:

• Understand the given data: Before starting any calculations, carefully read the problem to identify all the provided measurements and understand the context.
• Choose the right formula: Based on the type of measurement, select the appropriate formula. For example, to calculate the area of a rectangle, use length × width, and for the volume of a cube, use side³.
• Check the units: Make sure all measurements are in the same unit before performing calculations. If they are not, convert them to the same unit.
• Break down the problem: If the problem involves complex shapes or multiple measurements, break it into smaller, manageable parts. Calculate the area of each shape or figure separately, then combine the results.
• Use estimation when possible: For quick checks, estimate the answer before performing the exact calculation to ensure your result makes sense.
• Double-check your work: After solving, review your steps to verify that all calculations were correct and that you’ve used the proper formulas.

By following these steps, you can approach measurement problems with confidence. Whether you’re calculating distances, areas, or volumes, having a structured approach will help you solve problems more efficiently and accurately.

Review of Pythagorean Theorem Applications

The Pythagorean Theorem is a fundamental principle in mathematics, widely used to solve problems involving right-angled triangles. It establishes a relationship between the lengths of the sides of a right triangle, where the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This powerful tool is essential for many practical applications, ranging from construction to navigation.

Here are some common applications of the Pythagorean Theorem:

• Finding the length of a side: Given two sides of a right triangle, the theorem allows you to calculate the missing side. For example, if you know the lengths of the legs, you can find the hypotenuse using the formula c² = a² + b².
• Distance between two points: In coordinate geometry, the theorem can be used to find the straight-line distance between two points. By treating the horizontal and vertical differences as the legs of a right triangle, you can apply the formula to determine the shortest distance between the points.
• Real-life scenarios: The theorem is often used in construction to ensure right angles, such as in laying out a building’s foundation. It’s also applied in navigation to calculate direct routes, whether on a map or in aerial paths.
• 3D applications: The Pythagorean Theorem can also be extended to three-dimensional space. For instance, to find the diagonal distance across a rectangular box, you apply the theorem twice to the lengths of the sides.

By mastering the use of the Pythagorean Theorem, you can tackle a wide range of practical and theoretical problems. Understanding its applications ensures that you can approach both simple and complex problems with confidence, making it one of the most valuable concepts in mathematics.

Properties of Polygons You Need to Remember

Polygons are multi-sided figures that play a key role in various mathematical problems. They have distinct properties that determine their shape, size, and symmetry. Whether you’re working with triangles, quadrilaterals, or more complex shapes, understanding these characteristics is essential for solving problems efficiently and accurately.

Here are some important properties of polygons that you should keep in mind:

• Sum of interior angles: The sum of the interior angles of a polygon can be calculated using the formula (n – 2) × 180°, where n represents the number of sides. For example, the sum of the interior angles of a hexagon (6 sides) is 720°.
• Exterior angles: The exterior angles of any polygon always add up to 360°, regardless of the number of sides. This is true for all polygons, including irregular ones.
• Types of polygons: Polygons are classified based on the number of sides. For example, a triangle has 3 sides, a quadrilateral has 4, a pentagon has 5, and so on. Regular polygons have equal sides and angles, while irregular polygons do not.
• Symmetry: Regular polygons have rotational and reflective symmetry. For instance, an equilateral triangle has three lines of symmetry, while a square has four lines of symmetry.
• Diagonals: The number of diagonals in a polygon can be calculated using the formula n(n – 3) / 2, where n is the number of sides. This helps you understand how the interior of a polygon is divided by lines connecting non-adjacent vertices.

Mastering these properties will help you solve a variety of problems involving polygons. From calculating angles to determining symmetries and diagonals, these key concepts are essential for anyone studying the fundamentals of shape and space.

Using Algebra to Solve Geometry Problems

Algebra is a powerful tool for solving problems that involve shapes, distances, and measurements. By applying algebraic techniques, you can express geometric relationships in terms of equations, making it easier to find unknown values such as lengths, angles, or areas. This combination of algebraic methods with geometric principles allows for more efficient problem-solving and deeper understanding.

One common method is using variables to represent unknown quantities. For example, if you are given the lengths of some sides of a shape but need to find others, you can set up an equation based on the known relationships between the sides. Solving these equations will give you the required values. Here are some key ways in which algebra can be applied:

• Solving for unknown lengths: If you know some dimensions of a figure, you can use algebra to find the missing lengths by setting up an equation based on the perimeter or other known properties.
• Using the Pythagorean theorem: Algebra is often used to solve right-angled triangle problems. By substituting known values into the Pythagorean theorem formula, you can easily solve for unknown sides.
• Expressing area and volume: You can use algebraic expressions to calculate the area or volume of different shapes, such as squares, circles, or cylinders, by substituting variables for dimensions and solving for the unknowns.
• Working with coordinate systems: Algebra helps in solving problems related to coordinates, such as finding the distance between points or the equation of a line that passes through two given points.

Using algebra in geometry not only simplifies complex problems but also enhances your problem-solving skills by teaching you to think logically and systematically. Mastering this technique can make many challenging problems more manageable and provide a solid foundation for understanding more advanced topics.

Common Mistakes to Avoid on the Exam

During tests that involve solving mathematical and spatial problems, students often make a few key errors that can significantly affect their performance. Recognizing these common mistakes in advance allows you to approach questions more carefully, improving both your accuracy and confidence. Here are some pitfalls to watch out for:

• Skipping Steps in Calculations: It’s tempting to skip intermediate steps in your calculations to save time, but this often leads to errors. Always work through each part of the problem and double-check your work before finalizing the answer.
• Misinterpreting the Question: Sometimes, students read a question too quickly and overlook important details. Take the time to carefully read the instructions and ensure you understand what is being asked before starting to solve the problem.
• Not Labeling Units Properly: Failing to include proper units or forgetting to convert between different units can lead to mistakes. Always include the correct units in your final answer and be consistent throughout your calculations.
• Confusing Formulas: Different problems may require different formulas or methods for solution. It’s crucial to know which formula applies to each type of problem and to apply it correctly. Double-check that you’re using the right approach.
• Overlooking Special Cases: Some problems have special conditions or exceptions. These can be easy to overlook if you’re rushing through a test. Make sure to review your work and check for any specific conditions or constraints mentioned in the question.
• Rushing Through Geometry Constructions: In problems that require drawing or constructing shapes, rushing can lead to inaccurate or incomplete diagrams. Take your time to draw neat, accurate figures to avoid errors in subsequent calculations.

Avoiding these common mistakes can make a significant difference in your performance. With careful attention to detail and proper preparation, you’ll be able to approach the test with confidence and accuracy.

How to Manage Time During the Test

Effective time management during an assessment is crucial for ensuring that you can address all the problems with care and accuracy. It’s easy to get caught up in difficult questions, leaving less time for simpler ones. By adopting a strategic approach, you can avoid feeling rushed and ensure a more balanced performance throughout the test.

Set a Time Limit for Each Question

Before you begin solving, quickly scan through the entire test to get an overview of the problems. Allocate a specific amount of time to each section based on its complexity. For example, if there are multiple-choice questions, assign a shorter time per question compared to open-ended problems. Keep track of time as you progress and avoid spending too much time on any single problem.

Prioritize the Easier Problems

Start with the questions that seem the most straightforward. These are usually the problems that you can solve quickly and with confidence. By finishing these first, you can secure those points and have more time for the tougher questions later on. If you get stuck on a more difficult problem, don’t dwell on it for too long–move on and come back to it if time allows.

Incorporating these strategies will help you stay organized and maintain a steady pace. Being mindful of time will prevent unnecessary stress and ensure that you’re able to answer every question thoroughly and thoughtfully.

Final Review Checklist Before the Exam

As you approach the final moments before an important test, it’s essential to ensure you’ve covered all the necessary material and are ready to perform at your best. This checklist serves as a guide to help you stay organized and confident, making sure no critical areas are overlooked during your last-minute preparations.

Key Areas to Double-Check

To make sure you’re fully prepared, focus on the following aspects during your final review:

Task	Status	Notes
Understand Core Concepts	✔	Review key theories, formulas, and definitions
Practice Problems	✔	Work through sample questions to test your skills
Time Management Strategies	✔	Plan how to allocate time for each section of the test
Check for Understanding of Complex Topics	✔	Ensure clarity on the more difficult subjects

Final Tips for Success

In addition to reviewing the material, consider these last-minute tips to help you feel confident and prepared:

• Stay Calm and Relax: Manage pre-test anxiety by taking deep breaths and staying positive.
• Get Adequate Rest: A well-rested mind performs better, so ensure you get enough sleep the night before.
• Bring Necessary Supplies: Double-check that you have everything you need, such as pens, pencils, erasers, and a calculator if required.

By following this checklist and staying focused, you can maximize your chances for success on the test day.