Geometry Semester 2 Practice Exam Answers
Achieving success in advanced mathematics requires a deep understanding of key concepts and the ability to apply them effectively under timed conditions. This section provides a comprehensive guide to solving complex questions, focusing on essential strategies and techniques that can help you excel in your studies.
Practicing problem-solving skills is crucial to reinforcing your knowledge and building confidence. By working through a variety of challenging exercises, you can improve both your speed and accuracy, ensuring that you are well-prepared for any test or assessment. In this guide, we will cover essential methods for tackling different types of questions, from simple calculations to more intricate proofs.
Furthermore, you will learn how to identify common pitfalls and how to avoid them. Strengthening your logical reasoning and spatial awareness will significantly enhance your ability to interpret and solve mathematical problems effectively. With the right approach and plenty of practice, you will be ready to face any challenge with ease.
Geometry Semester 2 Practice Exam Answers
To succeed in advanced mathematical assessments, it is essential to practice problem-solving skills through a variety of exercises. Reviewing key topics and refining techniques for tackling complex questions will build confidence and improve performance. This section focuses on strategies and methods to approach common types of questions, providing step-by-step insights that will enhance understanding and speed during assessments.
Key Topics to Review
Familiarity with the following areas will be essential for success:
- Understanding shapes, properties, and relationships
- Applying formulas for calculating area, volume, and perimeter
- Mastering problem-solving techniques for word problems
- Working with coordinate-based challenges
- Solving complex proofs and logic-based questions
Effective Strategies for Answering Questions
To navigate through challenging problems, keep these tips in mind:
- Read each problem carefully and identify key information
- Break down complex questions into manageable steps
- Use diagrams and visual aids to clarify relationships
- Check calculations for accuracy before finalizing your response
- Practice solving problems under timed conditions to build speed
By following these strategies and focusing on the key topics, you will be able to approach assessments with confidence and efficiency, achieving the best possible results. Consistent practice is key to mastering these skills and performing well in any mathematical evaluation.
Understanding Key Geometry Concepts
Mastering the foundational principles of mathematics is crucial for solving complex problems efficiently. A clear understanding of fundamental ideas not only simplifies calculations but also strengthens your overall problem-solving skills. In this section, we focus on essential concepts that form the basis of many advanced questions, helping you approach them with confidence.
Shapes and their properties are at the core of this subject. Familiarity with different figures and their characteristics–such as angles, sides, and symmetries–allows you to identify relationships and apply relevant formulas. Whether working with triangles, quadrilaterals, or circles, each shape has its own unique set of rules that can guide you toward the solution.
Another crucial aspect is spatial reasoning. This involves visualizing and manipulating objects in space, which is particularly important when dealing with complex three-dimensional problems. Understanding how shapes relate to each other, both in two and three dimensions, is key to solving more intricate tasks.
Working with formulas is equally important. From calculating area and perimeter to finding the volume of solid objects, knowing how and when to apply the correct formula will speed up your calculations. Each formula is derived from the basic properties of shapes and can often be adapted or combined to suit different types of questions.
By reinforcing these basic concepts and principles, you’ll build a strong foundation that will help you tackle more advanced problems with ease and accuracy.
How to Approach Practice Problems
To effectively solve challenging mathematical questions, it is essential to have a structured approach. Understanding how to break down problems and identify the best methods for finding solutions can drastically improve both your accuracy and speed. This section outlines key strategies to help you efficiently tackle complex problems, step by step.
Breaking Down Complex Questions
Start by carefully reading the problem to fully understand what is being asked. Often, the key to solving a problem lies in recognizing the most important information. Identify the given data, the unknowns, and any relationships between different elements. Then, break the question into smaller, more manageable parts. This process will make it easier to find the right approach and reduce the likelihood of making mistakes.
Choosing the Right Strategy
Once you have broken down the problem, consider the most appropriate method to apply. There are often several different techniques that can be used, so it’s essential to choose one that fits the context of the question. Whether it’s applying formulas, constructing a diagram, or using logical reasoning, selecting the right strategy will help you progress smoothly toward the solution.
Regularly practicing these strategies will help you become more efficient and confident in solving difficult problems. With a methodical approach, you will be able to approach even the most challenging questions with ease.
Step-by-Step Solutions for Geometry Problems
Breaking down complex mathematical problems into manageable steps is an essential skill for success. By following a clear, structured process, you can avoid feeling overwhelmed and increase your chances of arriving at the correct solution. In this section, we outline a detailed approach to solving common types of challenges, ensuring a systematic and logical progression from start to finish.
Steps to Solve a Problem
Here’s a general framework to follow when tackling challenging questions:
- Read and Understand: Carefully read the problem to understand what is being asked. Identify the known values and what needs to be found.
- Draw a Diagram: Whenever possible, create a visual representation of the problem. A diagram can help clarify relationships between different elements.
- Choose the Right Formula: Identify which formulas or mathematical principles are relevant to the problem. Make sure to apply them correctly.
- Perform the Calculations: Carry out the necessary calculations carefully, step by step, and check your work as you go.
- Verify the Solution: After obtaining the answer, review the problem and solution to ensure everything makes sense. Check if the result is reasonable and matches the given data.
Common Problem Types and Solutions
Different types of questions may require varying approaches. Below are some common examples:
- Area and Perimeter: Use the appropriate formulas for each shape (e.g., area of a rectangle = length × width) and substitute the known values to solve.
- Angles and Triangles: Apply angle relationships and theorems such as the sum of angles in a triangle equaling 180°.
- Coordinate-Based Problems: Use the distance and midpoint formulas to solve problems involving points on a plane.
By following this step-by-step approach, you can simplify even the most complex problems, making it easier to achieve accurate solutions. Consistent practice with these methods will build confidence and improve your problem-solving abilities over time.
Common Mistakes in Geometry Exams
When solving complex mathematical problems, it’s easy to make errors that can cost valuable points. Recognizing and avoiding these common mistakes can help improve your performance and reduce the chances of overlooking important details. This section highlights typical pitfalls students face and offers tips on how to avoid them during assessments.
Rushing Through the Problem
One of the most frequent mistakes is rushing through a question without fully understanding it. In the pressure of a timed test, students often overlook key information or skip steps in their calculations. This can lead to incorrect answers or incomplete solutions. To avoid this, take your time to read the question carefully and ensure that you are addressing all parts of the problem. Never skip over details, even if they seem trivial at first glance.
Misapplying Formulas
Another common mistake is using the wrong formula or applying a formula incorrectly. It’s easy to confuse formulas, especially when dealing with similar concepts, such as calculating area or volume for different shapes. To prevent this, always double-check which formula is appropriate for the given problem. Write down the formula first, substitute the values, and ensure that all variables are accounted for properly.
Ignoring Units and Precision
Ignoring units of measurement or rounding too early in your calculations can also lead to errors. Always keep track of the units throughout the problem-solving process and make sure they are consistent. Additionally, avoid rounding numbers too soon–round only at the final step to maintain accuracy.
By being aware of these common mistakes and taking steps to avoid them, you can approach challenges more carefully and increase the likelihood of achieving the correct solution. Remember, accuracy and attention to detail are key components of success.
Geometry Formulas You Should Know
To successfully solve mathematical challenges, it is essential to have a solid grasp of key formulas. These formulas are the foundation for most calculations and will help you quickly and accurately solve problems related to various shapes and figures. In this section, we cover the most important formulas you need to be familiar with when solving problems involving two-dimensional and three-dimensional objects.
Essential Formulas for Two-Dimensional Shapes
Below are the key formulas for common two-dimensional shapes:
| Shape | Formula | Explanation |
|---|---|---|
| Rectangle | Area = length × width | Used to calculate the area of a rectangle, where length and width are the dimensions. |
| Circle | Area = π × radius² | Used to calculate the area of a circle, where π is approximately 3.14159 and radius is the distance from the center to any point on the circle. |
| Triangle | Area = ½ × base × height | Used to calculate the area of a triangle, where base is the length of the bottom side and height is the perpendicular distance from the base to the top vertex. |
| Parallelogram | Area = base × height | Used to calculate the area of a parallelogram, similar to a rectangle but with slanted sides. |
Key Formulas for Three-Dimensional Shapes
For three-dimensional objects, the following formulas are essential:
| Shape | Formula | Explanation |
|---|---|---|
| Cube | Volume = side³ | Used to calculate the volume of a cube, where side represents the length of one edge. |
| Cylinder | Volume = π × radius² × height | Used to calculate the volume of a cylinder, where radius is the distance from the center of the base to its edge and height is the vertical distance between the two bases. |
| Sphere | Volume = ⁴/₃ × π × radius³ | Used to calculate the volume of a sphere, where radius is the distance from the center to any point on the surface. |
| Rectangular Prism | Volume = length × width × height | Used to calculate the volume of a rectangular prism, where length, width, and height are the dimensions of the object. |
By memorizing and practicing the application of these formulas, you’ll be able to approach a wide variety of problems with ease. Understanding when and how to use these formulas is essential to performing well in any related challenges.
Tips for Efficient Problem Solving
Solving mathematical challenges efficiently requires a combination of strategy, focus, and practice. By following a few key tips, you can streamline your approach, reduce errors, and increase your confidence when tackling complex problems. This section provides practical advice on how to approach tasks more effectively, helping you to maximize both your speed and accuracy.
Plan Your Approach
Before diving into any problem, it’s important to take a moment to plan your strategy. A clear plan can prevent you from making unnecessary mistakes and help guide your thought process. Here are a few steps to consider:
- Understand the Problem: Read the question carefully and identify the known information and what needs to be solved.
- Break It Down: Divide the problem into smaller, manageable parts to make it less overwhelming.
- Choose the Right Method: Select the most efficient approach, whether it’s using a formula, diagram, or logical reasoning.
Stay Organized and Methodical
Staying organized is key to solving problems accurately. Keeping track of steps and ensuring each calculation is clearly laid out helps prevent confusion. Consider these tips:
- Show Your Work: Write down each step of the solution clearly to ensure you don’t skip any details and to make it easier to spot errors later.
- Check Units: Pay close attention to units of measurement and convert them as needed. This ensures your calculations are consistent and correct.
- Review Your Work: After solving, always take a moment to review your calculations and answer. Checking for minor mistakes can save you from losing marks.
Practice Regularly
Finally, one of the best ways to improve problem-solving skills is consistent practice. The more you engage with various types of challenges, the more familiar you will become with common patterns and strategies. This will naturally increase both your efficiency and accuracy over time.
By implementing these tips, you can approach each problem with a clearer mindset and a more efficient strategy, helping you to work smarter, not harder.
How to Manage Time During the Exam
Effective time management is crucial during any assessment, as it allows you to complete all tasks without feeling rushed or missing key details. By using smart strategies, you can maximize your performance and ensure that you have enough time to address each problem with the attention it deserves. This section will provide useful tips on how to manage your time efficiently while under pressure.
Plan Ahead
Before you begin solving problems, take a few moments to assess the time available and plan how you will allocate it across the different sections. Here are some steps to help you plan effectively:
- Scan the Entire Paper: Quickly review all the questions to get an overview. This will help you gauge how much time each section might take.
- Prioritize Easier Questions: Tackle the questions you find easiest first. This will help you gain momentum and boost your confidence early on.
- Allocate Time Per Section: Estimate how much time you should spend on each section based on its difficulty. Keep track of the time as you work through the problems.
Maintain a Steady Pace
It’s easy to get caught up on a particularly challenging question, but spending too much time on one problem can cause you to run out of time for others. Here are some strategies to maintain a steady pace:
- Set Time Limits: Give yourself a fixed amount of time for each problem. If you get stuck, move on to the next one and return later if needed.
- Use a Timer: Keep track of time using a watch or timer. This can help you stay aware of how much time you have left.
- Don’t Overthink: Trust your first instinct and move forward. Overthinking a problem can waste time and increase stress.
By applying these time management techniques, you can approach your tasks more efficiently, ensuring that you complete all sections of the assessment in a timely and organized manner. Managing your time effectively helps you remain calm and focused, allowing you to perform at your best.
Reviewing Common Geometric Theorems
Understanding fundamental principles is essential for solving problems in any field of mathematics. Recognizing and applying key theorems can significantly simplify complex tasks, providing a solid foundation for tackling a variety of questions. This section focuses on some of the most important theorems that often appear in assessments, ensuring that you are prepared to handle them effectively.
Key Theorems to Remember
There are several theorems that frequently come up in problems involving shapes, angles, and distances. Having a clear grasp of these principles will allow you to quickly identify the best approach for each question. Below are some of the core theorems:
| Theorem | Description |
|---|---|
| Pythagorean Theorem | Relates the sides of a right triangle, stating that the square of the hypotenuse is equal to the sum of the squares of the other two sides. |
| Triangle Sum Theorem | States that the sum of the interior angles of any triangle is always 180 degrees. |
| Angle Bisector Theorem | States that the angle bisector of an angle in a triangle divides the opposite side into two segments that are proportional to the other two sides of the triangle. |
| Congruence Theorems (SSS, SAS, ASA) | Describe conditions under which two triangles are congruent based on the equality of their sides and angles. |
| Exterior Angle Theorem | States that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. |
How to Apply These Theorems
Once you are familiar with the key theorems, the next step is understanding when and how to apply them in problem-solving scenarios. Here are some strategies:
- Identify Key Information: Carefully read each problem to identify which theorems are relevant. Look for right angles, parallel lines, or other clues that suggest a particular theorem.
- Draw Diagrams: A visual representation can help you see the relationships between elements more clearly, making it easier to apply the correct theorem.
- Practice Regularly: The more you apply these theorems, the more comfortable and intuitive they will become, allowing you to solve problems quickly and efficiently.
Mastering these theorems and understanding how to use them in different contexts will greatly improve your problem-solving skills. With continued practice and familiarity, you will be able to tackle even the most challenging tasks with confidence.
Solving Word Problems in Geometry

Word problems can be challenging, especially when they involve abstract concepts and complex relationships. However, breaking down these problems step by step can make them more manageable. The key to solving these problems is to translate the information given in the text into mathematical relationships and then apply the appropriate methods to find the solution. This section will guide you through effective strategies to approach word problems, ensuring that you can tackle them confidently.
Steps to Solve Word Problems
Approaching word problems requires a systematic process. Following these steps can help simplify the task:
- Read the Problem Carefully: Ensure you understand what is being asked. Identify the key pieces of information and the relationships between them.
- Draw a Diagram: If the problem involves shapes or figures, sketching a diagram can help visualize the situation. Label all the known and unknown values clearly.
- Translate Words into Equations: Convert the information given in the problem into mathematical expressions or equations. This will make it easier to solve.
- Apply Relevant Formulas: Once you have set up the equations, use the appropriate formulas or theorems to find the unknowns.
- Check Your Solution: After solving, verify that the result makes sense in the context of the problem.
Example Problem

Let’s consider an example to better understand how to apply these steps:
| Problem | Solution |
|---|---|
| Find the length of the diagonal of a rectangle with sides of 3 cm and 4 cm. |
|
By following this step-by-step process, you can successfully solve word problems. Practice regularly to improve your ability to quickly identify relevant information, set up equations, and apply the appropriate methods.
How to Check Your Exam Answers
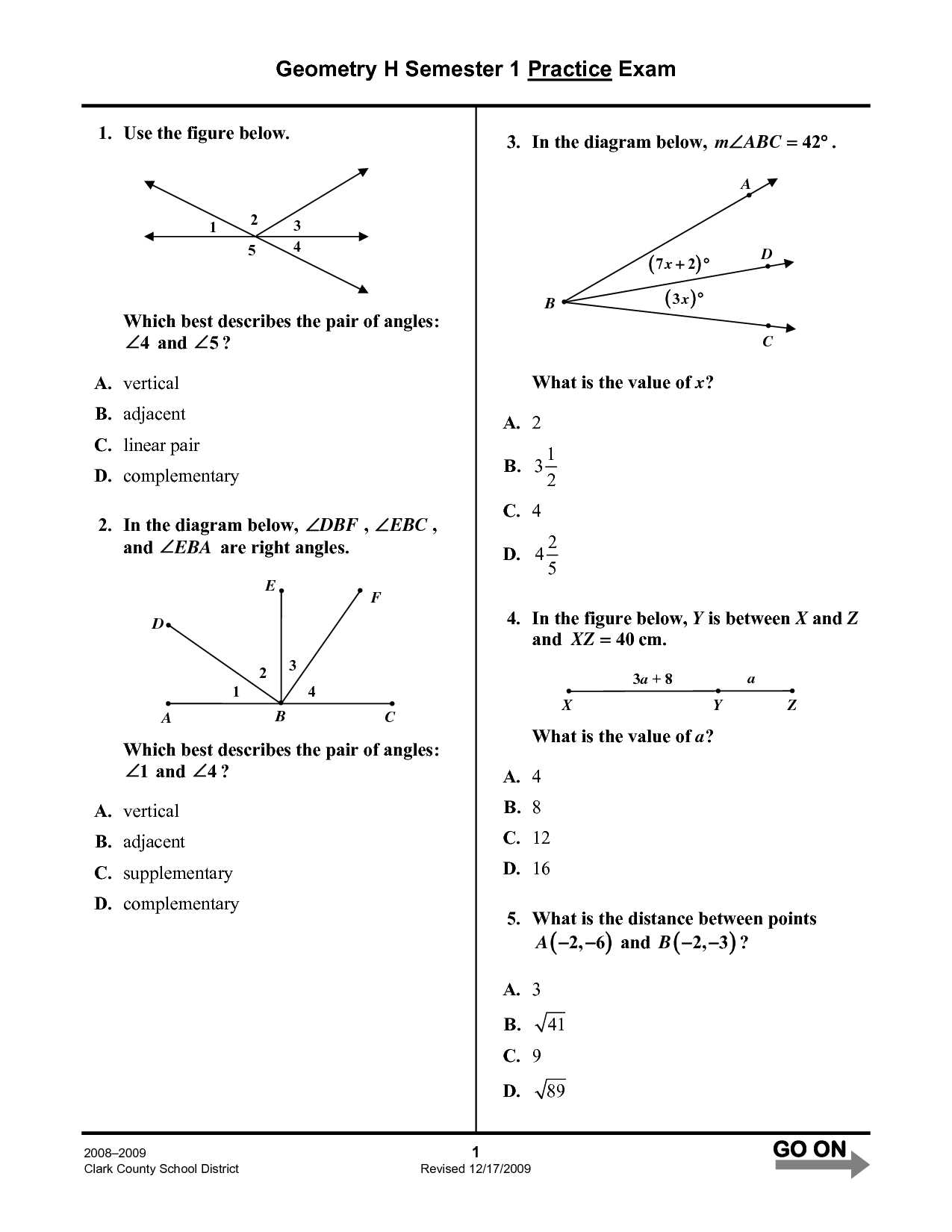
After completing a test, it’s essential to thoroughly review your solutions to ensure accuracy and identify any possible errors. This process not only boosts confidence but also helps in improving problem-solving skills for future assessments. Checking your work methodically can uncover mistakes, validate correct responses, and increase your overall performance. In this section, we’ll explore practical steps to effectively review your work.
Start by going through each question carefully, ensuring that you understand what was asked and that your answers align with the problem requirements. Double-check the math or logic used to solve each problem, and confirm that you didn’t overlook any important steps. Additionally, reviewing your calculations or applying the reverse method can help catch errors that may not be obvious at first glance.
Here are some strategies to check your work:
- Review Step-by-Step: Go through each solution step-by-step, ensuring that all necessary operations and procedures were followed correctly.
- Cross-Check with Formulas: Verify that the correct formulas or methods were used for each type of problem. Misapplying formulas is a common mistake.
- Check Units and Labels: Ensure that all units are correctly applied and that all values are properly labeled. Units are often overlooked but are crucial to understanding the result.
- Recalculate Where Necessary: If a particular answer seems off, recalculating can often help identify errors made in the process. It’s important to verify complex calculations.
- Use the Reverse Method: For certain problems, applying the reverse method or plugging the solution back into the original question can confirm that your answer is correct.
By following these steps and taking the time to check your work carefully, you can ensure a more accurate set of solutions and reduce the risk of mistakes during assessments.
Working with Geometric Proofs
Working with logical arguments is an essential skill in mathematics. When solving problems that require a formal justification, it’s important to follow a clear, structured approach. The goal is to prove a statement true by using previously established facts, definitions, and logical reasoning. This process involves making connections between different concepts and applying theorems and properties in a systematic way. In this section, we will explore strategies for approaching and solving problems that involve formal reasoning.
To approach a proof successfully, consider the following steps:
- Understand the Problem: Carefully read the problem to identify what needs to be proved. Break it down into smaller parts and understand the given information.
- List Known Facts: Make a list of all the known information, theorems, and definitions that may be useful in the proof. This provides a foundation for the reasoning.
- Choose the Appropriate Method: Decide on a method for the proof, such as direct proof, proof by contradiction, or proof by induction, depending on the nature of the statement.
- Build the Argument: Begin connecting the known facts logically. Work step-by-step, making sure each conclusion follows from the previous one. Use appropriate definitions and theorems to support your reasoning.
- Verify Each Step: Ensure that each step of your argument is valid and necessary. Avoid making jumps in reasoning that can weaken the proof.
Here are some common strategies used in solving proofs:
- Direct Proof: This involves starting with the given information and applying logical steps to reach the desired conclusion.
- Proof by Contradiction: Assume the opposite of what you are trying to prove and show that it leads to a contradiction, thus proving the original statement true.
- Proof by Induction: Used for proving statements that involve natural numbers. It involves proving the base case and then showing that if the statement holds for one case, it holds for the next.
Proofs can seem challenging at first, but with practice, the process becomes clearer. By focusing on logical structure and carefully verifying each step, you can build solid, well-supported arguments that establish the truth of a given statement.
Test Review for Mathematical Concepts
Reviewing a set of problems after completing a test is an essential step in reinforcing understanding and improving performance. By carefully analyzing each question, you can identify strengths and areas for improvement. This process involves revisiting problems, breaking down each step, and understanding the rationale behind the solutions. Through effective review, you can enhance your problem-solving skills and be better prepared for future assessments.
During a review session, focus on the following steps to maximize learning:
- Analyze Mistakes: Look at each mistake carefully and understand why it happened. Was it due to a misinterpretation of the question, a calculation error, or incorrect application of a concept?
- Clarify Concepts: For problems you struggled with, review the underlying concepts. Ensure you fully understand the methods and formulas needed to solve similar problems in the future.
- Practice Similar Problems: Reattempt problems with similar concepts to reinforce the methods you’ve learned. This helps solidify your understanding and makes you more comfortable applying the concepts in different contexts.
- Identify Patterns: Identify recurring types of problems. By recognizing common problem types, you can anticipate the methods and approaches you need to use in future tests.
- Manage Time Effectively: During review, consider how long you took on each question. Did time pressure contribute to mistakes? Practicing under time constraints can help with pacing in future assessments.
By actively reviewing your test, you can strengthen your knowledge, gain insight into your problem-solving approach, and build confidence for the next challenge. Taking the time to reflect on each part of the process not only improves your ability to solve similar problems but also prepares you to think more critically and strategically in future situations.
Mastering Coordinate Geometry for Exams
To succeed in problems involving points and lines on a coordinate plane, it’s essential to have a strong grasp of key concepts and techniques. Mastering this area of mathematics requires understanding how to manipulate equations, calculate distances, slopes, and midpoints, as well as how to interpret and solve geometric relationships in a two-dimensional plane. With these skills, you can confidently tackle questions that require applying algebraic methods to geometric shapes and solve complex problems efficiently.
Key Concepts to Focus On
Understanding the following core concepts is crucial for performing well:
- Distance Formula: This formula helps you find the length between two points. Knowing how to derive and apply it quickly is vital for solving many problems in coordinate geometry.
- Slope of a Line: Calculating the slope is necessary to determine whether two lines are parallel or perpendicular. Understanding its application in different contexts is key.
- Equation of a Line: Knowing how to write the equation of a line in slope-intercept form (y = mx + b) or point-slope form is critical for expressing relationships between points and lines.
- Midpoint Formula: The midpoint formula helps you find the center of a line segment. This skill is often tested in problems involving symmetry or geometric construction.
Effective Techniques for Success
Here are some tips to help you master coordinate geometry:
- Visualize the Problem: Whenever possible, draw a diagram of the situation to better understand the relationships between points, lines, and shapes. Visualizing the problem often simplifies the process of solving it.
- Practice with Real-World Applications: Coordinate geometry is widely used in areas such as engineering, architecture, and computer science. Studying real-world examples can deepen your understanding and make abstract concepts more relatable.
- Use Systematic Methods: Break down each problem into manageable steps, and avoid skipping important parts of the solution. Writing out each step clearly helps ensure that you don’t miss anything.
By mastering these concepts and techniques, you’ll build the confidence needed to tackle coordinate geometry problems with ease. Consistent practice and a methodical approach will help you perform at your best and solve problems accurately during any assessment.
Best Resources for Geometry Exam Preparation
When preparing for assessments in mathematical topics, having access to high-quality resources is crucial for success. Whether you’re studying fundamental concepts or reviewing complex problems, using a variety of tools can help reinforce your understanding and boost your performance. The right materials not only provide practice but also clarify difficult concepts and offer step-by-step explanations to solidify your knowledge.
Here are some of the best resources to consider while getting ready for your upcoming test:
Online Learning Platforms
Websites and platforms that offer video tutorials and interactive lessons can be particularly helpful. These resources allow you to work at your own pace and revisit challenging sections as needed. Popular platforms include:
- Khan Academy – Offers detailed video lessons and practice problems on various mathematical topics.
- Coursera – Features courses from top universities and institutions, allowing you to learn directly from experts.
- edX – Provides courses that cover both basic and advanced topics with a focus on application and problem-solving.
Books and Study Guides
Traditional study guides and textbooks still hold great value. These resources often provide more in-depth explanations of concepts and include plenty of practice problems to test your skills. Here are some highly recommended books:
- “Algebra and Trigonometry” by Robert F. Blitzer – A comprehensive textbook that covers essential concepts and includes practice questions.
- “The Art of Problem Solving” by Richard Rusczyk – A series designed to teach problem-solving techniques in mathematics.
- “For Dummies” Series – Offers easy-to-understand explanations and practice problems in many subjects.
Incorporating these resources into your study routine can make a significant difference in your preparation, helping you approach problems with confidence and sharpen your skills for the test ahead.