Physics Classroom Free Body Diagrams Answers Guide

Understanding how forces interact within various systems is crucial to mastering the principles of mechanics. In many scenarios, identifying these forces and their effects on objects is essential for solving complex problems. This process involves representing forces visually and analyzing their relationships to predict motion and equilibrium.

By mastering the techniques of force representation and interpretation, students can gain valuable insights into how different factors influence an object’s state. Whether dealing with static or dynamic conditions, a solid grasp of these concepts can significantly improve problem-solving skills and deepen understanding of motion and force interactions.

Effective problem-solving starts with clear visualization, allowing for a structured approach to complex challenges. Knowing how to break down forces and their effects is an essential skill that enhances both theoretical and practical comprehension of mechanical systems.

Understanding Free Body Diagrams in Physics

To analyze motion and forces acting upon an object, it is crucial to have a clear method of representing those forces in a simplified manner. Visual tools are essential for breaking down complex physical scenarios into understandable parts, allowing for easier calculations and predictions. These tools help isolate individual forces while providing a clear perspective on their directions and magnitudes.

The Role of Force Representation

Proper representation of forces is key in understanding how different interactions affect an object. By illustrating all the forces at play, one can better analyze their contributions to the overall motion or stability of the system. Such depictions are particularly helpful in problems involving motion, tension, friction, and external influences.

How These Visual Tools Help Solve Problems

Once forces are represented visually, it becomes much easier to apply mathematical principles to solve for unknown quantities. Whether dealing with equilibrium situations or calculating acceleration, this method allows for a step-by-step breakdown that simplifies complex concepts into manageable tasks.

What Are Free Body Diagrams?

When studying the forces acting on an object, a key approach is to represent those forces in a visual format. This helps in isolating the object from its surroundings and focusing solely on the interactions influencing its motion or stability. By identifying and illustrating all forces, it becomes possible to predict how an object will behave under various conditions.

Visualizing Forces in a Simple Form

These representations typically consist of an object shown as a simple shape, with arrows indicating the direction and magnitude of forces applied to it. The arrows make it easier to see the relationships between different forces and how they combine to affect the object’s movement or lack thereof.

Applications and Importance

Such visualizations are used in a variety of problems, from calculating acceleration to understanding static equilibrium. They offer a systematic way to analyze complex systems by breaking them down into manageable components, making problem-solving more straightforward and effective.

Importance of Free Body Diagrams in Physics

Representing forces visually plays a pivotal role in understanding how objects interact within a system. By isolating forces acting on an object, these tools help reveal the underlying dynamics that govern motion and equilibrium. Without this visual breakdown, complex physical phenomena would be significantly harder to analyze and solve.

Key Benefits of Force Representations

• Clarity: Simplifying complex interactions into clear, visual components makes it easier to identify the impact of individual forces.
• Problem-Solving: These tools provide a structured approach to solving problems by clearly illustrating how forces combine and affect motion.
• Understanding Motion: By focusing on the forces acting on an object, one can better understand its acceleration, velocity, and the overall dynamics of the system.

Practical Applications in Real-World Problems

1. Engineering: Used in designing structures, machinery, and vehicles by analyzing forces acting on components.
2. Structural Analysis: Helps engineers calculate stresses and strains in materials to prevent failure.
3. Aerospace: Used to understand and predict the behavior of objects under various aerodynamic forces.

How to Draw Free Body Diagrams

Creating a visual representation of the forces acting on an object requires a systematic approach. The goal is to isolate the object, identify all the forces applied to it, and represent them in a clear and understandable way. This process simplifies complex scenarios and provides a foundation for solving problems involving motion and equilibrium.

Steps to Draw Force Representations

1. Step 1: Choose the object of interest and represent it as a simple shape, often a box or circle, depending on the context.
2. Step 2: Identify all forces acting on the object, including gravity, friction, normal force, tension, and applied forces.
3. Step 3: Draw arrows to represent each force. The length of the arrow indicates the force’s magnitude, while the direction shows the force’s action.
4. Step 4: Label each force clearly to avoid confusion and ensure accuracy in calculations.
5. Step 5: Review the diagram to ensure that all forces have been accounted for and are accurately depicted.

Example of a Force Representation

Force	Symbol	Direction
Gravitational Force	Fg	Downwards (toward the center of the Earth)
Normal Force	Fn	Upwards (perpendicular to the surface)
Frictional Force	Ff	Opposite to the direction of motion
Applied Force	Fa	In the direction of motion (applied externally)

Key Forces in Free Body Diagrams

When analyzing the interactions that affect an object, several forces must be considered to understand the system’s behavior. These forces play a critical role in determining how an object will move or stay in equilibrium. Each force has specific characteristics and directions, and understanding them is essential for accurately solving physical problems.

Common Forces in Force Representations

There are several key forces that commonly appear in these visual tools. These include both contact and non-contact forces, each contributing to the object’s overall motion or state of rest.

Force	Symbol	Type	Direction
Gravitational Force	Fg	Non-contact	Downward, towards the center of the Earth
Normal Force	Fn	Contact	Perpendicular to the surface (upwards)
Frictional Force	Ff	Contact	Opposite to the direction of motion
Tension Force	Ft	Contact	Along the direction of the rope or cable
Applied Force	Fa	Contact	In the direction of the applied force

Understanding the Interactions Between Forces

Each force affects the object in specific ways, and their combination determines the object’s overall behavior. For example, the balance between gravitational and normal forces is key in situations where an object is at rest. On the other hand, the interaction between applied and frictional forces governs motion. Correctly identifying and representing these forces allows for accurate predictions of how an object will respond to external conditions.

Common Mistakes in Free Body Diagrams

When creating visual representations of forces acting on an object, it’s easy to make mistakes that can lead to inaccurate conclusions. These errors can stem from improper identification of forces, incorrect directions, or missing forces entirely. Recognizing and avoiding these common mistakes is crucial for accurate problem-solving and analysis.

Common Errors to Watch For

• Forgetting to Include All Forces: One of the most common mistakes is overlooking a force acting on the object. Always check for forces like friction, tension, or normal force that may not be immediately obvious.
• Incorrect Force Directions: Forces must be represented with arrows pointing in the correct direction. For example, gravitational force always points downward, while normal force acts perpendicular to the surface.
• Mislabeling Forces: Clear labeling is essential. Confusing forces like tension and applied force can lead to incorrect calculations and interpretations.
• Incorrect Magnitude Representation: The length of the force arrows should correspond to the relative magnitudes of the forces. Arrows that are too long or short can distort the understanding of the force balance.
• Not Considering the Object’s Orientation: The orientation of the object in the diagram should reflect its actual position and motion. A rotated object may affect the direction of forces, especially friction or applied forces.

How to Avoid These Mistakes

1. Double-check all forces: Ensure that all forces acting on the object are included, even those that may not be immediately obvious, like air resistance or tension from surrounding objects.
2. Ensure correct directions: Revisit the problem to verify that each force is acting in the correct direction according to the scenario.
3. Label forces clearly: Use consistent labels for each force and avoid confusion by clearly distinguishing between forces that are applied, reactive, or due to gravity.
4. Accurately scale arrows: Represent the magnitude of each force correctly by adjusting the length of the arrows according to their relative size.
5. Align the object’s orientation: Make sure the object’s position and rotation in the diagram reflect its actual orientation in the scenario being analyzed.

Analyzing Forces in Motion

Understanding how forces interact to cause motion is fundamental to solving many physical problems. When an object is in motion, various forces, such as gravity, friction, and applied forces, influence its velocity and direction. By analyzing these forces, it becomes possible to predict the object’s behavior, whether it’s accelerating, decelerating, or maintaining a constant speed.

Key Principles of Motion Analysis

In any motion-related problem, it’s essential to break down the forces acting on the object and understand how they interact. The overall effect on the object’s motion depends on the combination of forces in action. For example, the net force determines whether an object will accelerate, decelerate, or maintain a steady velocity.

Applying Newton’s Second Law

Newton’s second law of motion, F = ma (force equals mass times acceleration), is crucial when analyzing forces in motion. This equation allows you to calculate the acceleration of an object when the net force and mass are known. By identifying all the forces involved and applying this law, you can determine how the object will move over time.

Free Body Diagrams and Newton’s Laws

Visual representations of forces are a key tool for applying Newton’s laws to real-world problems. These tools help simplify the analysis of complex systems by isolating an object and focusing solely on the forces acting upon it. By understanding how forces interact, one can apply Newton’s three laws of motion to predict and explain the object’s behavior.

Newton’s laws provide the foundation for understanding the relationship between forces and motion. In particular, the second law, F = ma, directly relates the net force acting on an object to its acceleration. Using force representations, this relationship can be applied more easily, as the forces are clearly depicted in a manner that facilitates calculation and interpretation. Whether solving for acceleration, velocity, or equilibrium, these laws offer the necessary framework for accurate predictions.

Friction and Free Body Diagrams

When analyzing the motion of an object, friction plays a significant role in resisting movement. This force acts in the opposite direction of the object’s motion and is essential to consider in many real-world scenarios. In visual representations, friction is represented as a force opposing the movement, and accurately including it is crucial for determining the net force and predicting the object’s behavior.

Understanding the Role of Friction

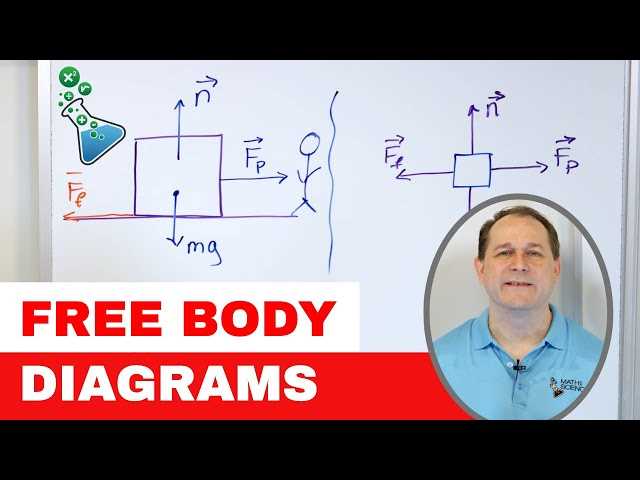
Friction can be divided into two main types: static and kinetic. Static friction resists the initiation of motion, while kinetic friction acts once the object is already moving. Both types need to be accounted for in the analysis of forces, as they affect how easily an object starts moving or slows down.

• Static Friction: Occurs when an object is at rest and prevents it from moving until a certain threshold force is applied.
• Kinetic Friction: Acts on an object in motion and generally has a lower magnitude than static friction.

Representing Friction in Force Analysis

In a force representation, friction is drawn as an arrow opposite to the direction of motion. Its magnitude depends on the coefficient of friction and the normal force acting on the object. The frictional force is calculated using the equation:

Ff = μ * Fn

Where μ is the coefficient of friction, and F_n is the normal force. It’s important to note that friction only affects objects in contact with a surface, and its magnitude can change depending on the nature of the surface and the object’s motion.

Free Body Diagrams for Circular Motion

When an object moves in a circular path, it experiences forces that continuously change direction, even if the object’s speed remains constant. This type of motion requires careful analysis of the forces acting on the object, particularly the forces that contribute to the centripetal acceleration. Understanding these forces is essential for predicting the behavior of the object and for calculating quantities like velocity, acceleration, and tension in circular motion problems.

Forces Involved in Circular Motion

In circular motion, several forces come into play, but the most significant is the centripetal force, which is responsible for keeping the object on its curved path. This force is directed towards the center of the circle and prevents the object from moving off in a straight line due to inertia. Other forces such as gravitational, normal, and frictional forces may also act depending on the context of the problem.

• Centripetal Force: Always directed towards the center of the circle, this force is responsible for changing the direction of the object’s velocity.
• Gravitational Force: If the object is moving in a vertical circle, gravity will affect the motion, especially at the highest and lowest points.
• Tension: In cases like a ball on a string or an object attached to a rotating arm, tension in the string or rod provides the necessary force for circular motion.

Representing Circular Motion Forces

When drawing a force representation for circular motion, it’s important to include both the centripetal force and any other relevant forces. The centripetal force is typically shown as an arrow pointing toward the center of the circle. In cases where the motion is vertical or the object is influenced by other forces, such as gravity or tension, those forces are drawn in their respective directions.

For example, in a scenario where a car moves in a circular path on a flat road, friction provides the centripetal force, and gravity acts downward, while the normal force from the road surface acts upward. Properly representing all the forces involved is essential for calculating the net force and determining the object’s acceleration and velocity in circular motion.

Work and Energy in Free Body Diagrams

When analyzing the forces acting on an object, it’s essential to consider the work done by those forces and how they affect the object’s energy. The relationship between work and energy plays a significant role in understanding how an object moves and interacts with its environment. In force representations, work and energy are linked through the concept of force acting over a distance, resulting in a change in kinetic or potential energy.

Understanding Work in Force Analysis

Work is done when a force causes an object to move in the direction of the applied force. The amount of work depends on the magnitude of the force, the distance the object moves, and the angle between the direction of the force and the object’s motion. In force representations, the work done by each force can be calculated using the equation:

W = F * d * cos(θ)

Where W is the work done, F is the magnitude of the force, d is the distance moved, and θ is the angle between the force and the direction of motion. The work-energy principle tells us that the work done on an object is equal to the change in its energy.

Energy Considerations in Force Representations

In force analysis, energy is often discussed in terms of kinetic energy (the energy of motion) and potential energy (the energy stored due to position). When forces are applied to an object, they can either increase or decrease its energy. For example, when an object is lifted, work is done against gravity, increasing its potential energy. Conversely, when an object is set into motion, work is done to increase its kinetic energy.

• Kinetic Energy: The energy an object possesses due to its motion, calculated as KE = 1/2 * m * v², where m is the object’s mass and v is its velocity.
• Potential Energy: The energy stored in an object due to its position, commonly calculated as PE = m * g * h, where m is the mass, g is the gravitational acceleration, and h is the height above a reference point.

By considering the work done by forces and the resulting energy changes, a more complete understanding of an object’s motion can be gained. In practical terms, force representations help visualize how energy flows through a system and how different forces contribute to the overall energy balance, whether it’s kinetic energy, potential energy, or a combination of both.

Free Body Diagrams in Equilibrium Problems

When solving problems related to objects at rest or moving with constant velocity, the concept of equilibrium plays a key role. In these scenarios, the forces acting on the object must be balanced, meaning the net force is zero. Using visual representations of the forces helps simplify the problem and ensure all forces are properly accounted for, making it easier to solve for unknown quantities such as tension, friction, or force components.

Types of Equilibrium

There are two main types of equilibrium to consider: static equilibrium and dynamic equilibrium. Both types involve the idea that the sum of all forces acting on an object must be zero, but the conditions under which they apply differ slightly.

• Static Equilibrium: Occurs when an object is at rest, and the sum of forces and the sum of torques (moments) are both zero. This is the most common scenario in which force balance is used.
• Dynamic Equilibrium: Happens when an object is moving at a constant velocity, and the sum of forces still equals zero. The object is not accelerating, but it may be in motion.

Analyzing Equilibrium with Force Representations

In equilibrium problems, force representations help illustrate the forces acting on an object and their directions. The key is to ensure that the vector sum of all forces equals zero, both in the horizontal and vertical directions. This often involves breaking down forces into their components and applying the equilibrium conditions to solve for unknown values.

Force	Horizontal Component	Vertical Component
Gravitational Force (Weight)	0	mg
Tension Force	T * cos(θ)	T * sin(θ)
Friction Force	Ffriction	0

In the table above, the forces acting on an object in equilibrium are broken down into their horizontal and vertical components. By applying Newton’s first law (ΣF = 0) in both directions, you can solve for unknown forces or components, ensuring the object remains in equilibrium.

Once the force components are calculated, they can be substituted back into the equilibrium equations. For example, if an object is suspended by a rope, the vertical components of tension must balance the gravitational force, and any horizontal forces should also cancel out. Using these principles, equilibrium problems can be systematically solved, providing clarity on the forces acting on the object.

Gravitational Forces in Diagrams

When analyzing the forces acting on an object, gravitational forces play a central role in many scenarios, particularly in objects near the Earth’s surface. These forces pull objects downward and must be accurately represented in force analysis to fully understand the object’s motion. In visual force representations, gravity is usually depicted as a downward arrow, indicating its direction and magnitude.

Representing Gravitational Force

Gravitational force is directly proportional to an object’s mass and the acceleration due to gravity. In most cases, this force can be calculated using the formula:

Fg = m * g

Where F_g is the gravitational force, m is the object’s mass, and g is the acceleration due to gravity (approximately 9.8 m/s² on Earth). This force acts vertically downward, and in diagrams, it is often the most significant force to consider when analyzing the vertical equilibrium of an object.

Common Scenarios Involving Gravitational Force

Gravitational force is essential in various contexts, such as objects in free fall, on inclined planes, or hanging from a rope. Below are some common scenarios where gravitational forces are crucial:

• Objects at Rest: When an object is resting on a surface, gravitational force is balanced by an upward normal force.
• Free Fall: In free fall, the only force acting on an object is gravity, resulting in acceleration toward the ground.
• Inclined Planes: When an object is on an inclined surface, gravitational force must be broken into components to analyze motion.
• Suspended Objects: For objects hanging vertically, gravitational force is balanced by the tension in the rope or cable.

In each of these cases, accurately representing gravitational force is key to understanding the overall force balance and solving for unknown quantities such as acceleration, tension, or friction. By including this force in your visual analysis, you ensure a more accurate and complete solution to the problem at hand.

Application of Free Body Diagrams in Real Life

In everyday life, understanding the forces acting on objects is essential for ensuring safety, optimizing performance, and solving practical problems. Whether designing a bridge, analyzing the motion of a car, or even understanding the forces on a person lifting an object, visualizing these forces helps make better decisions and predictions. By representing all forces acting on an object, we can simplify complex systems and solve for unknowns, such as tension, friction, or acceleration.

One of the key benefits of force analysis is its broad application across various fields. Engineers, architects, and even athletes use these visual representations to model forces and optimize performance in real-world situations. Below are some examples of how this method is used in practical applications.

• Engineering and Construction: Structural engineers use force analysis to design buildings, bridges, and other structures that can withstand environmental forces like wind, gravity, and seismic activity.
• Vehicle Motion: In automotive engineering, force diagrams help engineers understand how forces like friction, air resistance, and engine power affect the motion of vehicles. This analysis helps improve fuel efficiency and vehicle safety.
• Sports Science: In athletics, coaches and athletes analyze the forces during running, jumping, or lifting to improve performance and prevent injury. For example, understanding the forces acting on a gymnast during a vault can help improve technique.
• Aerospace and Aviation: Aircraft and spacecraft designers rely on force analysis to understand how lift, drag, thrust, and gravity interact to ensure safe and efficient flight.

In each of these examples, the principles of force analysis help predict outcomes and ensure that designs are functional and safe. By using these models, professionals can make informed decisions and apply corrective measures when needed. As technology advances, the use of these visual tools becomes even more crucial for developing innovative solutions across industries.

Using Free Body Diagrams for Problem Solving

In any situation involving forces, breaking down the problem into visual components can significantly simplify the process of finding solutions. By representing the forces acting on an object, it becomes easier to apply fundamental principles such as Newton’s laws to calculate unknowns like acceleration, tension, or friction. This approach turns complex scenarios into manageable steps, making it possible to systematically solve for the desired quantities.

When solving problems, a clear step-by-step process is essential. The process typically begins by identifying all the forces acting on an object, followed by creating a simplified representation of those forces. Once the forces are represented, the next step is applying the relevant equations of motion, often involving force balance or energy principles, to find unknown values. Below is an overview of how to use force models effectively in problem-solving.

Steps for Problem Solving

• Step 1: Identify All Forces: Consider all forces acting on the object, such as gravity, friction, normal force, and any applied forces. Represent each of these forces with vectors in the appropriate directions.
• Step 2: Break Forces into Components: For forces at angles, decompose them into horizontal and vertical components. This simplifies calculations and helps ensure that each force is considered correctly.
• Step 3: Apply Equilibrium Conditions: If the object is at rest or moving at constant velocity, use the equilibrium condition (sum of forces equals zero) to set up equations for the unknowns. If the object is accelerating, apply Newton’s second law (sum of forces equals mass times acceleration).
• Step 4: Solve the Equations: Use the information from the previous steps to solve for the unknown quantities, such as tension, force, or acceleration.

Example Problem

Consider an object being pulled by a rope on a flat surface. To solve for the tension in the rope, we would follow these steps:

• Identify the Forces: The forces acting on the object are the applied force (tension in the rope), the normal force, friction, and the gravitational force.
• Break Forces into Components: The applied force may have both horizontal and vertical components, depending on the angle at which the rope is being pulled.
• Set Up the Equations: Use Newton’s second law for both the horizontal and vertical components. For example, in the horizontal direction, the net force is the applied force minus friction, and in the vertical direction, the normal force and gravitational force are balanced.
• Solve for the Unknowns: Use the equations to calculate the tension in the rope and check for consistency.

By following this approach, complex problems become easier to handle, and the accuracy of solutions is increased. Force models not only aid in calculation but also enhance conceptual understanding of how forces interact in real-world scenarios.

Understanding Tension Forces in Diagrams

Tension forces arise when an object is being pulled or stretched by a rope, cable, or string. These forces are directed along the length of the connector, acting to resist deformation and maintain equilibrium. When visualizing such forces, it is important to correctly represent the direction and magnitude of the tension, as they are critical for solving problems related to forces in motion, structural integrity, and dynamic systems. Tension is always directed away from the object, along the line of the rope or string, and it varies depending on factors like the angle of application and the object’s mass.

Key Characteristics of Tension Forces

Tension forces have specific characteristics that distinguish them from other forces like gravity or normal force. Some of these key features include:

• Direction: Tension always pulls along the line of the rope, string, or cable, in the direction away from the object being connected.
• Magnitude: The magnitude of the tension force depends on the force applied, the angle at which the rope or cable is pulled, and the system’s overall dynamics.
• Equilibrium Conditions: In systems where tension is present, the sum of forces must be balanced. For example, if an object is hanging from a rope, the upward tension force is balanced by the downward gravitational force.

Applications of Tension in Real-World Scenarios

Tension is an essential force in many practical situations. Some common examples where tension forces are significant include:

• Elevator Cables: The tension in the cables of an elevator must be carefully controlled to lift the elevator and its passengers safely.
• Suspended Objects: When an object is hanging from a rope or cable, the tension is responsible for holding the object in place and counteracting its weight.
• Pulley Systems: In systems involving pulleys, the tension force in the ropes is critical for determining the mechanical advantage and efficiency of the system.

Accurately modeling tension forces in diagrams is essential for understanding the dynamics of objects in motion or at rest. These forces help ensure proper system functionality and are indispensable in engineering and design tasks where safety and performance are critical.

Free Body Diagrams for Inclined Planes

When an object is placed on an inclined surface, the forces acting on it become more complex due to the angle of the surface. Unlike horizontal surfaces, an inclined plane introduces both vertical and horizontal force components that must be carefully considered in force models. By breaking down these forces into components, we can solve for unknowns such as the object’s acceleration, frictional force, or the force required to move it along the slope.

Key Forces on an Inclined Plane

Several forces act on an object placed on an inclined surface, and understanding their directions and magnitudes is essential for accurate analysis:

• Gravitational Force: The weight of the object, which acts vertically downward. This force can be decomposed into two components: one parallel to the plane (affecting the object’s motion along the slope) and one perpendicular to the plane (affecting the normal force).
• Normal Force: The support force exerted by the inclined surface, perpendicular to the plane. This force balances the perpendicular component of gravity and prevents the object from sinking into the surface.
• Frictional Force: The force opposing the motion of the object. It acts parallel to the surface and depends on the normal force and the coefficient of friction between the object and the plane.
• Applied Force: If an external force is acting on the object (e.g., someone pushing it), it will also be represented in the diagram, typically parallel to the surface.

Steps to Create a Force Model for Inclined Planes

To properly analyze an object on an inclined plane, follow these steps:

1. Identify the Forces: Consider all forces acting on the object, including gravity, normal force, friction, and any external applied forces.
2. Decompose the Gravitational Force: Split the gravitational force into two components: one parallel to the inclined plane and one perpendicular to it. The parallel component causes the object to slide down the plane, while the perpendicular component determines the normal force.
3. Include Friction: The frictional force is proportional to the normal force and acts in the opposite direction to the motion of the object along the plane.
4. Draw the Force Model: Represent the forces as vectors in a diagram, ensuring they are drawn to scale and in the correct directions relative to the inclined plane.

Once the force model is established, the next step is to apply Newton’s second law or energy principles to solve for the unknowns, such as acceleration or the required applied force to move the object. By breaking down the forces into components and understanding their interactions, solving for objects on inclined planes becomes more straightforward and accurate.

Interpreting Free Body Diagram Answers

When analyzing a system using visual force models, the next crucial step is to interpret the results derived from these models. Understanding the interaction of forces, their magnitudes, and directions is essential for solving real-world problems in mechanics. Interpreting these results involves recognizing how each force affects the object’s motion and behavior, whether it’s at rest or in motion. Clear comprehension of the force components in the diagram helps predict outcomes like acceleration, equilibrium, and the effects of applied forces.

Understanding Force Magnitudes and Directions

One of the key elements in interpreting results is recognizing the relative sizes and directions of forces. The size of a vector in the visual model often corresponds to the magnitude of the force, and its direction indicates the way the force is acting on the object. A few key points to remember include:

• Balance of Forces: In systems at rest or in uniform motion, the forces should balance out. If the forces are unequal, the object will accelerate in the direction of the unbalanced force.
• Opposing Forces: Frictional forces, for example, often oppose motion. Analyzing these oppositions helps determine the net force and how it impacts the object’s movement.
• Sum of Forces: The total force in each direction (horizontal and vertical) must be calculated to find the net force. A proper interpretation will reveal if the system is in dynamic motion or static equilibrium.

Applications of Interpreted Results

Once the forces are interpreted, they can be used to solve for unknown variables, such as acceleration, velocity, or tension. These values can be calculated using Newton’s laws or energy principles, depending on the system’s conditions. Additionally, interpreted results can help in various real-world applications:

• Predicting Motion: Understanding the forces at play allows for predicting how an object will move, whether it’s sliding down a slope or being pulled along a surface.
• Determining Structural Integrity: Force analysis is essential for engineers to ensure that structures, such as bridges or buildings, can withstand applied loads.
• Optimizing Systems: In mechanical systems, such as engines or pulleys, analyzing force interactions can help optimize performance and efficiency.

Accurate interpretation of the results from force models is the key to solving complex mechanical problems. It allows for deeper insights into the behavior of systems and helps engineers and scientists design, predict, and control physical interactions effectively.