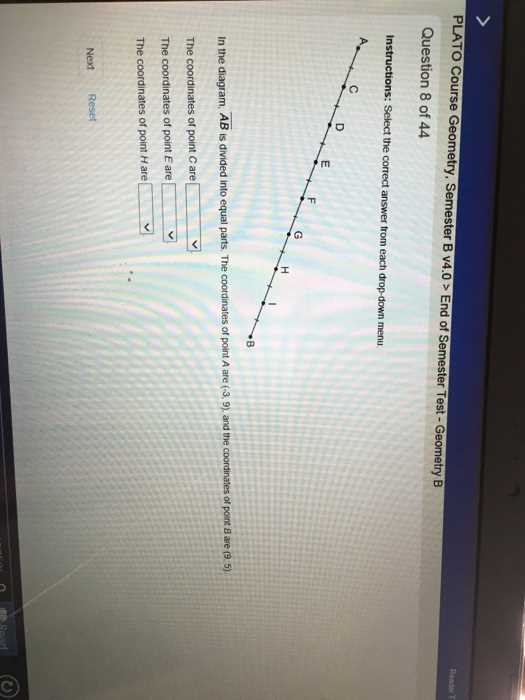
Geometry B Cumulative Exam Answers and Explanations

Preparing for a final evaluation in a mathematical subject can be overwhelming, but understanding key principles and strategies can make all the difference. This section provides a structured approach to tackling a variety of problems commonly encountered during a comprehensive test. By focusing on essential techniques and common challenges, students can boost their confidence and readiness.

Clear understanding of foundational concepts is critical for success. Whether you’re revisiting basic formulas or refining problem-solving skills, every piece of knowledge plays a crucial role in achieving a strong performance. By approaching each question with a strategic mindset, you can minimize errors and maximize your potential.

Through consistent practice and review, you’ll be able to recognize patterns and apply effective methods to any situation. The goal is not only to prepare for the test but to build a deeper understanding that can be applied beyond the classroom. With the right approach, complex topics can become manageable, and the final assessment will feel like just another step toward mastering the material.

Geometry B Cumulative Exam Answers

As you prepare for your final assessment, it’s important to focus on mastering the core principles that will help you succeed. This section highlights key techniques and strategies designed to assist in solving complex problems encountered during the evaluation. By understanding the logic behind various tasks, you can effectively apply your knowledge and confidently approach each challenge.

Key Topics to Focus On

Throughout your studies, you’ve been exposed to a range of concepts that play a vital role in your performance. By revisiting important topics such as shapes, measurements, and spatial reasoning, you can reinforce your understanding and increase your accuracy. Reviewing sample problems and understanding their solutions will allow you to recognize patterns and apply the correct methods to new questions.

Problem-Solving Techniques

One of the most effective ways to succeed is by honing your problem-solving skills. Break down each question into smaller, manageable parts and work step by step. Remember to apply proven strategies such as drawing diagrams, using formulas, and simplifying expressions where possible. With consistent practice, these techniques will become second nature, enabling you to solve problems quickly and accurately.

Understanding the Geometry B Exam Format

Familiarizing yourself with the structure of the final assessment is crucial to achieving success. The test typically consists of various sections, each focusing on a different set of skills and concepts. Understanding the format will help you manage your time effectively and approach each part with confidence. Knowing what to expect in terms of question types and difficulty will enable you to prepare more efficiently.

Types of Questions

The evaluation includes a mix of question formats, such as multiple choice, short answer, and problem-solving tasks. Each question type requires a different approach, and it’s important to practice strategies for each. For example, multiple-choice questions often require quick recall, while problem-solving questions may involve more detailed work, including logical reasoning and the application of formulas.

Time Management During the Test

Proper time management is essential to complete the assessment on time. Typically, the test has a set duration, and each section may have a recommended time limit. Practice working through problems within time constraints to improve your ability to move through the test efficiently. Prioritize the sections based on your strengths, but ensure you allocate time for all areas to maximize your overall score.

Key Concepts Covered in Geometry B

In preparing for the final assessment, it’s essential to revisit the fundamental topics that form the backbone of your studies. The material you’ll encounter focuses on various mathematical principles related to space, shapes, measurements, and spatial relationships. Mastery of these key concepts is crucial for solving a wide range of problems efficiently and accurately.

Properties of Shapes and Figures

A significant portion of the content revolves around understanding the characteristics and relationships of different shapes, including polygons, circles, and three-dimensional objects. Recognizing the properties of angles, sides, and symmetry will allow you to tackle complex problems, such as calculating area, perimeter, volume, and surface area. This knowledge is essential when solving tasks related to measurements and spatial analysis.

Transformations and Coordinate Geometry

Another critical area covered is transformations, which include translations, rotations, reflections, and dilations. Understanding how objects change in relation to their position and orientation is key to solving related problems. Additionally, coordinate geometry plays a vital role in analyzing relationships between points, lines, and shapes on the plane. Solving problems in this area requires a solid understanding of the Cartesian coordinate system and the ability to apply algebraic principles to geometric situations.

How to Approach the Cumulative Exam

Approaching your final assessment with a clear strategy can significantly impact your performance. Rather than rushing through the questions, take a moment to plan your approach. Understanding the structure of the test and knowing how to allocate your time wisely can help you stay focused and organized throughout the process. Proper preparation is key to reducing anxiety and improving efficiency.

Start by reviewing the instructions carefully to ensure you understand what is being asked in each section. Pay attention to any specific requirements or restrictions, such as time limits or allowed materials. Once you begin working through the problems, make sure to read each question thoroughly before answering, and consider what methods or formulas might be most useful.

Manage your time wisely by setting aside enough minutes for each section based on its difficulty and the number of questions. If you encounter a particularly challenging problem, move on and return to it later to avoid getting stuck. Staying calm and maintaining a steady pace will allow you to perform your best under pressure.

Essential Tips for Geometry Success

Achieving success in your final assessment requires more than just memorizing formulas and concepts. It’s about developing a solid understanding of the material and applying effective strategies when faced with problems. By following a few key tips, you can improve both your performance and confidence, ensuring that you’re well-prepared for any challenge.

Master the Fundamentals

A strong grasp of the basics is essential for tackling more advanced tasks. Focus on understanding core principles such as shapes, measurements, and angles. Once these foundational concepts are clear, you can build on them to solve more complex problems. Regular practice with basic problems will reinforce your skills and increase your speed during the test.

Practice Problem-Solving Techniques

Familiarity with different types of problems is critical for success. Work through a variety of practice exercises, paying attention to both straightforward and more intricate tasks. Use diagramming to visualize relationships, and always double-check your calculations. The more problems you solve, the easier it will be to identify patterns and apply the correct methods during your final assessment.

Common Mistakes to Avoid in Geometry

When tackling problems in a mathematical subject, it’s easy to overlook small details that can lead to incorrect solutions. Avoiding common mistakes is crucial for ensuring accuracy and improving your overall performance. Being aware of typical pitfalls will help you approach each question with greater confidence and precision.

Misunderstanding Problem Requirements

One of the most frequent errors is misinterpreting the question itself. Always take the time to carefully read and understand what is being asked. Look for key words like “find,” “calculate,” or “prove,” and ensure that you’re addressing the right elements of the problem. Skipping this step can result in solving the wrong part of the task, leading to wasted effort and incorrect answers.

Skipping Steps and Rushing Through Calculations

Rushing through calculations without showing all necessary steps is another common mistake. It may seem faster, but skipping intermediary steps can lead to errors that are difficult to spot. Take the time to write down each step of your work, double-check your operations, and ensure that you’re following the correct sequence. This practice not only helps avoid mistakes but also makes it easier to review your work if needed.

Step-by-Step Solutions for Practice Problems

One of the best ways to prepare for a final assessment is by working through practice problems and solving them step by step. This approach not only reinforces your understanding of key concepts but also helps you become more familiar with the types of tasks you may encounter. By breaking each problem down into smaller, manageable parts, you can gain clarity and confidence in your problem-solving abilities.

Solution Process for Common Problems

Here’s a general approach to solving common types of problems:

• Identify what is being asked: Read the problem carefully and highlight the key information.
• Draw a diagram: If applicable, sketch the situation to better visualize the relationships between elements.
• Choose the correct formula or method: Depending on the type of problem, determine which formula or strategy will lead to the solution.
• Execute the calculations: Perform the necessary arithmetic or algebraic steps, ensuring accuracy at each stage.
• Verify your answer: Once you have a solution, check it by plugging it back into the original problem or using a different method to verify the result.

Practice Problem Example

Let’s apply this process to a sample problem:

1. Problem: Calculate the area of a rectangle with length 8 units and width 5 units.
2. Solution:
   • Step 1: Recognize that the area of a rectangle is found by multiplying length by width.
   • Step 2: Use the formula: Area = Length × Width.
   • Step 3: Substitute the known values: Area = 8 × 5 = 40 square units.
   • Step 4: Verify the calculation: 8 × 5 = 40, so the answer is correct.

Using Geometry Formulas Effectively

Mastering key formulas is essential for solving problems efficiently and accurately. The correct application of formulas allows you to streamline your problem-solving process and tackle even the most complex tasks with ease. Understanding not just how, but when and why to use each formula is critical for success. This section will explore how to use mathematical formulas effectively to improve your results.

Memorizing Key Formulas

Before you can apply formulas correctly, it’s important to commit the most frequently used ones to memory. Common formulas for areas, volumes, perimeters, and angles should be at your fingertips. However, it’s not enough to simply memorize them–you should understand the meaning behind each formula and when to use it. Create flashcards or charts to help reinforce these formulas, and practice applying them in different contexts.

Applying Formulas to Solve Problems

Once you’ve memorized the formulas, focus on how to apply them correctly in various scenarios. Break down each problem into smaller components, identifying which quantities are given and which need to be calculated. Use the appropriate formula for each step, making sure that all measurements are in the correct units. Additionally, don’t forget to check if the formula needs to be adjusted based on the specific conditions of the problem.

Strategies for Solving Geometry Proofs

Proving geometric relationships requires a logical approach and a clear understanding of the relationships between shapes, angles, and lines. The goal of a proof is to establish the truth of a given statement based on previously established facts, postulates, and theorems. Developing a systematic strategy is key to solving these problems effectively and ensuring that your reasoning is both valid and coherent.

Steps to Approach Proofs

When tackling a proof, it’s important to follow a structured process. Here are the key steps to help guide you:

Step	Description
1. Understand the Given Information	Carefully read the problem to identify all known facts, such as angles, side lengths, and other relationships. Write down what is given.
2. State What You Need to Prove	Clarify exactly what the problem asks you to prove. This will help direct your logical steps.
3. Look for Relationships and Theorems	Identify applicable theorems, postulates, or previously proven results that can be used in your proof. Common tools include congruence and similarity rules.
4. Plan the Proof Steps	Think through the sequence of logical steps that will connect the given information to the desired result. Each step should be justified by a theorem or postulate.
5. Write the Proof Clearly	Present each step in a clear and concise manner, ensuring that each conclusion follows logically from the previous one.

By following this methodical approach, you can ensure that your reasoning is sound and that you are progressing logically from the given information to the desired conclusion.

Reviewing Geometry B Vocabulary

Understanding the key terminology used in mathematical reasoning is essential for solving problems accurately. Mastery of terms related to shapes, measurements, and relationships between elements will not only help in comprehension but also make it easier to apply the appropriate concepts in different scenarios. A solid grasp of vocabulary is crucial for effective communication of ideas and solutions.

Essential Terms to Remember

Here are some important terms you should review to build a strong foundation:

Term	Description
Angle	A figure formed by two rays (sides) with a common endpoint (vertex).
Parallel Lines	Lines that are always the same distance apart and never meet.
Perpendicular Lines	Two lines that intersect at a right angle (90 degrees).
Congruence	When two figures or shapes have the same size and shape.
Symmetry	The property of a figure where one half is a mirror image of the other.
Area	The amount of space inside a two-dimensional figure, measured in square units.
Volume	The amount of space inside a three-dimensional object, measured in cubic units.
Ratio	A relationship between two numbers showing how many times the first number contains the second.

By reviewing and familiarizing yourself with these terms, you’ll be able to approach problems with a clear understanding and communicate solutions more effectively. Consider making flashcards or creating diagrams to visualize these concepts for better retention.

Time Management for the Cumulative Exam

Effective time management is crucial when preparing for and tackling assessments that cover a broad range of material. Balancing the need to review concepts, solve problems, and ensure you finish on time requires careful planning. By allocating time wisely, you can enhance your performance, reduce stress, and approach each section with confidence.

Strategies for Preparing in Advance

Start your preparation well before the test day by creating a study schedule. Break down your study sessions into manageable blocks, focusing on different topics or types of problems during each block. Prioritize areas where you need the most improvement, but don’t neglect topics you’re already comfortable with. Consistent review and practice will help reinforce your knowledge and build confidence.

Managing Time During the Assessment

Once the assessment begins, it’s important to allocate time wisely. Start by quickly reviewing the entire set of questions to get a sense of the difficulty and the time each section might require. Divide your time based on the number of questions and their complexity, ensuring you don’t spend too long on any one item. If you encounter a difficult question, move on and return to it later if time permits. This way, you can maximize your opportunities to complete all sections.

Test-Taking Strategies for Geometry

Approaching assessments that involve mathematical reasoning requires a clear strategy to manage time, apply knowledge, and solve problems efficiently. By understanding the structure of the test and knowing how to break down complex questions, you can maximize your chances of success. These strategies will help you stay focused, minimize mistakes, and optimize your performance throughout the assessment.

Review the Instructions Carefully

Before diving into the problems, take a few moments to read through the instructions and any given information. Make sure you understand what each question is asking and note any specific requirements or constraints. Misinterpreting the question can lead to unnecessary errors, so clarify any doubts early on to avoid wasting time later.

Work Through Problems Systematically

When solving problems, focus on a methodical approach. Break down each problem into smaller, manageable steps. First, identify the known and unknown variables, and then determine the appropriate formula or theorem to apply. If you’re stuck on a problem, move on and revisit it later with a fresh perspective. Prioritize completing all questions, even if it means leaving a few challenging ones for later.

Practice Problems for Geometry B Review

Practicing problems is an essential part of reinforcing your understanding and preparing effectively for assessments. By working through various types of problems, you can test your knowledge, identify weak areas, and improve your problem-solving skills. Regular practice helps you become more comfortable with applying concepts and enhances your ability to think critically under pressure.

Sample Practice Problems

Below are some example problems to help you review key concepts and develop your problem-solving abilities:

Problem	Solution
Find the perimeter of a rectangle with a length of 8 units and a width of 5 units.	The perimeter is calculated by adding the lengths of all four sides. P = 2(l + w) = 2(8 + 5) = 26 units.
Calculate the area of a triangle with a base of 10 units and a height of 6 units.	Area = ½(base × height) = ½(10 × 6) = 30 square units.
What is the volume of a rectangular prism with a length of 4 units, width of 3 units, and height of 5 units?	Volume = length × width × height = 4 × 3 × 5 = 60 cubic units.
Find the measure of an unknown angle in a triangle where two angles are 40° and 75°.	The sum of angles in a triangle is always 180°. So, the unknown angle is 180° – (40° + 75°) = 65°.
Find the area of a circle with a radius of 7 units.	Area = π × r² = π × (7)² ≈ 153.94 square units.

By consistently solving these types of problems, you can solidify your understanding of key concepts and enhance your problem-solving abilities. Try to challenge yourself with more complex problems as you progress, and make sure to review any mistakes to learn from them.

How to Handle Challenging Questions

When faced with difficult questions, staying calm and methodical is key to solving them effectively. Instead of feeling overwhelmed, approach each problem step by step, breaking it down into smaller parts. Recognizing the challenge is the first step toward overcoming it, and a clear, logical process can help you find a solution. Here are some strategies to handle tough questions with confidence.

Steps for Tackling Difficult Problems

• Read Carefully: Ensure you fully understand the question before attempting to solve it. Reread the problem and highlight important details.
• Identify Known Information: Write down or mentally list the given values and conditions. This will help you see the structure of the problem more clearly.
• Look for Patterns: In many cases, recognizing familiar patterns or similar problems can guide you toward a solution.
• Break the Problem into Parts: Complex questions can often be simplified by dividing them into smaller, manageable steps.
• Skip and Return: If you’re stuck, move on to the next question and return later with a fresh perspective.

When to Seek Help

• Ask for Clarification: If a question feels unclear or confusing, don’t hesitate to ask for clarification from a teacher or peer.
• Use Resources: Don’t be afraid to refer to notes, formulas, or other study materials that can help you solve a problem.
• Practice Similar Problems: If a particular type of problem is difficult, look for additional practice problems on the same topic to improve your skills.

By following these steps, you can improve your ability to handle challenging problems. The key is to approach them with patience and persistence, knowing that each tough question is an opportunity to learn and grow. Remember, consistent practice and applying these strategies will make difficult problems more manageable over time.

Understanding the Test Rubric

Having a clear understanding of the evaluation criteria is essential for performing well on any assessment. The rubric outlines how your performance will be assessed and what specific aspects are being graded. By familiarizing yourself with the rubric, you can better align your responses with what is expected. Here’s a breakdown of key elements typically included in the rubric and how to leverage them for success.

Key Criteria in the Test Rubric

• Accuracy: Points are awarded based on the correctness of your responses. Ensure that every calculation or answer aligns with the given information and is logically sound.
• Clarity: Clear and concise explanations are crucial. Avoid unnecessary complexity, and make sure your reasoning is easy to follow.
• Methodology: Your approach to solving problems matters. Rubrics often reward the correct method, even if the final answer is incorrect, as long as the process is logically sound.
• Completeness: A thorough response includes all necessary steps or components. Incomplete answers or missing steps may lead to a loss of points.
• Presentation: Neatness and organization are important. Well-structured responses with properly labeled diagrams or calculations demonstrate a higher level of understanding.

How to Maximize Your Score

• Review the Rubric Before the Test: Familiarize yourself with the specific criteria used for grading. Understanding how points are allocated will help you focus on what matters most.
• Practice Using the Rubric: When reviewing practice problems, score yourself based on the rubric. This can give you insight into areas you need to improve.
• Ensure Detailed Explanations: Even if you know the answer, explaining your steps clearly can boost your score, especially for problems that require showing your work.
• Stay Organized: Keep your work neat and clearly marked. Avoid cluttered solutions, and make sure your reasoning is easy to follow.

By understanding and utilizing the test rubric, you can better focus your efforts on areas that will earn you the most points. Adopting a strategic approach to each question, paying attention to both the method and presentation of your work, can significantly improve your performance.

Preparing for the Final Assessment

Preparation is key to performing well in any comprehensive evaluation. Whether you are reviewing fundamental concepts or refining your skills, a structured approach to studying can make all the difference. The goal is to ensure you have a strong understanding of the material, so you are confident and ready when it’s time to demonstrate your knowledge. Here are some strategies to guide your preparation for the final assessment.

Review Core Concepts and Skills

Start by revisiting the core concepts and formulas that have been covered throughout the course. These are the building blocks that will be tested, and having a firm grasp on them will provide a solid foundation for tackling more complex problems. Focus on:

• Understanding Key Theorems: Ensure you can apply theorems and properties to different situations, such as those related to shapes, angles, and transformations.
• Mastering Problem-Solving Techniques: Practice solving a variety of problems that require different approaches, from basic calculations to more intricate proofs.
• Reviewing Formulas: Familiarize yourself with important formulas that may be needed, such as those for areas, perimeters, volumes, and surface areas.

Strategize Your Study Sessions

Efficient studying is about quality, not just quantity. Here are some tips to help you stay focused:

• Break Down Study Sessions: Avoid long, tiring study marathons. Break your study time into focused blocks, with breaks in between to refresh your mind.
• Use Practice Tests: Simulate the testing environment by taking practice tests under timed conditions. This will help you become comfortable with the format and time constraints.
• Group Study: Consider studying with a group. Explaining concepts to others and discussing challenging problems can reinforce your understanding and highlight areas that need more attention.

By carefully reviewing key concepts, practicing problem-solving techniques, and planning your study sessions, you can approach the final assessment with confidence. Preparation is not only about knowledge, but also about managing your time and resources effectively.

Resources for Extra Practice

Supplementary practice can be invaluable when reinforcing your understanding of important concepts. Whether you are seeking additional exercises to challenge yourself or looking for resources to solidify your skills, there are many options available. These resources can help you hone your problem-solving abilities and provide new perspectives on the material.

Online Platforms for Practice

Numerous websites and apps offer interactive exercises and step-by-step solutions, allowing you to practice at your own pace. Some popular platforms include:

• Khan Academy: A comprehensive resource with a wide range of video tutorials and practice exercises covering various topics.
• IXL: Provides personalized practice problems and immediate feedback to help you track your progress.
• Brilliant.org: A platform that offers in-depth problem-solving exercises and courses for students looking to expand their critical thinking skills.

Books and Workbooks for Practice

If you prefer a more traditional approach, books and workbooks offer structured exercises and detailed explanations. Some excellent options include:

• Practice Problems for All Levels: Books designed for specific topics often break down problems into manageable steps, allowing you to progress from beginner to advanced levels.
• Shmoop: A resource that combines study guides with practice problems, helping you understand the theory while reinforcing key concepts with exercises.
• Barron’s Test Prep: Provides focused practice with explanations for various types of problems, perfect for additional review before an important assessment.

Interactive Tools and Apps

In addition to traditional resources, there are several apps and interactive tools that can aid in extra practice:

• GeoGebra: A dynamic mathematics software that allows users to visualize geometric concepts and explore interactive problems.
• Photomath: An app that lets you solve problems by taking pictures of them and provides step-by-step solutions, perfect for understanding the process behind each solution.
• Wolfram Alpha: A powerful computational tool that can assist with problem-solving and provide detailed explanations for various types of problems.

By utilizing these resources, you can strengthen your skills, identify areas for improvement, and ensure you are well-prepared for any challenge. Whether you prefer online platforms, books, or interactive tools, consistent practice will help solidify your knowledge and build confidence.

Staying Calm During the Test

Managing stress and maintaining focus are key factors in performing well during a timed assessment. When faced with difficult questions or time pressure, staying composed allows you to think clearly and approach each task with confidence. Employing strategies to stay calm can help you remain productive and avoid making impulsive mistakes.

One effective method is to take a few deep breaths when you begin to feel overwhelmed. This simple technique helps lower anxiety and brings your focus back to the task at hand. It’s also important to pace yourself; avoid rushing through questions and remember that it’s okay to skip difficult ones and return to them later. Prioritize those you’re confident in to build momentum and boost your morale.

Another useful strategy is to break down complex problems into smaller, manageable steps. By isolating parts of the question, you can more easily understand what is being asked and avoid feeling overwhelmed. Additionally, maintaining a positive mindset and reminding yourself that you’ve prepared can reduce self-doubt and keep you on track.

Lastly, take advantage of the time allotted wisely. If you find yourself getting stuck, it’s better to move on to the next question and come back later with a fresh perspective. Time management plays a big role in staying calm and organized throughout the assessment.