Edgenuity Cumulative Exam Answers for Geometry

When preparing for a challenging assessment in advanced mathematics, it’s crucial to focus on the most important concepts and skills. This guide will help you navigate through complex topics and provide insights into how to tackle problems effectively. Mastery of key principles and techniques is the foundation for success.

By reviewing essential formulas, understanding problem-solving strategies, and practicing with various exercises, you can build confidence in your ability to approach any question. Whether you’re dealing with intricate calculations or visualizing mathematical relationships, the right strategies can make all the difference in achieving a high score.

Preparation is key. Developing a clear understanding of each area allows you to solve problems quickly and accurately, giving you an edge during the test. This section will help you organize your study plan and ensure that you are fully equipped to handle the challenges ahead.

Edgenuity Geometry Exam Guide

Preparing for a mathematics assessment requires a strategic approach, focusing on key concepts and mastering essential skills. By building a solid foundation, you can approach even the most challenging problems with confidence and clarity. This guide will help you understand the crucial areas of focus, from formulas to practical application, so you can excel.

To perform well, it’s important to review the following topics that are commonly assessed:

• Basic geometric shapes and properties
• Understanding theorems and postulates
• Solving equations involving angles and lengths
• Working with proofs and logical reasoning
• Interpreting and analyzing diagrams effectively

Each of these topics plays a significant role in your overall performance. To ensure you’re fully prepared, focus on developing your skills in both theoretical understanding and practical problem-solving. Here are some tips to help you:

1. Review key concepts regularly: Make sure to refresh your understanding of important principles such as Pythagorean theorem, angle relationships, and properties of triangles and circles.
2. Practice solving problems: Engage with various exercises that challenge you to apply what you’ve learned in different contexts.
3. Visualize problems: Many questions require interpreting diagrams. Improve your ability to visualize shapes and their relationships to solve problems more effectively.
4. Use study aids: Online resources, practice worksheets, and interactive tools can enhance your understanding and provide additional practice.

By focusing on these strategies, you’ll be prepared to tackle even the most difficult questions with precision and accuracy. A consistent and focused approach will make all the difference in achieving your goals.

Understanding the Assessment Structure

In order to excel in a challenging mathematics assessment, it’s important to understand how the test is structured. Knowing the format, types of questions, and the topics that will be covered can help you manage your time effectively and approach each section with confidence. This section will guide you through the overall structure and provide insights on what to expect.

Types of Questions You Will Encounter

The assessment will consist of a variety of question types, each designed to test your understanding and problem-solving skills. These may include:

• Multiple-choice questions that assess your ability to select the correct answer based on given information.
• Short-answer questions that require you to show your work and explain the steps you took to reach a solution.
• Diagram-based questions where you will need to analyze and interpret visual representations of shapes, angles, or other mathematical concepts.
• Long-form problems that may involve multiple steps or the application of several different concepts to find the solution.

Topics Covered in the Assessment

The content of the test will focus on a broad range of mathematical concepts. These topics will test your ability to apply theoretical knowledge to practical problems. Some of the key areas include:

• Properties and relationships of shapes, including angles, lengths, and areas.
• Logical reasoning and proof techniques, such as understanding and applying theorems.
• Problem-solving strategies that require a deep understanding of formulas and principles.
• Diagram analysis, requiring you to work with visual representations and geometric figures.

Understanding the structure of the test will allow you to plan your preparation effectively, focus on the areas that matter most, and approach each question with the right mindset. By practicing different types of questions and reviewing the topics in-depth, you can ensure that you are well-prepared for the assessment.

Key Topics in Geometry You Should Know

To succeed in any mathematical assessment, it is essential to focus on the core concepts that will be tested. Understanding the fundamental principles not only helps you solve problems efficiently but also allows you to apply your knowledge to new and unfamiliar situations. The following key topics will ensure you are well-prepared for any challenges you may face.

1. Properties of Shapes

Understanding the properties of basic shapes, including triangles, quadrilaterals, and circles, is crucial. These shapes often form the basis of more complex problems, so knowing their properties–such as the sum of angles in a triangle or the relationship between the sides of different polygons–is essential for solving questions.

2. Angle Relationships

Angles are a central element of many problems. You should be familiar with concepts such as complementary, supplementary, and vertical angles, as well as angle relationships in parallel lines. Being able to recognize and calculate these angles quickly will help in both simple and advanced problems.

3. Theorems and Proofs

A strong understanding of key theorems, such as the Pythagorean theorem and properties of parallel lines, is necessary. Being able to prove or apply these theorems effectively is a skill that will come in handy when solving complex problems or demonstrating your knowledge.

4. Coordinate Geometry

Coordinate geometry involves working with points on a grid, using algebraic methods to solve geometric problems. You will need to understand concepts such as the distance formula, midpoint formula, and the slope of a line to solve problems involving straight lines and circles.

5. Area and Perimeter Calculations

Calculating the area and perimeter of different shapes is fundamental in geometry. Be sure to memorize formulas for various figures such as rectangles, triangles, circles, and complex polygons. These formulas are often used in a wide range of questions.

6. Volume and Surface Area

For three-dimensional objects, understanding how to calculate volume and surface area is essential. Whether dealing with cubes, spheres, or cylinders, knowing how to apply the correct formulas will allow you to answer a variety of questions efficiently.

Mastering these key topics will ensure that you are well-equipped to tackle any mathematical challenges. Focus on these areas during your preparation to build a strong foundation, enabling you to solve even the most complex problems with confidence.

How to Prepare for Geometry Assessments

Preparing for a mathematics assessment involves a combination of understanding key concepts, practicing problem-solving techniques, and developing strategies for managing time during the test. By organizing your study plan effectively, you can ensure that you cover all the essential topics and boost your confidence before taking the test.

Organize Your Study Plan

A well-structured study plan is the foundation of effective preparation. Start by breaking down the material into smaller sections and allocate enough time to review each one. Focus on topics that are more challenging for you, but don’t neglect the areas where you are already confident. Regular review and repetition will help solidify your knowledge.

• Break the syllabus into manageable sections.
• Set specific goals for each study session.
• Review practice problems regularly to reinforce learning.

Practice Problem-Solving Techniques

To improve your problem-solving skills, practice is key. Work through different types of problems, starting with simpler ones and gradually progressing to more complex questions. Be sure to focus on the steps involved in solving each problem, as understanding the process is just as important as arriving at the correct answer.

• Work through past exercises and sample problems.
• Practice applying formulas and theorems in various contexts.
• Review common mistakes and learn from them to avoid repeating them in the future.

By consistently practicing, you will build the necessary skills to approach any question with confidence and accuracy. Remember, the more you practice, the more familiar you will become with the types of problems and the best strategies for solving them.

Common Mistakes to Avoid During the Assessment

During a mathematical assessment, it’s easy to fall into certain traps that can negatively impact your performance. Being aware of these common mistakes can help you avoid unnecessary errors and improve your overall score. By paying attention to detail, managing your time, and following a structured approach, you can minimize the chances of making these mistakes.

Rushing Through Questions

One of the most common mistakes students make is rushing through problems in an attempt to finish quickly. This can lead to careless errors, such as misreading questions or making simple arithmetic mistakes. Instead of hurrying, take the time to carefully read each question and check your work before moving on to the next one. Proper time management can help you maintain a steady pace without feeling rushed.

• Read each question carefully before answering.
• Double-check your calculations, especially with complex problems.
• Do not skip over parts of a problem; ensure all steps are completed.

Neglecting to Show Your Work

Many students make the mistake of not showing their work, especially when solving multi-step problems. While it might seem like an unnecessary task, showing your steps not only helps you stay organized but also allows you to catch mistakes earlier. In some cases, even if the final answer is incorrect, you may still earn partial credit for demonstrating the correct process.

• Write down all the steps involved in your calculations.
• Clearly label your diagrams and solutions.
• Use formulas and show how you apply them step by step.

By avoiding these common mistakes, you can ensure that you approach the test with a clear, focused strategy. Proper preparation, careful attention to detail, and a methodical approach to each problem will help you perform at your best.

Effective Study Strategies for Geometry

Mastering mathematical concepts requires a strategic approach to studying. To excel in spatial reasoning and shape-related problems, it’s essential to focus on both theoretical understanding and practical application. The right study techniques can help reinforce these concepts and improve problem-solving abilities. Below are some effective strategies that will help you succeed.

1. Break Down Complex Concepts

Mathematical principles can seem overwhelming at first glance. Breaking down complex topics into smaller, manageable parts makes it easier to understand. Focus on one concept at a time, and don’t rush through them. Once you feel confident in one area, move on to the next.

• Start with basic shapes and their properties.
• Gradually move on to more complex topics like proofs and theorems.
• Use diagrams to visualize and simplify abstract ideas.

2. Practice Regularly and Consistently

Mathematics is a skill that improves with regular practice. Working through problems daily helps reinforce key principles and build confidence. It also allows you to identify areas where you may need more focus. Consistency is the key to mastering both simple and advanced topics.

• Set aside time each day for solving problems and reviewing concepts.
• Use a variety of resources, such as textbooks, online practice problems, and apps.
• Start with easier problems and gradually tackle more difficult ones as your skills improve.

3. Focus on Active Learning Techniques

Passive reading or watching videos can only take you so far. To truly master a topic, engage with the material through active learning. This could include working through problems, teaching someone else, or creating your own notes and study aids.

• Use flashcards to memorize key formulas and theorems.
• Teach the concept to a peer or study group to reinforce your understanding.
• Summarize each chapter or topic in your own words to ensure comprehension.

By incorporating these study strategies into your routine, you will be better equipped to understand key concepts and solve problems with confidence. A combination of practice, active learning, and concept breakdown will lead to stronger skills and better overall performance.

How to Tackle Difficult Geometry Questions

When faced with challenging problems, it’s essential to stay calm and approach each question methodically. Difficult questions often require deeper analysis and a more strategic approach. Instead of becoming frustrated, break the problem down step by step, identify key concepts, and apply logical reasoning to find a solution.

1. Identify Key Information

The first step in solving a complex problem is to carefully read the question and identify all relevant information. Often, difficult questions will include extraneous details that may seem important but aren’t necessary for solving the problem. Focus on the key data points that will help you apply the correct formulas or principles.

• Highlight important values such as side lengths, angles, and coordinates.
• Look for key relationships between different parts of the problem, such as symmetry or congruency.
• Ignore irrelevant information that doesn’t affect the solution.

2. Break the Problem Into Smaller Parts

Large, complicated questions can be overwhelming, but breaking them into smaller, more manageable tasks can make the process easier. Work through the problem step by step, solving one part at a time. This approach will help you stay organized and ensure you don’t overlook any details.

• Start with the simplest elements of the problem before tackling the more complex parts.
• Use diagrams to visualize relationships and simplify your understanding of the problem.
• Apply relevant formulas progressively, checking each step for accuracy.

3. Look for Patterns and Relationships

Many difficult questions are designed to test your ability to recognize patterns and relationships between different elements. Once you’ve identified the key information, try to see how different parts of the problem are connected. This can often lead to shortcuts or insights that make the solution process easier.

• Look for symmetrical properties or congruent shapes that simplify the problem.
• Apply known theorems or rules that might reveal hidden relationships between elements.
• Use logical reasoning to predict the next step or check your solution’s consistency.

By following these strategies, you can turn difficult questions into manageable tasks and increase your chances of success. Stay focused, break down the problem, and apply your knowledge step by step to find the solution with confidence.

Top Resources for Geometry Practice

To improve your understanding of spatial reasoning and shape-related problems, it’s essential to utilize a variety of resources. Practicing with different tools can help reinforce key concepts, enhance problem-solving skills, and provide diverse perspectives on mathematical concepts. Below are some of the best resources that can aid in your studies.

Resource	Description	Best For
Online Practice Platforms	Interactive websites offering a wide range of practice problems and step-by-step solutions.	Building problem-solving skills and reinforcing concepts.
Textbooks	Traditional textbooks with detailed explanations, examples, and exercises for self-study.	In-depth understanding of theories and structured learning.
Mobile Apps	Apps designed for on-the-go learning, offering practice problems and quizzes.	Convenient, flexible practice, and quick assessments.
Video Tutorials	Instructional videos from educators and experts that explain complex topics with visual aids.	Visual learners and those needing step-by-step walkthroughs.
Study Groups	Collaborative groups where learners can discuss problems and share insights.	Peer learning, motivation, and idea exchange.

By using a combination of these resources, you can enhance your comprehension and become more confident in solving various types of problems. Make sure to integrate both theoretical study and hands-on practice to strengthen your skills in the most effective way.

Understanding Geometry Formulas and Equations

Formulas and equations are the foundation of solving mathematical problems related to shapes, spaces, and dimensions. A strong grasp of these relationships allows you to calculate areas, volumes, angles, and other essential properties accurately. Understanding the principles behind these formulas is key to applying them effectively in various situations.

Formula	Purpose	Example
Area of a Circle	Calculates the space inside a circle.	A = πr², where r is the radius.
Circumference of a Circle	Measures the perimeter or boundary of a circle.	C = 2πr, where r is the radius.
Pythagorean Theorem	Relates the sides of a right triangle.	a² + b² = c², where c is the hypotenuse.
Volume of a Sphere	Calculates the space inside a three-dimensional sphere.	V = (4/3)πr³, where r is the radius.
Area of a Rectangle	Finds the area of a two-dimensional rectangle.	A = l × w, where l is length and w is width.

Each formula has specific applications depending on the shape or structure you’re working with. Learning when and how to apply these formulas is essential for solving problems efficiently. As you study, ensure you not only memorize the formulas but also understand their derivations and underlying principles to enhance your problem-solving abilities.

Geometry Proofs and How to Solve Them

Proofs are a vital part of mathematics that allow you to demonstrate the truth of a statement based on logical reasoning and established principles. The process involves starting with known facts or axioms and systematically showing how they lead to the conclusion. Solving proofs requires clear thinking, understanding of geometric concepts, and careful application of rules.

Step	Action	Purpose
Step 1	Identify Given Information	Understand the facts and conditions presented in the problem.
Step 2	State the Goal	Clarify what you are trying to prove or demonstrate.
Step 3	Draw a Diagram	Visualize the problem and label all known elements.
Step 4	Use Theorems and Definitions	Apply known geometric principles to build a logical chain of reasoning.
Step 5	Conclude with a Proof	Arrive at the solution by logically linking all steps together.

By following a structured approach, you can break down a proof into manageable parts and apply your knowledge step by step. Make sure to always justify each step with appropriate reasoning or a known rule, ensuring the proof is valid from start to finish. Consistent practice will enhance your ability to solve proofs more efficiently and accurately.

Time Management Tips for the Exam

Effective time management is crucial to performing well in any assessment. By organizing your time wisely, you can ensure that you complete each section of the test without feeling rushed, and have enough time to review your work. Here are some strategies to help you manage your time effectively during the test.

• Prioritize Difficult Questions – Start by identifying the most challenging questions and tackling them first. This allows you to allocate more time to complex problems while you’re still fresh.
• Set Time Limits for Each Section – Break the test into sections and allocate a set amount of time to each part. Use a timer to stay on track and avoid spending too much time on any one section.
• Use the Process of Elimination – When uncertain about an answer, eliminate clearly wrong options. This approach can save time and increase your chances of selecting the correct answer.
• Move On if Stuck – If you’re stuck on a question, move on and come back to it later. Spending too much time on one problem can affect your performance on others.
• Leave Time for Review – Ensure you have time at the end to review your answers. Double-check calculations, ensure all questions are answered, and correct any mistakes if necessary.

By following these strategies, you can approach the test with confidence, manage your time efficiently, and increase your chances of success. Remember, practice with time constraints will help you become more comfortable with pacing yourself during the actual assessment.

What to Do if You Are Stuck

It’s common to feel stuck during a challenging task, especially when dealing with complex problems. Instead of panicking, there are effective strategies you can use to get back on track. The key is to stay calm, reassess the problem, and apply your knowledge in a systematic way.

Take a Break and Refocus

If you’re finding it difficult to move forward, take a short break. Step away from the problem for a few minutes to clear your mind. Often, a quick pause will allow you to return with a fresh perspective and new ideas on how to proceed.

Break the Problem Down

Sometimes large problems can feel overwhelming. To overcome this, break the problem into smaller, manageable parts. Focus on one aspect at a time, solving each section before moving to the next. This approach helps simplify complex issues and provides a sense of progress.

Additionally, if you feel stuck for too long, move on to the next question. You can always return to the challenging problem later, and often, the answers to other questions can offer hints or refresh your thinking. This strategy ensures you’re using your time efficiently and not getting bogged down by a single obstacle.

Reviewing Key Geometry Concepts Quickly

When preparing for an assessment, it’s essential to review the most important concepts efficiently. Focusing on the core principles allows you to reinforce your understanding and recall essential information when needed. Below are some strategies to help you review key ideas swiftly and effectively.

Utilize Summary Sheets – One of the most effective methods for quick revision is using summary sheets or cheat sheets. These can include important formulas, definitions, and key concepts. Keep them concise and organized, making it easy to reference them during study sessions or before an assessment.

Practice with Sample Problems – Solving practice questions related to the most important topics can greatly enhance your understanding. Focus on areas that you find difficult, and work through a variety of problems to solidify your skills. By repeating these exercises, you’ll reinforce concepts and identify any remaining gaps in your knowledge.

Review Visual Aids – Diagrams, charts, and graphs are crucial in understanding spatial concepts. Review common figures such as circles, triangles, and polygons, paying close attention to their properties and relationships. This will help you visualize problems more clearly and recall relevant information during assessments.

Group Study – Collaborating with peers can also speed up the revision process. Sharing different perspectives and discussing concepts can help reinforce your understanding and highlight areas that need more focus. Group study sessions can be especially beneficial for reviewing complex topics in a more interactive way.

By applying these strategies, you can quickly reinforce your understanding of key topics and be better prepared for any assessment. Effective time management and focus on core principles will allow you to maximize your preparation in a short amount of time.

Using Online Tools for Geometry Help

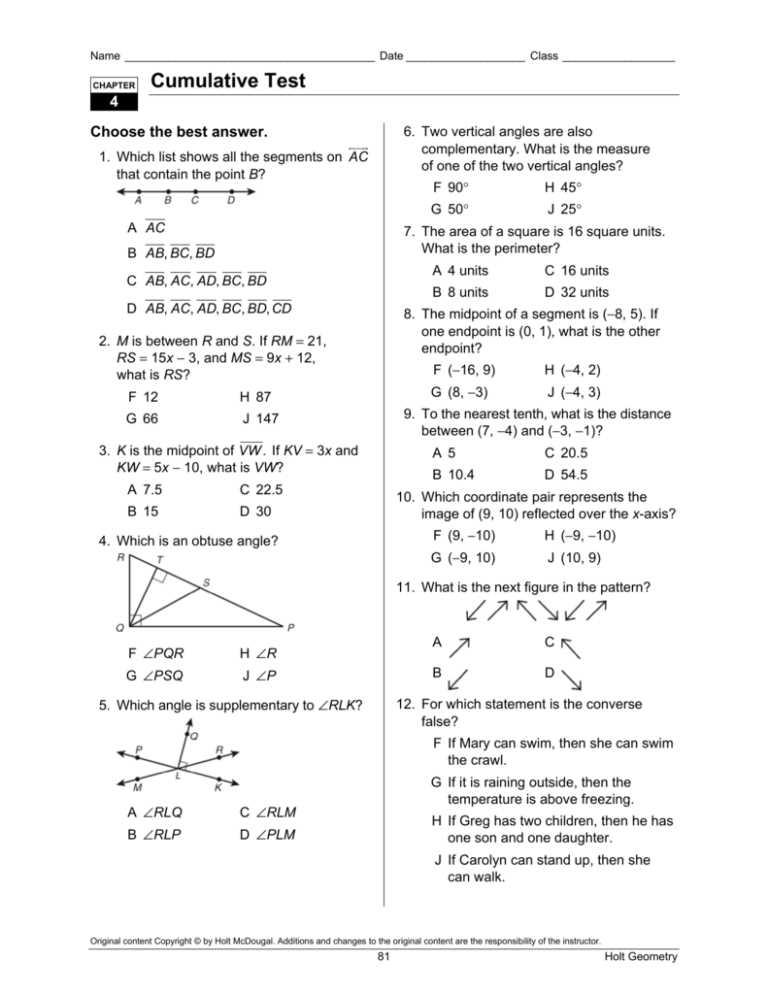
The internet offers a wealth of resources that can significantly enhance your understanding of complex concepts. By using the right online tools, you can quickly access tutorials, solve problems step by step, and clarify any areas where you may feel uncertain. These tools can be especially helpful when you need immediate assistance or prefer self-paced learning.

Interactive Problem Solvers

One of the most effective online tools are interactive solvers. These platforms allow you to input specific problems and receive detailed solutions. Some even break down the steps involved in solving each problem, giving you a deeper understanding of the methods used. This step-by-step guidance is invaluable for mastering challenging problems.

Video Tutorials and Courses

Another powerful tool for learning is video content. Many websites offer free or paid video tutorials that cover essential topics in detail. These tutorials often include visual aids and demonstrations, which can help you understand spatial concepts more clearly. Whether you’re looking for a quick review or an in-depth explanation, video lessons are a great way to enhance your knowledge and skills.

By incorporating these online resources into your study routine, you can take a more active approach to learning. These tools provide the flexibility to work at your own pace while offering immediate solutions and explanations for any questions you may have.

How to Use Practice Tests Effectively

Practice tests are an essential tool for reinforcing your knowledge and assessing your readiness. They simulate the real assessment environment, allowing you to familiarize yourself with the types of questions that may appear and how to manage your time effectively. By utilizing these practice sessions, you can identify your strengths and focus on areas that need improvement.

To maximize the benefits of practice tests, approach them with a strategic mindset. Start by taking a full-length test to get a sense of the overall structure and question formats. After completing the test, review your mistakes thoroughly to understand where you went wrong and what concepts need more attention. This targeted review is key to improving your performance over time.

Incorporating these practice sessions into your study routine will help build confidence, reduce anxiety, and ensure you’re well-prepared for the actual assessment. Make sure to use the results of each test as a guide to focus your future study efforts on the areas where you need the most improvement.

Tips for Analyzing Diagrams Effectively

Interpreting diagrams correctly is a crucial skill in tackling problems that involve visual representations. Diagrams provide key insights into the relationships between different elements, and understanding these connections can greatly simplify complex problems. To make the most of these visual aids, it’s important to follow a systematic approach that helps you decode the diagram accurately.

• Identify Key Elements: Begin by identifying all labeled points, lines, angles, and shapes. Make sure you know what each symbol and label represents to avoid confusion later on.
• Look for Patterns: Many problems rely on geometric patterns. Check for symmetry, parallel lines, or congruent shapes that could provide valuable clues.
• Use Known Theorems: Apply any relevant geometric theorems, such as the Pythagorean theorem, properties of triangles, or circle theorems, to analyze the diagram further.
• Mark Important Information: As you study the diagram, highlight or annotate key information such as given lengths or angle measures. This will make it easier to refer back to them when solving the problem.
• Break Down Complex Figures: If the diagram is complex, break it into smaller, manageable parts. Analyzing each part separately can help you understand the bigger picture more clearly.

By practicing these steps regularly, you can improve your ability to extract useful information from diagrams and solve related problems with greater ease. Being thorough and methodical in your analysis will ensure you don’t overlook important details and help you reach the correct solution more efficiently.

How to Improve Your Scores in Mathematics

Achieving higher marks in mathematical assessments requires a combination of practice, understanding, and strategy. Many students find certain concepts challenging, but with the right approach, it is possible to improve performance and develop a stronger grasp of the material. The key is to focus on both theoretical knowledge and problem-solving skills, ensuring that you are well-prepared for any task at hand.

• Understand Core Concepts: Make sure you have a solid understanding of the foundational principles. Without a strong grasp of the basics, it becomes difficult to tackle more advanced problems. Revisit key topics and seek additional resources if necessary.
• Practice Regularly: Consistent practice is essential to improving your skills. Work on a variety of problems to reinforce your understanding and identify areas where you need more attention. The more problems you solve, the more confident you will become.
• Focus on Mistakes: Don’t just move on after making a mistake. Analyze where you went wrong and understand why the correct solution works. This will help you avoid similar mistakes in the future and deepen your understanding of the material.
• Use Study Aids: Take advantage of available study materials such as textbooks, online tutorials, and interactive tools. Visual aids and practice problems can clarify difficult concepts and provide a fresh perspective.
• Seek Help When Needed: If you’re struggling with a particular topic, don’t hesitate to ask for help. Whether it’s a tutor, teacher, or online forum, getting clarification on tough concepts can save you time and frustration.
• Time Management: Effective time management is crucial. Allocate enough time to study each topic thoroughly, but also make sure you leave time to review and solve practice problems. Create a study schedule to stay on track.

By consistently applying these strategies, you can gradually improve your understanding and boost your performance in any math-related assessment. Persistence, practice, and proactive learning are the keys to success.