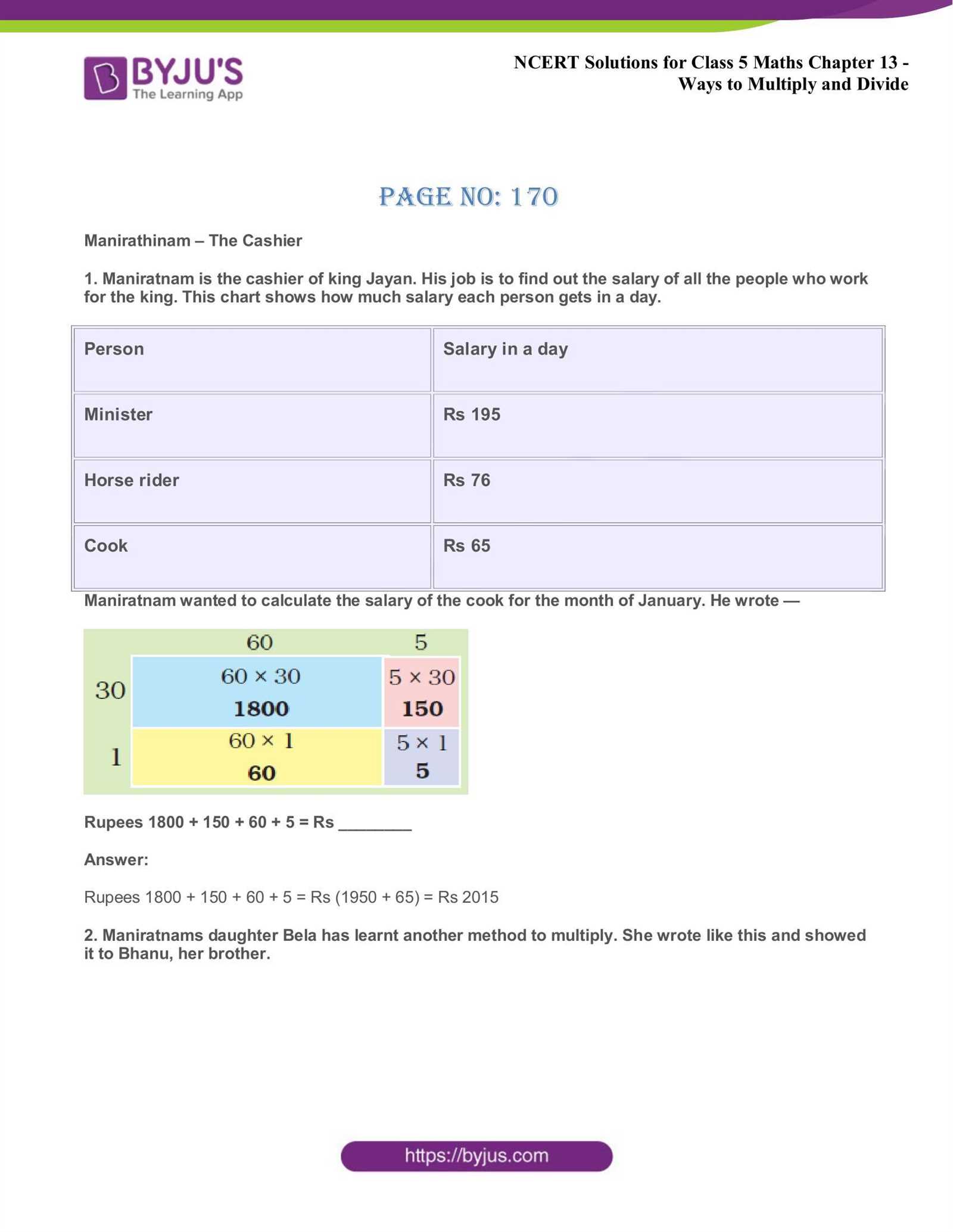
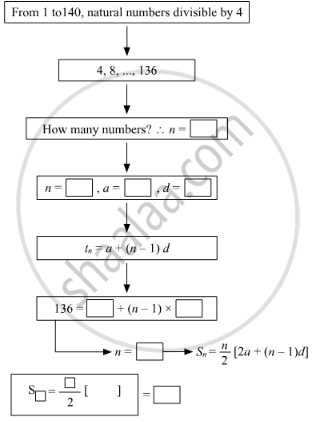
Core Connections Algebra 1 Chapter 3 Answers
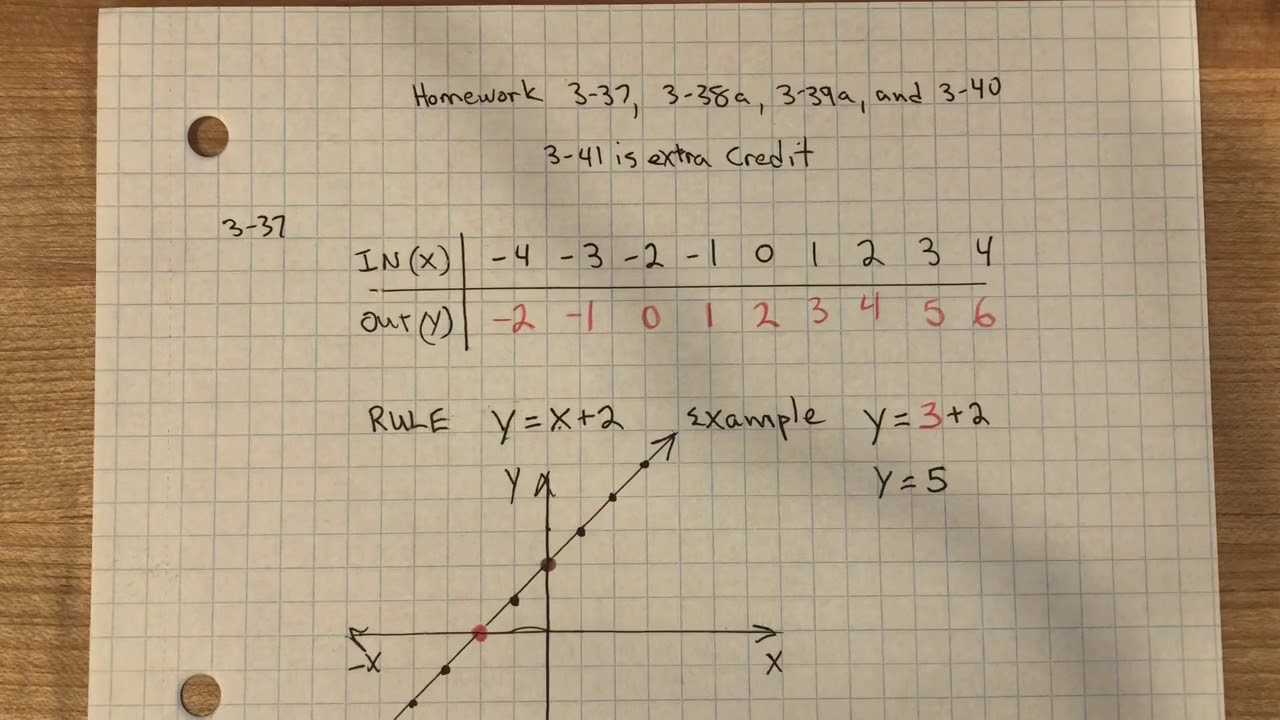
The third section of this learning material focuses on essential mathematical skills that provide the foundation for understanding complex equations. This segment is designed to guide students through a series of problem-solving techniques, helping to build confidence in approaching mathematical challenges. It covers methods that simplify the process of working with equations, ensuring that key concepts are clearly explained and accessible to learners.
Throughout this section, students will engage with various types of expressions and operations, developing their ability to manipulate and solve them effectively. Emphasis is placed on providing logical steps that lead to correct solutions, with numerous examples illustrating how these strategies can be applied in different scenarios. By the end of the section, learners will be equipped with the necessary tools to handle a wide range of mathematical problems with ease.
Mathematical Problem Solving in Section 3
This section delves into essential problem-solving techniques, guiding students through various types of mathematical expressions and operations. The goal is to enhance their ability to understand and manipulate equations, laying a solid foundation for more advanced topics. With clear examples and step-by-step solutions, learners can grasp fundamental concepts and build confidence in tackling similar problems on their own.
Key Problem-Solving Strategies
In this part of the material, students will explore methods for simplifying and solving different types of equations. By focusing on systematic approaches, the section ensures that each concept is clearly broken down and easy to follow. Students will gain a deeper understanding of how to approach problems logically and how to apply various techniques to reach correct solutions.
Practical Applications
Beyond theoretical knowledge, this section emphasizes real-life applications of mathematical concepts. Understanding how to use mathematical principles in practical situations helps students connect what they learn to everyday scenarios, reinforcing the relevance of these skills.
| Problem Type | Strategy | Example |
|---|---|---|
| Linear Equations | Isolate the variable | x + 5 = 10 → x = 5 |
| Quadratic Equations | Factor or use the quadratic formula | x² – 5x + 6 = 0 → (x – 2)(x – 3) = 0 |
| Word Problems | Translate to an equation and solve | If 3 times a number plus 2 equals 11, find the number: 3x + 2 = 11 → x = 3 |
Understanding Algebraic Expressions
This section explores the structure and components of mathematical expressions. It focuses on how variables, constants, and operators come together to form a meaningful statement that can be solved or simplified. A strong understanding of these elements is crucial for working with more complex equations and solving real-world problems.
Breaking Down Mathematical Components
At the heart of every expression are its parts: variables represent unknown quantities, constants are fixed values, and operators define the relationships between them. Mastering the way these components interact allows students to manipulate and simplify expressions effectively. Understanding how to identify and work with each element is key to progressing in more advanced mathematical concepts.
Operations on Expressions
Once an expression is broken down, students learn various operations such as addition, subtraction, multiplication, and division. These operations allow for the transformation and simplification of expressions. The goal is to equip students with the tools to handle expressions efficiently, making complex tasks easier to approach.
Solving Linear Equations Step by Step
This section focuses on the systematic approach to solving equations involving a single variable. By following a clear set of steps, students can simplify these equations and isolate the unknown, ultimately finding the correct solution. The process involves using basic operations to manipulate the equation and make the variable stand alone on one side.
The first step is to eliminate any constants or coefficients from the side with the variable. This is done by performing inverse operations such as adding, subtracting, multiplying, or dividing. Each action taken must maintain the equality of the equation, ensuring that the solution remains accurate. By carefully following these steps, students will be able to solve even more complicated problems with ease.
Once the variable is isolated, the remaining calculations will give the final solution. This method not only helps in solving straightforward problems but also builds a solid foundation for tackling more advanced mathematical challenges.
Key Concepts in Section 3
This section introduces fundamental principles essential for mastering mathematical problem-solving. It provides an overview of core techniques that students need to understand in order to work efficiently with different types of equations and expressions. By focusing on these core ideas, learners can build a strong foundation that will help them tackle more complex topics in the future.
Understanding Variables and Operations
One of the key ideas in this section is the concept of variables and how they interact with mathematical operations. Knowing how to manipulate variables in an equation is crucial for finding solutions. By mastering the basic operations–addition, subtraction, multiplication, and division–students can simplify problems and work towards the correct answer with confidence.
Strategies for Simplifying Expressions
Simplifying expressions is another important concept covered in this section. By combining like terms and using distributive properties, learners can reduce complex expressions into simpler forms. This step is critical in making equations easier to solve and ensures that students can progress smoothly to more advanced mathematical challenges.
How to Simplify Algebraic Fractions
This section focuses on the techniques used to simplify mathematical fractions involving variables. The goal is to reduce expressions to their simplest form by eliminating unnecessary terms and common factors. Simplifying these fractions makes it easier to perform calculations and solve problems effectively.
Identifying Common Factors
The first step in simplifying a fraction is identifying common factors in both the numerator and denominator. By factoring out these common terms, you can cancel them out, making the fraction simpler and easier to work with. This process is essential for reducing fractions to their most basic form.
Using the Greatest Common Factor (GCF)
Another important concept is finding the greatest common factor (GCF) of both the numerator and denominator. By dividing both terms by their GCF, you can simplify the fraction significantly. This technique ensures that the fraction is in its simplest and most manageable form, which is especially useful when dealing with more complex equations.
| Expression | Factoring | Simplified Form |
|---|---|---|
| 6x / 9 | 6x / 9 = (2 × 3x) / (3 × 3) | 2x / 3 |
| 10x² / 15x | 10x² / 15x = (5 × 2x) / (5 × 3x) | 2x / 3 |
| 12x²y / 18xy | 12x²y / 18xy = (6 × 2x) / (6 × 3) | 2x / 3 |
Graphing Equations in Section 3
This section introduces the process of graphing mathematical relationships on a coordinate plane. By representing equations visually, students can better understand how variables interact and how changes in one variable affect the other. Graphing provides a clear, intuitive way to solve and analyze equations, making abstract concepts more accessible.
Steps to Graph an Equation
To graph an equation, follow these key steps:
- Identify the variables and their coefficients in the equation.
- Choose a set of values for the independent variable (usually x).
- Calculate the corresponding values of the dependent variable (usually y).
- Plot each pair of values on the coordinate plane.
- Connect the points to reveal the graph of the equation.
Types of Graphs
Different types of equations will produce different types of graphs. Some common graph forms include:
- Linear graphs: Straight lines, typically representing equations in the form of y = mx + b.
- Quadratic graphs: Parabolic curves, often seen in equations like y = ax² + bx + c.
- Exponential graphs: Curves that grow or decay rapidly, representing equations such as y = a * b^x.
Understanding how to graph different types of equations allows students to visualize solutions and better interpret the relationships between variables.
Common Mistakes in Section 3
This section highlights frequent errors that students make when working with mathematical problems. Understanding these common mistakes is crucial, as it allows learners to avoid pitfalls and improve their problem-solving skills. By recognizing these issues early, students can develop better strategies and gain a deeper understanding of the material.
Errors in Solving Equations
One of the most frequent mistakes involves incorrectly isolating the variable. Here are some common errors to watch out for:
- Forgetting to apply the same operation to both sides of the equation.
- Confusing the steps when solving for the variable, leading to incorrect results.
- Incorrectly handling negative signs or fractions during operations.
Graphing Mistakes
When graphing equations, students often make the following mistakes:
- Plotting points incorrectly by miscalculating values.
- Failing to connect the points in the correct sequence, leading to incorrect graphs.
- Forgetting to label axes or not using the correct scale on the coordinate plane.
By identifying these common mistakes, students can take proactive steps to avoid them, ensuring more accurate and successful problem-solving in future tasks.
Practice Problems for Mastery

In this section, students will find practice problems designed to reinforce key concepts and improve problem-solving skills. By working through these exercises, learners can strengthen their understanding and gain confidence in applying the techniques they’ve learned. Regular practice is essential for mastering mathematical principles and building a solid foundation for more advanced topics.
Basic Practice Problems
Start by solving these fundamental problems to test your understanding of the material:
- Solve for x: 2x + 3 = 11
- Simplify: 4x – 5 + 2x
- Graph the equation: y = 2x + 3
Challenging Practice Problems
Once you’re comfortable with the basics, try these more challenging problems:
- Solve for x: 3(x – 4) = 2x + 5
- Simplify the expression: (x² + 2x + 1) / (x + 1)
- Graph the equation: y = x² – 4x + 3
By consistently practicing these problems, you can refine your skills and achieve greater proficiency in solving various types of equations and expressions.
Tips for Efficient Problem Solving
In this section, we provide strategies to improve the efficiency and accuracy of your problem-solving process. Developing the right approach can help you tackle problems more quickly and effectively, reducing the chances of making errors. These tips will guide you through common obstacles and help you refine your skills as you work through mathematical tasks.
Plan Your Approach
Before jumping into solving a problem, take a moment to plan your steps. Identify what is given and what is being asked, then choose the best method to solve it. This will help you avoid confusion and stay focused on the task at hand.
Break Down Complex Problems
If a problem seems overwhelming, break it down into smaller, more manageable parts. Solve each part step by step, and you’ll find that the overall problem becomes much easier to handle.
| Problem | Step 1 | Step 2 | Step 3 |
|---|---|---|---|
| 3x + 5 = 14 | Subtract 5 from both sides: 3x = 9 | Divide both sides by 3: x = 3 | Solution: x = 3 |
| 2(x – 4) = 6 | Expand: 2x – 8 = 6 | Add 8 to both sides: 2x = 14 | Divide by 2: x = 7 |
| (x + 2)² = 16 | Take the square root of both sides: x + 2 = ±4 | Subtract 2 from both sides: x = 2 or x = -6 | Solution: x = 2 or x = -6 |
By breaking down problems into smaller steps, you’ll increase your chances of solving them correctly and more efficiently.
How to Interpret Algebraic Models
Interpreting mathematical models is crucial for understanding real-world problems and finding solutions. Algebraic models, in particular, are often used to represent relationships between variables. By translating these models into concrete terms, you can gain valuable insights into how different factors interact. This process involves analyzing the structure of the equation and understanding the meaning behind each term and variable.
Understanding Variables and Constants
In any algebraic model, variables represent unknown quantities, while constants are fixed values. It’s important to distinguish between these elements, as they help define the relationships within the model. For example, in a formula like y = mx + b, m represents the slope, which shows the rate of change, and b represents the y-intercept, which indicates the starting point on the graph.
Interpreting Relationships in Models
Once you understand the individual components, the next step is to interpret how the variables and constants interact. For example, in a linear equation, the slope dictates how one variable changes in response to another. In contrast, in quadratic models, the relationship may involve more complex factors such as acceleration or curvature. Understanding these interactions helps you apply the model to real-world situations, like predicting future outcomes or analyzing trends.
By practicing these skills, you’ll be better equipped to interpret and solve problems represented by algebraic models.
Strategies for Solving Word Problems
Word problems can often feel overwhelming, but breaking them down into manageable steps makes them easier to tackle. The key to solving these types of problems is to carefully analyze the information provided and identify the relationships between different elements. With a systematic approach, you can simplify complex scenarios and find the solution more effectively.
Step-by-Step Approach
To effectively solve a word problem, follow these essential steps:
- Read the Problem Carefully: Read the problem more than once to ensure you understand the context and what is being asked.
- Identify Key Information: Highlight or underline important numbers, terms, and relationships that will help you form an equation or model.
- Define Variables: Assign symbols to unknown quantities. This will make it easier to set up the problem mathematically.
- Translate Words into Mathematical Expressions: Convert the word problem into an equation or expression that represents the situation.
- Solve the Equation: Use appropriate mathematical techniques to solve the equation or simplify the expression.
- Check Your Work: Once you have an answer, review the problem to ensure the solution makes sense in the context of the question.
Example of Solving a Word Problem
Consider the following example: “A car travels 60 miles per hour for 3 hours. How far will the car travel?” To solve this:
- Identify key information: 60 miles per hour, 3 hours.
- Define the variable: Let d represent the distance.
- Translate into an equation: d = speed × time.
- Substitute the values: d = 60 × 3 = 180 miles.
- Verify the solution: The car travels 180 miles in 3 hours.
By using a consistent approach and taking your time to understand the problem, you can successfully solve word problems with confidence.
Understanding Functions and Their Graphs
Functions are essential concepts in mathematics, representing relationships between two sets of values. A function takes an input, processes it, and produces an output. These relationships are often visualized using graphs, which help to represent the behavior of the function over a given range of values. By analyzing these graphs, you can gain a deeper understanding of how variables interact and how changes in one variable affect another.
When examining a function and its graph, there are several key points to consider. The x-axis represents the independent variable, while the y-axis shows the dependent variable. The graph of a function can take various forms, depending on the type of function being represented. A linear function, for example, produces a straight line, while more complex functions can create curves or other shapes.
Key Features of a Function’s Graph
There are several important features to observe when interpreting a function’s graph:
- Intercepts: The points where the graph crosses the axes. The x-intercept occurs where y = 0, and the y-intercept occurs where x = 0.
- Slope: In the case of linear functions, the slope indicates the rate of change between the variables. It is calculated as the rise over run (change in y divided by change in x).
- Domain and Range: The domain represents all possible input values (x-values), while the range includes all possible output values (y-values).
Analyzing Graphs of Functions
Understanding the graph of a function is crucial for interpreting real-world problems. For example, a straight-line graph suggests a constant rate of change, while a curve can indicate acceleration or deceleration in a system. By identifying the type of function and its graph, you can make predictions and solve problems more efficiently. Whether the function is linear, quadratic, or another form, interpreting the graph allows for a deeper understanding of the underlying relationship between variables.
Reviewing Key Vocabulary and Terms
Understanding the fundamental terminology used in mathematics is crucial for solving problems effectively. Each concept has its own set of specific terms that define relationships, operations, and techniques. By reviewing these terms regularly, you can develop a deeper understanding of the material and approach problems with greater confidence. This section highlights key terms that are commonly used in solving mathematical expressions and equations, ensuring a stronger grasp of the subject matter.
Important Terms to Know
Here is a table of essential vocabulary terms and their meanings that are frequently encountered when working with mathematical models:
| Term | Definition |
|---|---|
| Variable | A symbol, often a letter, used to represent an unknown value or a value that can change. |
| Expression | A mathematical phrase that can include numbers, variables, and operators but does not have an equal sign. |
| Equation | A statement that asserts the equality of two expressions, often containing variables. |
| Coefficient | A numerical factor that multiplies a variable in an expression or equation. |
| Constant | A fixed value that does not change and does not contain any variables. |
| Solution | The value or values that satisfy an equation or inequality. |
| Intercept | The point where a graph crosses an axis, either the x-axis or the y-axis. |
Understanding the Significance
Familiarity with these terms allows for more efficient problem-solving and clearer communication when discussing mathematical concepts. By regularly reviewing and applying these definitions, you can enhance your ability to solve equations, interpret graphs, and apply concepts to real-world situations. Mastery of vocabulary is a foundational skill that supports success in higher levels of mathematics and related fields.
Application of Algebra in Real Life
Mathematical concepts play a crucial role in everyday life, influencing decisions and problem-solving in various fields. The ability to manipulate numbers and solve equations is not just for the classroom–these skills are used in countless real-world situations. From managing finances to analyzing data, mathematical reasoning helps individuals make informed choices and understand complex relationships. Understanding how abstract concepts apply to practical scenarios can make these ideas more tangible and relevant.
Financial Planning
One of the most common uses of mathematical principles is in personal finance. Whether it’s calculating interest rates, budgeting monthly expenses, or saving for future goals, mathematical models provide the tools to evaluate options and plan effectively. For example, understanding how compound interest works can help determine the best investment strategy.
Engineering and Architecture
In fields like engineering and architecture, mathematical principles are applied to design structures, optimize resources, and solve practical challenges. Calculations for load-bearing, area, and volume ensure that buildings, bridges, and other structures are both safe and cost-effective. Algebraic techniques are used to design systems that meet specific requirements, from roads to electrical grids.
Data Analysis
Many industries rely on data to make decisions. Whether it’s market trends, customer behavior, or scientific research, mathematical models help interpret and predict patterns. Algebra is often used to understand relationships between variables and identify key factors influencing outcomes. Statistical analysis and regression models rely on algebraic skills to analyze large datasets.
Everyday Problem-Solving
In daily life, algebraic thinking can be applied to solve various challenges. For instance, when planning a road trip, determining fuel efficiency, or calculating travel time, algebra can help find the most efficient routes or estimate costs. Whether you’re cooking, shopping, or planning an event, mathematical reasoning is always there to assist with decision-making.
By recognizing the practical applications of algebra, individuals can appreciate the value of learning these concepts and apply them effectively in a variety of situations. The ability to solve real-world problems with mathematical reasoning can lead to smarter, more confident decisions across all aspects of life.
Using Tools for Algebraic Solutions
In the study of mathematical equations, there are various tools and methods available to simplify and solve complex problems. These tools not only aid in quick calculations but also help in visualizing and understanding abstract concepts. By utilizing these resources, learners can streamline their approach to problem-solving and achieve more accurate and efficient results. Whether it’s using calculators, software, or graphing techniques, these tools are essential in gaining deeper insights and mastering mathematical principles.
Popular Tools for Problem-Solving
- Scientific Calculators: These devices are equipped with advanced functions, allowing users to perform complex operations such as solving equations, working with fractions, and calculating exponents. They are invaluable for solving multi-step problems quickly and accurately.
- Graphing Calculators: A step up from standard calculators, graphing calculators allow users to graph equations and visualize the relationships between variables. This helps in understanding the behavior of functions and finding intersections or solutions more easily.
- Mathematical Software: Programs like Wolfram Alpha or GeoGebra offer robust solutions for solving algebraic equations, graphing functions, and performing symbolic manipulations. They can handle a variety of mathematical tasks, from basic equations to more complex integrals.
- Online Tools and Apps: Various online platforms and apps provide tools for solving algebraic problems, offering step-by-step solutions and explanations. These can be particularly useful for learners who need extra practice or clarification on specific topics.
Graphical and Visual Tools
One of the most powerful ways to understand equations is through visualization. Graphs provide a visual representation of equations, making it easier to identify trends and solutions. Some of the key tools for graphical analysis include:
- Graphing Paper: Traditional graphing paper is still an effective way to manually plot equations and observe their behavior. It encourages hands-on learning and helps reinforce the connection between algebraic expressions and their graphical representations.
- Online Graphing Tools: Websites such as Desmos allow for instant graphing of equations, enabling students to explore different types of functions and their properties. These tools offer an interactive way to experiment with variables and understand their effects on the graph.
- Interactive Whiteboards: In classrooms, interactive whiteboards can be used to demonstrate solving equations and graphing functions in real-time. This provides an engaging and collaborative learning environment where students can participate and visualize problem-solving steps.
Incorporating these tools into daily study routines enhances learning and problem-solving skills. They provide immediate feedback, guide learners through the solution process, and support the development of a deeper understanding of mathematical concepts.
Test Preparation for Chapter 3
Preparing for an upcoming assessment requires careful review and focused practice. The key to mastering the material is understanding the concepts and applying them effectively in various scenarios. Whether you’re studying individually or with a group, it’s essential to approach the test with a clear strategy. By identifying the core topics and practicing problem-solving techniques, you can build confidence and improve your performance.
Effective Review Strategies
- Review Key Concepts: Start by revisiting the main ideas covered in the lessons. Pay attention to definitions, properties, and theorems that are central to the subject matter. Understanding the foundational concepts will help you solve problems more efficiently.
- Practice Problem Sets: The best way to reinforce your understanding is by solving practice problems. Focus on a variety of questions, from basic exercises to more complex challenges. This will help you become familiar with the types of problems you may encounter on the test.
- Identify Common Mistakes: Review past errors and understand where you went wrong. Whether it’s a miscalculation or misunderstanding a concept, recognizing your mistakes is essential for avoiding them in the future.
- Time Yourself: As you practice, set a time limit for each problem to simulate test conditions. This will help you manage your time during the real assessment and avoid rushing through questions.
Test-Taking Tips
- Read Questions Carefully: On the test day, read each question thoroughly before attempting to solve it. Ensure you understand what is being asked before jumping into calculations.
- Work Through Step-by-Step: Break down each problem into smaller, manageable steps. This approach ensures that you don’t miss important details and helps you stay organized during the test.
- Check Your Work: If time permits, review your answers before submitting the test. Double-check calculations and ensure that you’ve answered all parts of the question correctly.
By following these strategies and practicing consistently, you’ll be well-prepared to tackle any questions that come your way. A structured approach to preparation will not only improve your skills but also boost your confidence during the exam.