HS Chemistry POGIL Activity Basic Stoichiometry Answers

In the study of chemical processes, one of the most important aspects is understanding how different substances interact with one another to produce new compounds. This involves applying mathematical techniques to predict quantities and outcomes of these transformations. By mastering these skills, students can develop a deeper insight into the underlying principles that govern chemical changes and learn to approach complex problems systematically.
Learning how to solve these problems effectively requires familiarity with essential tools such as conversion factors and mole relationships. These techniques allow students to bridge the gap between theoretical knowledge and practical applications. With practice, learners become adept at calculating the amount of products or reactants involved in various processes, ensuring accurate predictions for laboratory and industrial scenarios.
As students progress, they encounter more intricate examples that test their ability to think critically and apply learned concepts in real-world contexts. Success in these challenges depends on understanding not just the formulas, but also the reasoning behind each step. This foundation enables learners to build confidence in their problem-solving abilities and approach new topics with curiosity and clarity.
HS Chemistry POGIL Activity Basic Stoichiometry Answers
In this section, we explore key concepts that help students navigate the mathematical side of chemical processes. The focus is on understanding how to quantify substances involved in reactions and calculate the required amounts of reactants and products. By honing these skills, learners can develop a more thorough grasp of the transformation processes that occur in labs and industry.
Throughout this process, students will encounter various tools that aid in breaking down complex problems into manageable steps. Using relationships between different components of a reaction, learners can predict how much of each substance will be consumed or produced, ensuring the correct balance is maintained. This approach promotes a methodical way of solving problems, helping students develop both analytical and computational skills.
By engaging with these types of exercises, students become better equipped to apply their understanding to real-world scenarios. Mastering these calculations enhances their ability to approach new challenges with confidence, as they build a strong foundation for more advanced topics in the field.
Understanding Stoichiometry in Chemistry
In the study of chemical reactions, understanding the relationships between the quantities of different substances is crucial. This area focuses on how to calculate the amounts of reactants needed and products formed in various reactions. Mastering these concepts is essential for accurately predicting the outcomes of chemical processes in both laboratory settings and industrial applications.
At its core, this topic revolves around converting between different units and using mathematical relationships to maintain the balance between reactants and products. By doing so, it ensures that reactions occur in the correct proportions, maximizing efficiency and minimizing waste. Key concepts include:
- Mole ratios that relate the amounts of substances involved in a reaction
- Conversion factors used to switch between units like grams, moles, and liters
- Limiting reactants that determine the maximum amount of product that can be formed
- Excess reactants that remain after the reaction has completed
By learning how to apply these principles, students can predict the quantities of substances involved in reactions with greater accuracy. This systematic approach helps not only in theoretical problems but also in real-world applications where precise measurements are essential.
POGIL Approach to Learning Chemistry
The method of learning that emphasizes active participation and guided discovery is a powerful tool for developing a deeper understanding of scientific concepts. This approach allows students to engage directly with the material, uncovering the relationships and principles that govern chemical processes. Rather than passively absorbing information, learners are encouraged to think critically, collaborate with peers, and apply concepts to real-world scenarios.
Key Elements of the Approach
Through structured activities, learners explore core concepts in a collaborative environment. Key aspects of this approach include:
- Interactive Problem Solving: Students work through challenges that require them to apply their knowledge and reasoning skills.
- Collaborative Learning: Working with peers fosters deeper understanding through discussion and shared insights.
- Guided Discovery: Instead of being told the answers, students are led to uncover principles on their own through targeted questions and exercises.
Benefits of the Approach
This learning method is particularly effective for mastering complex topics. By actively engaging in the material, students develop a stronger grasp of the subject and are better equipped to tackle difficult problems. Some of the benefits include:
- Improved Critical Thinking: Learners become adept at analyzing and solving problems independently.
- Enhanced Retention: Active involvement leads to better long-term retention of concepts.
- Better Preparation for Future Challenges: The approach builds the skills necessary to approach new and more advanced topics with confidence.
Key Concepts in Stoichiometry Explained
Understanding the relationships between substances in chemical reactions is fundamental for solving many types of problems in the field. By calculating the amount of reactants required or products formed, it becomes possible to predict the outcomes of reactions accurately. These calculations are essential in ensuring that reactions proceed efficiently and in the right proportions, whether in a laboratory or industrial setting.
At the heart of these calculations are several core concepts that must be mastered. The first is the concept of mole ratios, which describe the proportional relationship between reactants and products in a balanced equation. This allows students to determine how much of each substance will be involved in a reaction.
Another critical idea is the use of conversion factors to switch between different units, such as grams, liters, and moles. This is essential for making the correct calculations and ensuring that all quantities are expressed in consistent units.
Additionally, limiting reactants play a key role in determining how much product can be formed. The limiting reactant is the substance that runs out first, thus limiting the overall amount of product. Understanding how to identify the limiting reactant is crucial for accurately predicting the yield of a reaction.
By mastering these fundamental principles, students can solve complex problems and apply their knowledge to practical situations with greater ease and precision.
Steps to Solve Stoichiometry Problems
Solving problems involving the quantities of substances in chemical reactions requires a structured approach. By following a series of logical steps, students can ensure they calculate the correct amounts of reactants or products involved. This methodical process helps simplify complex problems and ensures accurate results.
The first step is to balance the chemical equation. This ensures that the number of atoms of each element is the same on both sides of the reaction, providing a correct starting point for calculations.
Next, convert the given information into moles, as this is the standard unit used for chemical calculations. Whether you are given mass, volume, or another unit, it is essential to convert it into moles using appropriate conversion factors.
After that, use mole ratios from the balanced equation to relate the moles of the known substance to the moles of the unknown substance. This step involves applying the coefficients of the balanced equation to determine the proportional amounts of reactants and products.
Once you have the correct ratio, convert moles back to the desired units, such as grams or liters, depending on the problem’s requirements. This final conversion step ensures that the result is in the correct format for interpretation or application.
By following these systematic steps, students can solve stoichiometric problems with greater accuracy and confidence, applying these principles to a wide variety of chemical reactions and scenarios.
Types of Stoichiometric Calculations
When performing calculations related to chemical reactions, there are several types of problems that can be encountered. Each type requires a different approach depending on the information provided and the goal of the calculation. By understanding the various types, students can develop a more comprehensive ability to tackle complex scenarios.
Here are the most common types of calculations that arise in chemical processes:
- Mass-to-Mass Calculations: These calculations involve converting the mass of one substance to the mass of another substance in a reaction. The process includes using the molar mass of the substances and mole ratios to determine the required amounts.
- Mass-to-Mole Calculations: In this case, the mass of a substance is converted to moles, which are then used to calculate the amount of a related substance in moles. This is a common type of calculation when determining quantities needed for a reaction.
- Mole-to-Mole Calculations: These problems focus on finding the number of moles of one substance when given the number of moles of another substance. The mole ratio from the balanced equation is used to relate the amounts.
- Volume-to-Volume Calculations: For reactions involving gases, volume-to-volume calculations can be made using the ideal gas law or molar volume at standard conditions. This calculation relates the volumes of gaseous reactants and products in a reaction.
- Limiting Reactant Calculations: These problems involve determining which reactant is used up first in a reaction. The limiting reactant determines how much product can be formed and helps calculate the maximum yield of the reaction.
- Yield Calculations: This type includes determining the actual yield of a product and comparing it to the theoretical yield to calculate the percent yield. It is essential for evaluating the efficiency of a chemical reaction.
Each type of calculation provides valuable insight into different aspects of chemical reactions. By practicing these calculations, students can enhance their understanding of how substances interact and improve their ability to predict outcomes accurately.
Balancing Chemical Equations for Stoichiometry
Balancing equations is a crucial step in understanding how substances interact in a reaction. Without properly balanced equations, it becomes impossible to accurately determine the quantities of reactants and products involved. By ensuring that the number of atoms on both sides of the equation is the same, we can maintain the law of conservation of mass and proceed with accurate calculations for the amounts of each substance involved.
Importance of Balancing
Balancing ensures that the chemical equation accurately represents the physical reality of a reaction. It allows us to relate the amounts of reactants to the amounts of products in a way that reflects the true proportions in which they combine or break apart. This is essential for making reliable predictions and solving problems related to reaction yields and quantities.
Steps to Balance an Equation
There are several key steps to follow when balancing an equation:
- Write the unbalanced equation: Start with the correct chemical formulas of the reactants and products.
- Balance one element at a time: Begin with the most complex molecule, adjusting coefficients to balance the atoms of each element on both sides.
- Check for mass balance: Ensure that the number of atoms for each element is the same on both sides of the equation.
- Adjust coefficients as necessary: Make sure the smallest whole number coefficients are used to balance the equation.
Once the equation is balanced, it is ready for use in stoichiometric calculations. This balanced form will provide the correct mole ratios needed to relate reactants to products and determine quantities involved in the reaction.
Using Moles in Stoichiometric Calculations
In any reaction, understanding the relationship between the amounts of reactants and products is key to performing accurate calculations. Moles provide a standardized way to express quantities of substances, making it easier to work with chemical reactions. By converting masses or volumes into moles, and then using mole ratios from a balanced equation, we can predict how much of a product will form or how much of a reactant is needed.
The Role of Moles
Moles act as a bridge between the mass of a substance and the number of particles involved in a reaction. This concept is essential for making conversions in chemical calculations. Here’s why moles are so important:
- Standardization: Moles allow for consistent comparisons between different substances, regardless of their chemical nature or physical state.
- Atomic and Molecular Scale: Using moles, we can work with quantities of atoms, molecules, or ions in a practical and measurable way, even when dealing with extremely small amounts.
- Conversion of Units: Moles enable the conversion of mass (grams) and volume (liters) to a common unit that can be used in further calculations.
Steps to Use Moles in Calculations
To effectively use moles in stoichiometric calculations, follow these general steps:
- Convert mass to moles: Use the molar mass of the substance to convert the given mass into moles.
- Use mole ratios: From the balanced equation, apply the appropriate mole ratio to find the relationship between the known and unknown substances.
- Convert moles to desired units: After calculating the moles of a substance, convert them into the required units (grams, liters, etc.) using the molar mass or molar volume as needed.
By following these steps, moles allow you to simplify complex chemical reactions and solve for quantities of interest with greater accuracy and ease.
Conversion Factors in Stoichiometry
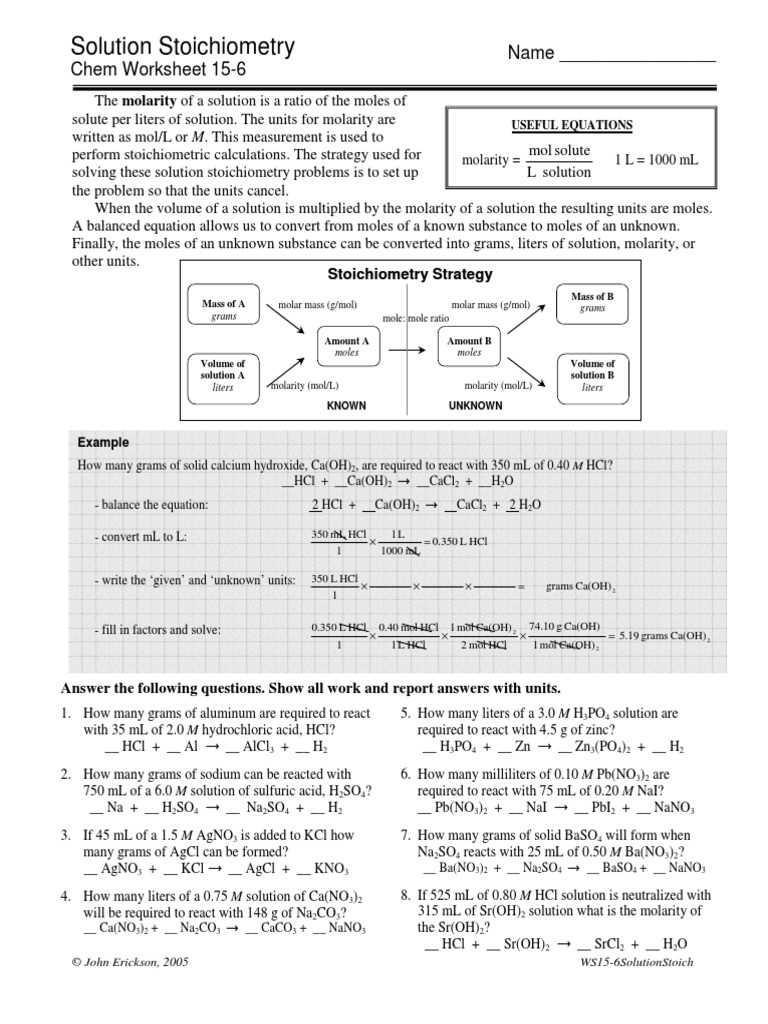
In chemical calculations, conversion factors play a crucial role in transforming one set of units into another. These factors are essential for ensuring that the units in an equation match and that calculations are done accurately. By using the appropriate conversion factors, we can convert between mass, volume, moles, and other units, making it easier to work with different substances and chemical reactions.
Conversion factors are derived from known relationships between different quantities. These relationships allow us to express quantities in different units while keeping the calculations consistent. Understanding how to apply these factors is key to solving a wide variety of chemical problems.
Common Conversion Factors
Below are some of the most frequently used conversion factors in chemical calculations:
- Molar Mass: The molar mass of a substance (in grams per mole) is used to convert between grams and moles.
- Avogadro’s Number: This number (6.022 × 1023) is used to convert between moles and individual particles (atoms, molecules, etc.).
- Gas Volume at STP: At standard temperature and pressure, one mole of an ideal gas occupies 22.4 liters. This is useful for converting between moles and volume of gases.
- Density: Density (grams per milliliter or grams per liter) allows conversion between mass and volume for liquids and gases.
Using Conversion Factors
To apply conversion factors in calculations, follow these general steps:
- Identify the given quantity and units: Determine what information is provided and what needs to be converted.
- Select the appropriate conversion factor: Choose the factor that connects the given quantity to the desired units.
- Set up the calculation: Multiply or divide by the conversion factor, ensuring that units cancel out properly to give the final result.
By using conversion factors correctly, you can simplify complex problems and make accurate predictions about the amounts of reactants and products involved in a chemical reaction.
Limiting Reactant in Stoichiometry Problems
In chemical reactions, the limiting reactant is the substance that determines how much product can be formed. When two or more reactants are involved, one of them will be completely consumed first, halting the reaction. This reactant is known as the limiting reactant, and it plays a crucial role in calculating the yield of a reaction. Understanding how to identify and work with the limiting reactant is essential for solving many types of chemical problems.
Identifying the Limiting Reactant

To find the limiting reactant, follow these general steps:
- Calculate the moles of each reactant: Use the given quantities (masses or volumes) of each reactant and convert them to moles using the molar mass or appropriate conversion factors.
- Determine the mole ratio: Using the balanced equation, identify the required ratio of reactants needed for the reaction to proceed.
- Compare the available moles to the required ratio: The reactant that runs out first is the limiting reactant. This is the one that determines how much product will be formed.
Impact of the Limiting Reactant
The limiting reactant sets an upper limit on the amount of product that can be formed. Once the limiting reactant is consumed, the reaction stops, even if other reactants are still available. Therefore, calculating the limiting reactant is essential for accurately predicting the amount of product produced in a reaction.
By understanding the concept of the limiting reactant, you can avoid wasting reactants and make precise predictions about the outcome of a reaction. This is especially useful in laboratory settings or industrial processes where maximizing efficiency is crucial.
Excess Reactant and Stoichiometry
In a chemical reaction, the excess reactant is the substance that remains after the reaction has gone to completion. Unlike the limiting reactant, which is entirely consumed during the reaction, the excess reactant is left over. Understanding how to account for the excess reactant is vital for determining the efficiency of a reaction and for practical applications such as reagent optimization and waste reduction in laboratory and industrial settings.
After identifying the limiting reactant and calculating the amount of product formed, the next step is to determine how much of the excess reactant is used up in the reaction. The remaining excess reactant can then be calculated and measured, providing insight into how much material was unnecessary for the reaction to proceed.
Calculating the Excess Reactant
To calculate the amount of excess reactant remaining, follow these steps:
- Determine the amount of excess reactant required by using the limiting reactant and the mole ratio from the balanced equation.
- Calculate how much excess reactant has been consumed based on the limiting reactant.
- Subtract the amount consumed from the initial amount of the excess reactant to find the leftover quantity.
Example of Excess Reactant Calculation
Below is an example of how to calculate the excess reactant in a chemical reaction:
| Substance | Initial Amount (moles) | Amount Consumed (moles) | Remaining Amount (moles) |
|---|---|---|---|
| Reactant A (Limiting) | 3.0 | 3.0 | 0.0 |
| Reactant B (Excess) | 5.0 | 3.0 | 2.0 |
In this example, Reactant A is the limiting reactant, and Reactant B is the excess reactant. After the reaction, 2.0 moles of Reactant B remain unreacted.
By understanding how to calculate and manage excess reactants, you can optimize reactions, reduce waste, and use materials more efficiently in both educational and industrial environments.
The Role of Mole Ratios
In any chemical reaction, the relationship between reactants and products is governed by specific proportions. These proportions, often referred to as mole ratios, determine how much of each substance is required or produced. By understanding and applying these ratios, one can predict the quantities of materials needed or formed, ensuring that reactions are carried out efficiently and accurately. Mole ratios are an essential tool for solving various reaction-based problems, especially when working with limited amounts of reactants.
The mole ratio comes from the coefficients in a balanced chemical equation, reflecting the proportions of each substance involved. These ratios allow chemists to convert between different substances in a reaction, making it possible to determine how much of a given reactant will produce a certain amount of product or how much product can be made from a given amount of reactant.
Using Mole Ratios in Calculations
To use mole ratios in calculations, you must first identify the relevant reactants and products involved in the equation. Then, using the mole ratio derived from the balanced equation, you can perform conversions between substances. Below is a simplified approach:
- Write the balanced equation for the reaction.
- Identify the mole ratio between the reactant and the product of interest.
- Use the mole ratio to convert moles of one substance to moles of another, ensuring the correct proportions are followed.
Example of Mole Ratios in Action
Consider the following balanced equation:
| Substance | Coefficient |
|---|---|
| 2 H2 + O2 → 2 H2O | 2 : 1 : 2 |
This equation tells us that two moles of hydrogen gas react with one mole of oxygen gas to produce two moles of water. If you have 4 moles of hydrogen, you would need 2 moles of oxygen to fully react with it. Using the mole ratio allows you to calculate how much product can be produced or how much reactant is required based on the given quantities.
By mastering the use of mole ratios, you can solve a wide range of reaction-related problems, from determining the required amounts of reactants to predicting the yield of products. This concept is fundamental for effective planning and execution of chemical reactions in both theoretical and practical settings.
Calculating Theoretical Yield
In any chemical reaction, it is often essential to determine the maximum amount of product that can be obtained based on the quantities of reactants. This calculation, known as theoretical yield, helps predict the outcome of a reaction under ideal conditions, where all reactants are completely converted into products with no loss or side reactions. Theoretical yield serves as a benchmark for assessing the efficiency of a reaction and comparing it with the actual yield obtained.
To calculate the theoretical yield, you need to follow a series of steps that involve understanding the relationship between the reactants and products, typically through the use of mole ratios. These ratios, derived from the balanced chemical equation, allow you to convert the amount of limiting reactant into the maximum possible amount of product. The limiting reactant is the substance that determines the maximum amount of product that can be formed in the reaction.
Steps to Calculate Theoretical Yield
Follow these steps to determine the theoretical yield of a reaction:
- Write the balanced equation: Ensure that the chemical equation is balanced, as this provides the necessary mole ratios.
- Identify the limiting reactant: Determine which reactant will be completely used up first and thus limit the amount of product formed.
- Convert the limiting reactant to moles: If necessary, convert the mass of the limiting reactant into moles using its molar mass.
- Use the mole ratio to find the moles of product: Use the appropriate mole ratio from the balanced equation to calculate how many moles of product can be produced from the limiting reactant.
- Convert moles of product to grams: Finally, if the product is required in grams, multiply the moles of product by its molar mass to obtain the theoretical yield in grams.
Example Calculation
Consider the following balanced equation for the reaction between hydrogen gas and oxygen gas to form water:
| Substance | Coefficient |
|---|---|
| 2 H2 + O2 → 2 H2O | 2 : 1 : 2 |
Suppose you have 4 moles of hydrogen gas and an excess amount of oxygen. To find the theoretical yield of water, you would follow these steps:
- Use the mole ratio from the balanced equation: For every 2 moles of hydrogen, 2 moles of water are produced.
- Since you have 4 moles of hydrogen, the theoretical yield of water would be 4 moles of water.
- If the problem asks for the mass of water, multiply the moles of water (4 moles) by the molar mass of water (18 g/mol) to get the theoretical yield in grams: 4 moles × 18 g/mol = 72 g.
By using the theoretical yield calculation, you can estimate how much product you should obtain under perfect conditions. Comparing the theoretical yield with the actual yield obtained from a reaction helps assess the reaction’s efficiency and pinpoint areas for improvement in real-world applications.
Understanding Percent Yield
In any reaction, the actual amount of product obtained often differs from the theoretical yield due to various practical factors such as incomplete reactions, side reactions, and losses during handling. To evaluate the efficiency of a reaction, chemists calculate the percent yield, which expresses the ratio of the actual yield to the theoretical yield as a percentage. This comparison provides insight into how well the process is working and whether improvements can be made.
Percent yield is a useful measure in both laboratory and industrial settings. It indicates how much of the expected product was actually produced and helps determine if the reaction conditions are optimal or if adjustments are necessary. A high percent yield suggests a well-controlled reaction, while a low percent yield can point to inefficiencies or issues in the reaction process.
Formula for Percent Yield
The percent yield is calculated using the following formula:
Percent Yield = (Actual Yield / Theoretical Yield) × 100
Where:
- Actual Yield: The amount of product actually obtained from the reaction, measured in grams or moles.
- Theoretical Yield: The maximum amount of product that could be produced, calculated based on the limiting reactant and the balanced equation.
Example Calculation
Suppose a reaction yields 80 grams of product, but the theoretical yield was calculated to be 100 grams. The percent yield would be:
Percent Yield = (80 g / 100 g) × 100 = 80%
This means that 80% of the expected product was obtained under the given conditions, and the remaining 20% was lost due to various factors such as inefficiency or experimental errors.
Understanding percent yield is essential for optimizing chemical reactions, whether in research labs or large-scale production. It allows chemists to identify areas of improvement, minimize waste, and ensure more effective use of resources.
Real-World Applications of Stoichiometry
The principles of balancing and calculating chemical reactions extend far beyond the classroom and are crucial in numerous real-world fields. From manufacturing processes to environmental protection, understanding how materials interact and how to calculate their quantities ensures that resources are used efficiently, and reactions proceed as intended. Whether in industrial production or environmental conservation, these calculations are integral to solving complex problems and optimizing processes.
In everyday life, many industries rely on precise measurements to control chemical processes and minimize waste. In sectors such as pharmaceuticals, energy production, and agriculture, accurate calculations of reactant and product amounts help companies maximize efficiency, reduce costs, and meet safety regulations. These applications highlight the practical value of theoretical knowledge in tackling real-world challenges.
Pharmaceutical Industry
In the pharmaceutical industry, the synthesis of drugs requires exact quantities of reactants to ensure that the desired product is produced in the correct amount. By applying these concepts, drug manufacturers can calculate the precise number of molecules needed for a reaction, ensuring that the drug is both effective and safe for human use. Understanding the limitations of reactants also helps avoid unnecessary waste and expensive raw material losses.
Environmental Impact and Sustainability
Another important application is in environmental science, where understanding the ratios of various compounds can help mitigate pollution. For example, when treating waste or neutralizing harmful chemicals, stoichiometric calculations are essential to ensure that the correct amount of chemicals is used to safely and completely react with contaminants. This approach helps to minimize the environmental footprint of industrial processes and waste management efforts.
Real-world applications of these fundamental calculations are all around us, contributing to the efficiency and sustainability of many industries. From designing cleaner energy systems to producing life-saving medications, the ability to predict and control chemical reactions is essential in driving innovation and improving quality of life.
Common Mistakes in Stoichiometry
While mastering the calculations involved in chemical reactions is essential, it’s common for individuals to make mistakes that can lead to incorrect results. These errors can stem from misunderstanding basic concepts, misapplying formulas, or simply overlooking details during problem-solving. Recognizing and avoiding these mistakes is key to improving accuracy and efficiency in calculations.
Many errors arise from failing to properly balance the equations before starting calculations. Without a balanced equation, the ratio between reactants and products cannot be accurately determined, leading to incorrect mole conversions. Another common mistake is misinterpreting the units, which can result in improper conversions and misleading results.
Incorrect Unit Conversion
One of the most frequent mistakes in this area is failing to correctly convert between different units. For example, converting grams to moles or vice versa requires careful attention to the molar mass of substances. Using the wrong conversion factor or forgetting to convert all necessary units can throw off the entire calculation.
Assuming Excess Reactant
Another common issue is neglecting to properly account for limiting and excess reactants. In many cases, assuming an excess of one reactant without performing the necessary calculations can lead to an inaccurate estimate of the product produced. It is crucial to determine the limiting reactant first, as it dictates the maximum possible yield in the reaction.
By being aware of these common mistakes, individuals can approach problems with greater care and precision. Ensuring a balanced equation, accurate conversions, and a correct understanding of reactant limitations are fundamental steps in obtaining correct results in chemical calculations.
Tips for Mastering Stoichiometry Problems
Solving chemical reaction problems involving quantities of reactants and products can often feel challenging. However, with the right approach, it’s possible to simplify these calculations and solve them with confidence. Here are some practical tips to help master this skill and minimize mistakes when tackling complex problems.
One of the first steps in mastering these calculations is ensuring a clear understanding of the relationships between the substances involved in the reaction. Properly identifying and interpreting these relationships allows you to convert units and find unknown quantities more effectively. Following a systematic approach, such as working through each step methodically, can greatly improve accuracy.
Key Strategies for Success
To improve problem-solving skills, it’s essential to practice consistent habits and focus on key areas. Here are some useful strategies:
- Balance the Reaction First: Always ensure that the equation is properly balanced. This step is crucial for accurate mole ratios and conversions between reactants and products.
- Organize the Information: Write down all known quantities and units clearly, and pay close attention to the units as you proceed through the calculations.
- Use Conversion Factors Wisely: When converting from one unit to another, ensure the correct molar masses or constants are used. Units must cancel out properly to avoid errors.
- Check for Limiting Reactants: Make sure to identify the limiting reactant before performing any calculations. This will determine the maximum amount of product that can be formed.
Visualizing the Problem
Sometimes, it helps to visually organize the problem. Drawing diagrams or tables can provide clarity and help you avoid overlooking important details. For example, a table can help you keep track of the amounts of reactants and products involved and simplify the conversion process.
| Substance | Amount (grams) | Moles |
|---|---|---|
| Reactant 1 | 10.0 g | 0.05 mol |
| Reactant 2 | 15.0 g | 0.075 mol |
| Product | 0.05 mol |
By consistently applying these strategies and organizing your approach, you will become more comfortable and efficient in solving these types of problems. Mastery comes with practice, and over time, the process will become more intuitive, leading to quicker and more accurate results.
How POGIL Enhances Learning
This approach to learning promotes deep understanding and critical thinking by actively engaging students in the problem-solving process. Instead of passively receiving information, learners become part of the process, applying concepts, analyzing data, and drawing conclusions. This active participation helps them internalize knowledge and develop problem-solving skills that are crucial for mastering complex concepts.
By focusing on group collaboration and inquiry-based learning, students are encouraged to think critically and communicate their reasoning. This method strengthens their ability to apply theoretical knowledge to practical situations. The key benefit of this approach is that it allows learners to build on their own experiences and insights, creating a more personalized and effective learning experience.
In addition to fostering individual problem-solving skills, this method also enhances teamwork. Working in groups allows students to discuss ideas, clarify doubts, and learn from each other’s perspectives. This interaction creates a dynamic environment where ideas can be challenged and refined, leading to a deeper understanding of the material.