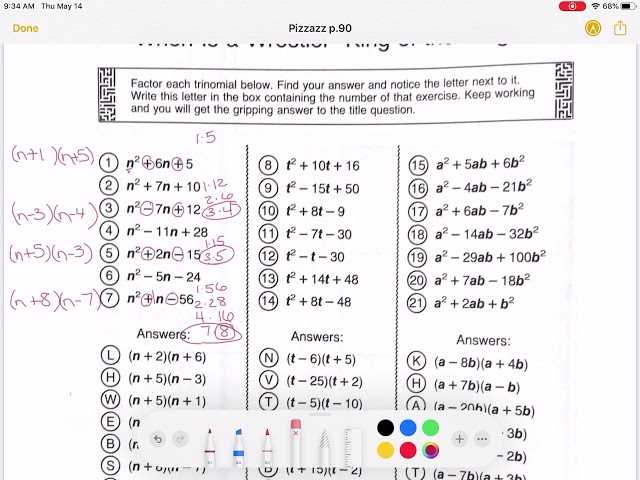
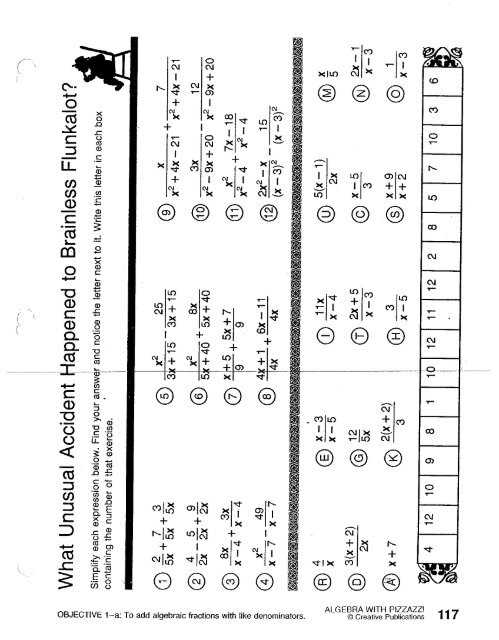
Creative Publications Algebra with Pizzazz Answers Key

Effective learning in mathematics relies on understanding and practicing core concepts. When faced with complex tasks, having a resource that guides you step by step can significantly boost your confidence and competence. This approach is designed to help learners navigate challenging mathematical exercises and gain a deeper understanding of the methods used to solve them.

In this section, you will find a comprehensive guide that supports the process of tackling different types of problems. By focusing on clear, detailed solutions and systematic explanations, this tool makes it easier to break down each challenge into manageable steps. Whether you are a student looking to reinforce your skills or someone seeking a better grasp of key techniques, this guide offers valuable insights for improving your problem-solving abilities.

Empower yourself with the right strategies and techniques, making each problem-solving journey a step closer to mastering mathematical skills. Whether you’re looking to work independently or enhance your study sessions, these resources will provide you with the tools to succeed.

Understanding the Algebra with Pizzazz Approach

This method emphasizes an engaging and structured way of tackling mathematical problems. It integrates creativity and logic to make complex tasks more accessible and enjoyable. By focusing on foundational techniques and presenting them through an interactive style, this approach encourages deeper understanding and active participation in learning.

Key elements of this approach include:

• Clear, step-by-step guidance for solving problems
• Engaging examples designed to maintain interest
• Practical exercises that reinforce core concepts

Students are encouraged to explore different problem-solving strategies, gaining confidence as they progress through various levels of difficulty. The goal is to create a supportive environment where learners feel motivated to think critically and apply what they’ve learned in real-world scenarios.

By using a variety of methods, this approach caters to different learning styles, making it a versatile tool for a wide range of students. Whether you’re a beginner or looking to refine your skills, this system provides a balanced mix of challenge and support to guide you towards success.

Exploring Creative Publications Algebra Resources

In order to build a solid foundation in mathematics, it’s important to have access to reliable and varied resources. These materials not only offer practical exercises but also provide essential guidance that helps learners understand fundamental principles and enhance their problem-solving abilities. The right resources can make a huge difference in grasping complex concepts and fostering a deeper interest in mathematical topics.

Types of Resources Available

The materials available in this system come in a variety of formats, each catering to different learning preferences and needs. Some offer detailed examples, while others focus on hands-on activities to help reinforce the lessons learned. Below is an overview of the most common types of resources:

Resource Type	Description	Purpose
Practice Problems	Challenging tasks designed to test understanding	Improve problem-solving skills
Step-by-Step Guides	Detailed explanations for solving specific problems	Provide clarity and support during learning
Interactive Exercises	Activities that engage learners through practice	Promote active participation and learning

How These Resources Support Learning

These materials are designed not only to support individual learning but also to be used in group study settings. Whether you’re revisiting a concept or tackling a new challenge, having access to a variety of resources ensures that you’re equipped to tackle any problem. By regularly practicing and using these tools, learners can continuously build on their knowledge and gain the confidence needed to solve even the most difficult tasks.

How to Use the Answer Key Effectively

Maximizing the benefits of any solution guide requires a thoughtful approach. While it’s tempting to jump directly to the correct answers, it’s important to view these resources as a learning tool, not just a shortcut. The goal should be to understand the steps that lead to the solution, rather than simply memorizing the final result. By analyzing how each problem is solved, you can improve your problem-solving skills and reinforce your understanding of the underlying concepts.

One of the most effective ways to use the guide is to work through problems on your own first. Once you’ve attempted a task, compare your method and solution with the steps outlined in the resource. Focus on the reasoning behind each step and how it connects to the next. This process will help you identify any mistakes and understand why they occurred, giving you a clearer picture of the correct approach.

Additionally, it’s helpful to revisit the guide when you’re stuck on a particular type of problem. Don’t just glance at the answer–take the time to walk through the entire process again to reinforce the lesson. If necessary, try similar problems using the same strategies to further cement your understanding.

Step-by-Step Solutions to Algebra Problems

One of the most effective methods for mastering mathematical challenges is to break down each problem into manageable steps. This approach helps you understand the logic behind each calculation, enabling you to tackle even the most complex exercises with confidence. By following a structured process, you can identify where you may have gone wrong and learn from your mistakes, improving both your skills and understanding of the subject.

Each solution is designed to guide you through a sequence of logical steps, starting from the initial problem and progressing toward the final solution. This method not only reinforces the core concepts but also enhances your ability to think critically and apply what you’ve learned to new problems.

For example:

1. Read the problem carefully and identify the key information.
2. Determine the best approach or formula to use for solving the problem.
3. Apply the necessary operations step by step, simplifying the expressions as you go.
4. Check your work to ensure each step follows logically from the previous one.
5. Review the final solution and confirm it makes sense in the context of the problem.

Practice is crucial to mastering this approach. By repeatedly following these steps for various problems, you’ll develop a deeper understanding of the methods and become more confident in your ability to solve similar challenges in the future.

Key Concepts in Algebra with Pizzazz

Mastering any mathematical discipline requires a solid understanding of its fundamental principles. These core ideas form the building blocks for solving more complex problems and for applying mathematical reasoning in real-life situations. The process of learning becomes easier when these concepts are presented in a clear and engaging way, helping students connect abstract ideas with practical problem-solving techniques.

Some of the essential concepts include:

• Equations and Inequalities: Understanding how to balance both sides of an equation and solve for unknowns is crucial in many mathematical tasks.
• Operations on Variables: Mastery of how to manipulate variables, including addition, subtraction, multiplication, and division, is foundational for solving various types of problems.
• Functions and Graphing: Learning how to graph equations and interpret the relationships between variables is vital in understanding more advanced topics.
• Polynomials and Factoring: Recognizing how to expand, factor, and simplify polynomials is key for solving a wide range of problems.
• Exponents and Radicals: Understanding the rules for working with powers and roots enables students to approach equations with confidence.

Building a deep understanding of these concepts allows learners to not only solve problems more efficiently but also to gain a greater appreciation for the underlying structure of mathematics. By developing a strong foundation, students can progress to more advanced topics and build the skills necessary to approach complex challenges with ease.

Understanding the Structure of the Workbook

To get the most out of any educational resource, it’s essential to understand how it is organized. A well-structured workbook not only presents problems and solutions but also ensures that each section builds upon the last. This structure allows learners to progress at a steady pace, reinforcing concepts before moving on to more advanced material. By recognizing how the workbook is laid out, students can navigate it more effectively and make the most of their study time.

How the Workbook is Organized

The workbook is divided into several key sections, each focusing on different aspects of the subject. These sections are arranged in a logical sequence, starting with foundational concepts and gradually increasing in complexity. Typically, each chapter or section begins with an explanation of new concepts, followed by a series of practice problems that allow students to apply what they have just learned.

Utilizing the Workbook for Maximum Benefit

To fully benefit from the workbook, it’s important to approach each section strategically. Start by reviewing the explanations and examples carefully before attempting the problems. Then, as you work through the exercises, refer back to the examples and guidelines to ensure you’re following the correct process. If you find yourself stuck, take a moment to analyze the steps, identify where you might have gone wrong, and try again. This cycle of learning, practicing, and reviewing will help reinforce the material and solidify your understanding.

Common Algebraic Mistakes and Fixes

Even the most experienced learners make errors while solving mathematical problems. Recognizing these common mistakes is crucial for improving accuracy and avoiding repeated errors. Understanding the root causes of mistakes and knowing how to correct them can help students refine their skills and approach problems more effectively. Below are some of the most frequent issues encountered and how to fix them.

• Sign Mistakes: Forgetting to change signs when distributing negative numbers or simplifying expressions.
• Incorrect Order of Operations: Failing to follow the proper sequence of operations, often resulting in incorrect results.
• Misinterpreting Parentheses: Not correctly applying the distributive property when parentheses are involved.
• Combining Unlike Terms: Attempting to add or subtract terms that are not similar, such as adding x and y terms together.
• Forgetting to Simplify: Leaving expressions unsimplified or not factoring completely when required.

To correct these mistakes, try the following tips:

1. Always double-check the signs, especially when multiplying or distributing terms.
2. Remember to follow the order of operations–PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction)–at all times.
3. When using parentheses, carefully distribute terms and ensure each term is handled properly.
4. Only combine like terms (terms with the same variable and exponent).
5. Take the time to simplify or factor expressions fully to make further calculations easier.

By being aware of these common errors and knowing how to address them, learners can enhance their problem-solving efficiency and accuracy, leading to better overall performance.

Tips for Mastering Algebraic Techniques

Mastering mathematical techniques requires consistent practice, patience, and a deep understanding of the methods used to solve problems. While it can seem daunting at first, breaking down each task into smaller, manageable steps can make the learning process more approachable. With the right strategies, anyone can build confidence and achieve proficiency in solving complex equations and expressions.

Effective Strategies for Improvement

To gain mastery in mathematical techniques, consider the following helpful strategies:

• Practice Regularly: The more problems you solve, the more comfortable you will become with different types of problems and solutions.
• Understand the Theory: Don’t just memorize formulas–take the time to understand why and how they work.
• Work Through Examples: Start by carefully going through solved examples to see the reasoning behind each step.
• Ask for Help: If you’re stuck on a particular concept or problem, don’t hesitate to ask teachers or peers for clarification.

Additional Tips for Success

Here are some further suggestions to help you master mathematical techniques more effectively:

1. Stay Organized: Keep your work neat and organized to avoid mistakes and make it easier to identify errors.
2. Check Your Work: After solving a problem, always double-check your work to ensure that every step is correct.
3. Use Multiple Resources: Explore different textbooks, online tutorials, and forums to gain various perspectives on solving problems.
4. Take Breaks: If you find yourself feeling frustrated or overwhelmed, take a short break to reset and approach the problem with a fresh mind.

By implementing these techniques, you can gradually improve your mathematical abilities and build the confidence needed to tackle even the most challenging problems with ease.

Improving Problem-Solving Skills in Algebra

Enhancing problem-solving abilities is essential for mastering any mathematical discipline. The key to becoming proficient at tackling problems lies in developing a strategic approach that allows you to break down complex tasks into smaller, more manageable steps. By practicing regularly and applying consistent methods, you can build your confidence and accuracy when solving mathematical challenges.

To improve your problem-solving skills, consider focusing on the following strategies:

• Understand the Problem: Before attempting to solve a problem, make sure you fully understand the question being asked. Read it carefully and identify key pieces of information.
• Break the Problem Into Smaller Parts: Divide complex problems into simpler subproblems. Solving each smaller part individually will make the overall task less overwhelming.
• Choose the Right Method: Depending on the type of problem, identify the most appropriate mathematical method or technique to use. This could involve simplification, factoring, or applying formulas.
• Work Step-by-Step: Avoid jumping ahead to the solution. Taking it one step at a time allows you to catch potential errors and stay organized throughout the process.
• Check Your Work: After solving a problem, always go back and verify your solution. This helps to catch any mistakes and ensures accuracy.

With regular practice and a focused approach, anyone can strengthen their problem-solving skills. By developing a strong foundation in these techniques, you will be able to tackle more challenging problems and build the necessary skills to succeed in mathematical reasoning.

The Role of Practice in Algebra Mastery

Mastering any mathematical discipline relies heavily on consistent practice. The more time spent solving problems, the more familiar and comfortable you become with the techniques and strategies required to find solutions. Practice not only reinforces knowledge but also helps develop the problem-solving skills necessary for success. It’s through repeated exposure to different problem types and methods that proficiency is achieved.

Regular practice offers several key benefits:

• Reinforcement of Concepts: By working through problems repeatedly, the core principles and methods become ingrained, making them easier to apply in various situations.
• Increased Confidence: The more problems you solve, the more confident you become in your ability to approach even unfamiliar challenges.
• Improved Speed: As you practice, you’ll find that you can solve problems more quickly, allowing you to complete tasks more efficiently.
• Better Problem-Solving Skills: Regular exposure to new types of problems helps you develop flexibility in your thinking, allowing you to apply different strategies to a variety of challenges.

Ultimately, mastery comes from a combination of understanding the underlying concepts and applying them regularly. The more you practice, the more natural and intuitive solving problems will become, paving the way for continued success in mathematical reasoning.

Analyzing the Answer Key’s Purpose

The main function of a solution guide is to provide clarity and guidance after solving problems. It acts as a valuable tool for verifying the accuracy of one’s work and for learning the proper steps in arriving at a correct result. By reviewing the solutions provided, students can identify their mistakes, understand the correct approach, and improve their overall problem-solving skills.

Understanding the role of a solution guide involves recognizing its educational benefits:

• Verification: It helps confirm whether the solution to a problem is accurate, offering students a clear sense of whether their approach was correct.
• Learning from Mistakes: By comparing their work to the provided solutions, learners can pinpoint errors and understand how to avoid them in the future.
• Step-by-Step Guidance: The solution guide often breaks down each problem into smaller steps, allowing students to follow the reasoning process behind the solution.
• Building Confidence: Having access to correct solutions boosts confidence, reassuring students that they are on the right track as they learn new concepts.

While solution guides are helpful, it is important to use them as a learning tool rather than simply relying on them to find answers. Active engagement with the guide promotes deeper understanding and better retention of mathematical techniques.

How to Tackle Challenging Algebra Problems

Facing complex problems can be intimidating, but breaking them down into smaller, more manageable steps can make them less overwhelming. To tackle difficult tasks effectively, it is essential to approach them with a structured mindset, utilizing strategies that help in understanding and solving each part of the problem. The key is not to rush, but to stay organized and persistent.

Here are some strategies to consider when encountering tough mathematical challenges:

• Analyze the Problem Thoroughly: Read through the problem carefully to identify the key information and what is being asked. Understanding the question is the first step toward finding a solution.
• Start with What You Know: Begin by solving the parts of the problem you are already familiar with. This can give you a foundation to build upon for the more difficult aspects.
• Break the Problem into Smaller Steps: Divide the problem into smaller, logical segments. Solving each piece individually can make the entire problem seem more manageable.
• Look for Patterns: Many challenging problems can be simplified by recognizing patterns or relationships between the variables. Identifying these connections can provide clues to finding a solution.
• Use Visual Aids: Sometimes drawing diagrams, charts, or tables can help visualize the problem, making it easier to understand and solve.
• Take Breaks: If you’re stuck, stepping away for a short period can help refresh your mind and lead to new insights when you return to the problem.

By applying these techniques and practicing regularly, you will become more comfortable with complex problems and develop a stronger ability to solve them with confidence.

Practical Applications of Algebraic Concepts

Mathematical techniques are not just theoretical; they have real-world applications that are essential for solving everyday problems. From budgeting personal finances to designing technology, the principles learned in mathematics are utilized across various fields. Understanding how these concepts are applied in practical scenarios not only makes learning more interesting but also highlights the importance of mastering them.

Here are a few examples where mathematical ideas are used in everyday life:

Application	Description
Financial Planning	Using equations to calculate expenses, savings, loans, and interest rates helps in budgeting and managing money effectively.
Construction and Engineering	Mathematics is essential for determining measurements, angles, and structures in construction projects, ensuring safety and accuracy.
Data Analysis	Statistical and algebraic techniques are used to interpret data, find trends, and make informed decisions in business and research.
Technology Development	Software and hardware engineers use mathematical formulas to design systems, optimize processes, and troubleshoot problems.
Environmental Science	Mathematical models help in predicting environmental changes, such as climate change, and in managing resources effectively.

These examples demonstrate how mathematical knowledge plays a key role in both practical and professional settings. By applying mathematical concepts, individuals can solve problems more efficiently and make more informed decisions in their daily lives and careers.

How Algebra with Pizzazz Supports Learning

Learning mathematics can sometimes be a daunting task, especially when it involves complex problem-solving and abstract concepts. However, an engaging and structured approach can transform the learning experience, making it both enjoyable and effective. The structured exercises, varied problem types, and stimulating challenges found in such resources support not only the acquisition of mathematical knowledge but also the development of critical thinking skills. By presenting problems in a more interactive and dynamic way, students can gain a deeper understanding and appreciation of mathematical concepts.

Engaging and Interactive Approach

One of the key features of this method is its ability to make learning engaging. It incorporates a variety of activities designed to capture the student’s attention while reinforcing key mathematical concepts. Here’s how it works:

• Variety of Problem Types: The material includes a broad range of exercises that cater to different learning styles, from simple equations to more complex word problems.
• Clear Instructions: Each problem comes with step-by-step guidance, ensuring that students can follow along and understand the reasoning behind each solution.
• Interactive Elements: Visuals and interactive challenges encourage active participation and help students apply what they’ve learned in a hands-on way.

Building Confidence in Problem-Solving

The step-by-step nature of these exercises not only helps in mastering mathematical concepts but also builds confidence in problem-solving. Students are gradually introduced to more challenging problems, which promotes a sense of achievement as they progress. The emphasis on incremental learning ensures that foundational skills are developed before moving on to more advanced topics.

• Confidence Boost: By tackling increasingly complex problems, students develop a sense of accomplishment and confidence in their problem-solving abilities.
• Encouraging Critical Thinking: The exercises stimulate critical thinking, teaching students how to approach and solve problems systematically.
• Peer Collaboration: Collaborative exercises and discussions allow students to work together, reinforcing their understanding and learning from one another.

Overall, this approach creates an environment where students not only understand mathematical concepts but also enjoy the process of learning. By making complex ideas more accessible and interesting, it fosters a positive attitude toward mathematics and equips learners with the skills they need for success.

Creating a Study Plan for Algebra Success

Achieving success in mathematics requires a well-structured approach and consistent effort. One of the most effective ways to ensure steady progress is by creating a personalized study plan that targets specific learning goals. By breaking down the subject into manageable sections and scheduling dedicated study sessions, students can stay on track and build their skills gradually. A clear study plan not only helps students focus on their strengths but also allows them to address their weaknesses more effectively.

When designing a study plan, it’s essential to consider factors such as time availability, difficulty level of the material, and personal learning preferences. A well-organized plan allows students to stay motivated and make consistent improvements over time. The following strategies can help you create an effective study plan:

• Set Specific Goals: Start by identifying your learning objectives. Are you looking to improve on certain topics or prepare for an exam? Setting measurable goals helps track progress and stay focused.
• Break Down the Material: Divide the content into smaller, manageable sections. This approach makes complex topics easier to digest and reduces the risk of feeling overwhelmed.
• Incorporate Different Learning Methods: Combine various study techniques such as reading, solving practice problems, watching instructional videos, and discussing concepts with peers. Different methods enhance understanding and retention.
• Schedule Regular Study Sessions: Consistency is key. Set aside time each day or week for focused study sessions. Make it a habit, and try to stick to your schedule as much as possible.
• Monitor Your Progress: Periodically review your progress to ensure you’re meeting your goals. If you find certain areas challenging, adjust your plan to allow more time for those topics.

By following these strategies, students can maximize their study time, reduce stress, and gain a deeper understanding of the material. A thoughtful and flexible study plan fosters a proactive approach to learning and ensures long-term academic success.

Why Algebra with Pizzazz is Popular

Many students and educators find that certain learning materials offer an engaging and effective way to approach mathematical concepts. One such resource has gained widespread attention for its unique ability to combine instruction with an element of fun. By presenting topics in an interactive and visually appealing manner, it captures students’ interest and makes learning more enjoyable. This approach not only enhances comprehension but also fosters a positive attitude toward mathematics, something that is often perceived as challenging or intimidating.

The popularity of this resource can be attributed to several key factors:

• Engaging Activities: The material includes a variety of exercises designed to encourage active participation. These activities are not just about solving problems–they also prompt critical thinking and creative problem-solving, which keeps students engaged and motivated.
• Interactive Learning: Instead of relying solely on traditional methods, this resource incorporates interactive elements that cater to different learning styles. Visual learners, in particular, benefit from the use of graphics and diagrams that make abstract concepts more tangible.
• Relatable Examples: The problems are often framed in real-world contexts, making them more relatable to students. This helps learners connect the material to their own lives, enhancing both understanding and retention.
• Fun Challenges: The format is designed to keep students entertained while still challenging their skills. This balance between fun and difficulty helps build confidence and encourages learners to tackle even the most complex problems.

Ultimately, the combination of an engaging format, interactive features, and real-world applications has made this resource a favorite among students and educators alike. It creates an enjoyable and effective learning experience that goes beyond the conventional approach, making mathematical concepts more accessible and enjoyable.

Factor	Benefit
Engaging Activities	Encourages active participation and critical thinking
Interactive Learning	Cater to various learning styles, especially visual learners
Relatable Examples	Helps students connect lessons to real-life situations
Fun Challenges	Balancing fun and difficulty promotes confidence and engagement

Final Thoughts on the Answer Key’s Value

When it comes to mastering mathematical concepts, the role of a solution guide cannot be overstated. It serves as an essential tool for reinforcing learning, providing clarity on problem-solving methods, and ensuring students grasp the key principles. While it may seem like a simple reference, its value extends far beyond just showing correct results. It offers insight into the approach used to arrive at the solution, which can enhance a learner’s understanding of the process and improve their overall skills.

Here are a few reasons why this resource is highly valued by both learners and instructors:

• Clarification of Methodology: A solution guide helps learners see the step-by-step process that leads to the final result. Understanding the reasoning behind each step can clarify any confusion and improve problem-solving abilities.
• Independent Learning: It encourages students to try solving problems on their own first. When they encounter difficulties, they can consult the guide to check their approach and understand where they went wrong, promoting a more independent learning experience.
• Instant Feedback: Immediate access to solutions helps students correct mistakes quickly, preventing misconceptions from taking root. This timely feedback is crucial for maintaining progress and motivation.
• Confidence Boost: By seeing correct solutions, students gain confidence in their own abilities, reinforcing the idea that they can tackle complex problems successfully.

In conclusion, while a solution guide may not directly teach new material, it is an indispensable tool for solidifying understanding, fostering self-confidence, and encouraging continuous improvement. Its value lies not just in providing answers but in offering a deeper understanding of the problem-solving process, which is essential for mastering any subject.