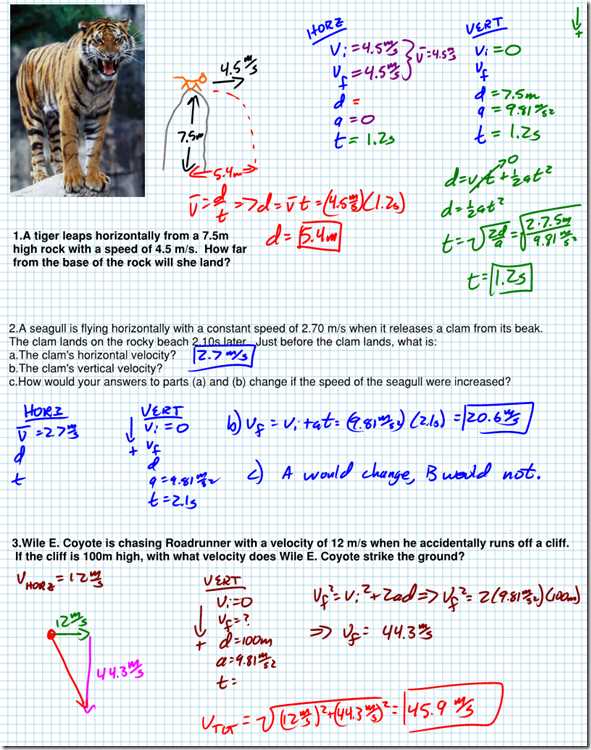
Section 12.1 Forces Worksheet Answers Explained

section 12.1 forces worksheet answers

In this part of the study, we delve into the core principles that govern the interactions between objects. By analyzing the relationships between various physical quantities, we can uncover how different forces influence motion and stability. The goal is to develop a deeper understanding of how the world around us works from a scientific perspective.

The exercises are designed to enhance your problem-solving skills by applying theoretical concepts to real-world scenarios. You will be guided through step-by-step solutions, helping you grasp how different factors, such as mass, acceleration, and external influences, interact within physical systems. These practical applications are essential for building a solid foundation in basic mechanics.

Mastering these principles not only strengthens your understanding of physics but also provides the tools to tackle more complex topics in the field. With a clear focus on fundamental equations and methods, this section aims to solidify your knowledge and prepare you for advanced studies in science and engineering.

Forces Concepts and Problem Solving Overview

This section provides a comprehensive review of key physical principles related to how objects interact with each other. By examining how various forces affect motion, we aim to develop the ability to solve related problems. The goal is to strengthen the understanding of fundamental concepts and apply them to practical scenarios. Through this, we build a strong foundation for tackling more advanced topics in mechanics and physics.

Problem-Solving Approach

The problems presented in this section focus on applying theoretical knowledge to real-world situations. They are designed to challenge your understanding and improve your ability to analyze physical systems. The exercises involve breaking down complex situations into simpler steps and using relevant formulas to find the unknown variables.

Common Techniques for Calculation

Several key methods are used to solve the problems. These include identifying the right relationships between different quantities, converting units as needed, and using free-body diagrams to visualize the forces acting on objects. Understanding these techniques ensures that the solutions are accurate and the concepts are well understood.

Concept	Description	Example
Newton’s First Law	Object will remain at rest or in uniform motion unless acted upon by an external force.	Car continues to move until brakes are applied.
Newton’s Second Law	The force acting on an object is equal to the mass of the object multiplied by its acceleration.	F = ma (force equals mass times acceleration).
Newton’s Third Law	For every action, there is an equal and opposite reaction.	Push against a wall, the wall pushes back with equal force.

Understanding the Basics of Forces

section 12.1 forces worksheet answers

In this section, we explore the fundamental principles that explain how objects interact with each other. At the core of this discussion is the idea that physical interactions cause changes in motion, whether that be movement, speed, or direction. These interactions, influenced by various external factors, are central to understanding how the world works from a scientific perspective.

The Role of Interaction in Motion

Every object that moves or stays still is subject to some form of external influence. These influences, or interactions, can be push or pull forces that alter the state of motion. Whether an object is at rest or moving at a constant speed, the forces acting on it determine how it will behave when subjected to other influences. By recognizing and calculating these interactions, we can predict how objects will respond in different situations.

Types of Influences and Their Effects

Different types of forces have varying effects on objects. For example, contact forces occur when objects physically touch, while action-at-a-distance forces, like gravity, act over a distance without direct contact. Understanding these forces and how they interact with an object’s mass and velocity is essential for analyzing motion and predicting future behavior.

Key Concepts in Physics Problem Solving

This section focuses on essential principles that help analyze and solve problems related to object motion and external influences. Understanding the underlying concepts is crucial for solving complex problems and applying theoretical knowledge to practical situations. The main concepts explored include the relationships between mass, acceleration, and applied influences.

Fundamental Ideas to Grasp

Before diving into problem-solving, it’s important to understand the following key concepts:

Inertia: The resistance of an object to changes in its state of motion.
Acceleration: The rate at which an object’s velocity changes over time.
Mass: A measure of the amount of matter in an object, which affects how much it resists acceleration.
External influences: Factors like gravity, friction, and applied pushes or pulls that affect an object’s movement.

Applications of These Concepts

Once these core concepts are understood, they can be applied in real-world scenarios to solve various problems. Here are some common steps:

Identify the objects involved and the forces acting on them.
Use appropriate formulas to calculate acceleration, force, or mass based on known values.
Check for equilibrium, where all forces are balanced, or calculate the net force when forces are unbalanced.
Apply Newton’s laws of motion to predict the outcome of various scenarios.

How to Approach Forces Problems

When tackling problems related to the movement and interaction of objects, it’s crucial to follow a systematic approach. This method helps break down complex scenarios into manageable steps, allowing you to apply the right principles and techniques for accurate solutions. By clearly identifying the known and unknown quantities, and understanding how they relate, you can solve even the most challenging problems with confidence.

The first step is to carefully analyze the situation. Identify the objects involved, the forces acting upon them, and their direction. A visual representation, such as a free-body diagram, can often make this process easier. Once you have a clear picture of the situation, the next step is to apply relevant formulas and concepts to calculate the unknown values, such as acceleration, mass, or applied force.

After solving the equations, it’s important to double-check your results. Ensure that the units are consistent and that the solution makes sense in the context of the problem. By following these steps, you will be able to approach any related problem methodically and efficiently.

Common Mistakes in Force Calculations

When solving problems involving physical interactions between objects, certain errors are frequently made. These mistakes can lead to incorrect results and hinder understanding of key concepts. Recognizing and avoiding these common pitfalls is essential for accurate problem solving and for developing a solid grasp of the principles involved.

Incorrect Application of Formulas

One of the most common mistakes is using the wrong formula or misapplying the correct one. For example, confusing the equations for different types of motion, such as linear versus rotational, can lead to significant errors. It’s crucial to identify the correct relationship between variables before solving for unknowns. Additionally, be sure to substitute the correct values into the formulas and check for unit consistency.

Ignoring Direction and Sign Conventions

Another frequent mistake is neglecting the direction of forces or forgetting to apply the correct sign conventions. Many problems involve forces acting in opposite directions, and failing to account for this can result in incorrect solutions. Always remember that forces are vector quantities, meaning they have both magnitude and direction. Properly assigning positive and negative signs based on the chosen coordinate system is key to accurate calculations.

Unit Conversion in Force Problems

In many physical problems, the units of measurement for different quantities may not match, requiring conversion before calculations can be made. Converting between units is an essential skill to ensure the accuracy of results. Without proper unit conversion, even correctly applied formulas can lead to incorrect solutions. This process involves converting mass, distance, time, and other variables into consistent units before proceeding with calculations.

Understanding the Importance of Consistency

Unit consistency is critical in physics. When the units of different variables don’t align, the results can be misleading. For instance, if you have mass in kilograms but distance in centimeters, the units must be converted so they match the standard unit system, usually meters and kilograms. Without this alignment, applying formulas such as Newton’s Second Law becomes impossible. Properly converting units ensures that all quantities are expressed in terms that can be combined logically.

Common Unit Conversions in Physics

Some of the most common unit conversions in physics problems include:

Mass: Converting grams to kilograms (1000 grams = 1 kilogram).
Distance: Converting centimeters to meters (100 centimeters = 1 meter).
Time: Converting seconds to minutes (60 seconds = 1 minute).
Force: Converting newtons to dynes (1 newton = 10^5 dynes).

By mastering these conversions, you can ensure that all variables are in the correct units, leading to accurate and reliable results in your calculations.

Step-by-Step Problem Solving Guide

Solving physical problems involving interactions between objects can often seem daunting. However, by breaking the process into clear, manageable steps, you can tackle any challenge with confidence. The key is to follow a structured approach, ensuring that all aspects of the problem are addressed before jumping to conclusions. By understanding each phase, from identifying knowns to applying formulas, you’ll be able to arrive at accurate solutions every time.

Step 1: Analyze the Situation

The first step in solving any problem is to carefully read and understand the scenario. Identify the objects involved, the forces acting upon them, and any given values. It can be helpful to create a diagram or sketch to visualize the situation. This step is critical because having a clear understanding of the problem sets the stage for accurate calculations.

Step 2: Organize Known Information

Once the situation is understood, list out all known variables, such as mass, velocity, and applied forces. At this point, it’s essential to identify what you need to find. If needed, convert units to ensure consistency across all variables. Organizing the information in this way simplifies the next steps in the process.

Step 3: Apply the Appropriate Formula

With the known information laid out, select the appropriate formula to relate the variables. Be sure to consider any physical laws that apply, such as Newton’s laws of motion, and make sure you’re using the correct equations for the type of motion or interaction involved. Substituting the known values into the formula is a crucial step to finding the solution.

Step 4: Solve and Interpret Results

After applying the formula, solve for the unknown variable. Double-check your math and units to ensure accuracy. Once you have a solution, interpret the result in the context of the problem. Does the solution make sense given the physical scenario? If not, revisit earlier steps to identify potential errors.

Step	Action	Key Consideration
Step 1	Analyze the situation	Identify objects, forces, and given values
Step 2	Organize known information	Ensure unit consistency
Step 3	Apply the formula	Select the right equation for the problem
Step 4	Solve and interpret	Double-check calculations and interpret results

Following these steps will allow you to systematically approach and solve any related problem, ensuring clarity and accuracy in your work.

Newton’s Laws in Forces Problems

Understanding how objects move and interact is central to solving many physical problems. One of the most fundamental ways to approach these problems is through Newton’s laws of motion, which provide a clear framework for analyzing the behavior of objects under various influences. These laws describe the relationship between an object’s motion and the forces acting upon it, offering essential insights into how and why objects accelerate or remain at rest.

First Law: Inertia

The first law, also known as the law of inertia, states that an object at rest will remain at rest, and an object in motion will continue moving at a constant velocity, unless acted upon by an external force. This law explains why it is necessary to apply a force to change the state of motion of an object.

Example: A book resting on a table will stay still until someone applies a force to move it.
Real-World Application: Seat belts in cars prevent sudden movement when the car abruptly stops.

Second Law: Acceleration and Force

The second law explains how the velocity of an object changes when a force is applied. It states that the force applied to an object is equal to its mass multiplied by its acceleration. This relationship is expressed by the formula F = ma, where F is the force, m is the mass, and a is the acceleration.

First, determine the mass of the object.
Next, calculate the acceleration (if not already given).
Finally, use the formula to find the force required to produce the desired acceleration.

Example: A car with a mass of 1000 kg accelerates at 2 m/s². The force required is 2000 N (1000 kg * 2 m/s²).

Third Law: Action and Reaction

The third law states that for every action, there is an equal and opposite reaction. This means that if one object applies a force on another, the second object applies a force of the same magnitude but in the opposite direction.

Example: When you push against a wall, the wall pushes back with an equal force in the opposite direction.
Real-World Application: Rocket propulsion relies on the expulsion of gases in one direction, which propels the rocket forward in the opposite direction.

By applying these three laws, you can break down complex physical problems and analyze the interactions between objects under various conditions.

Examples of Force Worksheet Questions

To better understand how physical principles apply to real-world situations, it’s essential to work through practical examples. These types of questions allow you to apply theoretical knowledge to different scenarios and practice using formulas and concepts in problem-solving. Below are several examples that demonstrate how to approach force-related challenges in physics.

Example 1: Calculating Acceleration

In this example, we are tasked with determining the acceleration of an object when a known force is applied. Understanding how force relates to mass and acceleration is key to solving such problems.

Problem: A car with a mass of 1500 kg experiences a force of 4500 N. What is the acceleration of the car?
Solution: Use the formula F = ma, where F is the applied force, m is the mass, and a is the acceleration. Rearranging the equation to solve for a gives a = F/m. Substitute the values: a = 4500 N / 1500 kg = 3 m/s².

Example 2: Identifying the Net Force

This question requires finding the net force acting on an object when multiple forces are applied in different directions. It tests your ability to resolve forces and determine their combined effect.

Problem: Two forces, 10 N to the right and 5 N to the left, act on a box. What is the net force acting on the box?
Solution: The forces are in opposite directions, so subtract the smaller force from the larger force: Net force = 10 N – 5 N = 5 N to the right.

Example 3: Gravitational Force Calculation

Another common problem involves calculating the gravitational pull on an object. This helps you understand the relationship between mass, gravity, and the force exerted on an object near the Earth’s surface.

Problem: What is the weight of an object with a mass of 60 kg on Earth? (Use g = 9.8 m/s²)
Solution: Weight is calculated using the formula Weight = mass × gravity. Substituting the known values: Weight = 60 kg × 9.8 m/s² = 588 N.

Example 4: Balanced and Unbalanced Forces

Sometimes, it’s important to determine whether forces acting on an object are balanced or unbalanced, as this affects the motion of the object.

Problem: A box is being pushed with a force of 20 N to the right and a frictional force of 20 N to the left. What is the net force on the box?
Solution: Since the forces are equal in magnitude and opposite in direction, they cancel each other out. Therefore, the net force is 0 N, meaning the box will remain stationary or move with constant velocity.

These examples illustrate a variety of typical questions and demonstrate how to apply fundamental principles of physics to solve them. Working through such problems is an excellent way to solidify your understanding and develop problem-solving skills in the study of motion and interactions.

Tips for Solving Force Equations

When solving problems that involve physical interactions, it’s important to approach the equations systematically. Whether you’re calculating acceleration, determining net forces, or analyzing motion, understanding the relationships between variables is essential. Below are some helpful tips to guide you through solving these types of equations efficiently.

1. Identify Known and Unknown Variables

Before diving into calculations, always identify the values you already know and the quantities you need to find. This will allow you to choose the correct formula and make the problem more manageable.

Tip: Highlight or write down the given data (such as mass, force, acceleration) to avoid confusion later.
Example: If you’re asked to find the acceleration, make sure you know both the mass of the object and the applied force.

2. Use the Right Formula

Using the appropriate formula is crucial for solving the problem correctly. Some of the most common equations in these types of problems include:

Newton’s Second Law: F = ma (Force = mass × acceleration).
Weight Calculation: Weight = mg (Weight = mass × gravitational acceleration).
Frictional Force: F_friction = μN (Frictional force = coefficient of friction × normal force).

Make sure to select the equation that best fits the context of the problem you are solving.

3. Pay Attention to Units

One of the most common mistakes is forgetting to convert units. Always ensure that your units are consistent when applying formulas.

Tip: Convert all units to SI units before solving, such as mass in kilograms, distance in meters, and force in newtons.
Example: If you’re given mass in grams, convert it to kilograms (1000 grams = 1 kilogram).

4. Break Down Complex Problems

If a problem involves multiple forces or directions, break it down into smaller parts. Consider the forces acting in different directions and how they interact. Often, problems can be simplified by separating horizontal and vertical forces.

Tip: Draw a free-body diagram to visualize the forces acting on the object and their directions.
Example: If a box is being pushed on a ramp, separate the force components acting parallel and perpendicular to the surface of the ramp.

5. Double-Check Your Results

After solving, take a moment to review your results. Ensure that the answer makes sense in the context of the problem. Check that units are consistent and that your answer is reasonable based on the given data.

Tip: Look for any errors such as incorrect unit conversions or misapplied formulas.
Example: If the calculated acceleration seems unusually large or small, recheck your calculations for possible errors.

By following these tips, you can approach each problem with greater confidence and accuracy, ensuring a better understanding of physical interactions and more successful problem-solving outcomes.

Force Diagrams and Their Importance

In physics, visualizing the interactions between objects is essential for solving problems accurately. One of the most effective ways to represent these interactions is through diagrams that illustrate the various forces acting on an object. These diagrams help to simplify complex problems by providing a clear and organized view of the situation, making it easier to apply the correct equations and calculate the desired quantities.

Force diagrams, also known as free-body diagrams, are graphical representations that show the direction and magnitude of forces acting on an object. Each force is represented by an arrow, with the length indicating the strength of the force and the direction pointing in the direction of the force. By focusing on the object of interest, these diagrams exclude irrelevant forces, helping to isolate the key interactions that must be analyzed.

The importance of force diagrams lies in their ability to break down a problem into simpler, more manageable parts. They make it easier to identify which forces are involved, how they interact, and how they affect the object’s motion or equilibrium. These diagrams are especially useful when dealing with multiple forces, as they allow for a clear comparison of the forces acting in different directions.

Additionally, force diagrams play a crucial role in determining the net force acting on an object, which is essential for calculating acceleration or solving problems related to motion. By carefully analyzing the diagram, it becomes possible to calculate the resultant force by considering both the magnitude and direction of all acting forces.

Ultimately, creating and analyzing force diagrams provides a clear path to solving problems involving motion and interactions between objects. They not only help to visualize complex concepts but also offer a structured approach to problem-solving, making them an indispensable tool in the study of physics.

Understanding Force Units and Measurements

When analyzing physical interactions, it’s essential to quantify the strength of these interactions accurately. Force, being a fundamental concept in physics, is measured in units that allow scientists and engineers to describe the magnitude of these interactions. Understanding the units used to measure force is crucial for solving problems and ensuring that calculations are consistent with the system in question.

The standard unit for measuring force in the International System of Units (SI) is the newton (N). One newton is defined as the amount of force required to accelerate a 1-kilogram mass by 1 meter per second squared. This relationship is derived from Newton’s second law of motion, which states that force equals mass multiplied by acceleration (F = ma). Therefore, a force of 1 newton results in a 1-kilogram object accelerating at 1 m/s².

In addition to newtons, there are other units that may be used in different contexts. For example, in some non-SI systems, force might be measured in pounds (lb) in the United States or dynes in the centimeter-gram-second (CGS) system. While these units are still used in specific regions or fields, the newton remains the globally accepted unit in most scientific and engineering applications.

Understanding force measurements also involves recognizing the importance of converting between different units. For instance, to convert from pounds to newtons, you can use the following relationship: 1 pound is approximately equal to 4.448 newtons. Such conversions are vital when working with problems that involve multiple measurement systems.

In practice, it’s not just about knowing the unit of force; it’s about accurately measuring and interpreting the force in real-world scenarios. Using proper instruments, such as spring scales or load cells, helps determine the force exerted in various contexts, whether it’s lifting an object or analyzing the forces between particles in a material.

By mastering force units and measurements, you’ll be better equipped to tackle problems that require precision and consistency in calculating the effects of various interactions. These fundamental skills form the foundation of many physical theories and engineering applications.

Solving for Unknown Forces

In many physical scenarios, you may encounter situations where certain values, such as forces acting on an object, are unknown and need to be determined. The process of solving for these unknowns involves understanding the relationships between various factors, such as mass, acceleration, and applied forces. Using these relationships, you can rearrange and apply fundamental principles to isolate and solve for the unknown values.

To begin solving for an unknown, it’s crucial to first identify the known variables in the problem. These could include the object’s mass, acceleration, or other forces already acting on the system. Once the known variables are identified, the next step is to apply Newton’s laws, which provide the framework for understanding the dynamics of the system. By using these laws, you can manipulate the equations to solve for the missing force.

One common approach is to use Newton’s second law of motion, which states that force is the product of an object’s mass and its acceleration (F = ma). In situations where the acceleration and mass are known, this equation can be used to directly calculate the unknown force. However, when multiple forces act on an object simultaneously, it may be necessary to resolve the forces into components and solve for the net force.

For example, imagine a scenario where you need to determine the force required to accelerate an object. If the mass and acceleration are given, you can use the following steps:

Given Variables	Values
Mass (m)	10 kg
Acceleration (a)	3 m/s²
Unknown force (F)	F = m × a = 10 kg × 3 m/s² = 30 N

In this case, the force required to accelerate the object can be found by multiplying the mass by the acceleration. The result is 30 newtons. When more complex scenarios are involved, such as forces acting at angles or opposing forces like friction, the method remains the same, but you must account for these additional factors. For example, forces may need to be broken into horizontal and vertical components and combined using vector addition.

By following these systematic steps, you can confidently solve for unknown forces in various physical situations, whether they involve simple calculations or more complex multi-force systems.

Interpreting Forces in Real-Life Scenarios

In everyday life, the principles of motion and interaction play a crucial role in understanding how objects move and interact with their surroundings. Forces are constantly at work, influencing the behavior of everything from vehicles on the road to objects falling under gravity. Understanding how these invisible influences affect physical systems helps us interpret real-world phenomena, from the simple act of lifting a box to the complex dynamics of engineering structures.

When interpreting physical interactions, it’s important to consider both the visible and hidden forces at play. For instance, when you push an object, several forces are involved: the applied force, friction between the object and the surface, and sometimes gravitational forces, especially in inclined situations. Each of these forces must be understood in relation to one another to predict the behavior of the object accurately.

Real-Life Examples of Force Interactions

Let’s take a closer look at some common situations where force interpretation is key:

Driving a Car: As you accelerate, the engine generates force that propels the car forward. At the same time, friction with the road surface resists this motion, and air resistance plays a role at higher speeds. All these forces must be balanced for smooth motion.
Climbing a Ladder: When you climb a ladder, you exert an upward force on each step. The ladder itself also exerts a downward force due to gravity, and the friction between your shoes and the ladder prevents slipping.
Object Falling to the Ground: An object that is dropped experiences gravitational force pulling it downward, but air resistance acts in the opposite direction, slowing it down until it reaches its terminal velocity.

Factors Influencing Force Interpretation

Several factors influence how we interpret and analyze forces in these scenarios:

Surface Properties: The roughness or smoothness of surfaces can significantly affect the amount of friction between objects. A smooth surface like ice will produce less friction than a rough surface like concrete.
Mass of Objects: The greater the mass of an object, the more force is required to move it. This is especially noticeable when trying to accelerate heavier objects.
Angle of Interaction: When forces act at angles, their components need to be resolved into horizontal and vertical directions. This is particularly important in scenarios involving inclined planes or sloped surfaces.

By understanding how forces manifest in real-world contexts, we can make more accurate predictions and solve problems related to motion and interaction, whether in physics, engineering, or everyday life.

How to Check Your Answers

When solving problems related to motion and interaction, it’s crucial to verify that your results are accurate. Checking your work not only helps ensure that your calculations are correct, but it also allows you to identify any mistakes in your reasoning or approach. The following steps outline an effective method for reviewing your solutions and confirming that they align with the principles of physics.

Start by revisiting the problem statement to ensure you have understood the question correctly. This step is essential because misinterpreting the problem can lead to an entirely incorrect approach. Once you’re confident that you understand what is being asked, compare your results to the logical expectations based on the given data and conditions.

Steps for Verifying Your Solution

Recheck Your Calculations: Go through your math step by step to ensure no arithmetic errors were made. It’s easy to misplace decimal points or skip signs when dealing with multiple steps.
Review Your Assumptions: Have you made any assumptions about the system that might not hold true? For example, assuming friction is negligible when it might not be, or neglecting air resistance when it’s significant.
Use Units Consistently: Ensure all units are consistent and correctly converted. Unit discrepancies can lead to incorrect results even when the mathematical steps are otherwise sound.
Check Against Known Relationships: If your results seem unusually high or low, compare them with known formulas or expected values for similar situations. For instance, check if the calculated value for velocity makes sense given the distance and time.

Cross-Verification Methods

Estimate the Result: For simpler problems, you might be able to estimate an approximate solution and compare it to your calculated result. If your answer is far from the estimate, review your steps for errors.
Double-Check with Another Method: If possible, use a different approach or equation to solve the problem. If both methods give the same result, it’s more likely to be correct.
Use Software or Calculators: For more complex calculations, using tools like calculators or physics simulation software can help verify your results and catch errors you may have overlooked.

By systematically checking your solution, you can catch errors early and build confidence in your problem-solving abilities. Verification is an essential skill that enhances both your understanding of the material and your accuracy in applying it to real-world scenarios.

Using Online Resources for Assistance

In today’s digital age, online resources provide a wealth of information that can assist in solving complex problems. Whether you’re working on a physics problem or need to clarify a concept, the internet offers various tools and platforms to enhance your learning experience. By leveraging these resources, you can gain deeper insights and reinforce your understanding of key principles.

These resources come in many forms, from instructional videos and online calculators to interactive forums and problem-solving websites. The key is knowing where to look and how to use these tools effectively to supplement your studies.

Types of Online Resources

Educational Websites: Websites like Khan Academy, Coursera, and edX provide free or paid courses on various subjects, including physics. These platforms often offer in-depth explanations, examples, and exercises to help reinforce learning.
Online Forums and Communities: Websites like Stack Exchange, Reddit, or specialized subject forums offer a space where you can ask questions and engage with experts or peers who can provide solutions, clarifications, or advice on specific problems.
Interactive Simulations: Platforms like PhET Interactive Simulations offer free simulations that let you visualize concepts and experiment with variables. This is especially useful for visualizing how different factors affect physical systems.
Problem-Solving Tools: Websites like Wolfram Alpha can help you calculate solutions and check your work by simply entering the problem. These tools can also offer step-by-step explanations of how to arrive at the correct solution.

How to Use Online Resources Effectively

Cross-Check Your Work: Use online tools to double-check your calculations and verify your approach. Tools like Wolfram Alpha can provide an alternative method for solving problems and ensure your solution is accurate.
Watch Tutorials: When a specific concept is difficult to grasp, look for video tutorials that explain it in a step-by-step manner. Visual aids can make abstract concepts much easier to understand.
Engage in Discussions: If you’re stuck, don’t hesitate to ask for help in online communities. Engaging in discussions often leads to new insights and clarifies confusing aspects of the material.
Practice with Online Exercises: Many websites offer practice problems with varying levels of difficulty. Repeated practice not only solidifies concepts but also enhances problem-solving skills.

Resource Type	Examples	Best Use
Educational Websites	Khan Academy, Coursera	In-depth courses, structured learning
Forums and Communities	Stack Exchange, Reddit	Asking questions, peer support
Interactive Simulations	PhET, GeoGebra	Visualizing concepts, experimenting with variables
Problem-Solving Tools	Wolfram Alpha	Checking solutions, solving complex equations

Using online resources not only helps clarify doubts but also enriches the learning process. By integrating these tools into your study routine, you can develop a more comprehensive understanding of challenging concepts and improve your problem-solving abilities.

Practical Applications of Force Equations

Understanding the relationship between different variables in physical systems is essential for solving real-world problems. Equations that describe motion and interaction are used in a wide array of practical scenarios, from engineering to everyday activities. By applying these principles, engineers, scientists, and even technicians can predict and control the behavior of objects under various conditions.

In many situations, these mathematical relationships help us design better machines, optimize processes, and ensure safety. Whether calculating the stress on a bridge, determining the proper weight for lifting equipment, or designing efficient vehicles, force-related calculations play a central role in daily life and industrial applications.

Engineering and Construction

In engineering, force equations are critical for designing structures that are both safe and functional. These calculations help ensure that buildings, bridges, and other infrastructures can withstand the loads they will experience throughout their lifespan. For example:

Structural Integrity: Engineers calculate the forces acting on beams, columns, and other supports to ensure they don’t exceed their weight-bearing capacity.
Bridge Design: The weight of vehicles, wind, and seismic activity are all factors that must be considered when determining the forces acting on a bridge.
Machinery: Heavy machinery relies on these calculations to prevent mechanical failure and ensure longevity by accurately distributing weight and forces during operation.

Everyday Technology and Transport

In more familiar settings, force equations are also used to optimize the design and functionality of everyday products, from vehicles to appliances:

Automobile Safety: In crash testing, engineers use force equations to understand how vehicles respond to impact and to design features like airbags and crumple zones to protect passengers.
Aerospace: The design of airplanes and spacecraft involves calculating aerodynamic forces to ensure efficient flight and stability under different conditions.
Sports Equipment: Understanding how forces interact with sports equipment, such as tennis rackets or football helmets, helps in designing safer and more effective gear.

In all of these examples, applying the principles of physics allows for better decision-making, improved product designs, and increased safety standards. The ability to calculate forces and their effects is essential for creating systems that work efficiently and safely in the real world.