Go Math 5th Grade Answers and Solutions for Success

Success in elementary-level problem solving often relies on building a strong foundation and practicing key concepts regularly. The process of understanding various mathematical principles can be made simpler with the right guidance and tools. This section provides essential solutions and insights to help students excel in their studies.

Developing proficiency in core subjects is crucial at this stage. With step-by-step explanations and helpful tips, learners can navigate even the most challenging exercises with confidence. By addressing common difficulties and offering practical solutions, we aim to make each lesson more approachable.

Exploring different problem-solving techniques is essential for fostering a deeper understanding of concepts. Whether it’s handling numbers, solving puzzles, or applying strategies to real-world scenarios, this section ensures that students are well-equipped to tackle any challenge they encounter.

Go Math 5th Grade Answers Guide

When tackling the challenges presented in elementary problem-solving, having a clear and structured approach is essential. This guide provides students with detailed steps to tackle exercises, helping to clarify concepts and build confidence. From basic operations to more complex tasks, the objective is to equip learners with the tools they need to succeed and develop strong reasoning skills.

Key Strategies for Success

Understanding the foundational techniques is crucial for solving exercises efficiently. Each concept builds upon the previous one, requiring a solid grasp of earlier lessons. By following a logical sequence, students can avoid confusion and achieve mastery more easily.

How to Approach Complex Problems

When confronted with multi-step tasks or tricky scenarios, breaking the problem down into smaller parts is an effective method. Identifying the goal of each step and working through the process systematically allows learners to stay focused and find the right solution.

Step	Strategy	Example
1	Read and Understand the Problem	Identify key details and what is being asked.
2	Break Down the Information	Divide complex tasks into simpler steps.
3	Apply the Right Techniques	Use the appropriate operations or methods.
4	Check and Review	Ensure the solution makes sense and is accurate.

Key Concepts in Go Math 5th Grade

Mastering the fundamental ideas in elementary-level problem-solving is essential for success in more advanced topics. This section highlights the core principles that students need to grasp in order to perform well in their exercises. A solid understanding of these concepts sets the foundation for tackling more complex tasks with confidence and accuracy.

Among the critical topics covered are number operations, pattern recognition, and geometric reasoning. Each concept plays a pivotal role in helping learners develop the skills they need to approach a variety of problems. Focusing on these key areas helps students build a structured framework that supports their learning journey.

By practicing these core ideas, students not only prepare for upcoming challenges but also develop essential cognitive skills that will benefit them in many other areas of learning. From working with whole numbers and fractions to understanding measurement and spatial relationships, these foundational topics are designed to create a well-rounded understanding of how numerical relationships function.

Understanding Math Operations in 5th Grade

At this stage of learning, a strong grasp of fundamental operations is essential for tackling increasingly complex exercises. Whether it’s adding, subtracting, multiplying, or dividing, mastering these core techniques is key to solving more challenging tasks. This section focuses on the essential operations and how they serve as building blocks for more advanced concepts.

Each operation has specific rules and strategies that help students work through problems efficiently. It’s crucial for learners to understand not only how to perform these operations but also when and why they are applied in different situations. By strengthening these skills, students can approach various scenarios with confidence.

Operation	Definition	Example
Addition	Combining two or more numbers to get a total.	8 + 7 = 15
Subtraction	Finding the difference between two numbers.	12 – 5 = 7
Multiplication	Repeated addition of a number a certain number of times.	4 × 6 = 24
Division	Splitting a number into equal parts.	20 ÷ 5 = 4

How to Solve Word Problems Effectively

Word problems can seem intimidating, but with the right approach, they become much easier to solve. The key is to break down the problem into manageable steps and understand what is being asked. This process involves identifying important information, translating it into numbers or equations, and applying the correct methods to find a solution.

Here are some strategies to help solve word problems with greater ease:

• Read the problem carefully: Understand the context and the information provided.
• Identify key details: Look for numbers, relationships, and what the problem is asking for.
• Visualize the situation: Draw diagrams, use charts, or make lists to help organize the information.
• Translate into an equation: Convert the words into a mathematical expression or equation.
• Check your work: After solving, review the steps to make sure the solution makes sense in the context of the problem.

Following a systematic approach helps to avoid confusion and ensures that no important details are overlooked. By practicing these techniques, students will build confidence in solving word problems and develop stronger problem-solving skills overall.

Strategies for Mastering Fractions

Understanding fractions is an essential skill for problem-solving and advancing to more complex topics. Fractions represent parts of a whole, and mastering them requires practice and the ability to see the relationship between the numerator and denominator. By applying the right strategies, students can easily navigate operations involving fractions and improve their overall problem-solving skills.

Here are some effective strategies to help master fractions:

• Visualize the fractions: Use pie charts, number lines, or fraction bars to better understand how fractions are represented.
• Learn to simplify: Reduce fractions to their simplest form by finding the greatest common divisor (GCD) of the numerator and denominator.
• Practice finding equivalent fractions: Multiply or divide both the numerator and denominator by the same number to create equivalent fractions.
• Master common denominators: When adding or subtracting fractions, ensure the denominators are the same by finding a common denominator.
• Use real-world examples: Apply fractions to everyday situations like cooking or measuring to reinforce understanding and make learning more practical.

By practicing these strategies, students will be able to work with fractions confidently, whether they are performing basic operations or solving more complex problems.

Decimals and Their Importance in Go Math

Decimals play a crucial role in developing a deeper understanding of numerical relationships. They are used to express values that are less than one and are fundamental in both everyday life and advanced problem-solving. This section highlights the significance of decimals and how they connect to other mathematical concepts, ensuring students gain a comprehensive understanding of their use and application.

Understanding Decimal Representation

Decimals are simply another way to represent fractions, but in a more convenient form. They allow for easy comparison, addition, subtraction, and other operations. Recognizing the place value of digits in a decimal number is essential for understanding how small portions fit into the larger whole. By learning the value of tenths, hundredths, thousandths, and so on, students can see the relationship between parts of a number and better grasp its meaning.

Application of Decimals in Problem Solving

Once students are comfortable with decimal representation, applying them to solve real-world problems becomes much simpler. From measurements to financial calculations, decimals appear everywhere. In real-life scenarios, decimals help us deal with quantities that are not whole numbers, such as distances, prices, or time. Using decimals correctly ensures precision and accuracy in such tasks.

By mastering decimals, students can work through more complex problems with ease and develop a deeper understanding of how numbers interact in practical situations.

Geometry Basics in Go Math 5th Grade

Geometry forms a key part of understanding spatial relationships and shapes. This section introduces the fundamental concepts of geometry that students need to grasp in order to excel in solving problems related to shapes, angles, and measurements. Mastery of these basic concepts sets the stage for more advanced study in geometry and helps students think critically about the world around them.

Understanding Shapes and Their Properties

One of the first steps in geometry is recognizing and classifying different shapes. From simple polygons like squares and triangles to more complex figures such as parallelograms and trapezoids, each shape has unique characteristics. Understanding properties such as the number of sides, angles, and symmetry is crucial for solving problems and applying geometric concepts.

Working with Perimeter and Area

Another essential concept in geometry is the calculation of perimeter and area. The perimeter of a shape is the total distance around the figure, while the area represents the space enclosed within it. Learning to calculate both helps students understand how measurements are used in real-life situations, such as determining the amount of material needed to cover a surface or the distance around a fenced area.

By mastering these foundational concepts, students will develop a strong understanding of geometry and be well-prepared for more advanced topics in the subject.

Mastering Division and Multiplication Techniques

Building a strong foundation in division and multiplication is crucial for solving a wide variety of numerical problems. These two operations are not only essential in basic arithmetic but also serve as building blocks for more complex concepts. By mastering the techniques and strategies for both division and multiplication, students can solve problems more quickly and accurately, improving their overall problem-solving abilities.

Effective strategies for mastering these operations involve understanding the relationship between numbers, using mental math, and applying various methods such as long division and the distributive property in multiplication. Both operations require practice and familiarity with different techniques to build fluency and confidence.

For division, students should focus on:

• Understanding the division process and the relationship between dividends, divisors, and quotients.
• Using estimation to check the reasonableness of answers.
• Breaking down larger division problems into smaller, easier steps (partial quotients or long division).

For multiplication, the focus should be on:

• Mastering multiplication tables to improve speed and accuracy.
• Using the distributive property to simplify larger problems.
• Breaking down multiplication problems into smaller parts using strategies like the area model or partial products.

By practicing these strategies regularly, students will become proficient in division and multiplication, laying a strong foundation for more advanced math topics.

Algebraic Thinking for 5th Graders

Developing algebraic thinking at an early age helps students to understand the structure of numbers and relationships between them. By exploring patterns, recognizing variables, and solving simple equations, young learners build the necessary skills to tackle more complex mathematical challenges in the future. Algebraic thinking is about recognizing how numbers work together and how to manipulate them in logical ways.

Understanding Patterns and Relationships

One of the first steps in developing algebraic thinking is recognizing and understanding patterns. Patterns can be found in sequences of numbers or shapes and are the foundation of algebraic reasoning. By observing these patterns, students can predict future numbers in a sequence or identify relationships between variables. Recognizing that patterns follow specific rules helps students prepare for solving equations later on.

Introducing Variables and Simple Equations

Another critical aspect of algebraic thinking is learning to work with variables. A variable is simply a symbol, often represented by a letter, that stands for a number. By using variables, students can express general relationships and solve problems in a more abstract way. Simple equations such as x + 5 = 10 help students understand how to isolate a variable and find its value. Learning these skills lays the groundwork for more advanced algebraic concepts.

By mastering these basic concepts, students can develop a strong foundation for future algebraic learning, gaining confidence in their ability to solve more complex problems as they progress.

How to Approach Go Math Review Exercises

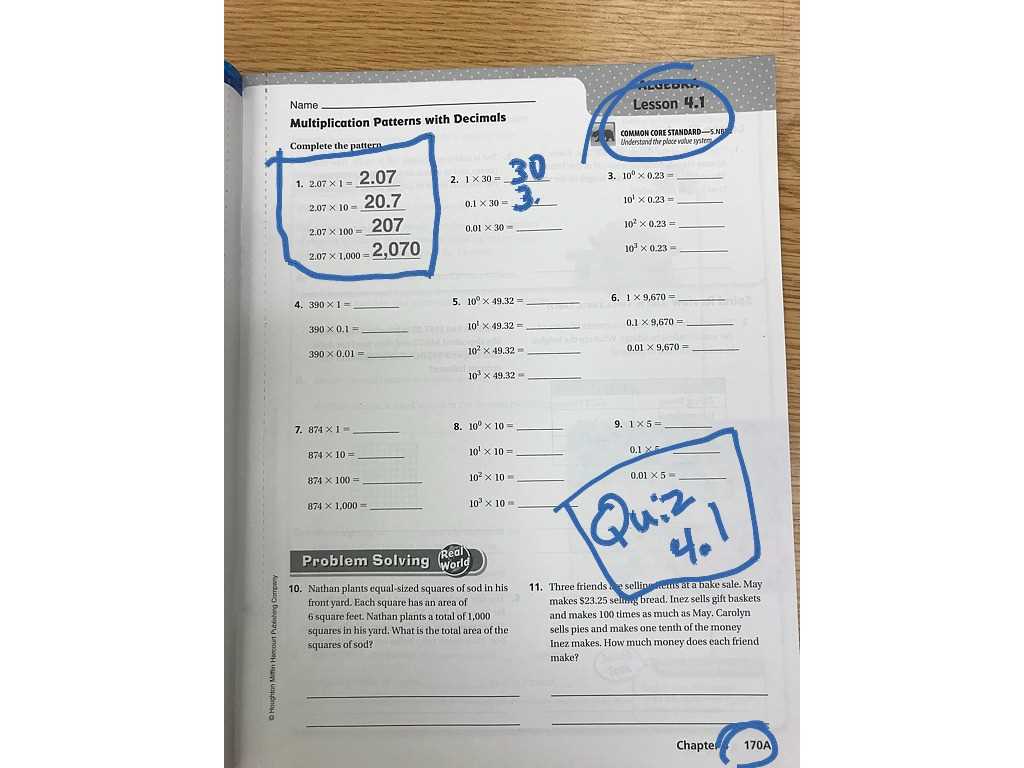
Review exercises are an essential part of reinforcing key concepts and ensuring mastery of topics. By tackling review exercises with a clear strategy, students can strengthen their understanding and identify areas that need improvement. These exercises are designed to help consolidate knowledge, providing an opportunity to apply what has been learned and boost problem-solving skills.

Here’s how to approach these review exercises effectively:

• Read instructions carefully: Before jumping into the problems, make sure to understand the instructions and the type of tasks you’re asked to complete.
• Start with the easy problems: Begin with problems that seem simple or familiar. This builds confidence and helps you focus on more challenging ones later.
• Break down complex questions: For more complicated problems, break them into smaller, more manageable steps. Identify what the question is asking and solve each part step-by-step.
• Use practice strategies: Apply techniques you’ve learned, such as estimation or drawing diagrams, to simplify and solve problems.
• Check your work: Once you’ve completed the exercise, go back and check your answers. Look for any mistakes in calculation or logic and correct them.

By following these steps, students can approach review exercises with a clear plan, making the process more effective and less overwhelming. Consistent practice and review are key to mastering the concepts and improving performance.

Tips for Improving Math Speed and Accuracy

Improving speed and accuracy in solving numerical problems is essential for building confidence and excelling in any subject that involves calculations. By focusing on specific techniques and practicing regularly, students can significantly enhance their problem-solving abilities. The key is to combine quick thinking with precision, ensuring that solutions are both fast and correct.

Here are some effective strategies to boost both speed and accuracy:

• Practice regularly: Consistent practice is crucial. The more frequently you work with numbers, the quicker and more accurate you will become.
• Master basic operations: Becoming fluent in addition, subtraction, multiplication, and division helps speed up problem-solving, as these skills serve as the foundation for more complex tasks.
• Use mental strategies: Develop mental math techniques, such as breaking numbers into smaller parts or rounding, to simplify problems and solve them faster.
• Set time limits: Challenge yourself by solving problems within a set time. This helps build speed while preventing mistakes caused by rushing.
• Check your work: After solving a problem, quickly review your solution to ensure there are no errors. This extra step increases accuracy over time.

Common Mistakes and How to Avoid Them

Being aware of common mistakes can help improve accuracy. Here are a few pitfalls and how to avoid them:

Common Mistake	Solution
Misplacing decimal points	Double-check your decimal placement before finalizing your answer.
Skipping steps in complex problems	Write out all steps to avoid overlooking important details.
Rushing through problems	Slow down and ensure that you fully understand each step before moving on.

By applying these strategies and learning from mistakes, students can develop a faster and more accurate approach to solving problems, enhancing their overall mathematical skills.

Common Mistakes in 5th Grade Math

As students progress through their learning journey, they often encounter common pitfalls that can hinder their understanding and problem-solving abilities. These mistakes typically stem from misconceptions, lack of practice, or rushing through tasks. Identifying and correcting these errors early can help build a strong foundation for more advanced concepts in the future.

Frequent Errors and How to Avoid Them

Here are some of the most common mistakes that students make and strategies to avoid them:

• Misunderstanding Word Problems: Many students struggle with interpreting word problems accurately. They may overlook key information or misinterpret the question.
• Incorrectly Performing Operations: Switching up operations, such as multiplying when division is needed, is a frequent mistake. Always double-check which operation the problem is asking for.
• Skipping Steps: In complex problems, skipping steps can lead to errors. Make sure to follow each step carefully, especially when solving multi-step problems.
• Not Checking Work: Rushing through problems often results in careless mistakes. Always take a moment to review your answers before submitting them.
• Confusing Place Value: Understanding the correct place value of digits in larger numbers can be tricky. Double-check your work when adding, subtracting, or multiplying large numbers.

How to Overcome These Mistakes

To reduce these errors, it’s essential to focus on the following:

• Practice regularly: The more you practice, the more confident you’ll become in solving problems accurately.
• Break down problems: Don’t rush through tasks. Take your time to break down complex problems into smaller, more manageable parts.
• Use visual aids: Drawing diagrams or using manipulatives can help clarify concepts and reduce confusion.
• Ask for help: If you’re unsure about a problem, don’t hesitate to ask for clarification. Understanding the concept fully before moving on is key.

By being aware of these common mistakes and taking steps to avoid them, students can improve their skills and gain confidence in solving problems.

Using Go Math to Prepare for Tests

Preparing for tests can often feel overwhelming, but with the right resources and strategies, students can approach them with confidence. One powerful tool in reinforcing key concepts and building problem-solving skills is the Go Math curriculum. By working through practice exercises, reviewing essential topics, and applying strategies taught throughout the year, students can ensure they are well-prepared for any upcoming assessments.

The key to successful test preparation is consistency and focus. Utilizing the structured lessons provided by Go Math helps reinforce concepts and provides plenty of opportunities to practice. By following a few essential strategies, students can maximize their study time and perform at their best during exams.

Effective Test Preparation Strategies

Here are some tips for using Go Math to prepare effectively:

• Review Key Concepts: Go through each chapter’s key points and examples to refresh your memory. This helps reinforce understanding and ensures you don’t miss any important details.
• Work on Practice Problems: The more you practice, the more confident you’ll become. Focus on both simple and complex problems to sharpen your skills.
• Use Online Resources: If available, utilize online platforms and interactive tools related to Go Math. These resources can provide instant feedback and offer additional practice.
• Time Yourself: Try solving problems within a set time limit. This helps simulate test conditions and improves your ability to manage time effectively during the exam.
• Focus on Weak Areas: Identify areas where you struggle the most and dedicate extra time to improving those skills. Go Math offers a variety of practice exercises tailored to different difficulty levels.

Staying Calm and Confident

Beyond practicing problems, it’s important to develop a calm and confident mindset. Stress can hinder performance, so take time to relax, get plenty of rest, and stay positive. Trust in your preparation and remember that consistent effort throughout the year will pay off during the test.

By using Go Math as a comprehensive tool for review, students can feel prepared and ready to take on any challenge that comes their way during assessments.

Interactive Tools for Go Math Success

In today’s digital age, interactive tools have become essential in supporting learning and enhancing student engagement. These resources allow students to practice concepts in a dynamic and engaging environment, providing instant feedback and allowing for a more personalized approach to problem-solving. By integrating technology with traditional learning methods, students can deepen their understanding and build confidence in their abilities.

When it comes to mastering complex topics, interactive tools offer a variety of features that make learning both fun and effective. From online quizzes to virtual manipulatives, these tools cater to different learning styles and help students develop critical thinking skills in a hands-on way.

Types of Interactive Tools

Here are some interactive tools that can support success in the learning process:

• Online Quizzes and Practice Tests: These quizzes allow students to test their knowledge in real-time, helping them identify areas that need more focus. Immediate feedback is provided, making it easier to learn from mistakes.
• Virtual Manipulatives: Tools such as virtual number lines, fraction bars, and geometric shapes give students a chance to visualize abstract concepts. These manipulatives help make complex ideas more accessible and easier to understand.
• Interactive Games: Learning games that focus on specific topics can engage students while reinforcing skills. Games provide a fun and competitive environment, which can increase motivation and participation.
• Interactive Tutorials and Videos: Video lessons with step-by-step explanations are a valuable resource for students who need extra clarification. These tutorials allow for a more thorough understanding of challenging topics, with the ability to pause and replay as needed.
• Progress Trackers: Many digital tools include progress tracking features that show how much a student has learned and where they still need improvement. This can guide future study sessions and ensure no topic is overlooked.

Maximizing Learning with Technology

To get the most out of interactive tools, it’s important to use them regularly. Consistent practice through these platforms can significantly improve skills and understanding. Additionally, many tools allow students to progress at their own pace, giving them the flexibility to master each concept before moving on to the next.

Incorporating these interactive resources into daily learning not only boosts performance but also makes the entire educational experience more enjoyable and rewarding. By making use of these tools, students are more likely to stay engaged and motivated as they work towards mastering the material.

How to Understand Go Math Explanations

Understanding the instructions and explanations provided in learning materials can be a challenge, especially when dealing with complex concepts. Effective guidance requires a structured approach that breaks down ideas into smaller, manageable steps. This allows learners to grasp key concepts more easily and apply them confidently. Understanding how to interpret and follow these explanations is crucial for mastering each topic and building a solid foundation for more advanced material.

When engaging with these resources, it’s important to approach each explanation with a clear strategy. Often, explanations are written in a way that progresses logically, moving from simpler concepts to more complex ones. Recognizing the structure of these explanations can help make them more comprehensible and less overwhelming.

Steps to Decoding Explanations

Here are some helpful steps for understanding and utilizing explanations effectively:

• Read Thoroughly: Start by reading the explanation carefully, without rushing. This will help you grasp the main idea before diving into the details.
• Highlight Key Concepts: As you read, highlight or underline important terms and steps. This will help you focus on the critical parts of the explanation and make it easier to reference later.
• Work Through Examples: Many explanations include worked-out examples. Take the time to go through these examples step by step. Understand why each action is being taken and how it leads to the solution.
• Ask Questions: If something is unclear, don’t hesitate to ask questions. Whether it’s through an online forum, a teacher, or a study group, getting clarification is key to improving comprehension.
• Practice Independently: Once you’ve understood the explanation, practice similar problems on your own. This will reinforce the concepts and help you build confidence in applying them.

Maximizing Your Understanding

As you continue working through the material, keep revisiting previous explanations. Repetition is a powerful tool for solidifying your understanding. Additionally, breaking down complex explanations into smaller sections and focusing on one piece at a time can prevent feeling overwhelmed and ensure that you’re not skipping over critical steps.

By following these strategies, you’ll improve your ability to understand and apply the explanations, making the learning process smoother and more effective.

Parental Support in 5th Grade Learning

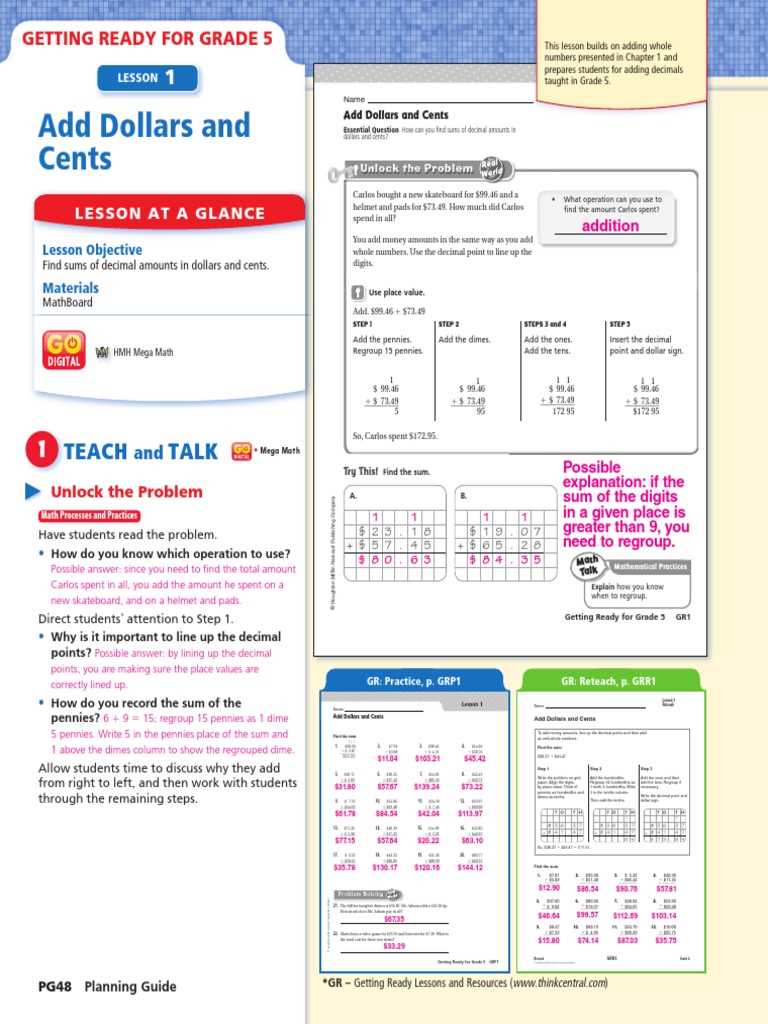
Active involvement from parents plays a critical role in helping children succeed in their academic journey. Providing the right guidance and creating a supportive environment at home can make a significant difference in a child’s confidence and ability to understand new concepts. When it comes to subjects that require problem-solving skills and logical thinking, parental encouragement and involvement can strengthen a child’s foundation and foster a love for learning.

Parents can offer a variety of forms of support, from helping with homework to encouraging independent learning. Being available to listen to questions, offering positive reinforcement, and creating opportunities for practice at home all contribute to a child’s academic growth.

How Parents Can Support Their Child

• Be Present and Engaged: Set aside time to work with your child on assignments. Your involvement shows them that learning is important and gives them a chance to ask questions they may not feel comfortable asking in class.
• Create a Productive Environment: Ensure that your child has a quiet, well-lit space where they can focus on their work without distractions. This simple step can make a big difference in their ability to concentrate.
• Encourage Independent Thinking: Rather than providing answers, encourage your child to think through problems on their own. Ask guiding questions that lead them to discover solutions and develop problem-solving skills.
• Use Resources: Explore additional resources, such as online videos, worksheets, and educational games, to reinforce lessons and provide more practice. These tools can offer a fresh perspective and help clarify challenging concepts.
• Communicate with Teachers: Stay in regular communication with your child’s teacher. This allows you to stay informed about their progress, understand areas where they may need extra help, and discuss strategies for improving performance.

Building a Positive Attitude Toward Learning

Parents also play an essential role in fostering a positive attitude toward learning. Encouragement and praise for effort–rather than just correct answers–help children feel confident in their abilities. Recognizing small victories and providing constructive feedback can motivate your child to approach challenges with a problem-solving mindset.

When parents actively participate in their child’s education, they contribute to a more confident and resilient learner. This collaborative approach not only supports academic achievement but also nurtures lifelong skills and a positive approach to learning.