Algebra 2 Extra Practice Answers to Enhance Your Skills

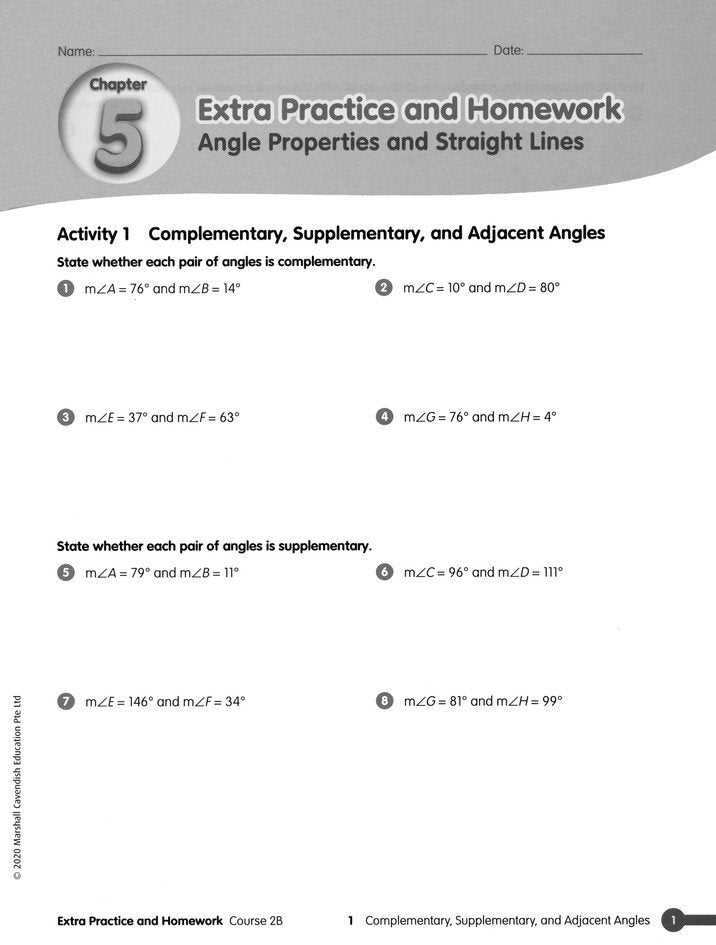
In any mathematical journey, strengthening foundational knowledge is key to mastering more complex topics. By delving deeper into challenging exercises, students can sharpen their problem-solving skills and build confidence in tackling intricate equations. Repetition and careful analysis are often the most effective ways to ensure a solid understanding of difficult material.
This section provides comprehensive solutions for a wide range of advanced problems. Each solution is broken down into clear steps, allowing learners to follow the thought process behind every calculation. The goal is not just to find the correct results, but to develop a logical approach to similar challenges that might arise in future studies or exams.
By working through these problems, learners can better understand the methods needed to solve various mathematical scenarios. With each completed problem, they will gain more insights into the reasoning behind the steps and how to apply similar techniques to different situations. Whether you are revising for an exam or enhancing your understanding of a specific topic, this guide offers valuable tools for success.
Advanced Math Problem Solving Guide
Mastering complex mathematical problems requires more than just memorizing formulas; it involves understanding underlying concepts and developing a systematic approach to problem-solving. This section offers a detailed breakdown of how to approach challenging exercises, with clear explanations at each stage of the process. The goal is to help learners not only reach the correct results but also develop a deeper comprehension of the strategies used to solve problems.
Step-by-Step Breakdown of Solutions
Each problem is solved step by step, ensuring that every calculation is explained clearly. By following the breakdown, students can see how individual steps build upon one another, leading to the final solution. This method reinforces learning and highlights the logical progression required to reach the correct conclusion, making it easier to replicate when encountering similar problems in the future.
Practical Tips for Success
While solving mathematical problems can be challenging, there are several strategies that can make the process more efficient. First, always identify the core concepts involved in the problem. Then, break the task into smaller, manageable parts. Stay organized and avoid rushing through steps. Consistency is key–practicing regularly and reviewing completed exercises will help solidify knowledge and enhance skills over time.
Understanding the Importance of Practice
Regular engagement with challenging problems is essential for improving mathematical skills. By consistently working through exercises, learners can refine their problem-solving techniques, gain confidence, and deepen their understanding of key concepts. The process of repetition not only helps in mastering various methods but also reinforces the ability to apply them effectively in different scenarios.
- Repetition builds confidence and familiarity with problem types.
- It helps identify patterns and relationships within mathematical concepts.
- Frequent practice enhances critical thinking and problem-solving speed.
- Regular exposure to various problem-solving techniques allows for flexibility in approaching new challenges.
By making problem-solving a routine part of the learning process, students can develop a more intuitive grasp of complex topics. In the long run, this consistent effort leads to improved performance and greater comfort with advanced mathematical tasks.
Key Concepts in Algebra 2
Mastering higher-level mathematics involves grasping a set of fundamental ideas that form the foundation for more advanced topics. These concepts are crucial for solving a wide variety of problems and understanding the relationships between different mathematical elements. By focusing on these core ideas, students can build the skills necessary to tackle complex equations and real-world applications.
Understanding Equations and Functions
One of the most important concepts is the ability to work with different types of equations and functions. From linear and quadratic equations to exponential and logarithmic functions, knowing how to manipulate and solve them is essential. Each function has its own properties and rules, and understanding these allows for more efficient problem-solving and graph interpretation.
Systems of Equations and Inequalities
Another crucial area is working with systems of equations and inequalities. Solving systems involves finding common solutions for multiple equations simultaneously, whether through substitution, elimination, or graphing methods. Additionally, understanding inequalities and their graphical representation is important for solving real-world problems that involve ranges of values rather than exact numbers.
Common Mistakes and How to Avoid Them
While working through complex mathematical problems, it’s easy to make errors that can hinder progress. These mistakes often arise from simple misunderstandings, rushing through steps, or overlooking important details. Identifying common pitfalls and learning how to avoid them is key to improving accuracy and mastering the material.
Misunderstanding Key Concepts

One frequent mistake is misinterpreting the underlying principles behind equations or functions. This often leads to incorrect approaches when solving problems. To avoid this, it’s essential to take the time to fully understand the rules and properties of the concepts involved. Revisiting foundational knowledge and clarifying any confusion can prevent errors in more complex tasks.
Skipping Steps and Rushing
Another common error is skipping steps in an attempt to save time, especially in multi-step problems. This can lead to missed calculations or incorrect simplifications. It’s important to follow a structured approach and double-check each step before moving forward. Taking time to ensure each part is correct will reduce the likelihood of mistakes and help build a more solid understanding of the material.
Step-by-Step Solutions Explained
Understanding complex problems becomes much easier when each step is broken down clearly. In this section, we will walk through detailed solutions to common types of problems, demonstrating the reasoning behind each step. This approach allows learners to follow the logical progression from start to finish, ensuring that each part of the problem is understood before moving to the next.
Breaking Down the Process
Every problem can be tackled by identifying key elements and applying the correct methods in sequence. By isolating each part of the task, we can focus on solving smaller, manageable sections one at a time. Whether simplifying expressions, solving for unknowns, or graphing functions, taking it step by step reduces errors and makes the process more comprehensible.
Applying the Right Techniques
Throughout each example, we will highlight the methods and formulas used to arrive at the solution. This approach not only clarifies the specific steps required but also demonstrates why each technique is chosen. By practicing these methods, learners will be better equipped to apply them to a variety of similar problems in the future.
How to Solve Complex Equations
Solving intricate mathematical equations can initially seem daunting, but with the right approach, it becomes a systematic process. The key is to break down the problem into smaller, more manageable parts, applying the appropriate methods at each stage. Whether dealing with multiple variables, exponents, or complex fractions, the steps remain consistent: simplify, isolate, and solve.
Step 1: Simplify the Expression
Before diving into the solution, it’s important to simplify the equation as much as possible. This includes combining like terms, eliminating parentheses, and reducing fractions. The more simplified the equation, the easier it is to identify the unknown values.
Step 2: Isolate the Variable
Once the equation is simplified, the next step is to isolate the variable. This can be done by performing inverse operations, such as adding, subtracting, multiplying, or dividing both sides of the equation. The goal is to get the variable on one side of the equation with a coefficient of 1.
Step 3: Check for Special Cases
Sometimes, equations can have special cases like no solution or infinite solutions. It’s essential to check if the variable cancels out entirely or if both sides of the equation are equivalent, which could indicate these scenarios. Identifying these situations early helps avoid unnecessary steps.
Mastering Quadratic Equations
Quadratic equations are a fundamental topic in advanced mathematics, often requiring different methods to solve. To truly master these equations, one must understand the underlying patterns and be able to apply various techniques efficiently. Whether solving by factoring, using the quadratic formula, or completing the square, each approach offers a unique advantage depending on the form of the equation.
Solving by Factoring
Factoring is one of the simplest methods for solving quadratic equations, especially when the equation is easily factorable. The goal is to express the equation as a product of binomials, which can then be set to zero. Once factored, finding the solutions is straightforward by setting each factor equal to zero.
Using the Quadratic Formula
For equations that are not easily factorable, the quadratic formula is a reliable method. It works for all quadratic equations and provides exact solutions, even when the roots are irrational or complex. The formula is:
| Formula | Explanation |
|---|---|
| x = (-b ± √(b² – 4ac)) / 2a | This formula gives the roots of any quadratic equation of the form ax² + bx + c = 0. |
To use the quadratic formula, simply plug in the values for a, b, and c from the equation and calculate the discriminant (b² – 4ac). The discriminant will tell you whether the solutions are real or complex.
Polynomials and Their Applications
Polynomials are powerful mathematical expressions that play a key role in many areas of mathematics and science. These expressions, which involve variables raised to whole-number exponents, are used to model a wide variety of real-world situations. Understanding polynomials and their applications is crucial for tackling more advanced topics and solving complex problems across different fields.
Real-World Uses of Polynomials

Polynomials are used to represent relationships in fields ranging from physics and engineering to economics and biology. Some of the most common applications include:
- Modeling Growth and Decay: Polynomials can model exponential growth or decay, such as population growth or the rate of chemical reactions.
- Physics and Engineering: In physics, polynomials are used to describe motion, energy, and force in systems. Engineers also use them to model structures and optimize designs.
- Financial Modeling: In economics and finance, polynomials can represent costs, profits, and other economic factors over time.
Solving Polynomial Equations

One of the main applications of polynomials is solving polynomial equations. These equations can be solved through a variety of methods, including factoring, synthetic division, or using the rational root theorem. The goal is to find the values of the variable that satisfy the equation, which can then be used in real-life scenarios to make predictions or decisions.
- Start by simplifying the polynomial expression.
- Look for common factors or try factoring the polynomial if possible.
- If factoring is not possible, use the rational root theorem or synthetic division to find solutions.
Rational Expressions Simplified
Rational expressions are fractions where the numerator and denominator are both polynomials. Simplifying these expressions is an essential skill, as it makes the equations easier to work with and solves real-world problems more efficiently. By reducing the complexity of a rational expression, we can perform operations such as addition, subtraction, multiplication, and division with greater ease.
Steps to Simplify Rational Expressions
To simplify rational expressions, follow these key steps:
- Factor the Numerator and Denominator: Begin by factoring both the numerator and the denominator completely. This step helps identify common factors that can be canceled.
- Cancel Common Factors: Once the expression is factored, cancel out any common factors that appear in both the numerator and denominator. Be careful to avoid canceling terms that aren’t common factors.
- Look for Simplified Terms: After cancellation, examine the remaining terms to see if further simplification is possible.
Example of Simplification

Consider the rational expression:
(x² – 4) / (x² – 2x)
- First, factor both the numerator and denominator:
- Numerator: x² – 4 = (x – 2)(x + 2)
- Denominator: x² – 2x = x(x – 2)
- Now, cancel out the common factor of (x – 2):
- The simplified expression is (x + 2) / x.
By following these steps, we simplify the rational expression and make it easier to work with in further calculations.
Exponents and Logarithms Demystified
Exponents and logarithms are two fundamental concepts in mathematics that are closely related. While exponents deal with repeated multiplication, logarithms offer a way to reverse this process. Understanding how these concepts work together is essential for solving a wide range of problems, from simple equations to complex applications in science and engineering. Once the principles behind exponents and logarithms are clear, working with them becomes a straightforward task.
Exponents Explained
Exponents describe how many times a number (the base) is multiplied by itself. For instance, 23 means 2 multiplied by itself three times, or 2 × 2 × 2 = 8. The exponent tells you the power to which the base is raised, and mastering exponents is key to simplifying and solving many types of equations.
Logarithms and Their Role
Logarithms are essentially the inverse of exponents. They answer the question: “To what power must a number be raised to obtain a given value?” For example, the logarithmic form log2 8 = 3 means that 2 raised to the power of 3 equals 8. Logarithms are particularly useful for solving equations involving exponential growth or decay, such as those found in finance, biology, and physics.
| Exponential Form | Logarithmic Form |
|---|---|
| 23 = 8 | log2 8 = 3 |
| 104 = 10000 | log10 10000 = 4 |
Both exponents and logarithms play a crucial role in solving real-world problems. By becoming proficient with these operations, you unlock the ability to solve complex equations and understand processes that vary exponentially over time.
Graphing Functions Effectively
Graphing functions is a critical skill for understanding how variables relate to one another. By representing equations visually, you can quickly identify patterns, behavior, and key characteristics such as intercepts, slopes, and asymptotes. Effective graphing simplifies complex problems and provides insight into the nature of the relationships described by mathematical expressions.
Key Steps for Graphing
To graph functions efficiently, it’s important to follow a systematic approach:
- Identify the Type of Function: Recognize the type of function you are dealing with, whether it’s linear, quadratic, exponential, or logarithmic. Each type has distinct features that will affect how you graph it.
- Plot Key Points: Start by calculating key points, such as intercepts and turning points. These values are often the most helpful when sketching the curve.
- Analyze the Behavior: Look at the behavior of the function for large and small values of x. For example, exponential functions grow rapidly, while linear functions increase at a constant rate.
Understanding the Coordinate Plane
While graphing, the coordinate plane is your most useful tool. The x-axis represents the independent variable, and the y-axis represents the dependent variable. Understanding how to use these axes effectively allows you to represent functions with precision.
In the case of polynomial functions, for example, you may need to consider how the function behaves near the x-axis, identifying critical points such as local maxima or minima. For trigonometric functions, understanding the amplitude, period, and phase shifts is key to accurate graphing.
By following these steps, you’ll be able to graph a wide range of functions quickly and accurately, which is crucial for solving many types of mathematical problems.
Systems of Equations Solved
Solving systems of equations involves finding the values of variables that satisfy multiple equations simultaneously. This is a fundamental technique in mathematics that applies to a variety of real-world problems, including those related to economics, engineering, and physics. By using different methods, such as substitution, elimination, and graphical representation, you can efficiently find the solution to a system.
Methods for Solving Systems
There are several methods for solving systems of equations, each useful in different scenarios. The most common approaches include:
- Substitution: Solving one equation for one variable and substituting this value into the other equation.
- Elimination: Adding or subtracting the equations to eliminate one variable, allowing you to solve for the other.
- Graphing: Plotting both equations on a graph and identifying the point of intersection, which represents the solution.
Example of Solving a System
Consider the following system of equations:
| Equation |
|---|
| 2x + 3y = 12 |
| x – y = 1 |
To solve this system using the substitution method, you would first solve one of the equations for one variable. For instance, solve the second equation for x:
x = y + 1
Next, substitute x = y + 1 into the first equation:
2(y + 1) + 3y = 12
Simplifying:
2y + 2 + 3y = 12
5y + 2 = 12
5y = 10
y = 2
Now substitute y = 2 back into the equation x = y + 1:
x = 2 + 1 = 3
The solution to the system is x = 3 and y = 2.
Working with Matrices in Algebra
Matrices are powerful tools used to represent and solve systems of linear equations, transformations, and much more. They provide a compact and organized way to handle data, and matrix operations like addition, multiplication, and inversion are essential for solving complex problems in various fields such as computer science, physics, and economics. Understanding how to manipulate matrices can significantly simplify mathematical computations and lead to more efficient solutions.
Matrix Operations

There are several key operations when working with matrices, including addition, subtraction, and multiplication. Each of these operations follows specific rules, and understanding them is crucial for solving problems efficiently. Below is an example of matrix addition and multiplication:
Example: Matrix Addition
Consider the following two matrices:
| Matrix A | Matrix B | ||||||||
|---|---|---|---|---|---|---|---|---|---|
|
|
The sum of these matrices is found by adding corresponding elements:
| Matrix A + Matrix B | ||||
|---|---|---|---|---|
|
Matrix Multiplication
Matrix multiplication involves multiplying rows of the first matrix by columns of the second matrix. For example, multiplying a 2×2 matrix by another 2×2 matrix is done as follows:
| Matrix A | Matrix B | ||||||||
|---|---|---|---|---|---|---|---|---|---|
|
|
To multiply these matrices, multiply each element of the row of the first matrix by each corresponding element of the column in the second matrix:
| Result of A × B | ||||
|---|---|---|---|---|
|
The result is a new matrix:
| Matrix A × Matrix B | ||||
|---|---|---|---|---|
|
Through these matrix operations, you can solve complex systems and perform various transformations, making matrices a vital tool in both theoretical and applied mathematics.
Solving Inequalities with Ease
Solving inequalities is a crucial skill in mathematics, allowing you to understand relationships between quantities and make decisions based on conditions. Whether you’re working with linear or more complex inequalities, the process typically involves isolating the variable and understanding how to manipulate the inequality symbol correctly. Mastering these techniques helps you solve real-world problems, from budgeting to engineering, where constraints and limits are important considerations.
Basic Steps for Solving Inequalities

To effectively solve inequalities, follow these basic steps:
- Step 1: Identify the inequality symbol (greater than, less than, etc.) and understand its meaning.
- Step 2: Simplify both sides of the inequality as much as possible, just like you would for an equation.
- Step 3: Isolate the variable on one side of the inequality.
- Step 4: Perform operations (such as addition, subtraction, multiplication, or division) while ensuring the inequality symbol remains correct.
- Step 5: If you multiply or divide by a negative number, flip the inequality symbol.
Example: Solving a Simple Inequality
Consider the inequality:
2x + 5 > 11
To solve this inequality:
- Subtract 5 from both sides: 2x > 6
- Divide both sides by 2: x > 3
The solution is x > 3, which means any value greater than 3 will satisfy the inequality.
Understanding the process and practicing with different examples will allow you to solve inequalities with confidence and efficiency. This knowledge is vital for many higher-level mathematical problems and real-world applications.
Exploring Algebraic Word Problems
Word problems are an essential part of mathematics, as they help bridge the gap between abstract concepts and real-world applications. These problems require you to translate everyday situations into mathematical expressions or equations. By carefully analyzing the given information and identifying the unknowns, you can set up and solve problems that mirror real-life challenges, such as budgeting, planning, or determining quantities in business or science.
Steps for Solving Word Problems
To tackle algebraic word problems effectively, follow these essential steps:
- Step 1: Read the problem carefully and identify the information provided and what needs to be determined.
- Step 2: Assign variables to represent unknown quantities. Make sure the variables are clear and meaningful in the context.
- Step 3: Translate the words into mathematical expressions or equations. Look for key terms such as “total,” “difference,” “product,” and “sum” to guide your formulation.
- Step 4: Solve the equation or expression you created, using appropriate mathematical operations.
- Step 5: Check your solution to ensure it makes sense in the context of the problem.
Example: A Business Problem
Imagine you are running a small business, and you need to determine how many products you can sell to cover your expenses. Here’s a word problem:
“You are selling handmade candles. The fixed cost of running your business is $200 per month, and you make $15 per candle. How many candles must you sell to break even?”
To solve this problem:
- Let x represent the number of candles to be sold.
- The total cost is $200, and the revenue from selling candles is 15x.
- Set up the equation: 15x = 200
- Divide both sides by 15: x = 200 / 15 ≈ 13.33
- Since you can’t sell a fraction of a candle, round up to 14 candles.
The solution is that you need to sell at least 14 candles to cover your costs and break even. By practicing this approach, you’ll become more comfortable with interpreting and solving word problems in various real-world situations.
How to Use Online Resources
The internet offers a wealth of tools and platforms to support learning and problem-solving in mathematics. Online resources can help you better understand complex concepts, provide practice opportunities, and give you access to step-by-step solutions. By utilizing these resources effectively, you can improve your skills and tackle difficult topics with confidence. Below are some key strategies to make the most out of available online learning tools.
1. Identifying Reliable Platforms
Before diving into online resources, it’s important to identify trusted platforms that offer quality content. Look for websites or tools that:
- Provide clear explanations: Choose platforms that break down concepts in a simple, understandable way.
- Offer interactive tools: Interactive exercises can help reinforce what you’ve learned.
- Include step-by-step guides: These can help you solve problems with detailed instructions, making it easier to grasp complex steps.
- Have a variety of resources: Access different formats, including videos, written tutorials, and quizzes, to cater to various learning preferences.
2. Using Online Tools Effectively
To maximize the benefits of online platforms, follow these tips:
- Use video tutorials: Videos are great for visual learners, providing demonstrations that show you how to approach and solve different problems.
- Take advantage of practice quizzes: Quizzes help solidify your understanding by testing your skills in real-time, offering immediate feedback on your performance.
- Participate in forums and communities: Engage with online communities to ask questions, share insights, and learn from others’ experiences.
- Follow structured learning paths: Some websites offer courses that are designed to guide you step-by-step through topics, making your learning journey more organized.
By consistently utilizing these strategies, you can make the most of online resources, enhancing your learning experience and tackling mathematical challenges more effectively.
Tips for Efficient Studying
Effective studying is key to mastering complex concepts and improving performance in any subject. With the right strategies, you can optimize your time, enhance your understanding, and retain information more efficiently. Below are some valuable tips to make your study sessions more productive and focused.
1. Set Clear Goals
Start each study session with a specific objective in mind. Whether it’s to understand a particular concept, solve a set of problems, or review key ideas, having a clear goal helps maintain focus and ensures that you’re working with purpose. Break down larger tasks into smaller, manageable steps to avoid feeling overwhelmed.
2. Create a Consistent Schedule
Consistency is important when it comes to retaining information. Set aside regular time each day or week for studying, and stick to it. Establishing a routine helps your brain stay in learning mode, making it easier to absorb new material over time. Find a time when you’re least likely to be distracted, whether in the morning, afternoon, or evening.
3. Eliminate Distractions
Minimize distractions during your study time to maintain full focus. Turn off notifications, put away your phone, and create a quiet, comfortable environment. If you’re studying online, use apps or browser extensions that block distracting websites to keep you on track.
4. Use Active Learning Techniques
Instead of passively reading or watching videos, engage with the material actively. Take notes, solve problems, explain concepts aloud, or teach someone else. These techniques reinforce your understanding and help identify any gaps in your knowledge.
5. Review Regularly
Don’t wait until the last minute to review material. Set aside time each week to go over what you’ve already studied. Spaced repetition helps improve long-term retention and ensures that you don’t forget important details.
6. Practice Problem-Solving
To deepen your understanding, solve as many problems as you can. Practical application of concepts is essential for solidifying what you’ve learned. Make sure to check your solutions, identify mistakes, and learn from them.
7. Take Breaks
Studying for long periods without breaks can lead to burnout. Use techniques like the Pomodoro Method–study for 25 minutes and then take a 5-minute break. This helps maintain focus and keeps your mind fresh throughout your study session.
By incorporating these strategies into your study routine, you can work more efficiently, boost your comprehension, and approach learning with confidence.
Preparing for Algebra 2 Exams
Effective preparation is crucial for performing well in any test. When it comes to exams that cover advanced mathematical concepts, it’s important to adopt a focused, organized approach. By reviewing key topics, honing problem-solving skills, and managing your time efficiently, you can approach your exam with confidence. Below are strategies that can help ensure success in your upcoming assessment.
1. Understand the Exam Format
Before diving into your study materials, take some time to understand the structure of the exam. Is it multiple-choice, open-ended, or a mix of both? Are there specific sections covering different topics? Knowing the format will help you prepare accordingly and focus on areas that are most likely to appear in the exam.
2. Review Key Concepts
Focus on understanding the fundamental concepts that the exam will test. Make a list of important topics, such as functions, equations, inequalities, and graphing techniques. Go over the definitions, formulas, and key procedures for solving problems related to these topics. Create summary notes or flashcards to reinforce your understanding.
3. Solve Past Papers and Sample Problems
Working through previous exams or sample problems is one of the most effective ways to prepare. By doing this, you familiarize yourself with the types of questions that may appear and practice solving them within a time limit. Pay attention to recurring patterns or themes in the problems, as these are likely to appear again.
4. Time Yourself During Practice
Simulate exam conditions by timing yourself while solving practice questions. This will help you manage time effectively during the actual exam, ensuring that you don’t spend too long on any one question. Practice pacing yourself so that you can move through the exam efficiently and complete all sections within the allotted time.
5. Identify Weak Areas and Focus on Them
As you review and solve practice problems, take note of any areas where you struggle. It’s important to focus on these weaknesses to build your confidence. Seek help from teachers, tutors, or online resources to clarify any concepts that you find particularly challenging.
6. Use Active Recall and Spaced Repetition
Instead of passively reviewing notes, use active recall by testing yourself on key concepts. Spaced repetition–reviewing material at increasing intervals–also helps strengthen your long-term memory. These techniques are proven to improve retention and understanding.
7. Stay Calm and Rested
On the day before the exam, avoid cramming. Instead, focus on reviewing your notes and staying relaxed. Ensure that you get a good night’s sleep so that your mind is fresh and alert. During the exam, stay calm, and take a deep breath before tackling each question.
By following these strategies and remaining disciplined in your preparation, you’ll be well-equipped to handle the challenges of the exam and perform to the best of your abilities.