Algebra 2 Placement Test Answers and Study Guide

Successfully navigating advanced mathematics challenges is crucial for students aiming to progress in their academic journey. Whether you are moving on to higher-level courses or seeking to demonstrate your skills, knowing how to tackle mathematical problems efficiently is essential. This guide will help you understand the core concepts and strategies that can improve your performance in assessments designed to evaluate your understanding of various mathematical principles.

Mastering topics such as functions, equations, and inequalities requires a solid foundation and a strategic approach. With the right preparation, you can confidently approach each question and solve complex problems more effectively. This article will provide insights into the areas most commonly tested, as well as techniques to help you overcome challenges and improve your problem-solving speed.

By breaking down key concepts and offering practical study tips, this guide is aimed at equipping you with the tools you need to succeed. Whether you’re practicing on your own or seeking additional resources, the focus here is on giving you the confidence to excel when it matters most.

Algebra 2 Placement Test Answers

When preparing for an advanced level mathematics assessment, it’s important to understand the structure and content of the problems you’ll encounter. Mastery of essential concepts can make a significant difference in achieving a high score. This section focuses on providing helpful insights into the types of problems typically found in such evaluations and offers guidance on how to approach them effectively.

Key Concepts to Focus On

Core topics in advanced mathematics are often designed to test your understanding of complex relationships between numbers and variables. From solving equations to analyzing functions, a strong grasp of each subject is crucial. Identifying the most frequently tested areas, such as solving quadratic equations, working with inequalities, and interpreting graphical data, will provide a solid foundation for tackling challenges efficiently.

Effective Strategies for Success

While knowing the material is important, having the right approach during the assessment can help you manage time and reduce errors. It’s essential to work through problems systematically, ensuring that you understand each step before proceeding. By practicing similar questions beforehand, you’ll increase your confidence and improve your ability to solve even the most challenging problems under pressure.

Understanding Algebra 2 Placement Tests

Evaluations designed to measure mathematical proficiency at an advanced level assess how well students can apply key concepts and solve complex problems. These assessments challenge individuals to demonstrate their ability to manipulate mathematical expressions, solve equations, and interpret data effectively. Understanding the structure and expectations of such evaluations is essential for success.

The purpose of these assessments is not only to gauge a student’s current knowledge but also to identify areas for growth. They typically cover a broad range of topics, from linear equations and polynomials to more advanced concepts such as exponential functions and systems of equations. Knowing what to expect can help students focus their preparation efforts and approach each problem with confidence.

Why Placement Tests Matter

Assessments at the advanced level are critical for determining the appropriate course of study for students. These evaluations provide insight into a student’s ability to handle more challenging material and ensure they are placed in the right academic environment. The results guide educators in tailoring instruction to meet individual needs, setting the stage for a more effective learning experience.

Beyond the immediate goal of course placement, such assessments also help identify strengths and areas for improvement. Understanding where a student excels and where they may need additional support ensures a focused approach to learning. Below is a table that outlines the key benefits of taking these assessments:

Benefit	Description
Personalized Learning	Helps identify the student’s current skill level, enabling targeted learning plans.
Course Alignment	Ensures that students are enrolled in the most appropriate courses for their abilities.
Progress Monitoring	Tracks the student’s development over time, allowing for adjustments in teaching strategies.
Confidence Building	Provides students with an understanding of their own academic strengths and weaknesses.

Key Topics in Algebra 2

Advanced mathematical evaluations cover a wide range of topics, each focusing on specific skills and concepts. Mastery of these key areas is essential for achieving success, as they form the foundation of more complex problem-solving and analysis. Understanding the primary subjects often tested helps students focus their study efforts on what matters most.

Essential Mathematical Concepts

The most commonly tested topics involve manipulating expressions, solving equations, and interpreting data. Mastery of these core areas ensures that students are prepared for more intricate challenges. Below is a table outlining the main topics students should focus on:

Topic	Description
Linear Equations	Understanding and solving equations with one or more variables.
Quadratic Functions	Solving equations and analyzing graphs of quadratic expressions.
Polynomials	Operations involving polynomials, including addition, subtraction, and factoring.
Rational Expressions	Manipulating and simplifying expressions with fractions involving variables.
Exponential Functions	Solving and graphing equations with exponential growth or decay.

Understanding Complex Numbers

Another important area is the study of complex numbers, which extends the concept of numbers to include imaginary components. Mastering complex numbers is crucial for solving more advanced equations that do not have real solutions. The ability to work with both real and imaginary numbers is a key skill in more advanced mathematics.

How to Prepare for the Test

Effective preparation for advanced mathematical evaluations requires a clear strategy and focused effort. Knowing which areas to concentrate on and how to approach practice problems can significantly improve your performance. Developing a study routine and familiarizing yourself with common problem types will build confidence and ensure that you’re ready when it matters.

Start by reviewing key topics that are most frequently covered, such as solving equations, graphing functions, and working with polynomials. Understanding these concepts will help you feel more comfortable during the assessment. Practice is key, so make sure to work through a variety of problems, gradually increasing the difficulty to match the level of the actual evaluation.

Another crucial aspect of preparation is managing your time effectively. Set aside dedicated time each day for focused study and avoid last-minute cramming. Try using practice exams to simulate real test conditions and identify areas where you may need additional review. The more prepared you are, the more confident you will feel during the actual assessment.

Common Types of Questions

Advanced assessments typically include a variety of problem types designed to test a student’s understanding of key mathematical concepts. These problems often involve solving equations, interpreting graphs, and applying formulas to real-world scenarios. Understanding the most common types of questions can help you prepare more effectively and avoid unnecessary stress during the evaluation.

Problem Types to Expect

Familiarizing yourself with common problem types will help you feel more confident. These can range from solving basic equations to working with more complex functions and expressions. Below is a table outlining the most frequently encountered question types:

Question Type	Description
Equation Solving	Finding the values of variables that satisfy the given equation.
Graphing Functions	Plotting functions on a coordinate plane and interpreting their behavior.
Word Problems	Solving practical problems by translating verbal descriptions into mathematical expressions.
Systems of Equations	Finding solutions to multiple equations that share common variables.
Factorization	Breaking down expressions into their simplest components.

Understanding the Structure

Questions may vary in complexity, but they are generally structured to assess both basic computational skills and deeper problem-solving abilities. Whether it’s simplifying expressions or solving for unknowns, practicing these common question types will prepare you to tackle any challenge efficiently during the assessment.

Test-Taking Strategies for Success

Approaching a mathematical evaluation with the right mindset and strategies can make a significant difference in your performance. While knowing the material is essential, how you manage your time, tackle problems, and stay focused during the assessment is just as important. Effective test-taking strategies can help you maximize your potential and reduce unnecessary stress.

Prioritize Easy Questions First

Start by quickly scanning the entire assessment and identifying the problems you feel most confident about. Answer these questions first, as they are typically easier to solve and will help build momentum. This will also give you a sense of accomplishment early on, boosting your confidence for the more challenging problems.

Manage Your Time Wisely

Time management is crucial, especially when facing a lengthy or challenging evaluation. Allocate a specific amount of time to each section and try to stick to it. If you encounter a particularly difficult problem, move on and return to it later, rather than spending too much time on a single question. This approach ensures that you have time to complete the entire assessment.

Solving Quadratic Equations in Tests

Solving equations that involve squared variables is a fundamental skill in advanced mathematics. These types of problems often appear in evaluations and require a clear understanding of different methods to find solutions. Whether you’re solving for real or complex roots, it’s essential to be familiar with the key techniques that will help you arrive at the correct answer efficiently.

Methods for Solving Quadratic Equations

There are several methods for solving these equations, each of which is useful depending on the specific form of the problem. The most common methods include factoring, completing the square, and using the quadratic formula. Mastering each technique allows you to choose the most efficient approach based on the structure of the equation.

Tips for Success with Quadratic Equations

When faced with a quadratic problem, start by identifying the form of the equation to determine which method will be most effective. If the equation can be factored easily, this is often the quickest solution. If factoring is not possible, completing the square or using the quadratic formula will be necessary. Always check your work by substituting your solution back into the original equation to verify that it satisfies the given expression.

Functions and Their Importance in Testing

Understanding functions and how they behave is crucial in any advanced mathematics evaluation. Functions serve as a foundational concept for solving a wide range of problems, from simple equations to more complex scenarios involving graphs and real-world applications. Knowing how to work with functions can make a significant difference in performing well during an evaluation.

In mathematical assessments, functions are often tested in various forms. Here are some common aspects of functions you should focus on:

• Understanding Function Notation: The ability to recognize and interpret function notation is essential for solving problems accurately.
• Graphing Functions: Many problems require you to plot functions on a coordinate plane and interpret their behavior, such as identifying intercepts and asymptotes.
• Transformations of Functions: Knowing how transformations such as shifts, stretches, and reflections affect the graph of a function is critical.
• Solving Function Equations: Being able to solve for unknown variables in function equations is a fundamental skill.

By mastering these key aspects of functions, you will be better prepared to tackle any problems that involve them, ensuring you can solve the problem accurately and efficiently.

Mastering Inequalities for Placement Tests

In mathematical assessments, solving inequalities is an essential skill. These types of problems require you to understand relationships between values and how they can be expressed in different forms. Whether dealing with linear or compound inequalities, mastering these concepts is key to performing well on assessments. Being able to manipulate inequalities effectively allows you to solve a wide range of problems accurately.

Types of Inequalities

Inequalities come in various forms, and each type requires a slightly different approach. The most common forms you’ll encounter include:

• Linear Inequalities: These involve expressions with one variable and can be solved by similar methods as equations, but with special attention to the direction of the inequality sign.
• Compound Inequalities: These involve two inequalities that are connected by “and” or “or.” It’s crucial to understand how to combine the solutions of both parts.
• Quadratic Inequalities: These require factoring or using the quadratic formula to find the boundary points, then testing intervals to determine the solution set.

Strategies for Solving Inequalities

When solving inequalities, always remember to reverse the inequality sign when multiplying or dividing by a negative number. This is a critical step that many students overlook. Additionally, graphing the solution set on a number line can provide a visual representation that makes it easier to understand the solution. Practice is key in building proficiency, so work through various examples to solidify your understanding.

Polynomials and Rational Expressions

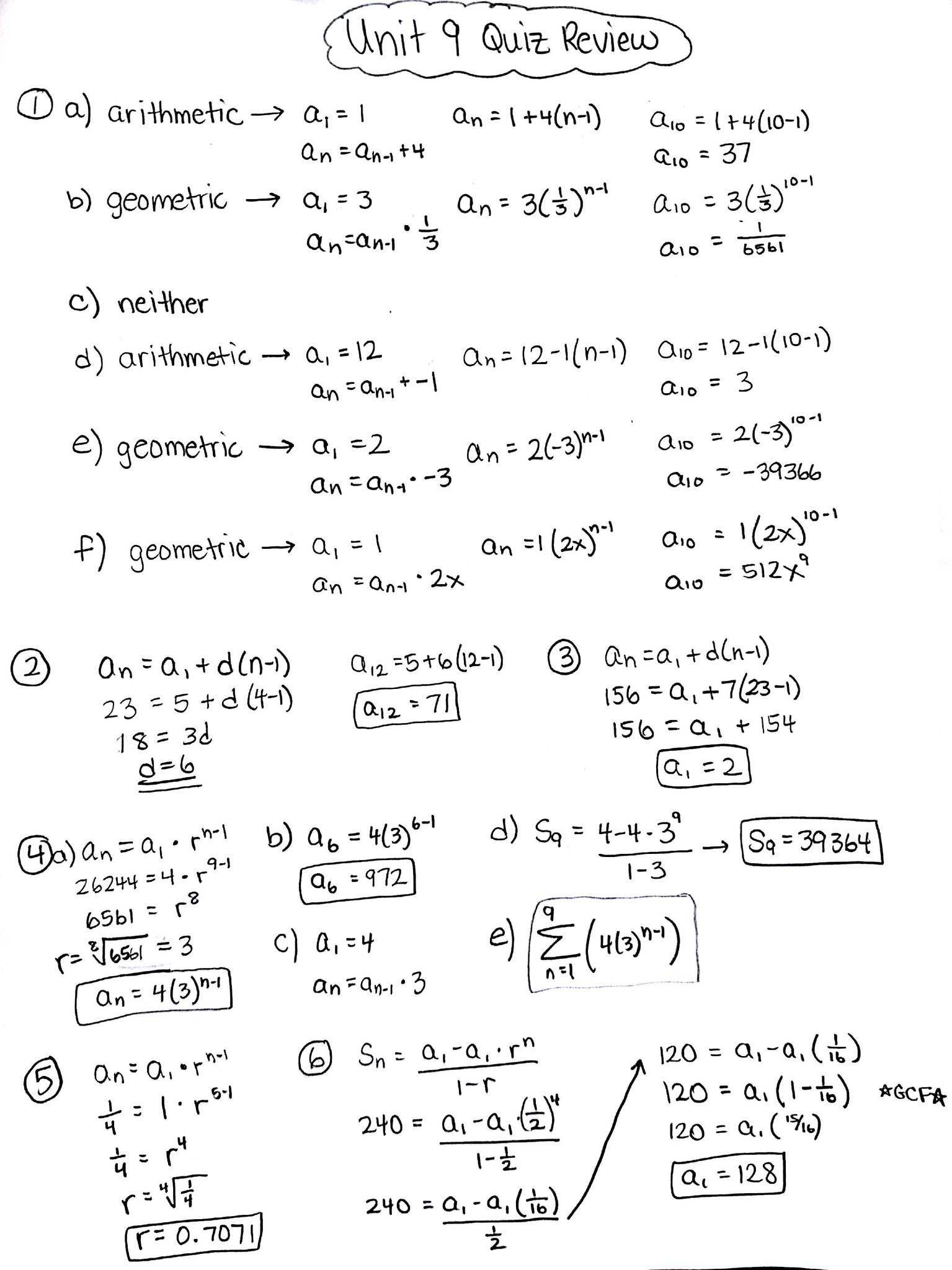
Working with expressions that involve variables raised to powers and fractions is a core skill in advanced mathematics. Polynomials and rational expressions are commonly tested topics that require understanding both basic operations and more complex problem-solving techniques. These concepts are often used to model and solve a wide range of mathematical scenarios.

Polynomials are expressions that involve sums of powers of a variable, while rational expressions are fractions where both the numerator and denominator are polynomials. Understanding how to manipulate these expressions is crucial for solving equations, simplifying expressions, and analyzing functions.

Key Concepts in Polynomials

• Adding and Subtracting Polynomials: Combine like terms by adding or subtracting the coefficients of terms with the same power.
• Multiplying Polynomials: Apply distributive properties to multiply each term in one polynomial by each term in the other.
• Factoring Polynomials: Break down complex polynomials into simpler factors using methods like factoring by grouping, difference of squares, or trinomial factoring.
• Finding Roots: Solve polynomial equations to find the values of the variable that make the polynomial equal to zero.

Working with Rational Expressions

• Simplifying Rational Expressions: Cancel common factors from the numerator and denominator to simplify the expression.
• Multiplying and Dividing Rational Expressions: Multiply or divide the numerators and denominators, making sure to simplify the result.
• Solving Equations Involving Rational Expressions: To solve these types of equations, find a common denominator and solve the resulting equation.
• Identifying Domain Restrictions: Determine values of the variable that would make the denominator zero, as these values are excluded from the domain.

Both polynomials and rational expressions require practice to fully understand their behavior and applications. By mastering these concepts, you’ll be well-prepared for handling a variety of problems that arise in advanced mathematics evaluations.

Preparing for Word Problems in Algebra

Word problems are a key component of many mathematical assessments. These types of questions present real-world scenarios and require you to translate them into mathematical expressions. Mastering the skills needed to solve word problems can greatly enhance your ability to tackle more complex questions efficiently and accurately.

To effectively approach word problems, it’s important to break down the information step by step and identify the key relationships between variables. By doing so, you can turn a seemingly complicated situation into a solvable equation. Practice is crucial, as it helps you recognize patterns and develop strategies for different types of word problems.

Steps for Solving Word Problems

• Read the Problem Carefully: Understand the context and identify what is being asked. Pay attention to units, quantities, and specific relationships mentioned in the problem.
• Define Variables: Assign a variable to each unknown quantity in the problem. This will help you organize the information mathematically.
• Translate Words into Math: Convert the verbal descriptions into equations or expressions. Look for keywords like “sum,” “difference,” or “product” that indicate mathematical operations.
• Set Up the Equation: Use the relationships between variables to form an equation that represents the problem.
• Solve the Equation: Use appropriate methods to solve the equation and find the value of the unknowns.
• Check Your Solution: After solving, review the problem to ensure the solution makes sense in the context of the question.

Common Types of Word Problems

• Rate Problems: These involve distance, time, and speed, where you use the formula distance = rate × time.
• Mixture Problems: Problems where two or more substances are mixed together, requiring you to set up equations based on their concentrations.
• Work Problems: These involve tasks completed by one or more people or machines, often requiring you to work with rates of work.
• Age Problems: These focus on relationships between the ages of different people, often involving algebraic expressions to represent their ages at different times.

By consistently practicing these types of word problems and following a systematic approach, you’ll become more confident and skilled in translating real-world situations into solvable mathematical equations.

Understanding Exponents and Radicals

Exponents and radicals are fundamental concepts that form the basis for a wide range of mathematical problems. These operations deal with powers and roots of numbers, and understanding them is crucial for simplifying expressions, solving equations, and analyzing functions. Whether you are working with integer exponents or dealing with square roots, mastering these concepts will improve your ability to approach and solve complex problems efficiently.

Exponents represent repeated multiplication, while radicals involve extracting roots of numbers. Both operations are often combined in various types of problems, requiring you to apply specific rules and properties to simplify and solve equations. A solid understanding of these concepts allows you to tackle a variety of mathematical challenges with confidence.

Key Concepts of Exponents

• Product Rule: When multiplying powers with the same base, add the exponents: a^m × a^n = a^(m + n).
• Quotient Rule: When dividing powers with the same base, subtract the exponents: a^m ÷ a^n = a^(m – n).
• Power of a Power: When raising a power to another power, multiply the exponents: (a^m)^n = a^(m × n).
• Negative Exponents: A negative exponent represents the reciprocal of the base raised to the positive exponent: a^(-n) = 1/a^n.
• Zero Exponent: Any non-zero number raised to the power of zero equals 1: a^0 = 1.

Understanding Radicals

• Square Roots: The square root of a number n is the value that, when multiplied by itself, gives n: √n.
• Cube Roots: The cube root of a number n is the value that, when multiplied by itself three times, equals n: ∛n.
• Rationalizing the Denominator: To simplify an expression with a radical in the denominator, multiply both the numerator and denominator by a suitable radical to eliminate the radical from the denominator.
• Multiplying Radicals: When multiplying two radicals with the same index, multiply the values inside the radicals: √a × √b = √(a × b).

By mastering the rules and properties of exponents and radicals, you can simplify complex expressions, solve equations more easily, and improve your overall problem-solving skills in advanced mathematics.

Complex Numbers and Their Applications

Complex numbers extend the concept of real numbers and are essential for solving equations that have no real solutions. These numbers consist of both a real and an imaginary part, and they are represented as a + bi, where a is the real part and b is the imaginary part. The introduction of complex numbers allows for the solution of problems in fields such as engineering, physics, and computer science, where real numbers alone cannot fully capture certain phenomena.

Understanding how to perform operations with complex numbers, such as addition, multiplication, and division, is essential for working with these quantities. Additionally, complex numbers can be represented graphically on the complex plane, which aids in visualizing their relationships and understanding their applications in various disciplines.

Operations with Complex Numbers

• Addition and Subtraction: To add or subtract complex numbers, simply add or subtract their real and imaginary parts separately. For example: (a + bi) + (c + di) = (a + c) + (b + d)i.
• Multiplication: To multiply complex numbers, apply the distributive property, remembering that i^2 = -1. For example: (a + bi) × (c + di) = (ac – bd) + (ad + bc)i.
• Division: To divide complex numbers, multiply both the numerator and denominator by the complex conjugate of the denominator. This will eliminate the imaginary part from the denominator and allow you to express the quotient in standard form.

Applications of Complex Numbers

• Electrical Engineering: In electrical engineering, complex numbers are used to represent impedance in AC circuits, as they combine both resistance and reactance into a single value.
• Quantum Mechanics: Complex numbers are used in quantum mechanics to describe wave functions, which represent the probability of finding a particle in a particular state.
• Control Systems: In control theory, complex numbers are used to analyze the stability of systems through the use of transfer functions and the analysis of poles and zeros.
• Signal Processing: Complex numbers are utilized in signal processing to manipulate and analyze signals, especially in Fourier transforms and in the representation of signals in the frequency domain.

Mastering complex numbers not only enhances mathematical understanding but also opens up numerous practical applications in various fields, providing powerful tools for solving real-world problems.

Tips for Time Management on Tests

Effective time management during assessments is crucial to ensure that you can complete all questions thoroughly without feeling rushed. The key to managing your time well is understanding the structure of the assessment and allocating your time wisely based on the difficulty and point value of each question. With the right approach, you can stay calm and focused while maximizing your performance.

One of the most important strategies is to pace yourself from the beginning. Start by quickly reviewing the entire assessment to gauge its length and complexity. This will help you create a mental plan for how to approach each section. Prioritize questions based on your strengths and the time they might require. This way, you can ensure that you tackle easier or more familiar problems first, leaving more time for challenging ones.

Key Time Management Strategies

• Read the Instructions Carefully: Begin by reading the instructions and questions carefully. Misunderstanding a question can cost valuable time during the exam.
• Allocate Time per Section: Set a specific amount of time for each section based on its difficulty and number of questions. Keep track of time to stay on schedule.
• Don’t Get Stuck on One Question: If you encounter a particularly difficult question, move on and return to it later if time allows. Don’t let one question take up too much of your time.
• Use Shortcuts and Estimations: For questions that don’t require exact answers, use estimation techniques to save time, especially for problems that involve large calculations.
• Check Your Work Efficiently: If you finish early, use the remaining time to review your answers. Focus on the most likely areas where mistakes could have occurred, rather than rechecking everything.

By following these strategies, you can improve your time management skills, reduce stress, and ensure that you approach each section of the assessment with a clear, focused mindset.

Common Mistakes to Avoid

During assessments, it’s easy to make errors that can cost valuable points. While some mistakes are due to lack of understanding, others stem from simple oversights or rushing through questions. Identifying common pitfalls and taking steps to avoid them can significantly improve your performance. By recognizing these frequent errors, you can approach each section with greater care and attention, ultimately ensuring more accurate results.

Whether it’s misinterpreting a question, making calculation mistakes, or not reviewing your work, it’s crucial to develop habits that prevent these errors. Awareness and preparation are key to minimizing mistakes and staying confident throughout the process.

Typical Mistakes to Watch For

• Misreading Questions: Carefully read each question to ensure you understand exactly what’s being asked. Skimming through questions or missing keywords can lead to incorrect interpretations and wrong answers.
• Skipping Steps in Calculations: When solving problems, it’s easy to skip steps or make quick assumptions. This often leads to errors. Always show your work and check each step for accuracy.
• Rushing Through the Exam: The pressure of time can cause you to rush, leading to careless mistakes. Stay calm and take a moment to think through each question before answering.
• Overlooking Negative Signs: Negative signs can be tricky, especially when working with equations. Always double-check that you’ve properly accounted for any negative numbers in your calculations.
• Not Managing Time Wisely: Poor time management can result in not completing the entire assessment or rushing through difficult sections. Be sure to pace yourself and prioritize accordingly.

How to Prevent These Mistakes

• Practice Regularly: The more you practice, the more familiar you’ll become with common types of problems, reducing the likelihood of making simple errors.
• Read Carefully: Take your time reading each question and look for keywords or phrases that help clarify what is required.
• Review Your Work: If time permits, always review your answers, especially for problems that involve complex calculations or multiple steps.
• Stay Calm: Staying calm under pressure can help prevent rushed mistakes. Remember to breathe and focus on the task at hand.

By being mindful of these common mistakes and employing strategies to avoid them, you’ll be better prepared to approach each question with precision and confidence.

Test Anxiety and How to Overcome It

Feeling anxious before an assessment is a common experience, and it can impact both performance and confidence. The pressure to succeed, the fear of failure, or simply the thought of being evaluated can cause stress that affects focus and clarity. However, understanding the root of this anxiety and learning how to manage it can significantly improve your ability to approach assessments with a clear mind and calm demeanor.

Test anxiety often stems from the fear of not performing well, which can cause physical symptoms like racing thoughts, increased heart rate, and difficulty concentrating. While some level of nervousness is natural, excessive anxiety can hinder your ability to think clearly and make sound decisions. By implementing certain strategies, you can reduce this stress and regain control over your mindset.

Techniques to Manage Anxiety

• Practice Relaxation Techniques: Deep breathing exercises, meditation, or visualization can help calm your nerves before and during the assessment. Practice these techniques regularly to make them more effective when you need them most.
• Prepare Thoroughly: Adequate preparation can reduce the uncertainty that often triggers anxiety. By practicing regularly and reviewing key concepts, you can increase your confidence and feel more prepared to face any challenges.
• Shift Focus Away from Perfection: Instead of obsessing over getting every answer right, focus on doing your best and learning from the experience. This mindset can ease the pressure and help you approach the situation more calmly.
• Arrive Early: Arriving early for the assessment allows you to settle in, get comfortable, and reduce feelings of rush or panic. Use this time to calm your mind and mentally prepare for the task ahead.
• Positive Self-Talk: Replace negative thoughts with affirmations that remind you of your preparation and capabilities. Positive reinforcement can help shift your mindset and reduce feelings of anxiety.

Maintaining a Calm Mindset During the Assessment

• Take Breaks When Needed: If you start feeling overwhelmed, take a brief pause. Close your eyes, breathe deeply, and refocus your thoughts. A short break can help reset your mind.
• Focus on the Present: Rather than worrying about how many questions are left or what might happen, focus on the question in front of you. Take each step as it comes, which will help prevent overwhelming thoughts.
• Trust Your Preparation: Trust in the effort you’ve put into your preparation. Acknowledge that you have prepared as best as you could and that you are capable of succeeding.

By implementing these strategies, you can significantly reduce test-related stress, improving both your performance and overall experience. With practice, you will find yourself approaching assessments with greater confidence and less anxiety.

Using Online Resources for Practice

In today’s digital age, the internet offers a wealth of resources that can help improve your skills and prepare for any challenge. Online platforms provide interactive exercises, tutorials, and practice problems, making it easier to access learning materials anytime and anywhere. Utilizing these tools can be an effective way to reinforce what you’ve learned and track your progress.

Whether it’s video lessons, quizzes, or forums where you can discuss problems with others, online resources cater to different learning styles. With the convenience of instant feedback, you can identify areas where you need more practice and focus your efforts accordingly. The ability to simulate real conditions and practice under timed scenarios also helps build confidence and readiness.

Types of Online Resources

• Interactive Quizzes: Websites that offer practice problems with instant feedback can help reinforce your understanding. These quizzes often cover a wide range of topics, allowing you to focus on specific areas where you need improvement.
• Video Tutorials: Video platforms like YouTube provide detailed explanations and step-by-step solutions to various problems. These resources can be particularly useful if you prefer visual learning or need a more in-depth explanation.
• Online Study Groups: Joining online forums or study groups allows you to collaborate with others, share insights, and solve problems together. This social learning environment can enhance your understanding and provide motivation.
• Mobile Apps: Several mobile apps are designed to help you practice on the go. These apps often feature flashcards, practice exercises, and progress tracking, making it easier to study during short breaks or downtime.

How to Maximize Online Resources

• Set Clear Goals: Before starting your practice, define what you want to achieve. Whether it’s mastering a specific topic or improving your speed, having a clear goal will keep you focused.
• Practice Regularly: Consistency is key. Use online resources to practice every day or several times a week to build muscle memory and retain information.
• Track Your Progress: Many online platforms offer progress trackers or scoreboards. Keep an eye on your results to identify strengths and weaknesses, and adjust your study plan accordingly.
• Challenge Yourself: Don’t just stick to easy questions. Gradually increase the difficulty level to ensure you’re being challenged and pushing your limits.

By effectively utilizing the many online resources available, you can enhance your learning experience, gain a deeper understanding of complex concepts, and improve your overall performance.