Algebra 1 Review Packet Answers and Solutions
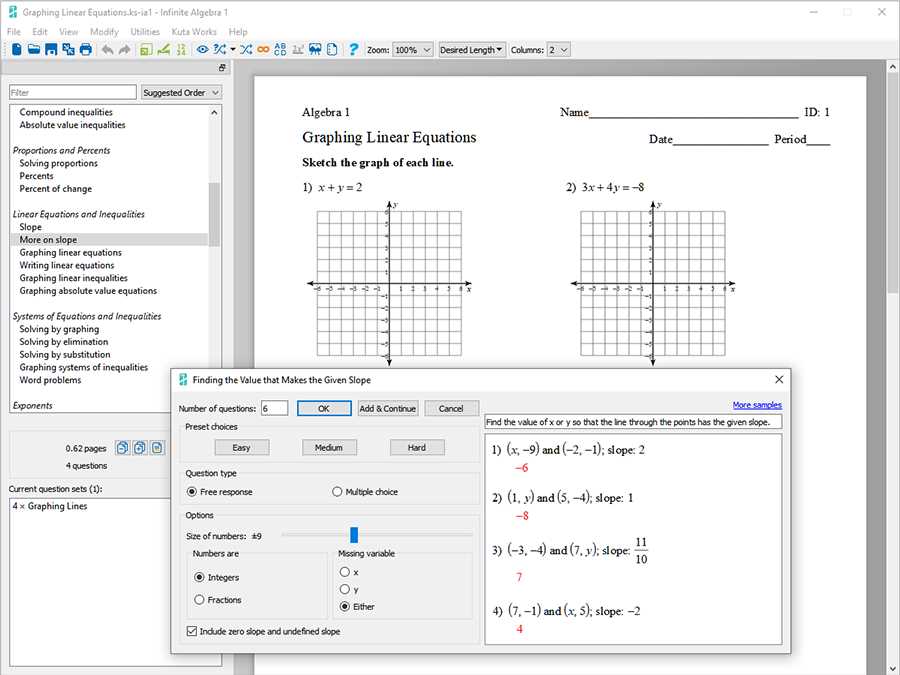
When tackling mathematical exercises, a structured approach can make all the difference. Whether you’re revisiting basic principles or refining your skills, focusing on the key concepts is crucial for success. This section is designed to help you better understand the steps involved in solving various types of equations and problems.
Understanding the underlying principles is the first step to mastering any set of challenges. By breaking down each problem into manageable parts, you’ll gain confidence in approaching more complex tasks. This guide will take you through a series of solutions that demonstrate essential techniques and strategies.
By practicing regularly, you’ll not only improve your problem-solving ability but also deepen your understanding of the fundamental operations. Through clear explanations and examples, this section will guide you to a higher level of proficiency, enabling you to tackle similar tasks with ease and precision.
Algebra 1 Review Packet Answers Overview
In any study session, understanding the core principles is the foundation for tackling mathematical challenges. This section highlights the fundamental steps and strategies needed to solve various equations effectively. By examining the essential processes, you’ll gain insight into the best approaches for solving problems and enhance your ability to find solutions independently.
Each example provided demonstrates a different method of solving problems, showcasing the diversity of techniques that can be applied. Focusing on both simple and more intricate tasks, the explanations aim to clarify the steps involved and help you develop a clear understanding of the material.
With practice, you’ll become more proficient in recognizing patterns and identifying the best strategies to apply in different situations. This section serves as a guide to improve your problem-solving skills, enabling you to approach mathematical exercises with confidence and clarity.
Understanding Key Algebra Concepts
To effectively solve mathematical equations, it is essential to grasp the foundational ideas that guide the process. Mastering these core principles allows for better comprehension and more accurate problem-solving. By focusing on the most important concepts, you’ll build a strong foundation that makes tackling increasingly complex problems much easier.
Fundamental operations such as addition, subtraction, multiplication, and division form the backbone of many tasks. Understanding how to manipulate numbers and variables is crucial to progressing through various types of equations. Additionally, the concept of balancing equations ensures that both sides of an expression are equal, an idea that plays a key role in many problem-solving strategies.
Beyond basic operations, recognizing the relationships between different components, such as terms, coefficients, and constants, is vital for deeper understanding. These building blocks help you decipher how equations are structured and how to apply the right techniques to simplify or solve them. Developing a strong grasp of these essential ideas will prepare you for more advanced challenges ahead.
How to Approach Algebra 1 Problems
When tackling mathematical exercises, having a structured approach is essential for efficient problem-solving. Each problem may look different at first glance, but with the right strategy, most tasks can be broken down into manageable steps. This method ensures that you don’t overlook important details and helps you stay organized throughout the process.
Step-by-Step Process
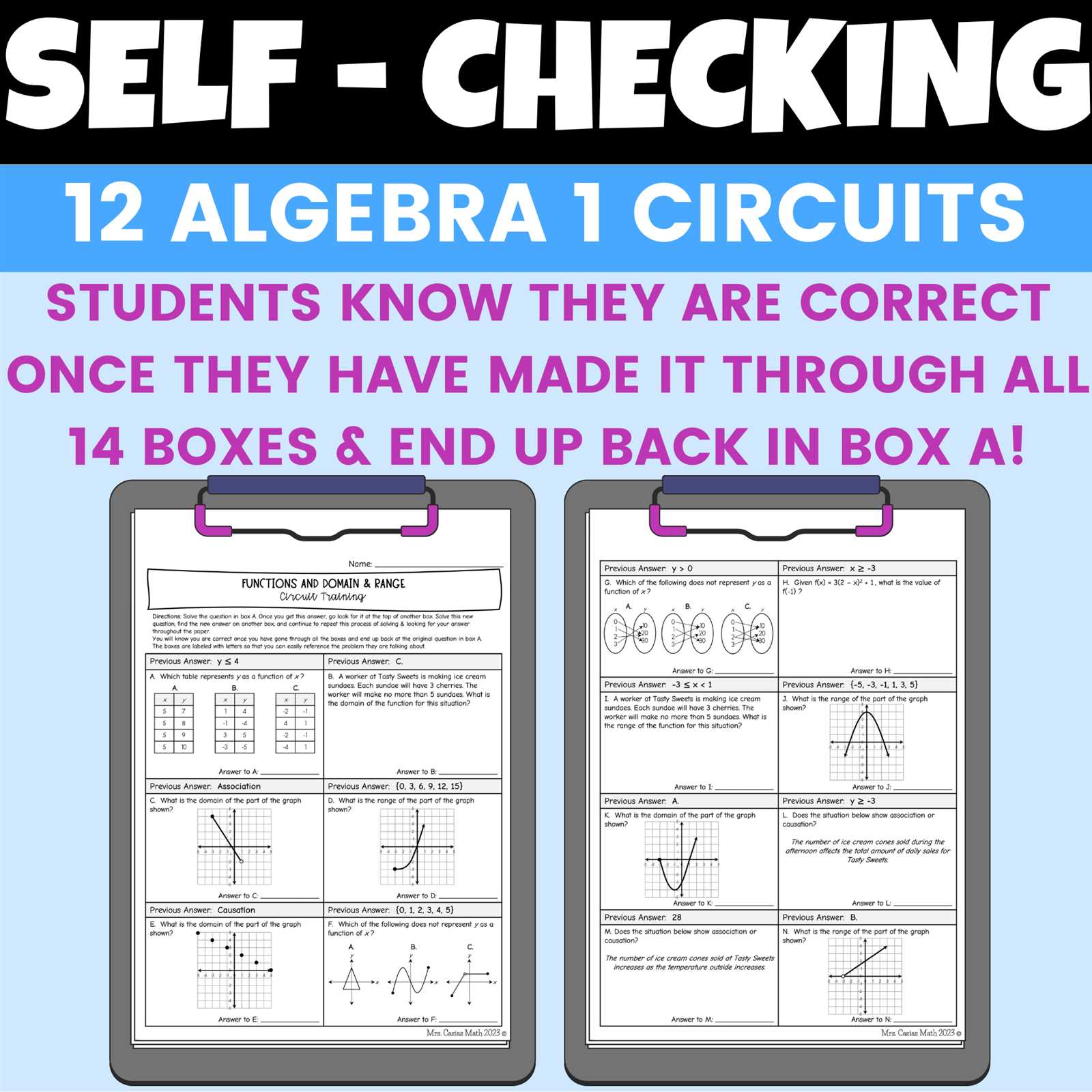
- Read the problem carefully: Make sure you understand the question before proceeding. Identify key information such as numbers, operations, and any specific instructions.
- Identify what is being asked: Determine what you need to find or solve for in the problem.
- Choose the appropriate method: Decide on the best technique based on the problem type, whether it involves solving for a variable, simplifying an expression, or working with equations.
- Perform calculations: Follow through with the necessary steps, ensuring accuracy at each stage.
- Check your work: After solving, review each step to confirm the solution is correct and makes sense in the context of the problem.
Common Strategies for Success
- Practice regularly: The more you practice, the more familiar you will become with different problem types and solution methods.
- Work backward: If you’re stuck, try solving the problem from the solution point backward to identify any missteps.
- Use visual aids: Diagrams, charts, or even number lines can provide clarity and help visualize relationships between variables.
- Stay organized: Writing out each step clearly can prevent confusion and make it easier to identify mistakes.
By following these steps and strategies, you’ll be better prepared to handle a variety of mathematical problems with confidence and accuracy. Consistent practice will make these steps feel natural and more intuitive over time.
Common Mistakes in Algebra 1 Review
As with any subject, there are frequent pitfalls students encounter when solving mathematical problems. Understanding these common errors is key to improving your accuracy and efficiency. Many mistakes stem from misunderstandings of basic principles or from rushing through problems without checking the work carefully.
Frequent Errors to Watch Out For
Below are some of the most common mistakes that can occur when solving equations or simplifying expressions. Recognizing these errors will help you avoid them and improve your problem-solving skills.
| Mistake | Description | How to Avoid |
|---|---|---|
| Misapplying Order of Operations | Forgetting to follow the correct sequence of operations (PEMDAS) can lead to incorrect solutions. | Always work from parentheses to exponents, multiplication/division, and then addition/subtraction. |
| Sign Errors | Incorrectly handling negative signs or failing to distribute negative numbers properly can distort results. | Be careful with signs when multiplying or dividing terms, especially with negative values. |
| Incorrectly Combining Like Terms | Failing to group and simplify like terms correctly often results in incorrect answers. | Ensure you only combine terms with the same variables and exponents. |
| Skipping Steps | Jumping to conclusions without showing intermediary steps can lead to mistakes being overlooked. | Write out every step in the process, even if it seems obvious. |
| Forgetting to Check Solutions | Not double-checking the final result can leave errors undetected. | Always substitute the solution back into the original equation to verify it works. |
How to Overcome These Challenges

To avoid these mistakes, practice is key. Pay close attention to detail, and don’t hesitate to double-check each step before moving forward. With time, these common errors will become easier to spot and avoid, ultimately strengthening your problem-solving abilities.
Step-by-Step Solutions for Algebra 1
Breaking down problems into clear, manageable steps is the most effective way to reach the correct solution. By following a systematic approach, you can simplify complex tasks and ensure accuracy in every calculation. This section will guide you through several examples, showing you how to approach each one step by step.
In the following table, you will see the breakdown of different types of problems, demonstrating how to solve them efficiently and correctly.
| Step | Action | Explanation |
|---|---|---|
| Step 1 | Read the problem carefully | Understand the variables, constants, and what is being asked. Identifying key information is crucial to solving the problem correctly. |
| Step 2 | Simplify the equation | If possible, combine like terms and simplify both sides of the equation to make it easier to solve. |
| Step 3 | Isolate the variable | Rearrange the equation to get the variable on one side by itself, using addition, subtraction, multiplication, or division as needed. |
| Step 4 | Perform operations | Carry out the necessary calculations, making sure to follow the correct order of operations (PEMDAS) for accuracy. |
| Step 5 | Check the solution | Substitute the solution back into the original equation to verify that it satisfies the equation. |
By following this step-by-step process, you can methodically approach each problem and arrive at the correct solution. With regular practice, these steps will become second nature, making it easier to handle even the most complex equations.
Helpful Tips for Algebra 1 Success
Success in mathematics is not just about memorizing formulas or solving equations; it’s about understanding the underlying principles and applying them effectively. With the right strategies and consistent practice, anyone can improve their skills and excel in mathematical tasks. This section offers valuable advice to guide you towards mastering the material and achieving your best results.
- Practice regularly: The more problems you solve, the more familiar you become with different types of exercises. Consistency is key to reinforcing what you’ve learned.
- Understand the concepts: Rather than memorizing steps, focus on grasping the reasoning behind them. When you understand why certain methods work, you can apply them to new problems more effectively.
- Stay organized: Keeping your work neat and structured helps you follow each step more easily. Write out each calculation and check your work to avoid skipping important details.
- Break down complex problems: Large tasks can be overwhelming. Break them into smaller, more manageable parts to simplify the process and make the problem easier to solve.
- Ask for help: Don’t hesitate to ask for clarification if you’re struggling with a concept. Collaborating with others or seeking additional resources can provide a fresh perspective and aid your understanding.
- Review mistakes: Don’t just focus on correct answers. Analyzing your errors helps identify gaps in understanding and improves your ability to avoid similar mistakes in the future.
By incorporating these tips into your study routine, you’ll build a stronger foundation, enhance your problem-solving skills, and boost your confidence in tackling mathematical challenges.
Breaking Down Complex Algebraic Equations
Solving complicated mathematical expressions can seem daunting at first, but with a methodical approach, even the most challenging problems can be simplified. The key to handling intricate equations lies in breaking them down into smaller, more manageable parts. This allows you to focus on one step at a time, reducing the risk of mistakes and improving your overall understanding.
Step 1: Identify Components
Start by carefully identifying all parts of the equation. Look for terms, constants, and variables. Recognizing how these components interact is essential for determining the correct approach. For example, when dealing with terms that have different exponents, you need to address them differently than terms with the same base.
Step 2: Simplify the Equation
Combine like terms where possible, and eliminate unnecessary complexity. This might involve removing parentheses or distributing factors across terms. Each simplification step should bring the equation closer to a form where the unknown variable can be isolated.
Once the equation is simplified, you can begin solving for the variable. Whether you’re working with addition, subtraction, multiplication, or division, apply the correct operations systematically. Remember to perform inverse operations carefully to avoid errors.
Checking your work after every significant step helps ensure that you’re on the right track and provides an opportunity to catch mistakes early on. With regular practice and attention to detail, solving complex expressions becomes a more intuitive and manageable process.
Why Practice is Essential in Algebra
Mastering any mathematical skill requires consistent practice, and this subject is no different. Repeatedly working through problems helps reinforce concepts, making them easier to recall and apply when needed. The more you practice, the more you familiarize yourself with various types of problems and their solutions, building confidence and proficiency over time.
Benefits of Regular Practice
- Improves Problem-Solving Speed: Frequent practice enables you to recognize patterns and shortcuts, speeding up your ability to solve problems efficiently.
- Builds Stronger Understanding: When you solve similar problems repeatedly, you develop a deeper understanding of the methods and principles behind them.
- Helps Retain Information: Regular engagement with the material ensures that you retain important formulas and methods for the long term.
- Boosts Confidence: The more problems you solve successfully, the more confident you become in your abilities, leading to less anxiety in exams or assignments.
- Reinforces Learning: Practice allows you to apply theoretical knowledge to practical situations, reinforcing what you’ve learned in a hands-on way.
How to Incorporate Practice Effectively
- Start with Basics: Begin with simple problems to build your foundation before moving on to more complex tasks.
- Challenge Yourself: Once comfortable with basic problems, increase the difficulty level gradually to push your limits and expand your knowledge.
- Practice Regularly: Set aside dedicated time each day or week to solve problems, ensuring continuous improvement.
- Review Mistakes: Analyze errors to understand where things went wrong and learn from them to avoid repeating the same mistakes.
Incorporating consistent practice into your study routine is crucial for improving mathematical abilities and gaining mastery. As you continue to practice, the material will become more intuitive, and you will be better prepared to tackle more challenging problems with confidence.
Real-World Applications of Algebra 1
The principles learned in this mathematical field are not confined to the classroom. They are essential in solving everyday problems and are used in a variety of fields. From personal finance to engineering, the skills gained here help us make informed decisions and understand the world around us more clearly. This section explores some of the ways these concepts are applied in real-life situations.
- Finance and Budgeting: Whether calculating interest rates, managing expenses, or planning savings, mathematical operations such as solving equations are vital in personal and business finances.
- Construction and Architecture: Professionals in these fields use mathematical concepts to determine measurements, dimensions, and structural integrity. Without the application of formulas and problem-solving techniques, building projects would not be possible.
- Computer Science and Coding: Programmers rely on mathematical logic to write code, solve algorithmic problems, and optimize systems. Understanding variables and equations is a foundational skill for anyone in the tech industry.
- Medicine and Health: From calculating medication dosages to interpreting statistical data on health trends, these skills are necessary for professionals to ensure accuracy and safety in medical settings.
- Economics: Economists use equations to analyze trends, model economic systems, and forecast future market behavior. Understanding how variables interact in different economic scenarios helps make informed decisions at both the micro and macroeconomic levels.
Understanding how these concepts are used in the real world provides context and relevance, showing that the skills developed through solving mathematical problems are crucial in many aspects of modern life. By recognizing their importance, students are more likely to stay engaged and see the value of their education in solving practical challenges.
Using Algebra 1 Review Packets Effectively
Using practice materials strategically can significantly improve your understanding of mathematical concepts and enhance problem-solving skills. When working with review exercises, it is essential to approach them with a clear plan in order to maximize learning and retention. By following a structured method and focusing on key areas, you can get the most out of each practice session.
- Start with the basics: Begin with simpler exercises to build confidence before moving on to more challenging problems. This helps solidify foundational concepts and ensures a smoother learning curve.
- Work in intervals: Break your practice into focused sessions. Use short, timed intervals to maintain concentration and avoid mental fatigue. For example, work for 25 minutes and then take a short break.
- Analyze mistakes: After solving each problem, thoroughly review your mistakes. Understanding where you went wrong is crucial for improving and preventing similar errors in the future.
- Use a step-by-step approach: Follow each problem-solving process carefully, taking note of every step. This ensures that you don’t skip over important details and helps you apply consistent methods to new challenges.
- Track your progress: Keep a record of the problems you’ve solved and the areas you’ve struggled with. Monitoring your improvement allows you to identify patterns and focus on specific topics that need more attention.
By incorporating these strategies into your study routine, you can transform practice exercises into an effective tool for mastering mathematical concepts. Staying organized, reviewing mistakes, and working in focused intervals will help reinforce your knowledge and prepare you for more complex tasks.
Test Prep Strategies for Algebra 1
Preparing for a test in this subject requires more than just reviewing material. It involves using effective strategies that help retain information and improve problem-solving skills under pressure. By employing structured study techniques, you can ensure you are well-prepared for any assessment and can approach problems with confidence.
Effective Study Habits
- Review Key Concepts: Focus on understanding the main principles and operations. Make sure you have a solid grasp of core topics, such as equations, graphing, and solving inequalities, as these form the basis for more complex problems.
- Practice Under Time Pressure: Simulate test conditions by solving problems within a set time limit. This helps improve speed and efficiency, which are critical during exams.
- Summarize Your Notes: Create condensed versions of your notes, highlighting the most important formulas, rules, and problem-solving methods. This makes reviewing quicker and ensures you focus on what matters most.
- Use Practice Tests: Take advantage of sample exams or past test papers to familiarize yourself with the format and identify any weak areas that need extra attention.
Managing Test Anxiety

- Stay Organized: Plan your study sessions ahead of time and break the material into manageable chunks. Avoid cramming the night before by spreading out your study sessions over a longer period.
- Stay Calm and Focused: Test anxiety can hinder performance. Practice relaxation techniques such as deep breathing to stay calm during the test and maintain focus on the task at hand.
- Get Enough Rest: Ensure you are well-rested before the test day. A good night’s sleep is essential for mental clarity and focus during the exam.
By combining solid study strategies with effective time management and stress-reduction techniques, you will be better equipped to tackle any test confidently. Consistent practice and a focused approach will not only prepare you for exams but also deepen your understanding of the material.
Common Algebra 1 Question Formats
In mathematics, it’s important to be familiar with the various formats in which problems can be presented. Understanding the common types of questions will help you approach them more efficiently and effectively. Whether it’s solving for variables, graphing equations, or working with expressions, each question type requires a unique strategy. This section outlines the most frequently encountered formats and offers tips on how to handle each one.
Solving Equations
- Linear Equations: These problems typically involve solving for an unknown variable by isolating it on one side of the equation. You may be required to perform operations such as addition, subtraction, multiplication, or division to find the value of the variable.
- Systems of Equations: In this type of question, you are given two or more equations and asked to find the values of multiple variables simultaneously. Methods like substitution or elimination are often used to solve these problems.
- Inequalities: These questions require solving for a variable within an inequality and interpreting the solution graphically on a number line. Pay attention to signs and make sure to reverse the inequality when multiplying or dividing by a negative number.
Graphing and Visual Interpretation

- Linear Graphs: These questions ask you to graph equations or interpret graphs. Understanding the slope-intercept form and being able to plot points on a coordinate plane are key skills here.
- Quadratic Functions: Some questions may involve graphing quadratic functions or interpreting their key features, such as the vertex, axis of symmetry, and direction of the parabola. Familiarity with standard and vertex forms is crucial for solving these problems.
- Word Problems: These questions involve translating real-world situations into mathematical equations or functions. Focus on identifying the variables, setting up the equation correctly, and using logical reasoning to find the solution.
Recognizing these formats allows you to develop a strategy tailored to each type of problem. Whether you need to solve for a variable, interpret a graph, or apply a formula, knowing the format will enable you to approach the task with confidence and accuracy.
Mastering Algebraic Expressions
Understanding and manipulating expressions is a core skill in mathematics. These foundational tools help in forming equations and solving problems in various contexts. Mastery of expressions involves recognizing their components, simplifying them, and being able to work with them in different problem-solving scenarios. Whether it’s combining like terms or applying the distributive property, becoming proficient in handling expressions is essential for progressing in more advanced topics.
Key Concepts in Simplifying Expressions

- Combining Like Terms: Identifying and merging terms that have the same variable and exponent is one of the first steps in simplifying expressions. This reduces the expression to its simplest form.
- Distributive Property: The distributive property allows you to multiply a term outside the parentheses by each term inside the parentheses, ensuring no term is left out during simplification.
- Factoring Expressions: Factoring involves rewriting an expression as a product of its factors. This can help in solving equations or simplifying complex expressions.
Techniques for Working with Complex Expressions
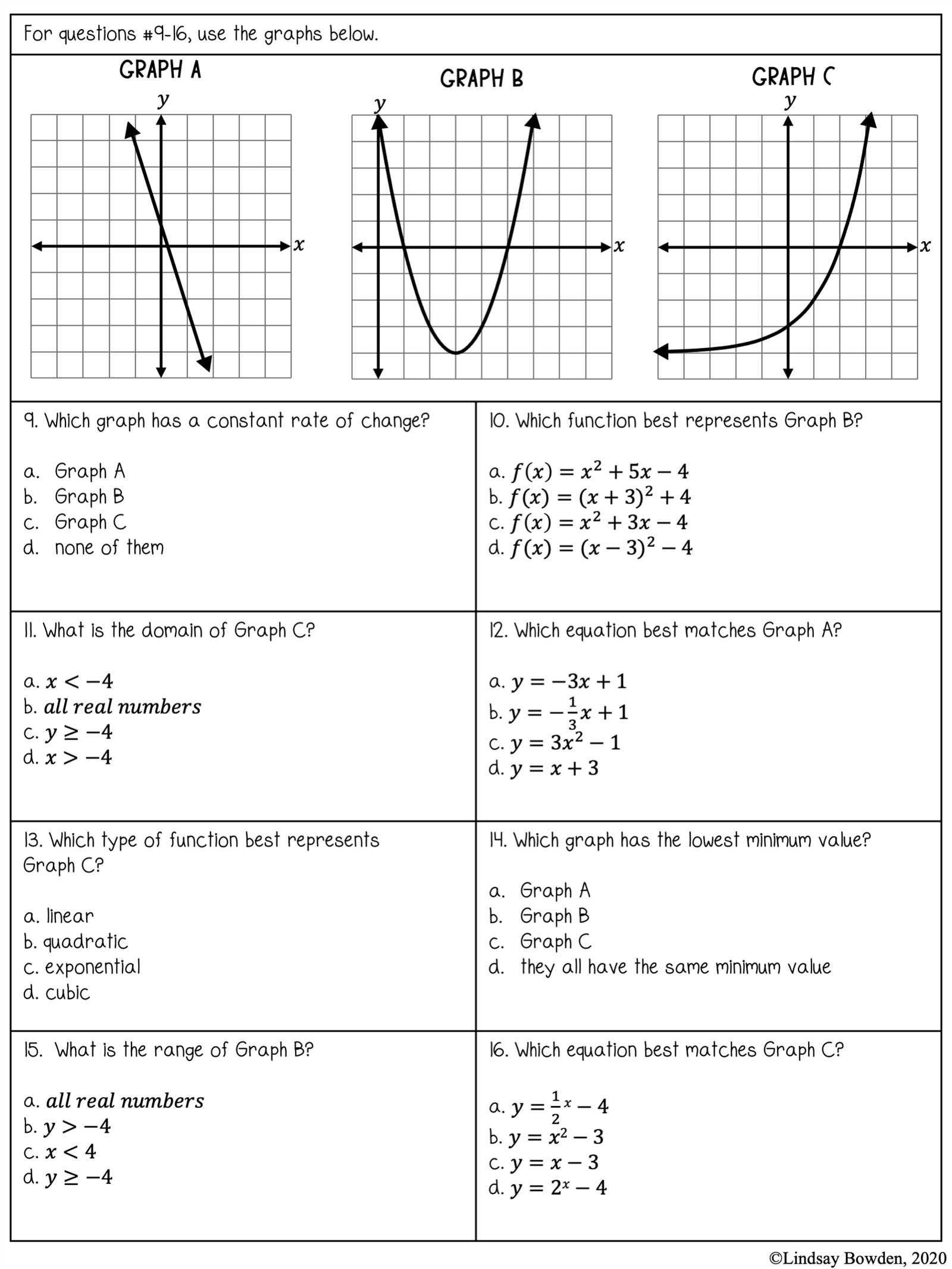
- Handling Fractions: When dealing with expressions that contain fractions, remember to find a common denominator, multiply across the numerator and denominator, and simplify as needed.
- Using Exponents: Understanding the rules for exponents–such as the product rule, quotient rule, and power rule–is key to simplifying and manipulating expressions with powers and roots.
- Substitution: Substituting known values into an expression is a common technique for solving problems and evaluating the value of an expression for different inputs.
With consistent practice, mastering these skills will enable you to tackle increasingly complex problems with confidence. Simplifying, factoring, and solving expressions are all crucial techniques that form the foundation for solving equations and understanding mathematical relationships.
Solving Linear Equations with Ease
Simplifying and solving linear equations is a fundamental skill in mathematics. These problems are often the building blocks for more advanced topics and require a clear understanding of how to isolate variables. By following a series of logical steps, solving these equations becomes a straightforward task, providing a pathway to deeper mathematical concepts. The key is to balance both sides of the equation while maintaining the integrity of the equality.
To solve these problems, one must focus on performing operations that simplify the equation step by step. This includes adding, subtracting, multiplying, or dividing both sides by the same number, keeping the equation balanced at all times. A strong grasp of these operations allows for quicker solutions and helps in recognizing patterns in equations.
Step-by-Step Process for Solving Equations
- Isolate the Variable: Begin by moving all terms involving the variable to one side of the equation and constant terms to the other side. This helps simplify the problem.
- Simplify Both Sides: Combine like terms on both sides of the equation to reduce the complexity of the expression.
- Perform Operations: Use appropriate operations (addition, subtraction, multiplication, or division) to isolate the variable completely. Be sure to apply the same operation to both sides of the equation.
Common Mistakes to Avoid
- Sign Errors: Always double-check signs when performing operations, especially when multiplying or dividing negative numbers.
- Incorrect Distribution: Be careful when distributing numbers across parentheses to ensure accuracy in your steps.
- Skipping Steps: Skipping over intermediate steps may lead to mistakes. It’s important to perform each operation carefully and methodically.
With practice, solving these equations will become more intuitive. The key is to stay organized, perform operations consistently, and double-check each step to avoid common errors. By mastering the process of solving linear equations, you will develop a strong foundation for tackling more complex mathematical challenges.
Understanding Algebraic Word Problems
Word problems often present mathematical challenges in the form of real-life scenarios, requiring the translation of written descriptions into mathematical equations. These problems can seem intimidating at first, but with the right approach, they become manageable. The key is to break down the problem step by step and identify the relationships between the different elements mentioned in the text.
To successfully tackle these problems, the first step is to carefully read and understand the context. Look for key pieces of information that define the variables and constants in the scenario. Once you identify the unknowns, you can begin formulating an equation that models the situation described. From there, applying the appropriate mathematical operations will help you find the solution.
Steps for Solving Word Problems
- Read Carefully: Begin by reading the problem thoroughly to understand the context and identify the relevant information.
- Define Variables: Assign a variable to represent the unknown quantity or quantities in the problem.
- Translate the Words into an Equation: Convert the relationships described in the problem into a mathematical expression.
- Solve the Equation: Use the appropriate mathematical techniques to solve for the unknown.
- Check Your Answer: After solving, verify that the answer makes sense in the context of the problem.
Common Challenges in Word Problems
- Misinterpreting the Question: Ensure that you fully understand what the problem is asking before starting to solve it. Rushing through the reading can lead to mistakes.
- Setting Up the Equation: It’s crucial to translate the words into an accurate equation. Watch for keywords that indicate specific operations like addition, subtraction, multiplication, or division.
- Overcomplicating the Problem: Sometimes, it’s easy to overthink the problem. Stay focused on the key details and avoid adding unnecessary steps.
By practicing these steps and learning to identify the underlying structure of word problems, you can develop a strong ability to approach and solve them efficiently. This skill is essential, as word problems often appear in various mathematical contexts, from basic calculations to more advanced scenarios.
Working Through Algebraic Functions
Understanding and solving algebraic functions is a crucial skill in mathematics. These functions represent relationships between variables, and being able to manipulate them correctly opens the door to solving a wide range of problems. The process typically involves evaluating the function for given values, understanding the rules of operations, and simplifying expressions to find the desired outcome.
To work through these types of functions, it’s important to identify the structure of the expression first. Once the function is clearly understood, substituting values for the variables allows you to calculate the result. The key is mastering the operations that define how these functions behave, such as addition, subtraction, multiplication, and division.
Key Steps to Solve Algebraic Functions
- Identify the Function: Start by recognizing the form of the equation or expression, such as linear, quadratic, or exponential functions.
- Substitute Values: Replace the variables with known values and perform the necessary operations to solve for the unknown.
- Simplify the Expression: Use algebraic rules to simplify the function, combining like terms and performing any operations required.
- Check Your Work: After solving, it’s always a good practice to substitute your answer back into the original function to ensure consistency.
Common Mistakes in Working with Functions
- Incorrect Substitution: Always double-check that the values you substitute are correct and that you’re using the right variable.
- Order of Operations: Ensure that you follow the correct order of operations (PEMDAS) to avoid errors in solving functions.
- Neglecting to Simplify: After performing calculations, always look for opportunities to simplify the result before concluding the problem.
By practicing the steps outlined and staying mindful of common mistakes, working with algebraic functions becomes a much more manageable task. The key to mastery is repetition and a thorough understanding of the principles that govern these mathematical relationships.
How Algebra 1 Builds Math Skills
Mastering foundational concepts in mathematics plays a significant role in developing strong problem-solving abilities. As students progress through different mathematical topics, they are introduced to strategies that enhance their analytical thinking and improve their ability to tackle increasingly complex challenges. These skills form the core of many advanced mathematical areas and real-world applications.
In this stage of learning, students refine their ability to work with variables, solve equations, and understand relationships between numbers. The logical thinking required to manipulate equations fosters precision and encourages a methodical approach to solving problems. This process also emphasizes pattern recognition, critical for higher-level math and many other fields of study.
Key Skills Developed

| Skill | Description |
|---|---|
| Problem-Solving | Students learn how to break down complex problems into manageable parts, finding solutions step by step. |
| Logical Thinking | Working with equations and variables teaches students to think logically and identify patterns. |
| Critical Reasoning | Solving for unknowns encourages critical reasoning, allowing students to approach problems from multiple angles. |
| Abstract Thinking | Understanding how numbers and variables interact in abstract terms sharpens mental flexibility and creativity. |
The mathematical practices acquired in this area extend beyond just solving equations. These skills can be applied in various situations, from budgeting and financial planning to engineering and scientific research. As students build on these foundational skills, they prepare themselves for success in more advanced mathematical topics and a broad range of career opportunities. The discipline learned here provides a framework for thinking logically and strategically in other areas of life as well.
Preparing for Algebra 1 Exams

Successfully preparing for math exams requires more than just reviewing past lessons. It involves a comprehensive approach that includes practicing problem-solving techniques, understanding core concepts, and developing effective strategies for tackling different types of questions. The goal is not only to recall information but to apply it efficiently under test conditions.
To achieve this, students should focus on building a solid foundation in the key topics covered during the course. Regular practice helps to reinforce learned material and uncover any areas that need further attention. Additionally, test-taking strategies, such as managing time and understanding question formats, play a crucial role in exam performance.
Effective Preparation Tips
- Start Early: Begin your study sessions well in advance to avoid cramming. This allows you to cover all topics and reduces stress closer to the exam.
- Identify Weak Areas: Focus on concepts that you find challenging. Reviewing these topics in detail helps ensure a thorough understanding.
- Practice Regularly: Solve a variety of problems regularly. Practice helps you become more comfortable with different types of questions.
- Use Study Guides: Utilize study materials that summarize key concepts and formulas. These can serve as helpful reference tools when preparing.
- Take Mock Exams: Simulate test conditions by taking practice exams. This helps you become familiar with the exam format and manage time effectively.
Test-Taking Strategies
- Read Questions Carefully: Pay attention to the details in each question. Misreading can lead to unnecessary mistakes.
- Work on Easy Questions First: Quickly answer the questions you find easiest. This boosts confidence and ensures that you secure those points before moving to more difficult ones.
- Show Your Work: Writing down your steps can help you avoid mistakes and make it easier to spot errors if your final answer doesn’t match expectations.
- Stay Calm and Focused: Keep a positive mindset and avoid getting bogged down by challenging questions. Take deep breaths and move on if you get stuck.
By incorporating these preparation and test-taking strategies into your study routine, you’ll be better equipped to perform confidently and effectively during your exams. The key is consistent practice, a deep understanding of the material, and an organized approach to managing both study time and test time.