Algebra 2 Final Exam Review Answers

Preparing for an important assessment in mathematics can be challenging, but with the right focus and approach, success is within reach. This section will help you navigate through essential topics, ensuring you understand the material thoroughly and can apply it effectively.

From solving complex equations to understanding advanced functions, it’s crucial to break down each topic into manageable parts. Whether you’re revisiting core principles or tackling more advanced concepts, structured practice and problem-solving techniques will support your learning process.

Strengthening problem-solving skills and developing a deep understanding of key mathematical methods are vital for performing well. By focusing on the areas that require the most attention, you can enhance your preparedness and gain confidence in tackling a variety of questions.

Mathematical Concepts for Assessment Success

Mastering key mathematical principles is crucial for approaching a comprehensive test. The process involves applying various strategies to simplify complex problems and ensuring each concept is well understood. By practicing with focused exercises, you can tackle a wide range of challenges with confidence.

Critical Areas to Focus On

Identifying the areas that require the most attention is essential for efficient preparation. Some of the core topics include solving equations, graphing functions, and working with polynomials. Having a solid grasp of these concepts allows you to confidently approach any related questions during the assessment.

Problem-Solving Techniques

Practice is key when it comes to honing your skills. By solving problems in a step-by-step manner, you can break down each task and apply the appropriate methods. Understanding how to manage time and prioritize difficult questions can also enhance performance during the test.

Additionally, incorporating different types of problems–ranging from simple to complex–will ensure that you’re prepared for any scenario. Regular practice with these techniques strengthens both your problem-solving ability and your understanding of the material.

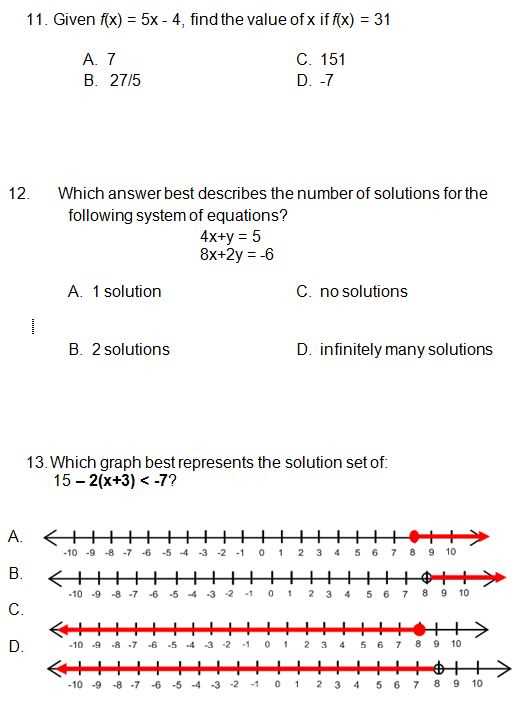
Key Topics to Focus On

To prepare effectively for an upcoming assessment, it’s essential to concentrate on the most significant mathematical concepts. Identifying these areas allows you to prioritize your study efforts and ensure you’re well-equipped to tackle a variety of questions. By focusing on the core topics, you can build confidence and improve your performance.

Core Concepts to Master

• Understanding quadratic functions and their properties
• Solving systems of equations using different methods
• Graphing and analyzing polynomial functions
• Working with rational expressions and simplifying them
• Manipulating exponents, radicals, and logarithmic functions

Advanced Problem Areas

• Complex numbers and their operations
• Exploring sequences, series, and their applications
• Mastering matrices and determinants
• Analyzing probability and statistics problems
• Working with conic sections and their equations

By revisiting these key topics and practicing problem-solving strategies, you will be prepared for any challenges that arise during the assessment. Regular review and focused practice will ensure that you have a strong understanding of the material.

Understanding Quadratic Equations

Quadratic equations are a fundamental part of higher-level mathematics, appearing in various contexts and applications. These equations can be solved using multiple methods, each of which provides insights into the behavior of the solutions. Understanding how to work with these expressions is crucial for tackling more advanced problems.

At their core, quadratic equations are polynomials of degree two, typically written in the form ax² + bx + c = 0. The solutions to these equations, known as roots, can be found through techniques such as factoring, completing the square, and using the quadratic formula. Each method has its own advantages depending on the equation’s structure.

By mastering quadratic equations, you can gain a deeper understanding of how variables interact in various mathematical situations, including graphing parabolas and solving real-world problems that involve rates of change or projectile motion.

Solving Systems of Equations

Systems of equations consist of multiple equations that share common variables. The goal is to find the values for these variables that satisfy all the equations simultaneously. Solving these systems is essential for addressing real-world problems where different relationships must be considered at once.

Methods for Solving Systems

• Graphical Method: Plotting each equation on a graph and identifying the point(s) of intersection.
• Substitution Method: Solving one equation for one variable and substituting the result into the other equation.
• Elimination Method: Adding or subtracting equations to eliminate one variable, making it easier to solve for the others.

Choosing the Right Approach

The method chosen often depends on the structure of the system. For simpler systems, the graphical method might suffice. For more complex systems, substitution or elimination may be necessary for efficient solutions. Practice with all methods ensures flexibility in problem-solving.

Exploring Polynomial Functions

Polynomial functions play a crucial role in understanding the behavior of complex mathematical relationships. These functions consist of terms with varying degrees, and their graphs exhibit a wide range of shapes and characteristics. By studying these functions, you gain insight into the properties of more advanced equations and how they can be applied to solve real-world problems.

Key Features of Polynomial Functions

• Degree of the Polynomial: The highest exponent of the variable determines the degree, which influences the function’s behavior and number of roots.
• End Behavior: The direction in which the graph of the polynomial heads as the variable increases or decreases.
• Roots and Factors: Solutions to the equation are the values where the function crosses the x-axis, and these can be found by factoring the polynomial.

Graphing Polynomial Functions

Graphing polynomials requires understanding the general shape of the function based on its degree and leading coefficient. By analyzing these features, you can predict where the function will cross the x-axis and how it will behave as the variable approaches positive or negative infinity.

Factoring Techniques Explained

Factoring is a key technique in solving mathematical expressions and equations, allowing for simplification and easier manipulation. By breaking down complex terms into simpler components, you can solve problems more effectively and uncover hidden relationships between variables. Mastering factoring methods is essential for success in higher-level problem-solving.

There are several strategies for factoring different types of expressions. The most common methods include recognizing common factors, applying the difference of squares, and using special trinomials. Each approach provides a way to express an equation in a form that is easier to solve or analyze.

Understanding when and how to use these techniques is vital for solving a variety of problems efficiently. Whether working with binomials, trinomials, or higher-degree polynomials, a solid grasp of factoring will streamline the process and help uncover solutions more quickly.

Mastering Rational Expressions

Rational expressions are a fundamental part of advanced mathematics, involving fractions with polynomials in the numerator and denominator. Mastering these expressions allows for simplifying complex relationships and solving problems involving rates, proportions, and more. Understanding how to manipulate and simplify rational expressions is crucial for tackling higher-level equations efficiently.

Simplifying Rational Expressions

The first step in working with rational expressions is simplifying them. This often involves factoring both the numerator and the denominator, then canceling out common factors. By reducing expressions to their simplest form, you can make calculations easier and reveal hidden patterns in the equation.

Operations with Rational Expressions

Once simplified, rational expressions can be added, subtracted, multiplied, or divided. Each operation requires careful attention to the rules for combining fractions, such as finding a common denominator for addition and subtraction. Mastery of these operations ensures you can handle more complex problems with ease.

Exponents and Radicals Simplified

Understanding how to work with exponents and radicals is key to solving many mathematical problems. These concepts often appear in equations that require simplification, and knowing how to handle them effectively makes the process much easier. Simplifying expressions with exponents and radicals helps to reveal their true structure and allows for more efficient problem-solving.

Working with Exponents

Exponents represent repeated multiplication, and simplifying expressions with exponents requires understanding the basic rules, such as the product rule, power rule, and quotient rule. By applying these rules, you can reduce complex expressions and make them more manageable. Additionally, negative exponents and fractional exponents are crucial for simplifying more advanced equations.

Understanding Radicals

Radicals are the inverse of exponents and involve extracting roots from numbers or expressions. Simplifying radicals often includes factoring out perfect squares or cubes to reduce the expression to its simplest form. Recognizing these patterns allows for quicker and more accurate simplification of square roots, cube roots, and higher-order roots.

Logarithms in Algebra 2

Logarithms provide a powerful way to express exponential relationships and solve equations involving exponents. Understanding logarithmic functions is essential for working with growth and decay models, as well as for simplifying complex mathematical expressions. This concept forms the foundation for solving a wide range of real-world problems, especially those involving large numbers or rapidly changing variables.

Properties of Logarithms

• Product Rule: The logarithm of a product is the sum of the logarithms of the factors. For example, log(a * b) = log(a) + log(b).
• Quotient Rule: The logarithm of a quotient is the difference of the logarithms. For example, log(a / b) = log(a) – log(b).
• Power Rule: The logarithm of a power is the exponent times the logarithm of the base. For example, log(a^n) = n * log(a).

Solving Logarithmic Equations

Solving logarithmic equations often involves rewriting them in exponential form or applying the inverse properties of logarithms. A solid understanding of logarithmic identities and how to manipulate them allows for quick and effective solutions to these types of problems.

Graphing and Analyzing Functions

Graphing and analyzing functions are crucial skills for understanding how different equations behave and interact. By visually representing functions, you can easily identify their key features, such as intercepts, asymptotes, and overall shape. These insights allow for better problem-solving and a deeper understanding of how various mathematical relationships work in practice.

Key Features of a Function’s Graph

Feature	Description
Intercepts	The points where the graph crosses the x-axis or y-axis. These points represent where the function equals zero or a specific value.
Asymptotes	Lines that the graph approaches but never touches. Asymptotes help identify the behavior of functions at extreme values.
Symmetry	Some functions have symmetrical graphs, meaning the function behaves similarly on either side of a central point or line.

Strategies for Graphing Functions

To graph a function effectively, it’s important to first identify its basic form and characteristics. Plot key points, determine the domain and range, and use transformations to shift, stretch, or reflect the graph. These steps help to create an accurate representation of the function, making it easier to analyze its behavior and solve related problems.

Complex Numbers and Operations

Complex numbers are an essential concept in mathematics, allowing us to solve equations that do not have real solutions. These numbers extend the idea of traditional numbers by incorporating both real and imaginary components. Understanding how to work with complex numbers is crucial for solving problems in advanced mathematics and physics, as they are often used to represent quantities that have both magnitude and direction.

Operations with complex numbers, such as addition, subtraction, multiplication, and division, follow specific rules that combine the real and imaginary parts. By mastering these operations, you can manipulate complex numbers and solve equations that involve them more easily. Whether adding or multiplying, it’s important to apply the appropriate steps to simplify expressions and obtain accurate results.

Probability and Statistics Essentials

Understanding the principles of chance and data analysis is vital for interpreting real-world phenomena. By studying probability, we learn how likely events are to occur, while statistics helps us collect, organize, and interpret data. Mastering these concepts is essential for making informed decisions based on mathematical evidence.

Concept	Description
Probability	Measures the likelihood of an event happening, ranging from 0 (impossible) to 1 (certain).
Mean	The average of a data set, found by summing all values and dividing by the number of values.
Median	The middle value in a data set when arranged in order. It divides the data into two equal halves.
Mode	The most frequent value in a data set.
Standard Deviation	A measure of how spread out the values in a data set are, indicating variability.

By learning these key concepts, you can apply mathematical reasoning to various scenarios, whether predicting outcomes or analyzing data trends.

Conic Sections and Their Equations

Conic sections are curves obtained by intersecting a plane with a cone. These shapes play an essential role in various fields such as physics, engineering, and astronomy. Each conic section has unique properties and can be represented algebraically by specific equations. Understanding how to derive and manipulate these equations is key to solving problems involving these geometric shapes.

There are four main types of conic sections: circles, ellipses, parabolas, and hyperbolas. Each shape is associated with a standard form equation that helps in graphing and analyzing its properties. Below are the basic forms and key characteristics of each conic section:

• Circle: The set of all points equidistant from a fixed center. The general equation is (x – h)² + (y – k)² = r², where h and k are the coordinates of the center and r is the radius.
• Ellipse: The set of points where the sum of the distances to two fixed points (foci) is constant. The equation for an ellipse is (x – h)² / a² + (y – k)² / b² = 1, where a and b are the distances from the center to the vertices along the x and y axes.
• Parabola: The set of points equidistant from a fixed point (focus) and a line (directrix). The equation for a parabola is y – k = a(x – h)² (for vertical orientation) or x – h = a(y – k)² (for horizontal orientation).
• Hyperbola: The set of points where the absolute difference in distances to two fixed points (foci) is constant. Its equation is (x – h)² / a² – (y – k)² / b² = 1, with a and b defining the distances from the center to the vertices along the x and y axes.

By understanding the structure and equations of conic sections, you can easily identify their characteristics, such as their focus, directrix, and asymptotes. These tools are essential for solving geometric and algebraic problems involving these shapes.

Sequences and Series Overview

Understanding sequences and series is essential for tackling a wide range of mathematical problems. A sequence is simply an ordered list of numbers, while a series is the sum of the terms of a sequence. These concepts form the foundation for more advanced topics in mathematics and have applications in fields like economics, physics, and computer science.

Sequences can be classified based on their patterns, such as arithmetic and geometric sequences. An arithmetic sequence has a constant difference between consecutive terms, while a geometric sequence has a constant ratio. On the other hand, series can be finite or infinite. A finite series sums a limited number of terms, while an infinite series continues indefinitely, often converging to a specific value.

Arithmetic Sequences and Series

An arithmetic sequence is defined by a fixed difference between successive terms. The general form of the nth term in an arithmetic sequence is given by:

Term Number (n)	General Formula
n	a_n = a_1 + (n – 1) * d

Here, a_1 is the first term, d is the common difference, and n is the term number. To find the sum of an arithmetic series, we use the following formula:

Sum of First n Terms	Formula
Sum (S_n)	S_n = n/2 * (2a_1 + (n – 1) * d)

Geometric Sequences and Series

A geometric sequence involves a constant ratio between consecutive terms. The nth term of a geometric sequence is given by:

Term Number (n)	General Formula
n	a_n = a_1 * r^(n – 1)

In this case, a_1 is the first term, and r is the common ratio. To find the sum of the first n terms of a geometric series, the following formula is used:

Sum of First n Terms	Formula
Sum (S_n)	S_n = a_1 * (1 – r^n) / (1 – r), for r ≠ 1

Both arithmetic and geometric sequences and series are powerful tools for solving a wide range of problems involving numbers that follow specific patterns. Mastering these concepts will help simplify many types of mathematical calculations and analysis.

Understanding Matrices and Determinants

Mathematical structures such as matrices and their associated determinants are crucial tools used for solving systems of equations, analyzing transformations, and understanding complex data relationships. A matrix is essentially a rectangular array of numbers arranged in rows and columns. Determinants, on the other hand, provide important information about the matrix, such as whether a system of equations has a unique solution or not.

To begin understanding these concepts, it is important to recognize that matrices come in different sizes, referred to as their “order” (i.e., the number of rows and columns they have). Operations can be performed on matrices, such as addition, multiplication, and finding their inverse, if it exists. Determinants are used to find the “invertibility” of a matrix and play a key role in solving linear systems, particularly when using methods such as Cramer’s rule or Gaussian elimination.

Key Matrix Operations

There are several basic operations you need to master to work effectively with matrices. These operations include:

• Matrix Addition: Only matrices of the same size can be added or subtracted. The elements of the resulting matrix are obtained by adding or subtracting corresponding elements.
• Matrix Multiplication: To multiply two matrices, the number of columns in the first matrix must equal the number of rows in the second. The resulting matrix’s element is calculated by taking the dot product of the corresponding row and column.
• Matrix Inversion: The inverse of a matrix is crucial for solving systems of equations. If a matrix is square and its determinant is non-zero, it will have an inverse.

Understanding Determinants

The determinant of a square matrix is a scalar value that can be computed from its elements. Determinants are helpful for assessing whether a matrix is invertible (a matrix is invertible if and only if its determinant is non-zero) and for solving linear systems. For a 2×2 matrix, the determinant is found using the formula:

Matrix	Formula
Matrix A [ begin{pmatrix} a & b \ c & d end{pmatrix} ]	Determinant: det(A) = ad – bc

For larger matrices, such as 3×3 matrices, the determinant is found using a more complex method, involving expansion by minors or cofactor expansion. The determinant is essential in many areas of mathematics and physics, especially when solving systems of linear equations.

Test-Taking Strategies for Algebra 2

Approaching a challenging assessment requires both preparation and effective strategies during the test itself. Understanding the structure and format of the questions is crucial for success. Proper time management, staying calm, and knowing when to skip and return to difficult problems can greatly improve your performance. By following certain techniques, you can maximize your efficiency and accuracy, ultimately achieving better results.

Start by reviewing all the questions quickly to get an overview. Identify the problems that seem more straightforward and tackle those first. This will help build confidence and ensure you secure easy points early. For more complex problems, break them down into smaller steps, and carefully re-read any instructions or definitions that might apply. Stay organized by keeping your work neat, which will reduce the chance of making simple mistakes.

• Prioritize Simple Questions: Begin with the easier questions to gain confidence and momentum. Leave more time-consuming problems for later.
• Work Systematically: Break down complex problems into manageable steps. If you are stuck, move on and return to it later with a fresh perspective.
• Use Process of Elimination: If you’re unsure about a multiple-choice question, eliminate obviously incorrect options to increase your chances of selecting the right answer.
• Double-Check Your Work: If time allows, go back over your answers, especially for calculations, to ensure no errors were made.

Remember that practicing regularly before the assessment can help reduce anxiety and make you more comfortable with various problem types. The more familiar you are with the material, the easier it will be to recognize patterns and apply strategies effectively.