Algebra 2 Final Exam Review Packet Answers

As you approach the end of your coursework, it’s essential to consolidate your knowledge and ensure that you’re ready for the upcoming comprehensive evaluation. This guide is designed to help you navigate through the various concepts that will be tested, providing clarity on the most critical topics you need to master. Whether you’re struggling with certain areas or just looking to sharpen your skills, this resource will serve as a valuable tool for your studies.

Key concepts such as solving equations, graphing functions, and understanding advanced problem-solving techniques will be highlighted, enabling you to tackle a wide range of questions with confidence. Each section is aimed at reinforcing your understanding of the material, ensuring that you can apply your knowledge effectively under pressure.

By focusing on problem-solving strategies, practicing core mathematical skills, and learning how to approach complex scenarios, you’ll be equipped to face challenges with ease. This guide will also assist you in identifying areas where you may need additional practice, allowing for a more targeted and efficient study session.

Algebra 2 Final Exam Review Packet Answers

In this section, you will find a detailed breakdown of key concepts that will be essential for mastering the upcoming assessment. The focus is on providing thorough guidance for tackling complex problems, reinforcing core skills, and ensuring a deeper understanding of the material. By exploring each topic, you will be better prepared to solve challenging questions with precision.

The following areas are critical to your success:

• Solving quadratic equations and inequalities
• Factoring and simplifying polynomials
• Working with rational expressions and equations
• Graphing functions and analyzing their properties
• Understanding exponential and logarithmic relationships
• Mastering systems of equations and inequalities
• Using matrices for problem-solving
• Exploring sequences and series
• Understanding the fundamentals of probability and statistics

Each topic is accompanied by step-by-step instructions and examples, which will help you grasp the necessary methods and techniques. Additionally, practicing a variety of problems will ensure you are ready for any variations you might encounter. To effectively prepare, focus on identifying common patterns and applying problem-solving strategies.

By systematically reviewing each of these concepts and practicing with the provided exercises, you will gain the confidence needed to excel. Ensure that you understand the underlying principles before moving on to more complex questions, as this will lay the foundation for your success.

Understanding Algebra 2 Exam Structure

To succeed in the upcoming assessment, it’s important to familiarize yourself with its overall structure and the types of problems you will encounter. This section will guide you through the typical format, providing insights into how the questions are organized and what to expect. Knowing the layout will help you manage your time effectively and approach each section with confidence.

Sections of the Assessment

The evaluation is generally divided into multiple sections, each focusing on a different set of skills and topics. You will be asked to demonstrate proficiency in solving equations, graphing functions, and manipulating expressions. Additionally, some sections will assess your ability to apply concepts to real-world problems, requiring a deeper understanding of the material.

Types of Questions

The questions can range from multiple-choice to open-ended problems, with some requiring detailed solutions and others focusing on quick, precise calculations. There may also be word problems that test your ability to interpret and apply mathematical concepts in practical situations. Understanding the question types in advance will help you prepare for both the theoretical and applied components of the assessment.

Key Topics Covered in Review Packet

This section highlights the fundamental concepts and topics that are essential for your preparation. These areas will provide a solid foundation for addressing various types of problems you may face. Mastering these concepts is crucial for achieving success in the upcoming assessment.

The main topics you should focus on include:

• Solving linear and quadratic equations
• Understanding and applying functions and their graphs
• Factoring and simplifying expressions
• Working with exponents and radicals
• Handling systems of equations and inequalities
• Analyzing polynomials and rational expressions
• Exploring exponential and logarithmic relationships
• Understanding sequences and series
• Utilizing matrices for problem-solving

Each of these topics plays a critical role in reinforcing your ability to approach different problem types, offering both theoretical insights and practical application strategies. A thorough understanding of these areas will help you excel in the test and strengthen your overall problem-solving skills.

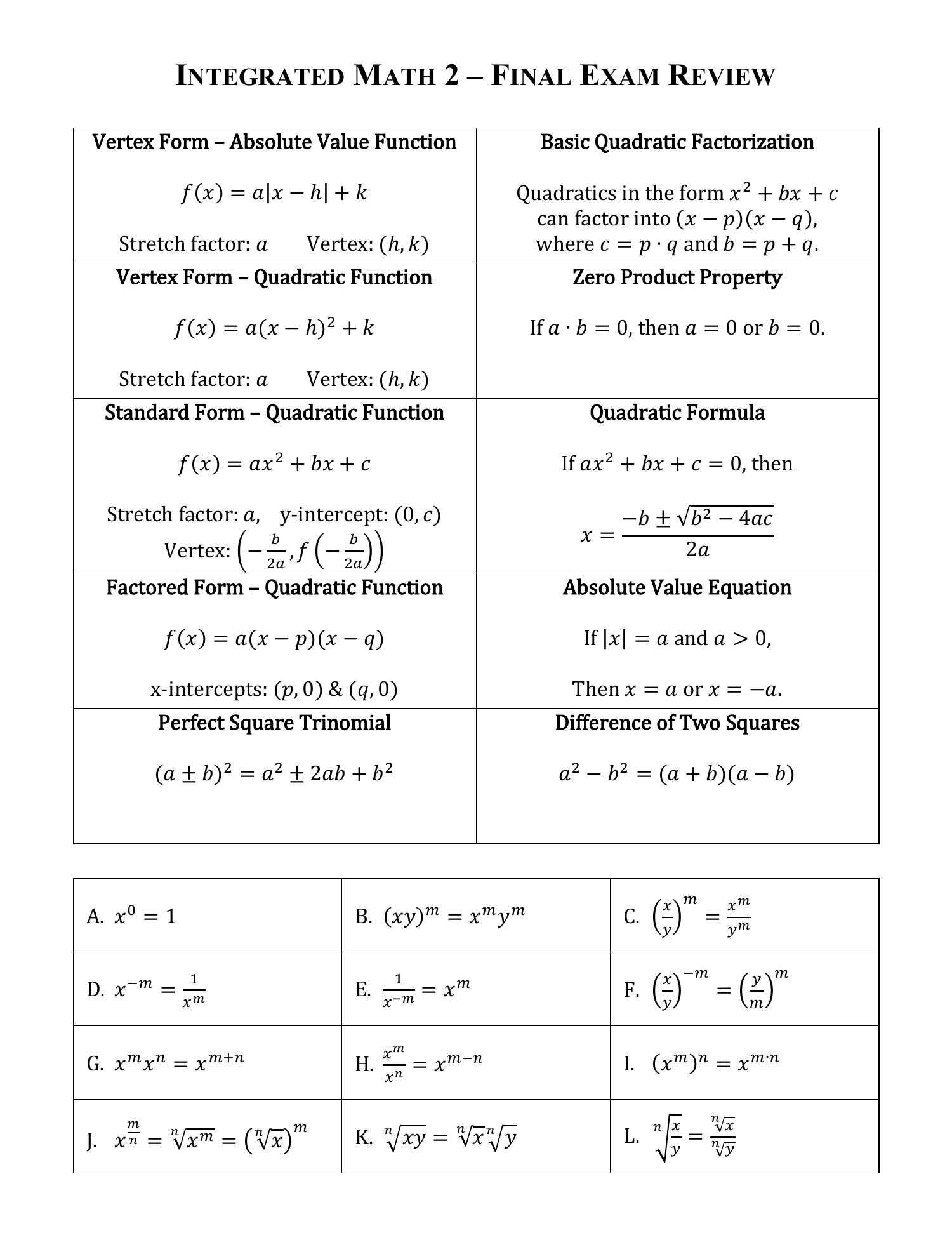
Solving Quadratic Equations Step by Step

Quadratic equations are a core component of many mathematical problems, and solving them with precision is essential for success. This section will guide you through the process of solving these types of equations systematically, helping you gain the skills needed to handle various forms efficiently.

To solve a quadratic equation, follow these general steps:

• Write the equation in standard form: Ensure the equation is in the form ax² + bx + c = 0, where a, b, and c are constants.
• Factor the quadratic expression: If possible, factor the equation into two binomials.
• Use the quadratic formula: If factoring is difficult, apply the quadratic formula: x = (-b ± √(b² – 4ac)) / 2a.
• Check for complex solutions: When the discriminant (b² – 4ac) is negative, the solutions will be complex.

By following these steps carefully, you’ll be able to solve most quadratic equations confidently, whether they are factorable or require the use of the quadratic formula. Practicing a variety of problems will help solidify these methods and prepare you for any variations you might encounter.

Factoring Polynomials for Exam Success

Factoring polynomials is an essential skill for solving many types of problems. By breaking down complex expressions into simpler components, you can gain insight into their structure and solve them more efficiently. This section will guide you through the steps to factor polynomials, offering strategies that will help you tackle even the most challenging questions.

Identifying the Greatest Common Factor

The first step in factoring is identifying the greatest common factor (GCF) of the terms in the polynomial. Factoring out the GCF simplifies the expression, making the remaining terms easier to work with. Always start by looking for any common factors that can be extracted.

Using Special Factoring Techniques

Once the GCF is factored out, apply specific techniques based on the form of the polynomial. For example, perfect square trinomials, difference of squares, and trinomial factoring all follow distinct patterns that can be recognized and utilized. Familiarizing yourself with these methods will help you factor more quickly and accurately.

With practice, these techniques will become second nature, allowing you to solve polynomial equations more effectively and efficiently. Remember, mastering factoring is not only about knowing the rules but also about recognizing patterns and applying strategies to simplify complex problems.

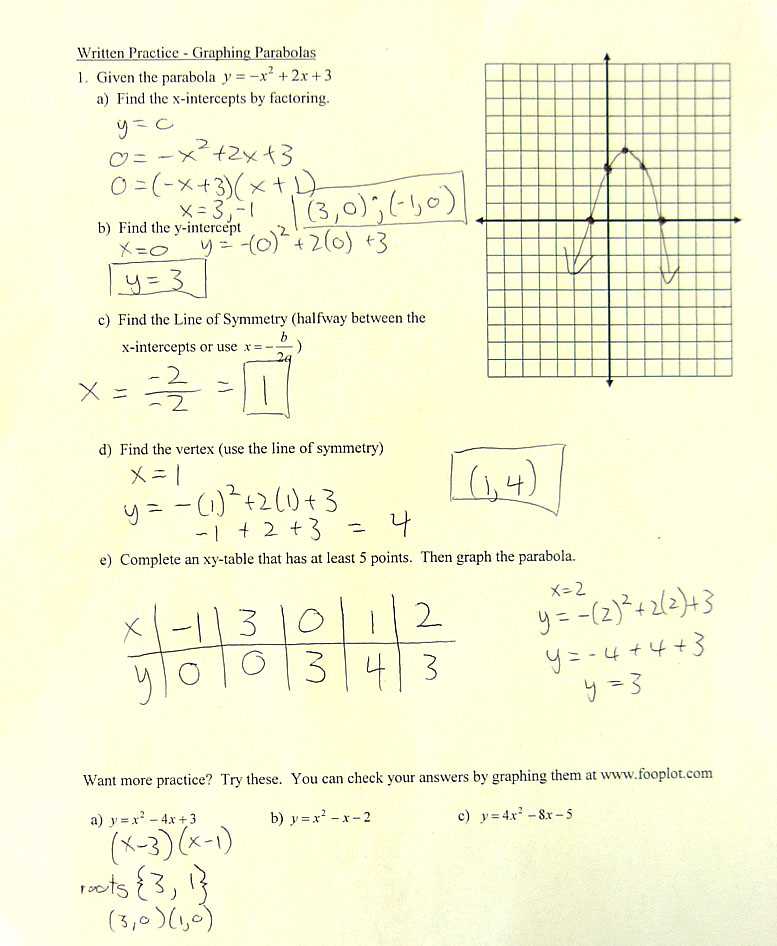
Graphing Parabolas and Functions

Graphing parabolas and other types of functions is a crucial skill for understanding their behavior and solving related problems. Whether you are dealing with quadratic functions or other types of curves, being able to visualize the function’s graph can help you better understand its properties, such as the vertex, axis of symmetry, and roots.

To graph parabolas effectively, follow these steps:

1. Identify the function’s form: Recognize whether the function is in standard form (ax² + bx + c) or vertex form (a(x – h)² + k), as this will affect how you plot the graph.
2. Find the vertex: The vertex is the highest or lowest point of the parabola. In standard form, use the formula x = -b / 2a to find the x-coordinate, and substitute it into the equation to find the y-coordinate.
3. Determine the axis of symmetry: The axis of symmetry is a vertical line that passes through the vertex. It can be found by the formula x = -b / 2a in the standard form.
4. Plot additional points: Once you have the vertex and axis of symmetry, plot additional points by substituting x-values into the function and calculating corresponding y-values.
5. Sketch the graph: Draw a smooth curve through the plotted points, ensuring the symmetry of the parabola on either side of the axis of symmetry.

For non-quadratic functions, such as linear or cubic functions, the process will vary slightly. However, understanding how to analyze the general shape and behavior of the function’s graph is key to interpreting its solutions and making accurate predictions.

Understanding Exponential and Logarithmic Functions

Exponential and logarithmic functions are powerful tools for solving a variety of problems, particularly when dealing with growth and decay, as well as complex equations. These functions are deeply connected, with logarithms being the inverse of exponentials. Understanding how they work and how to manipulate them is key to mastering more advanced mathematical concepts.

Exponential Functions

Exponential functions involve equations where the variable appears as an exponent. They often describe real-world phenomena such as population growth, radioactive decay, and compound interest. A general exponential function takes the form y = a * b^x, where a is the initial value, b is the base (a constant greater than 0), and x is the exponent. The base b determines the rate of growth or decay:

• If b > 1, the function models exponential growth.
• If 0 , the function models exponential decay.

Logarithmic Functions

Logarithmic functions are the inverse of exponential functions. They are used to solve equations in which the variable is in the exponent. The general form of a logarithmic function is y = log_b(x), where b is the base of the logarithm and x is the argument. The logarithmic function answers the question: “To what power must the base b be raised to obtain x?”

These functions are useful for simplifying complex problems, especially in fields such as science and engineering, where phenomena often follow exponential patterns. By converting exponential equations into logarithmic form, we can more easily solve for the unknown variable.

With a strong understanding of these functions, you will be able to analyze a wide range of problems involving growth, decay, and other dynamic systems, and apply these concepts to various real-world scenarios.

Solving Rational Expressions and Equations

Rational expressions and equations involve fractions where the numerator and denominator are polynomials. Solving these types of problems requires careful manipulation of algebraic fractions, simplifying the expressions, and solving for the unknowns. Mastery of these techniques is essential for simplifying complex expressions and solving equations involving rational functions.

Simplifying Rational Expressions

The first step in working with rational expressions is simplification. To simplify, find common factors in the numerator and denominator, and cancel them out. This reduces the expression to its simplest form, making it easier to solve or evaluate.

Expression	Simplified Form
(2x² + 4x) / (2x)	x + 2
(3x³ + 6x²) / (3x²)	x + 2

Solving Rational Equations

To solve equations involving rational expressions, follow these steps:

1. Find a common denominator: If the equation has multiple rational expressions, find the least common denominator (LCD) to combine the terms.
2. Multiply through by the LCD: This eliminates the fractions and simplifies the equation.
3. Solve the resulting equation: Once the fractions are gone, solve the remaining equation using standard methods like factoring or the quadratic formula.
4. Check for extraneous solutions: Always substitute your solutions back into the original equation to ensure they do not cause division by zero.

By following these steps, you can simplify and solve rational expressions and equations effectively, ensuring that your solutions are accurate and valid.

Mastering Systems of Linear Equations

Solving systems of linear equations is a key skill for tackling a wide range of problems in mathematics and real-life situations. These systems involve finding the values of multiple variables that satisfy two or more linear equations simultaneously. Mastering the methods for solving these systems is essential for progressing in more advanced topics.

There are several methods to solve systems of linear equations, each suited to different types of problems. These methods include substitution, elimination, and graphing. The goal is always to find the values of the variables that make all equations in the system true at the same time.

Methods for Solving Systems

The most common methods to solve systems of linear equations are:

• Substitution Method: Solve one equation for one variable and then substitute this expression into the other equation(s) to solve for the remaining variable(s).
• Elimination Method: Add or subtract the equations to eliminate one variable, making it easier to solve for the other variable.
• Graphing Method: Graph each equation on the same coordinate plane and identify the point(s) of intersection, which represent the solution(s) to the system.

Example of Solving Using Substitution

Consider the following system of equations:

1. 2x + y = 10
2. x – y = 4

To solve using substitution:

1. From the second equation, solve for x: x = y + 4
2. Substitute x = y + 4 into the first equation: 2(y + 4) + y = 10
3. Simplify and solve for y: 2y + 8 + y = 10 ⟹ 3y = 2 ⟹ y = 2/3
4. Substitute y = 2/3 back into the second equation to solve for x: x – (2/3) = 4 ⟹ x = 4 + 2/3 = 14/3

The solution to the system is x = 14/3 and y = 2/3.

By mastering these techniques, you’ll be able to solve systems of equations more efficiently and confidently, laying a solid foundation for tackling more complex problems in mathematics and applied fields.

Working with Complex Numbers in Algebra

Complex numbers extend the real number system by introducing the concept of the imaginary unit, denoted as i, where i² = -1. This allows for the solution of equations that do not have real number solutions, such as the square root of negative numbers. Mastery of complex numbers is essential for understanding more advanced topics and solving equations that involve both real and imaginary components.

Structure of Complex Numbers

A complex number is generally written in the form a + bi, where a is the real part and b is the imaginary part. The real and imaginary components can be combined, added, or subtracted, just like polynomials. The number i represents the square root of -1, and any multiple of i is considered imaginary.

Operations with Complex Numbers

When working with complex numbers, you can perform various operations, such as addition, subtraction, multiplication, and division. Here’s a breakdown of these operations:

• Addition: Add the real parts and the imaginary parts separately. For example, (3 + 4i) + (2 + 5i) = (3 + 2) + (4i + 5i) = 5 + 9i.
• Subtraction: Subtract the real and imaginary parts separately. For example, (7 + 3i) – (4 + 2i) = (7 – 4) + (3i – 2i) = 3 + i.
• Multiplication: Use the distributive property, remembering that i² = -1. For example, (2 + 3i) * (1 + 4i) = 2 + 8i + 3i + 12i² = 2 + 11i – 12 = -10 + 11i.
• Division: To divide complex numbers, multiply the numerator and denominator by the conjugate of the denominator. For example, (2 + 3i) / (1 – 4i) would be simplified by multiplying both the numerator and denominator by (1 + 4i).

By mastering operations with complex numbers, you’ll be able to solve more sophisticated problems and expand your mathematical toolkit to include solutions that involve both real and imaginary quantities.

Analyzing Sequences and Series

Understanding sequences and series is crucial for mastering patterns in mathematics. A sequence is an ordered list of numbers that follow a specific pattern, while a series represents the sum of the terms in a sequence. By studying the behavior of sequences and series, you can solve problems related to growth patterns, summing terms, and modeling real-world situations such as finance and population growth.

Types of Sequences

Sequences can be classified into various types based on their patterns. The two most common types are:

• Arithmetic Sequences: In these sequences, the difference between consecutive terms is constant. For example, 2, 5, 8, 11, 14, … has a common difference of 3.
• Geometric Sequences: These sequences have a constant ratio between consecutive terms. For example, 3, 6, 12, 24, … has a common ratio of 2.

Sum of Series

The sum of a sequence’s terms is referred to as a series. The sum can be finite or infinite depending on the number of terms involved. The two primary types of series are:

• Arithmetic Series: The sum of the terms in an arithmetic sequence. The formula for the sum of the first n terms is S_n = n/2 * (a₁ + a_n), where a₁ is the first term and a_n is the last term.
• Geometric Series: The sum of the terms in a geometric sequence. The sum of the first n terms is S_n = a₁ * (1 – rⁿ) / (1 – r), where a₁ is the first term and r is the common ratio.

By mastering the concepts of sequences and series, you can tackle complex mathematical problems involving growth, decay, and patterns. These techniques are also fundamental for understanding more advanced topics in calculus and other areas of mathematics.

Using Matrices for Solving Problems

Matrices provide a powerful tool for organizing and solving complex mathematical problems. They allow for efficient handling of systems of linear equations, transformations, and other applications in fields like economics, physics, and engineering. By representing data in matrix form, you can apply various operations to solve problems systematically and quickly.

Solving Systems of Equations with Matrices

One of the most common uses of matrices is solving systems of linear equations. Instead of solving equations individually, matrices provide a compact and organized way to handle multiple equations at once. A system of equations can be represented as a matrix equation, which can then be solved using methods like row reduction or matrix inversion.

The general process involves:

• Converting the system of linear equations into an augmented matrix.
• Using Gaussian elimination or row operations to simplify the matrix.
• Finding the solution by interpreting the matrix in terms of variables.

Matrix Operations and Their Applications

In addition to solving systems of equations, matrices can be used for a variety of other operations, such as:

• Matrix Addition and Subtraction: Combining matrices by adding or subtracting corresponding elements.
• Matrix Multiplication: Multiplying matrices to perform transformations or solve equations with multiple variables.
• Determinants and Inverses: Using the determinant and inverse of a matrix to solve equations and analyze properties of systems.

Understanding matrix operations and their applications provides a strong foundation for tackling more advanced mathematical problems, and is essential for fields requiring complex data analysis and transformations.

Understanding Probability and Statistics Concepts

Probability and statistics play a crucial role in analyzing data, making predictions, and drawing conclusions based on real-world observations. By understanding these concepts, you can better assess outcomes, evaluate risks, and make informed decisions. From calculating the likelihood of events to interpreting data distributions, mastering these topics equips you with essential tools for problem-solving across various fields such as finance, science, and social studies.

Key Concepts in Probability

Probability deals with determining the likelihood of an event occurring. Some of the essential concepts in probability include:

• Sample Space: The set of all possible outcomes in an experiment or trial.
• Event: A specific outcome or group of outcomes from the sample space.
• Probability of an Event: The measure of how likely an event is to occur, calculated as the number of favorable outcomes divided by the total number of possible outcomes.
• Independent and Dependent Events: Independent events do not affect each other’s probability, while dependent events do.

Statistics and Data Analysis

Statistics involves collecting, analyzing, interpreting, and presenting data. It helps in summarizing and making sense of large amounts of information. The main tools used in statistics include:

• Mean, Median, and Mode: These measures describe the central tendency of a data set, helping you understand the typical or most frequent value.
• Standard Deviation: A measure of how spread out the values in a data set are, indicating the variability of the data.
• Probability Distributions: Functions that describe the likelihood of different outcomes in a random experiment, such as normal and binomial distributions.

Understanding Data with Frequency Tables

Frequency tables are used to organize data and identify patterns. Below is an example of a simple frequency table that illustrates the distribution of data:

Data Range	Frequency
0-10	5
11-20	8
21-30	12
31-40	6

Understanding how to construct and interpret such tables allows you to quickly summarize and visualize data, making it easier to identify trends and patterns.

Exploring Conic Sections in Detail

Conic sections are curves formed by the intersection of a plane and a cone. These shapes play a significant role in mathematics and physics, appearing in diverse contexts such as the orbits of planets, parabolic reflectors, and even in the design of lenses. Understanding conic sections allows you to explore how these curves behave under different transformations and their geometric properties. The four primary types of conic sections–circles, ellipses, parabolas, and hyperbolas–each have unique characteristics and applications.

Types of Conic Sections

The four main types of conic sections are:

• Circle: A set of points equidistant from a fixed point called the center. The equation of a circle is given by (x – h)² + (y – k)² = r², where (h, k) is the center and r is the radius.
• Ellipse: A curve formed by the intersection of a plane with a cone that produces a symmetrical oval shape. The equation for an ellipse is (x – h)²/a² + (y – k)²/b² = 1, where a and b are the semi-major and semi-minor axes, respectively.
• Parabola: A curve where every point is equidistant from a fixed point (the focus) and a line (the directrix). The standard form of a parabola is y = ax² + bx + c.
• Hyperbola: A curve formed when a plane intersects a cone at a steeper angle, resulting in two branches. The equation of a hyperbola is (x – h)²/a² – (y – k)²/b² = 1, with a and b being constants that define the shape.

Applications and Properties of Conic Sections

Each conic section has distinct properties and applications in real-world scenarios:

• Circles: Used in navigation, mechanical engineering, and astronomy to model circular motion and uniform rotational systems.
• Ellipses: Commonly found in planetary orbits and satellite paths due to their unique geometric properties.
• Parabolas: Seen in the design of reflectors, such as satellite dishes and headlights, where parallel rays converge at a single point.
• Hyperbolas: Appear in the fields of telecommunications and navigation, particularly in the context of radio signals and GPS systems.

By studying these conic sections, one gains insight into their intrinsic geometric relationships and their diverse real-life applications, making them an essential part of advanced mathematical understanding.

Dealing with Rational Expressions Simplifications

Simplifying rational expressions is a fundamental skill that involves reducing complex fractions to their simplest form. It requires understanding how to work with numerators and denominators, cancel common factors, and identify opportunities for simplification. Mastering these techniques not only aids in solving mathematical problems but also helps in real-world applications such as engineering and computer science, where fractions are frequently encountered.

The process of simplifying rational expressions can be broken down into several key steps. First, factor both the numerator and the denominator, if possible. Next, cancel any common factors between the numerator and denominator. Finally, ensure that the expression is in its simplest form, avoiding unnecessary complexity.

Steps to Simplify Rational Expressions

To simplify a rational expression, follow these steps:

1. Factor both the numerator and denominator: Break down any polynomials into their prime factors, such as factoring quadratics or recognizing special factoring formulas like difference of squares.
2. Cancel common factors: Once factored, identify and cancel any terms that appear in both the numerator and denominator.
3. Simplify the remaining expression: After canceling out the common factors, combine the remaining terms to form the simplest possible expression.

Example of Simplification

Consider the rational expression (x² – 9) / (x² – 6x + 9). To simplify:

Step	Action
Step 1	Factor both the numerator and denominator:
Numerator	x² – 9 = (x – 3)(x + 3)
Denominator	x² – 6x + 9 = (x – 3)(x – 3)
Step 2	Cancel common factors:
Result	(x + 3) / (x – 3)

After simplifying the expression, you end up with the reduced form (x + 3) / (x – 3), which is much easier to work with than the original form.

Understanding and applying these simplification techniques can help streamline problem-solving processes and improve efficiency in solving more complex mathematical problems.

Strategies for Tackling Word Problems

Word problems are an essential part of mathematical challenges, as they involve applying theoretical concepts to real-world situations. These problems often require a combination of reading comprehension, logical reasoning, and systematic problem-solving techniques. By approaching word problems strategically, you can break down complex situations into manageable steps and find solutions more efficiently.

To solve word problems effectively, it’s crucial to follow a methodical approach. Start by carefully reading the problem and identifying the key information. Then, translate the problem into mathematical expressions or equations. Finally, solve the problem using appropriate methods and check your work for accuracy.

Steps to Solve Word Problems

Follow these steps to enhance your problem-solving abilities:

1. Read the problem carefully: Understand the context and focus on identifying important details such as quantities, relationships, and what is being asked.
2. Identify known and unknown variables: Define the variables that represent the unknowns and express them clearly in terms of the given information.
3. Set up equations: Translate the information into mathematical expressions, such as equations or inequalities, that can be manipulated to find the solution.
4. Solve the equation: Use appropriate mathematical operations, such as addition, subtraction, multiplication, or division, to solve for the unknown variable.
5. Check your solution: Review the solution to ensure it makes sense in the context of the problem and double-check your calculations.

Example of Solving a Word Problem

Let’s consider the following word problem:

Problem	A car travels at 60 miles per hour. How far will the car travel in 4 hours?
Step 1	Identify the known values: speed = 60 miles per hour, time = 4 hours
Step 2	Set up the equation: Distance = speed × time
Step 3	Substitute the values: Distance = 60 × 4
Step 4	Calculate the result: Distance = 240 miles
Step 5	Check: Does the solution make sense? The car is traveling 240 miles in 4 hours, which is consistent with the given speed.

By following this systematic approach, you can solve word problems with greater confidence and precision. This method helps ensure you consider all the relevant details and leads to clearer, more organized solutions.

Common Mistakes to Avoid During the Exam

When working through mathematical problems, especially under time constraints, it’s easy to make small yet significant mistakes that can lead to incorrect answers. Avoiding these errors requires careful attention to detail, clear thinking, and consistent problem-solving strategies. Recognizing common pitfalls can help you stay focused and increase your chances of achieving the correct solutions.

Here are some frequent mistakes to watch out for:

• Misreading the Problem: One of the most common errors is not fully understanding the problem. Read each question carefully to ensure you comprehend what is being asked before jumping into calculations. Pay close attention to keywords like “total,” “difference,” or “product” as they indicate specific operations.
• Forgetting to Simplify Expressions: After solving a problem, it’s important to simplify your final answer. Many times, students overlook the final step of simplifying fractions, expressions, or factoring terms, leaving answers in an incomplete or unsimplified form.
• Incorrect Sign Handling: Neglecting the proper handling of positive and negative signs, especially when dealing with equations, can lead to incorrect results. Double-check each step to ensure that you’ve accounted for all signs appropriately, especially when multiplying or dividing negative numbers.
• Skipping Units or Labels: In word problems or real-life applications, it’s crucial to include units of measurement or labels in your final answer. Leaving out these essential details may lead to confusion and cause you to lose marks, even if your calculations are correct.
• Overcomplicating Simple Problems: Sometimes, students overthink or complicate a straightforward problem by applying unnecessary methods or looking for patterns that don’t exist. Stick to the basic principles and use the simplest approach when possible.
• Rushing Through the Last Few Problems: It’s easy to make errors when you’re near the end of the test and you’re trying to finish quickly. Even if you’re running out of time, take a moment to double-check your work, especially on problems that carry more weight or are more complex.

Pro Tip: One effective strategy is to allocate time for a quick review at the end of the test. Reread the questions and your answers to catch any errors or omissions before submitting your work.

By being mindful of these common mistakes, you can approach your problems with greater confidence and improve the accuracy of your solutions. Staying focused and organized will help you perform to the best of your ability under any circumstances.

Final Tips for Exam Day Success

As the day of the test approaches, it’s essential to implement strategies that will help you perform at your best. The preparation process is important, but how you manage yourself on the day of the assessment plays an equally crucial role in achieving success. Keeping calm, focused, and organized will give you the edge needed to tackle the challenges ahead.

Here are some key tips for ensuring you are fully prepared for the test day:

• Get a Good Night’s Sleep: Rest is vital for cognitive function. Ensure you get enough sleep the night before, as fatigue can affect concentration and memory. Avoid cramming late into the night–your brain needs rest to work efficiently during the test.
• Eat a Healthy Meal: Fuel your body with a nutritious meal before the test. A balanced breakfast with protein and complex carbohydrates will provide the energy you need to stay focused and alert. Avoid heavy or sugary foods that can cause an energy crash.
• Bring All Necessary Materials: Make sure you have all the tools you need for the test, including pens, pencils, erasers, a calculator (if allowed), and any other materials specified by the instructor. Check your materials the night before to avoid last-minute scrambling.
• Arrive Early: Aim to arrive at the test location a little early to avoid feeling rushed. Arriving early allows you to settle in, review your notes if needed, and calm your nerves before starting.
• Stay Calm and Confident: It’s natural to feel nervous, but try to maintain a calm and positive attitude. Take deep breaths, stay focused on the task at hand, and remember that you’ve prepared for this moment. Confidence is key to staying on track.
• Manage Your Time Wisely: Be mindful of the time allocated for each section or question. Don’t spend too much time on any one problem–move on if you’re stuck, and come back to it later if needed. Make sure to leave some time at the end for review.
• Read Each Question Carefully: Carefully read each question to ensure you understand what is being asked. Pay attention to specific instructions, keywords, and the format of the answer. Misinterpreting a question can lead to unnecessary mistakes.
• Stay Organized During the Test: Keep your work neat and organized. If working on scratch paper, clearly show all steps for each problem, so it’s easier to spot errors later. A clean presentation can also help your thoughts stay organized.
• Don’t Panic if You Get Stuck: If you come across a challenging problem, take a deep breath and move on to the next one. Sometimes stepping away from a problem gives you a fresh perspective when you return to it. Keep moving forward to ensure you complete all the tasks.

By following these strategies, you’ll be setting yourself up for success. With proper preparation, a positive mindset, and a calm approach on test day, you will be able to navigate the challenges with confidence and perform at your best.