Algebra 2 Regents June 2025 Answer Key
Overview of Algebra 2 Regents Exam
What to Expect on the 2025 Exam
How to Prepare for Algebra 2
Key Topics Covered in Algebra 2
Tips for Success in Algebra 2
Understanding the Question Format
Common Mistakes to Avoid
How to Use Practice Tests Effectively

Time Management Strategies for the Exam
Scoring and Grading Breakdown
Answer Explanations for Common Questions

Online Resources for Algebra 2 Help
How to Review Algebra 2 Concepts
Additional Study Materials for Regents

How to Interpret Answer Key Results
Post-Exam Tips and Next Steps
Benefits of Understanding Algebra 2 Answers
Overview of Algebra 2 Regents Exam
What to Expect on the 2025 Exam
How to Prepare for Algebra 2
Key Topics Covered in Algebra 2
Tips for Success in Algebra 2
Understanding the Question Format
Common Mistakes to Avoid
How to Use Practice Tests Effectively
Time Management Strategies for the Exam
Scoring and Grading Breakdown
Answer Explanations for Common Questions
Online Resources for Algebra 2 Help
How to Review Algebra 2 Concepts
Additional Study Materials for Regents
How to Interpret Answer Key Results
Post-Exam Tips and Next Steps
Benefits of Understanding Algebra 2 Answers
Algebra 2 Regents June 2025 Answers
This section provides essential insights into the solution strategies for the upcoming mathematics exam. It will explore the key areas that students will encounter and how to approach them effectively. By understanding the structure and types of problems presented, students can better prepare for the test and improve their performance. The focus here is on refining problem-solving skills and mastering the concepts needed for success.
Preparation for this exam involves thorough practice with a variety of question types, each requiring different methods to solve. Understanding the logical steps and reasoning behind each problem is crucial. In addition, it’s important to familiarize oneself with the test format to ensure confidence during the actual exam. Reviewing sample problems and going through detailed solution explanations will help clarify any misunderstandings and solidify your grasp on the material.
Having a well-organized study plan and utilizing effective resources is key to improving performance. Practice tests, tutoring, and online resources can be extremely beneficial in reinforcing your knowledge. With diligent preparation and focused effort, students can tackle the exam with confidence and achieve the best results possible.
How to Prepare for Algebra 2
Preparing for a higher-level mathematics course requires understanding key concepts and developing strong problem-solving skills. To achieve success, it is essential to master the fundamentals before tackling more complex topics. Consistent practice, along with effective study habits, will help you build the confidence needed to perform well in assessments.
Master Core Concepts
The foundation of this course relies heavily on mastering core mathematical principles such as equations, functions, and graphing. Start by revisiting basic operations and ensure that you understand how to manipulate expressions and solve for unknowns. Working through sample problems will solidify your understanding and allow you to tackle more advanced questions with ease.
Practice Regularly
Consistency is key when preparing for any challenging subject. Set aside time each day to solve practice problems, review past material, and seek additional resources if necessary. The more problems you encounter, the more comfortable you will become with different question formats and methods. Don’t hesitate to reach out for help if you encounter difficulties; understanding your mistakes is crucial for improvement.
Key Topics Covered in Algebra 2
This course introduces several important mathematical concepts that are crucial for progressing to more advanced studies. Understanding these topics will not only prepare you for assessments but also provide the necessary tools to solve a wide range of problems. Mastering these areas is essential for building a strong foundation in mathematics.
Functions and Their Properties
One of the primary focuses of this course is the study of functions. You will learn how to analyze, graph, and manipulate various types of functions, including linear, quadratic, exponential, and rational. Understanding the behavior of functions, such as finding their domain, range, and intercepts, is fundamental for tackling complex equations and real-world problems.
Systems of Equations and Inequalities
Another key topic is solving systems of equations and inequalities. You will explore methods such as substitution, elimination, and graphical solutions to find the points of intersection for multiple equations. Additionally, understanding how to solve and graph inequalities will help in modeling a wide range of scenarios where multiple conditions must be satisfied simultaneously.
Tips for Success in Algebra 2
Achieving success in this challenging mathematical course requires more than just understanding the concepts–it involves developing strong problem-solving strategies and adopting effective study techniques. With dedication and the right approach, you can build confidence and perform well throughout the course.
Stay Organized
Staying organized is crucial for managing the various topics and assignments in this course. Keep track of your notes, assignments, and tests in an organized manner. A well-maintained notebook will help you quickly reference important concepts when needed, especially during revision sessions.
Seek Help When Needed
If you encounter difficulties with any topic, don’t hesitate to ask for help. Whether it’s from your teacher, a classmate, or online resources, getting clarification can prevent confusion from building up. Regularly reviewing material with others can also help reinforce your understanding.
| Strategy | Benefits |
|---|---|
| Practice regularly | Helps reinforce concepts and builds confidence in problem-solving. |
| Review notes after class | Strengthens retention and makes future lessons easier to understand. |
| Break down complex problems | Reduces overwhelming tasks into manageable steps, making them easier to solve. |
Understanding the Question Format
Being familiar with the structure of questions in a mathematics assessment is essential for effective preparation. Recognizing how questions are typically presented allows you to approach them with the right strategies, ensuring you can solve them efficiently within the given time. The format often includes different types of problems that test various skills, and understanding these patterns will help you feel more confident during the exam.
Types of Questions
- Multiple Choice: These questions present several possible answers, where you need to select the correct one. Practice eliminating incorrect options to improve your chances.
- Open Response: Here, you are required to show your work and explain your reasoning. Focus on writing clear steps and justifications.
- Graphing: These questions require you to plot data or functions on a graph. Ensure you understand how to interpret the axes and scale.
Question Strategies
- Read the Question Carefully: Ensure you fully understand what is being asked before attempting a solution.
- Identify Key Information: Highlight or note down important values, variables, or operations needed to solve the problem.
- Time Management: Don’t get stuck on one problem. Move on if necessary, and come back to it later.
Common Mistakes to Avoid
When tackling complex mathematical problems, it’s easy to make errors that can affect your performance. Recognizing and addressing these common pitfalls is key to improving accuracy and ensuring you don’t lose valuable points. By being aware of frequent mistakes, you can take steps to avoid them and approach each problem with confidence.
Frequent Errors
- Skipping Steps: Rushing through problems often leads to missed steps. Always show your work clearly and methodically, even when you think the answer is obvious.
- Incorrect Calculation: Simple arithmetic mistakes can add up, especially in multi-step problems. Double-check calculations, especially when working with fractions or decimals.
- Misinterpreting the Question: Read the problem carefully to ensure you understand what is being asked. A common mistake is solving for the wrong variable or missing a key detail in the problem.
- Not Reviewing the Work: If time permits, review your answers before submitting. Even small mistakes can be caught by taking a second look.
Ways to Minimize Mistakes
- Organize Your Work: Keep equations neat and well-organized to reduce confusion during problem-solving.
- Practice Regularly: The more problems you solve, the more comfortable you become with identifying and avoiding common mistakes.
- Take Your Time: Avoid rushing through questions. Time management is important, but speed should not come at the expense of accuracy.
Common Mistakes to Avoid
When tackling complex mathematical problems, it’s easy to make errors that can affect your performance. Recognizing and addressing these common pitfalls is key to improving accuracy and ensuring you don’t lose valuable points. By being aware of frequent mistakes, you can take steps to avoid them and approach each problem with confidence.
Frequent Errors
- Skipping Steps: Rushing through problems often leads to missed steps. Always show your work clearly and methodically, even when you think the answer is obvious.
- Incorrect Calculation: Simple arithmetic mistakes can add up, especially in multi-step problems. Double-check calculations, especially when working with fractions or decimals.
- Misinterpreting the Question: Read the problem carefully to ensure you understand what is being asked. A common mistake is solving for the wrong variable or missing a key detail in the problem.
- Not Reviewing the Work: If time permits, review your answers before submitting. Even small mistakes can be caught by taking a second look.
Ways to Minimize Mistakes
- Organize Your Work: Keep equations neat and well-organized to reduce confusion during problem-solving.
- Practice Regularly: The more problems you solve, the more comfortable you become with identifying and avoiding common mistakes.
- Take Your Time: Avoid rushing through questions. Time management is important, but speed should not come at the expense of accuracy.
Scoring and Grading Breakdown
The evaluation process for this examination involves a detailed and structured assessment of your performance, ensuring fairness and accuracy. The grading system is designed to reflect your understanding of the material covered and your ability to apply key concepts to various types of problems. Each section contributes to the final score, and your results will be broken down into specific categories to determine overall achievement.
Section Weighting and Contribution
The test consists of multiple sections, each with a different level of difficulty and weight in the final score. Typically, the questions are divided into categories based on topic areas, where more complex questions are weighted higher. Understanding how each section contributes to the total score is crucial for prioritizing study efforts and maximizing performance.
Grading Criteria and Interpretation
Grading standards vary by the institution or examining body, but they generally follow a consistent framework to ensure that results are comparable across different years. The scoring scale ranges from low to high, with specific thresholds that define passing marks. Performance on each individual section is analyzed, and the final grade reflects both the overall achievement and mastery in specific areas.
Online Resources for Algebra 2 Help
In today’s digital age, students have a wide array of platforms available to enhance their understanding of mathematical concepts. These resources offer everything from step-by-step tutorials to interactive problem-solving tools, making it easier than ever to tackle challenging topics. Whether you’re looking for explanations, practice exercises, or video lessons, these online platforms provide valuable support to help improve your skills.
Video Tutorials are one of the most effective ways to learn complex ideas. Many educational websites feature instructors breaking down difficult concepts into smaller, more digestible pieces. Popular platforms like YouTube offer channels dedicated to explaining various mathematical theories, while websites like Khan Academy offer structured lessons that guide you through topics from basic to advanced levels.
Interactive Practice Websites allow students to apply what they’ve learned in real-time. Platforms such as IXL or Mathway provide tailored exercises where you can receive immediate feedback. These websites often track your progress and suggest additional exercises based on areas where you need improvement, helping to reinforce your learning.
For those who prefer a more formal approach, online tutoring services are available for personalized support. Websites like Chegg and Tutor.com connect students with experienced tutors who can help clarify concepts, solve problems, and provide additional practice. This one-on-one help can make a significant difference in mastering difficult topics.
In addition, online forums such as Reddit or specialized math communities offer a place where you can ask questions and engage in discussions with other learners. These forums are ideal for sharing knowledge and finding solutions to problems that may be tricky to solve on your own.
How to Review Algebra 2 Concepts
Reviewing mathematical topics requires a strategic approach that combines understanding the theory with practical application. Focusing on key concepts, practicing regularly, and utilizing various study methods can significantly boost retention and performance. The following tips offer a clear guide on how to effectively revisit important ideas and strengthen problem-solving skills.
Start with Fundamentals to ensure a solid foundation. Revisiting basic principles and revising past lessons will help you connect more complex ideas with what you already know. Start with core topics such as linear equations, functions, and exponents, then gradually move towards more intricate subjects like quadratic equations and polynomial functions.
Practice with Problem Sets to build confidence and sharpen your skills. Use a variety of worksheets, online exercises, and textbook problems to test your knowledge. Consistent practice not only reinforces understanding but also highlights areas where you may need additional review. The more problems you solve, the more comfortable you will become with applying different methods.
Use Visual Aids like graphs and diagrams to understand abstract concepts. Drawing out equations or visualizing geometric shapes can make it easier to grasp relationships between variables. Tools like graphing calculators or software can also provide a more interactive way to explore and understand these relationships.
Teach What You’ve Learned to reinforce your understanding. Explaining concepts to someone else, whether a peer or tutor, can clarify your own comprehension. Teaching forces you to break down complex ideas into simpler steps, helping you to solidify your knowledge.
Finally, take regular breaks and review periodically to prevent burnout. Spacing out your review sessions over time ensures better long-term retention and reduces the stress of cramming before exams.
Additional Study Materials for Regents
Supplementing your study routine with extra resources can provide a deeper understanding of the material and improve your chances of success. Whether you’re looking for practice exams, instructional videos, or comprehensive guides, these additional study tools can help reinforce key concepts and clarify difficult topics. Utilizing a variety of materials will ensure that you are fully prepared for the assessment.
Practice Exams are one of the most effective ways to prepare. These exams simulate the actual test environment, helping you familiarize yourself with the format and time constraints. Many educational websites and textbooks offer sample questions or full-length practice tests, allowing you to assess your readiness and identify areas that need more focus.
Video Lessons can provide a more visual and interactive approach to studying. Platforms like Khan Academy and YouTube have countless videos explaining various topics in detail. These resources are especially useful for visual learners who benefit from step-by-step demonstrations of problem-solving techniques.
Study Guides and Textbooks often offer comprehensive overviews of the material. Well-organized study guides break down each topic into manageable sections and provide detailed examples, making complex concepts more accessible. Textbooks, on the other hand, often offer practice problems at the end of each chapter that reinforce the lessons taught throughout the book.
Online Tutoring and Forums can offer personalized assistance. Websites like Chegg, Tutor.com, and other tutoring services connect students with experienced educators who can provide explanations and help with difficult problems. Online forums, such as Reddit’s educational subreddits, are also great for discussing tough questions with peers and getting advice from others who may have struggled with similar topics.
Incorporating these additional materials into your study plan will help you build a strong foundation and approach the test with confidence.
How to Interpret Answer Key Results
Understanding the results from an answer key is an essential step in evaluating your performance and identifying areas for improvement. By carefully analyzing each response, you can gain insight into the types of mistakes made and better focus your study efforts. Interpreting these results effectively helps ensure that you address any gaps in knowledge before your next assessment.
Analyzing Correct and Incorrect Responses
Start by reviewing which questions you answered correctly and which you answered incorrectly. Pay attention to patterns that may emerge, such as consistent mistakes in specific topics or types of problems. This can help you pinpoint areas that need further review. For example, if you struggle with certain equation types, focus your next study sessions on mastering those particular concepts.
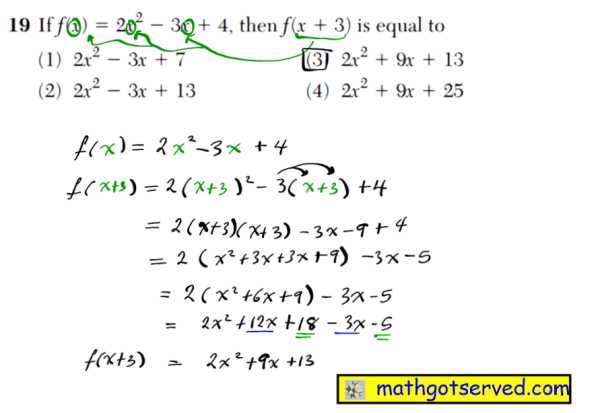
Understanding the Explanation Behind Each Solution
Most answer keys include explanations or step-by-step solutions. Even if you answered a question correctly, reviewing the solution process can help you understand the logic behind it and reinforce your approach. On the other hand, if you made an error, studying the solution steps will allow you to see where your reasoning went wrong and avoid repeating the same mistake in the future.
By taking the time to thoroughly interpret the results, you can maximize your learning and better prepare for upcoming assessments. Recognizing patterns in your mistakes and reviewing explanations will strengthen your problem-solving skills and improve your overall performance.
Post-Exam Tips and Next Steps
After completing any assessment, it’s important to reflect on your performance and plan your next steps. Whether you feel confident about your results or recognize areas where improvement is needed, taking proactive measures can help solidify your understanding and prepare you for future challenges. This phase is essential for reinforcing what you’ve learned and addressing any gaps in knowledge.
First, take some time to relax and clear your mind. It’s important to avoid overthinking the test immediately after it’s completed. Once you’ve had a break, start reviewing the material once again. Focus on the questions you found most challenging, and analyze the steps or concepts that led to mistakes. Understanding why a particular approach didn’t work will help prevent similar errors in the future.
If you felt unsure about certain topics, consider seeking additional help. This could be in the form of tutoring, peer study groups, or online resources. Reviewing past content with a fresh perspective can give you the chance to uncover nuances that might have been missed earlier. Use practice exercises to solidify your grasp of these areas, and regularly assess your progress to track improvements.
Finally, think ahead to your next academic steps. Reflect on the strategies that worked well during your preparation and consider how they might be applied to future exams or learning goals. Continue to refine your study habits and approach to problem-solving, and set clear, achievable goals for further growth.