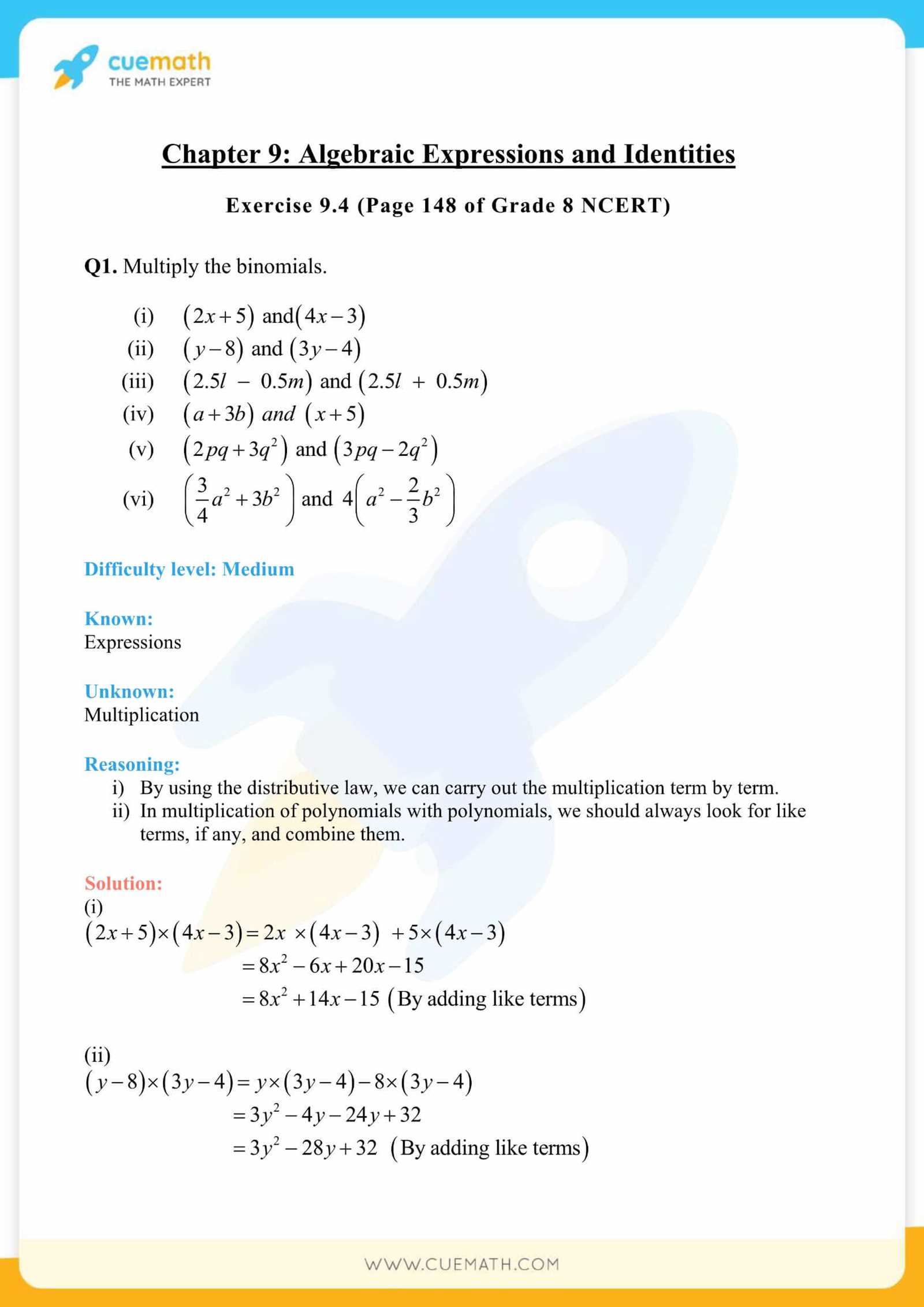
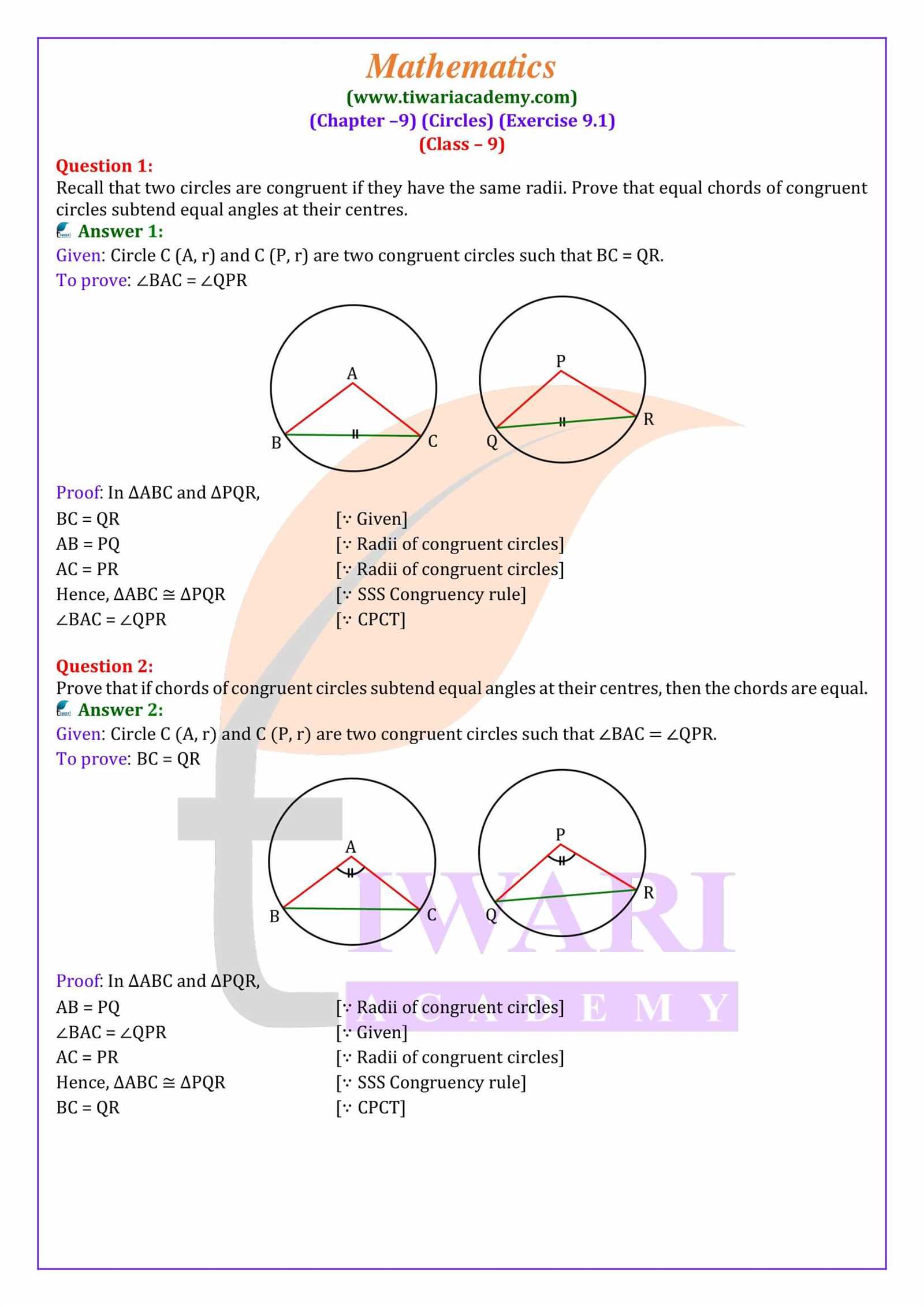
Algebra Connections Chapter 9 Answers and Solutions
In this section, we explore essential techniques for solving a range of mathematical problems. The focus is on applying various strategies to find solutions to complex equations and systems. These methods are designed to build a deeper understanding of mathematical principles and improve problem-solving skills.
Mastering core concepts plays a critical role in strengthening your ability to approach and solve different types of mathematical challenges. By understanding key principles, you’ll be equipped to navigate through intricate equations, inequalities, and geometric problems with confidence.
Through this guide, we break down each problem-solving method into manageable steps. Whether you’re working with polynomials, systems of equations, or quadratic expressions, this resource will provide the necessary tools to master the material and enhance your mathematical proficiency.
Mathematical Solutions and Techniques for Problem Solving
This section is dedicated to exploring methods for solving various types of mathematical problems. By breaking down complex concepts into simpler steps, it aims to provide clarity and improve understanding of key mathematical topics. The focus is on providing structured solutions that enhance problem-solving abilities and strengthen comprehension of important mathematical principles.
Step-by-Step Guide to Key Techniques
In this section, you’ll find a systematic approach to solving equations, inequalities, and other related mathematical problems. Each solution is broken down into manageable steps to ensure a clear understanding of the process. The techniques used here are designed to make problem-solving more intuitive and accessible for students at all levels.
- Understanding the structure of equations
- Breaking down complex problems into simpler parts
- Using substitution and elimination to solve systems
- Mastering polynomial factoring and quadratic methods
Common Mistakes and How to Avoid Them
As you work through different problems, it’s important to be aware of common mistakes that can arise. These errors, though simple, can lead to incorrect conclusions if not carefully avoided. This section highlights some frequent issues students encounter and offers practical tips to help prevent them.
- Confusing signs when simplifying expressions
- Misapplying formulas or methods in specific contexts
- Forgetting to check for extraneous solutions in inequalities
By applying the techniques and avoiding common pitfalls, you’ll improve your ability to solve a wide range of mathematical problems with confidence and accuracy.
Key Concepts in Chapter 9
This section focuses on the fundamental principles necessary for solving various mathematical problems. These concepts form the foundation for more advanced problem-solving strategies and help in simplifying complex equations and expressions. Understanding these core ideas is essential for mastering the material and progressing in mathematical studies.
Below is a summary of the key ideas explored in this section:
| Concept | Description |
|---|---|
| Solving Equations | Learn how to isolate variables and solve for unknowns in different types of equations. |
| Systems of Equations | Explore methods for solving systems of equations using substitution and elimination. |
| Quadratic Expressions | Understand how to work with quadratic expressions and solve using factoring or the quadratic formula. |
| Graphing Linear Functions | Master the technique of graphing linear equations and interpreting their solutions visually. |
| Polynomial Operations | Focus on adding, subtracting, multiplying, and factoring polynomials effectively. |
Grasping these essential concepts will allow for a more comprehensive understanding of advanced topics and provide the tools needed to tackle a variety of mathematical challenges.
Step-by-Step Problem Solutions

This section provides a detailed breakdown of solving various mathematical problems, guiding you through each step of the process. The goal is to make complex problems more manageable by focusing on the systematic approach needed to reach a solution. Following these steps will help you understand how to tackle different types of problems effectively.
Example 1: Solving a Linear Equation
Let’s solve a simple linear equation:
- Start with the given equation: 2x + 5 = 15
- Subtract 5 from both sides: 2x = 10
- Divide both sides by 2: x = 5
- The solution is x = 5
Example 2: Solving a Quadratic Equation
Now, let’s solve a quadratic equation using factoring:
- Start with the equation: x² – 5x + 6 = 0
- Factor the quadratic expression: (x – 2)(x – 3) = 0
- Set each factor equal to zero: x – 2 = 0 or x – 3 = 0
- Solve for x: x = 2 or x = 3
Example 3: Solving a System of Equations
Next, let’s solve a system of equations using the substitution method:
- Start with the system: y = 2x + 3 and x + y = 7
- Substitute y = 2x + 3 into the second equation: x + (2x + 3) = 7
- Simplify: 3x + 3 = 7
- Subtract 3 from both sides: 3x = 4
- Divide by 3: x = 4/3
- Substitute x = 4/3 back into the first equation to find y: y = 2(4/3) + 3 = 11/3
- The solution is x = 4/3 and y = 11/3
By following these detailed steps, you can break down complex problems into manageable parts and confidently arrive at the correct solution.
Understanding Algebraic Equations
This section focuses on the essential concepts behind solving equations that involve variables and constants. The goal is to help you understand how different terms in an equation interact with one another, and how to manipulate these relationships to find unknown values. By mastering these fundamental skills, you will be able to approach various types of problems with confidence.
At the core of solving equations is the concept of equality. Both sides of an equation must represent the same value, and the key to solving an equation is to isolate the variable on one side. This involves applying operations like addition, subtraction, multiplication, and division to simplify the equation while maintaining balance.
Understanding how to handle variables and constants, as well as how to perform the necessary operations on both sides of an equation, forms the foundation for more complex problem-solving techniques. Mastery of these concepts ensures that you can solve equations efficiently and accurately in a wide range of mathematical contexts.
Solving Linear Inequalities
This section focuses on the methods used to solve inequalities involving variables. The goal is to understand how to isolate the variable on one side while maintaining the inequality relationship between both sides. Solving these problems involves many of the same steps as solving equations, but with the added complexity of working with inequalities instead of equalities.
Linear inequalities differ from equations in that they express a range of possible solutions rather than a single value. These solutions can be represented as intervals on a number line. It’s important to remember that when multiplying or dividing by a negative number, the direction of the inequality sign must be reversed.
Here is a breakdown of the steps involved in solving a typical linear inequality:
| Step | Action |
|---|---|
| 1 | Identify the inequality and simplify the terms if necessary. |
| 2 | Isolate the variable by applying inverse operations (addition, subtraction, multiplication, or division). |
| 3 | If multiplying or dividing by a negative number, reverse the inequality sign. |
| 4 | Express the solution as an interval or on a number line. |
By following these steps, you can effectively solve linear inequalities and interpret the range of possible solutions. These techniques are applicable to a wide range of problems that involve inequality relationships.
Graphing Systems of Equations
This section explores the process of graphing systems of equations to find their solutions. A system consists of two or more equations that share common variables. The solution to the system is the point where the graphs of the equations intersect, which represents the values of the variables that satisfy all the equations simultaneously.
Graphing is a powerful method for visually understanding the relationship between equations and their solutions. By plotting each equation on the same coordinate plane, you can determine where the lines or curves meet. The point of intersection represents the values that satisfy both equations, providing a clear visual solution to the system.
To graph a system of linear equations, follow these basic steps:
- Rewrite each equation in slope-intercept form (y = mx + b) if necessary.
- Plot the y-intercept of each equation on the graph.
- Use the slope to determine additional points for each line.
- Draw the lines and identify the point where they intersect.
By following these steps, you can easily graph systems of equations and find their solutions. This method is particularly helpful in visualizing how changes in the equations affect the solutions.
Quadratic Equations in Chapter 9
This section delves into the study of equations where the highest power of the variable is squared. Quadratic equations often appear in many mathematical and real-world contexts, such as physics, engineering, and finance. Understanding how to solve these equations is crucial for progressing to more complex topics in mathematics.
Quadratic equations can typically be solved by factoring, completing the square, or using the quadratic formula. Each method offers a different approach depending on the specific form of the equation and the complexity of the terms involved. By mastering these techniques, you can solve a wide range of problems involving quadratic expressions.
Solving by Factoring
One common method for solving quadratic equations is factoring. This technique involves rewriting the equation as a product of two binomials and then solving for the variable. Here’s a general outline of the steps:
- Write the equation in standard form: ax² + bx + c = 0.
- Factor the quadratic expression.
- Set each factor equal to zero and solve for the variable.
Using the Quadratic Formula
If factoring is not feasible, the quadratic formula is another powerful tool for solving quadratic equations. The quadratic formula is:
x = (-b ± √(b² – 4ac)) / 2a
By substituting the values of a, b, and c from the equation into the formula, you can find the solutions, which may be real or complex numbers depending on the discriminant (b² – 4ac).
Understanding these methods and when to apply them will enable you to efficiently solve quadratic equations and handle more advanced problems in mathematics.
Factoring Polynomials Explained
Factoring polynomials involves breaking down complex expressions into simpler components, making it easier to solve equations or simplify mathematical problems. The goal is to express the polynomial as a product of factors, which can be further simplified or solved. This process is vital in algebra and is used in various applications across different fields of mathematics.
There are several methods to factor polynomials, depending on the structure of the expression. Some common techniques include factoring out the greatest common factor (GCF), factoring by grouping, and factoring trinomials. Mastery of these methods will provide a solid foundation for more advanced problem-solving tasks.
Factoring by Grouping
Factoring by grouping is a method used when a polynomial has four terms. The key idea is to group terms in pairs and factor each pair separately. Afterward, you can factor out the common binomial factor. Here’s an example of this method:
| Step | Action |
|---|---|
| 1 | Group the terms: ax + ay + bx + by |
| 2 | Factor out the common factors in each group: a(x + y) + b(x + y) |
| 3 | Factor out the common binomial: (x + y)(a + b) |
Factoring Trinomials
Factoring trinomials involves finding two binomials that multiply together to give the original expression. The general form of a trinomial is ax² + bx + c, and the goal is to find two numbers that multiply to ac and add up to b. Here’s how to factor a trinomial:
| Step | Action |
|---|---|
| 1 | Identify a, b, and c in the trinomial ax² + bx + c. |
| 2 | Find two numbers that multiply to ac and add to b. |
| 3 | Split the middle term bx using the two numbers found in the previous step. |
| 4 | Factor by grouping and simplify. |
Factoring polynomials is an essential skill in mathematics, helping to simplify expressions and solve equations more efficiently. By practicing these methods, you can handle increasingly complex problems with ease.
Identifying Functions and Their Properties
Understanding functions and their key characteristics is essential for solving many types of mathematical problems. A function establishes a relationship between a set of inputs and outputs, where each input corresponds to exactly one output. Recognizing this structure allows you to model real-world scenarios and analyze patterns effectively.
In this section, we explore how to identify functions, examine their properties, and understand how to represent them graphically. Key concepts include domain and range, behavior of functions, and identifying whether a given relation is a function. Additionally, we look at how the graphical representation of a function can help in recognizing its behavior, such as increasing, decreasing, or constant regions.
Key properties of functions include:
- Domain: The set of all possible input values for the function.
- Range: The set of all possible output values that result from applying the function to the domain.
- One-to-one relationship: Each input has exactly one corresponding output.
- Even and Odd Functions: Symmetry properties, with even functions having symmetry about the y-axis and odd functions about the origin.
By mastering these properties and how they apply to different types of relations, you’ll be able to identify functions more easily and work with them in both theoretical and applied contexts. Understanding these fundamental ideas is crucial for tackling more advanced mathematical topics.
Real-World Applications of Algebra
The ability to understand and manipulate mathematical relationships is essential for solving real-world problems across various fields. By using equations and functions, we can model situations, make predictions, and optimize processes. This section explores how these concepts are applied in everyday life and in professional fields such as engineering, economics, and technology.
Mathematical methods allow professionals to analyze patterns, calculate costs, predict future outcomes, and design solutions to complex problems. From determining the most cost-effective materials for construction to optimizing supply chains, the practical uses of these concepts are vast and diverse.
Some notable applications include:
- Finance: Budgeting, forecasting, and analyzing investments all rely on equations that model the behavior of financial systems.
- Engineering: Equations are used to design structures, analyze forces, and optimize systems for performance and safety.
- Technology: Algorithms, encryption, and software development often involve mathematical concepts that require a deep understanding of relationships between variables.
- Medicine: Modeling the growth of diseases, predicting patient outcomes, and understanding medical data all depend on mathematical techniques.
By applying these mathematical principles, professionals can solve real-life problems, improve efficiencies, and innovate in their respective fields. Understanding the role of mathematics in the world around us emphasizes its importance beyond the classroom and encourages its continued use in solving the challenges of the future.
Common Mistakes and How to Avoid Them
When working with mathematical concepts, it’s easy to make errors that can lead to incorrect solutions. Understanding common mistakes is key to improving accuracy and ensuring that problems are solved correctly. In this section, we explore frequent errors that students and professionals encounter, along with strategies to avoid them.
Often, mistakes arise from misinterpreting instructions, overlooking simple steps, or rushing through calculations. By recognizing these pitfalls, you can develop habits that minimize errors and lead to more reliable results. Whether it’s incorrect factoring, sign errors, or misunderstandings of mathematical properties, being aware of these common issues can significantly improve problem-solving efficiency.
Common Errors and How to Avoid Them
- Misinterpreting the Problem: Read each problem carefully and identify all components before attempting to solve it. Taking the time to understand the question can prevent errors in your approach.
- Incorrectly Applying Formulas: Double-check the formula you’re using to ensure it’s appropriate for the problem. Familiarize yourself with different formulae and when each should be applied.
- Sign Errors: A simple sign mistake can completely change the result. Always double-check your positive and negative signs during calculations.
- Forgetting to Simplify: After solving an equation, always simplify your answer. Leaving an equation in a complicated form may lead to confusion later on.
Effective Strategies for Avoiding Mistakes
By adopting a few best practices, you can minimize common mistakes. Always double-check your work, break problems into smaller, manageable steps, and ensure that you understand the underlying concepts before moving forward. Practicing regularly and reviewing solutions will also help reinforce your skills and reduce the likelihood of errors in future problems.
Understanding these common mistakes and being mindful of them will help you avoid pitfalls and develop more reliable problem-solving techniques, ultimately leading to greater success in mathematical tasks.
Tips for Mastering Algebraic Techniques
Mastering mathematical techniques requires a combination of practice, understanding, and strategy. Whether solving equations, factoring expressions, or working with functions, improving your skills is a gradual process that demands focus and persistence. This section provides essential tips that can help you enhance your ability to solve problems accurately and efficiently.
Success in mathematical problem-solving often comes down to building a strong foundation and applying strategic approaches. It’s important to be methodical and precise in your work, as small mistakes can lead to larger errors. Regular practice and understanding the logic behind techniques are key to mastering these skills over time.
Build a Strong Foundation
- Understand the Fundamentals: Before tackling advanced topics, ensure that you have a solid grasp of basic concepts. This foundational knowledge will make more complex problems easier to solve.
- Practice Regularly: Consistent practice is essential for improving your skills. The more problems you solve, the more proficient you become at identifying patterns and applying techniques effectively.
- Learn from Mistakes: Mistakes are part of the learning process. When you make an error, take the time to understand why it occurred and how to avoid it in the future.
Effective Problem-Solving Strategies
- Break Problems into Steps: Complex problems can often be solved more easily by breaking them down into smaller, manageable parts. Work through each step methodically and check your progress along the way.
- Use Visual Aids: Graphs, diagrams, and charts can be incredibly helpful in understanding relationships and visualizing solutions, especially when dealing with equations and functions.
- Master Different Techniques: Be versatile in your approach. Learn multiple methods for solving the same problem–whether through substitution, elimination, or factoring–as this will allow you to choose the most efficient method for any situation.
By following these strategies and committing to continuous improvement, you can strengthen your problem-solving abilities and master mathematical techniques. With time and effort, these skills will become second nature, allowing you to tackle increasingly complex challenges with confidence.
Understanding Exponents and Radicals
Exponents and radicals are essential concepts in mathematics that allow for the simplification and manipulation of expressions involving powers and roots. These two operations are closely related, yet they serve different purposes. Mastering how to work with them is crucial for solving more complex mathematical problems and understanding advanced techniques.
Exponents involve raising a number to a certain power, while radicals deal with extracting roots from numbers. Both operations have their own set of rules and properties that must be understood for effective problem-solving. Knowing how to manipulate these expressions efficiently can greatly improve your ability to tackle a wide range of mathematical tasks.
Working with Exponents
- Product Rule: When multiplying numbers with the same base, you can add the exponents. For example, am × an = am+n.
- Power of a Power Rule: To raise a power to another power, multiply the exponents. For example, (am)n = am×n.
- Zero Exponent Rule: Any non-zero number raised to the power of zero equals one. For example, a0 = 1.
- Negative Exponent Rule: A negative exponent indicates the reciprocal of the base raised to the positive exponent. For example, a-n = 1/an.
Understanding Radicals
- Square Roots: The most common radical is the square root, which is the inverse of squaring a number. For example, √a = b means b × b = a.
- Cube Roots: The cube root is the inverse of cubing a number. For example, ∛a = b means b × b × b = a.
- Rationalizing the Denominator: To simplify a fraction with a radical in the denominator, multiply both the numerator and denominator by the radical to eliminate it.
- Product Property of Radicals: The product of two radicals can be simplified as the radical of the product. For example, √a × √b = √(a × b).
Understanding the rules and properties of exponents and radicals is key to solving more complex problems in mathematics. By practicing these operations, you can improve your ability to simplify expressions and solve equations that involve powers and roots with ease.
Algebraic Word Problem Solutions
Solving word problems that involve mathematical expressions can be a challenging task, as they require translating real-life situations into algebraic equations. The key to solving these problems effectively is to break down the problem into manageable parts, identify the variables, and translate the text into an equation that can be solved step-by-step.
By understanding the language used in the problem, it becomes easier to identify relationships between quantities and formulate an equation. Once the equation is established, applying algebraic techniques, such as simplifying expressions, isolating variables, and solving for unknowns, can lead to the correct solution.
Step-by-Step Approach
- Read the Problem Carefully: Understand the scenario and identify what is being asked. Pay attention to key terms like “sum,” “difference,” “product,” and “quotient” as they indicate the mathematical operations involved.
- Define Variables: Assign variables to the unknowns in the problem. For example, let x represent the number of apples, or y the total cost.
- Translate into an Equation: Convert the words into a mathematical expression. For example, if the problem says, “The total cost is $5 more than three times the number of apples,” you can write the equation as 5 + 3x = total cost.
- Solve the Equation: Use algebraic methods to solve for the unknowns. This might involve simplifying the equation, isolating the variable, and performing operations to find the value of the variable.
- Check the Solution: After finding the solution, plug it back into the original word problem to ensure that it makes sense and satisfies all conditions.
Example Problem
Here’s an example of how to apply this approach:
Problem: A group of friends went to a movie. If the total cost of their tickets was $45 and each ticket costs $5, how many friends went to the movie?
- Step 1: Let x be the number of friends.
- Step 2: Translate the problem into an equation: 5x = 45.
- Step 3: Solve for x: x = 45 / 5 = 9.
- Step 4: Check the solution: If 9 friends went and each ticket costs $5, the total cost is indeed $45, so the solution is correct.
By following a structured approach, you can confidently solve algebraic word problems and apply these techniques to a wide range of real-life scenarios.
Using Algebra to Solve Geometry Problems
Mathematics often combines different branches, and geometry is no exception. Many geometry problems can be solved more efficiently by applying algebraic techniques. These problems usually involve finding unknown lengths, areas, or angles, which can often be expressed as equations. By recognizing the relationships between geometric properties and using algebraic methods, you can simplify complex problems and arrive at the solution more quickly.
In this section, we’ll explore how algebra can be used to solve geometric problems, such as finding unknown side lengths in triangles, determining areas of various shapes, and solving for angles in polygons. By combining both areas of math, you can develop a deeper understanding of both subjects and improve your problem-solving skills.
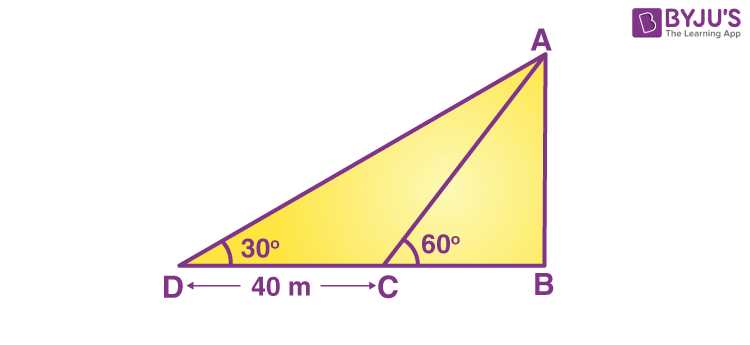
Example 1: Solving for a Side Length in a Triangle
Consider a right triangle where one leg has a length of 5 units, and the hypotenuse is 13 units. To find the length of the other leg, we can use the Pythagorean theorem, which states that a² + b² = c², where c is the hypotenuse and a and b are the legs.
- Step 1: Set up the equation: 5² + b² = 13².
- Step 2: Simplify: 25 + b² = 169.
- Step 3: Subtract 25 from both sides: b² = 144.
- Step 4: Solve for b: b = 12.
The length of the other leg is 12 units.
Example 2: Solving for the Area of a Rectangle
To find the area of a rectangle, we use the formula Area = length × width. If the length is expressed as 2x + 3 and the width is x – 1, we can set up the following equation:
- Step 1: Set up the equation: Area = (2x + 3)(x – 1).
- Step 2: Expand the expression: Area = 2x² – 2x + 3x – 3.
- Step 3: Simplify: Area = 2x² + x – 3.
Now the area of the rectangle is expressed as a quadratic equation. Depending on the value of x, you can substitute in numbers to find the exact area for a given length and width.
By using algebraic methods like equation solving and simplification, geometry problems become easier to approach and solve, providing you with a more powerful toolkit for tackling a wide range of mathematical challenges.
Reviewing Key Chapter 9 Strategies
In tackling complex mathematical problems, it’s crucial to employ effective strategies to ensure accuracy and efficiency. In this section, we’ll review essential methods that will help you approach and solve problems systematically. These strategies not only reinforce key concepts but also build a solid foundation for solving problems in a more structured manner. Whether it’s simplifying equations, working with functions, or graphing relationships, these techniques can be applied across a variety of problems.
1. Breaking Down Complex Problems
One of the most effective ways to solve difficult problems is by breaking them down into smaller, more manageable steps. By identifying the core components and focusing on solving each part separately, you can avoid feeling overwhelmed and make the problem more approachable.
- Step 1: Understand the problem’s requirements and what is being asked.
- Step 2: Identify the known values and the unknowns.
- Step 3: Choose the appropriate method or formula to apply.
- Step 4: Solve the smaller parts and then combine them to find the final solution.
2. Using Graphs for Visualizing Solutions
Graphs can be a powerful tool in understanding relationships between variables. Whether you’re dealing with linear, quadratic, or other functions, graphing can help you visualize how changes in one variable affect the other. This method is particularly useful when solving systems of equations or inequalities.
- Step 1: Plot the given equations on the same set of axes.
- Step 2: Look for the point of intersection, which often represents the solution to the system.
- Step 3: Use the graph to verify your algebraic solutions.
By incorporating these strategies, you can approach each problem with a clear plan, making it easier to find the solution and reinforce your understanding of the material.