Algebra Nation Section 4 Test Yourself Answers Guide

algebra nation section 4 test yourself answers

In this section, you’ll explore a variety of exercises designed to enhance your problem-solving abilities. The focus is on strengthening your foundational skills through hands-on practice, helping you gain confidence in tackling complex equations. By engaging with these challenges, you’ll sharpen your understanding of critical mathematical concepts and improve your analytical thinking.

Each problem offers an opportunity to apply your knowledge, test your comprehension, and learn from any mistakes. Whether you’re reviewing core principles or expanding on more advanced topics, the exercises provide the perfect balance of difficulty to foster growth. Through repetition and thoughtful reflection, you’ll develop a deeper understanding of how to approach mathematical problems with precision and ease.

By following this guide, you’ll be equipped with the tools needed to succeed in solving a wide range of problems. With careful attention to each step and consistent practice, you can strengthen your skills and build a solid foundation for future challenges.

Algebra Nation Section 4 Overview

This part of the platform focuses on strengthening essential problem-solving skills through structured exercises. The objective is to enhance your ability to understand, simplify, and solve a wide variety of mathematical challenges. Each task encourages deeper exploration of key concepts, ensuring a solid grasp of both foundational techniques and advanced methods.

Core Goals of This Section

Sharpening critical thinking skills through hands-on practice
Building a comprehensive understanding of mathematical principles
Developing problem-solving strategies for complex equations
Promoting accuracy and efficiency in calculations

How the Exercises Help You Grow

The exercises are designed to be progressively challenging, offering opportunities for improvement at every level. As you work through the problems, you’ll develop greater confidence in your ability to solve equations quickly and accurately. By continuously reflecting on your mistakes, you can refine your approach and learn how to handle more difficult problems with ease.

Through consistent practice, you’ll not only become proficient in solving specific types of problems but also improve your overall mathematical thinking. This section is built to support learners at every stage of their journey, helping you gain the skills and knowledge needed to excel in future tasks.

Understanding Key Algebra Concepts

Mastering the fundamental ideas behind mathematical equations is crucial for progressing to more complex topics. This part of the material is dedicated to exploring core principles that serve as the foundation for solving a wide range of problems. By focusing on these key concepts, you’ll improve your ability to analyze, simplify, and solve equations efficiently.

Core Ideas to Focus On

Variables and constants: Understanding how they interact in expressions
Operations and their properties: Addition, subtraction, multiplication, and division
Equations and inequalities: Learning how to balance both sides
Exponents and roots: Getting comfortable with powers and roots in equations

Building a Strong Foundation

As you explore these concepts, it’s important to strengthen your understanding through continuous practice. By practicing regularly, you’ll gain the confidence to handle more challenging problems in the future. Recognizing patterns, applying rules, and simplifying expressions will become second nature as you advance through the material.

Pay close attention to each concept, as they all build on one another. A solid grasp of the basics will not only improve your ability to solve equations but also help you think critically when approaching more advanced topics.

How to Approach Test Yourself Questions

Successfully tackling challenging problems requires more than just knowing the correct formula or procedure. It involves a strategic approach that helps break down complex issues into simpler, manageable parts. This section offers guidance on how to effectively approach each problem, ensuring that you maximize your understanding and accuracy while solving each equation.

Steps to Tackle Each Problem

Read carefully: Understand the problem statement and identify what is being asked.
Identify known and unknowns: Determine what values are provided and what needs to be solved.
Plan your approach: Decide on the best method to solve the problem, whether it’s through simplification, substitution, or balancing.
Work through step-by-step: Break down the solution into manageable parts and solve each one sequentially.
Check your work: Once you’ve solved, review your steps to ensure no errors were made during the process.

Common Techniques for Effective Problem Solving

Use of diagrams: Visualizing the problem can make it easier to comprehend and solve.
Substitution: Replacing variables with known values to simplify the equation.
Trial and error: Trying out different solutions to see which one fits best.
Factoring: Breaking down expressions into simpler components for easier calculation.

By following these steps, you can develop a systematic approach to solving problems. Consistent practice with this method will improve your efficiency and help you build confidence in your ability to tackle more advanced problems in the future.

Step-by-Step Solution Guide

Solving complex problems requires a structured approach that breaks down each task into manageable parts. This guide provides a clear framework for solving problems, helping you understand how to approach each step methodically. By following these steps, you can ensure that you not only arrive at the correct solution but also understand the underlying process.

Breakdown of Each Step

When faced with a challenging equation, follow these steps for an organized approach:

Step	Action	Explanation
1	Understand the problem	Read the problem carefully to identify what is being asked and what is known.
2	Set up the equation	Translate the problem into a mathematical form, using variables to represent unknowns.
3	Apply the correct operations	Decide on the method (substitution, elimination, etc.) based on the structure of the problem.
4	Simplify the equation	Combine like terms, eliminate unnecessary components, and make the equation easier to solve.
5	Solve for the unknown	Perform the necessary calculations to isolate the unknown variable.
6	Check your solution	Substitute your result back into the original equation to verify its correctness.

Key Considerations During the Process

Consistency: Follow each step carefully and avoid skipping any part of the process.
Accuracy: Double-check calculations at each stage to prevent errors.
Re-evaluate: If the result doesn’t make sense, review each step to identify potential mistakes.

By adhering to this step-by-step guide, you can approach even the most complex problems with confidence, ensuring both accuracy and understanding. Practice is key to mastering this method, so keep refining your process with each new problem you encounter.

Common Mistakes in Section 4

As you work through challenging mathematical problems, it’s easy to fall into certain pitfalls that can affect the accuracy of your solutions. Recognizing these common errors early on will help you improve your problem-solving skills and avoid unnecessary mistakes. By understanding where things typically go wrong, you can better focus your efforts and solve problems more efficiently.

Typical Errors to Watch Out For

Misreading the problem: Failing to fully understand the question can lead to solving the wrong problem or using incorrect methods.
Skipping steps: Rushing through the solution process or skipping intermediate steps can result in incorrect results.
Sign errors: Incorrectly handling positive and negative signs, especially when simplifying expressions or working with equations.
Incorrectly applying formulas: Using the wrong formula or applying it incorrectly can lead to mistakes in the final result.
Overlooking units or variables: Forgetting to account for all the variables or ignoring the units in word problems can skew results.

How to Avoid These Mistakes

Double-check your work: Before moving to the next step, verify that each calculation and assumption is correct.
Take your time: Resist the urge to rush. Break down each problem methodically and approach it step by step.
Practice regularly: The more problems you solve, the more you’ll develop the habits that prevent these common errors.
Review key concepts: Ensure that you’re comfortable with the fundamental principles behind each problem, so you can apply the correct strategies.

By staying aware of these frequent mistakes and adopting strategies to avoid them, you’ll significantly improve your ability to solve complex problems correctly. Consistent practice, attention to detail, and careful review will help you strengthen your mathematical skills over time.

How to Avoid Algebra Errors

Mathematical mistakes often arise from simple oversights or misunderstandings of core principles. These errors can be minimized by adopting a systematic approach to solving problems. Focusing on accuracy, clarity, and methodical execution is key to avoiding common pitfalls and improving problem-solving efficiency.

One of the most effective ways to avoid mistakes is to carefully follow each step of the process. Taking your time to fully understand the problem, verifying each calculation, and checking your work as you go will reduce the likelihood of making errors. Additionally, breaking complex problems into smaller, more manageable steps allows for easier tracking of your reasoning and calculations.

Another strategy is to regularly review basic concepts and techniques. Reinforcing your knowledge of mathematical rules, operations, and strategies ensures that you apply the correct methods consistently. Practice, too, plays a crucial role in honing your skills, as it helps you become familiar with common problem types and solutions, improving your accuracy over time.

Test Yourself: Practice Problems Explained

Engaging with practice problems is an essential part of mastering any mathematical concept. These problems provide an opportunity to apply learned skills, test your understanding, and identify areas where further improvement is needed. By breaking down each problem and carefully analyzing the steps, you can gain deeper insight into the methods required for solving similar challenges in the future.

When working through practice problems, it’s important to focus not just on finding the solution, but also on understanding the process behind it. Each problem has a logical flow that can be unraveled step by step. By practicing consistently, you develop both your analytical skills and your ability to approach problems efficiently.

In the following sections, we will explore how to break down and solve common problems, highlighting key steps and explaining the reasoning behind each one. This will give you a clearer understanding of how to tackle similar questions in the future with confidence and accuracy.

Breaking Down Complex Algebraic Problems

When faced with complex mathematical problems, it’s easy to feel overwhelmed. However, breaking the problem down into smaller, more manageable steps is key to solving it successfully. Understanding the structure of the problem and identifying the most efficient approach can help simplify even the most intricate equations. The goal is to deconstruct the problem, address each component individually, and then piece everything together for the final solution.

Steps to Simplify Complex Problems

Read the problem thoroughly: Carefully analyze the problem to identify what is being asked and which values are provided.
Break the equation into parts: If the problem involves multiple operations, divide it into smaller expressions or sub-problems.
Identify the key concepts: Recognize which techniques or formulas are needed to approach each part of the problem effectively.
Solve step-by-step: Tackle each component individually, applying the appropriate method for each step.
Check for errors: Once you’ve solved each part, double-check your work to ensure there are no mistakes.

Techniques for Managing Complex Problems

Substitution: Replace variables with known values to simplify the equation.
Factorization: Break down expressions into smaller factors to make the problem easier to handle.
Balancing: Use the process of balancing equations to isolate unknown values and solve them more effectively.
Graphical methods: Visualize the problem on a graph to gain a clearer understanding of the relationships between variables.

By adopting these strategies, you can approach even the most challenging problems with greater confidence and clarity. With practice, breaking down complex problems will become a more intuitive part of your problem-solving process.

Mastering Mathematics with Practice Exercises

One of the most effective ways to strengthen your mathematical skills is through continuous practice. By regularly engaging with exercises that challenge your understanding, you not only reinforce key concepts but also develop the problem-solving strategies necessary for tackling more difficult tasks. These exercises provide immediate feedback, allowing you to assess your progress and identify areas for improvement.

Why Practice Matters

Repetition and consistent exposure to different types of problems enable you to recognize patterns and refine your techniques. When you work through a series of exercises, you develop a deeper understanding of the underlying principles. Each problem becomes an opportunity to apply what you’ve learned, while simultaneously revealing areas where you may need more practice or clarification.

How to Maximize Practice Efficiency

Start with foundational problems: Begin with simpler problems to ensure a solid grasp of the basics before progressing to more complex tasks.
Focus on areas of difficulty: Identify specific topics that are challenging for you and dedicate extra time to mastering them.
Review your solutions: After solving a problem, go back and review each step to ensure you understand why each method works.
Track your progress: Keep a record of your performance to monitor improvements and identify any recurring issues that need attention.

By incorporating these strategies into your practice routine, you will gradually increase your proficiency and become more confident in solving complex mathematical problems. With time and dedication, mastery will follow.

Tips for Effective Problem Solving

Solving complex mathematical challenges requires more than just understanding formulas or methods. It involves a strategic approach that focuses on breaking down the problem, staying organized, and applying logical reasoning. By using effective problem-solving techniques, you can navigate even the most difficult problems with confidence and clarity.

Key Strategies for Success

Understand the problem: Take the time to read through the problem carefully and ensure that you understand what is being asked before you start solving it.
Plan your approach: Before jumping into calculations, decide on the most efficient method to solve the problem. This might involve choosing the right formula or identifying key steps.
Work step by step: Break the problem down into smaller, more manageable parts. Solve each part one at a time to avoid feeling overwhelmed.
Double-check your work: Once you’ve reached a solution, review your steps and calculations to ensure they are correct. This can help you catch any small errors before finalizing your answer.
Practice regularly: The more problems you solve, the better you’ll get at recognizing patterns and applying the right strategies efficiently. Make solving problems a habit to improve over time.

Overcoming Challenges

Stay patient: Don’t rush through problems. Take your time to understand each step and solve the problem methodically.
Ask for help: If you’re stuck, don’t hesitate to seek guidance from others, whether it’s a teacher, tutor, or peers. Sometimes a different perspective can offer valuable insights.
Learn from mistakes: If you make an error, treat it as an opportunity to learn. Understanding why you made a mistake will help you avoid repeating it in the future.

By incorporating these tips into your approach, you’ll enhance your problem-solving skills and develop the confidence needed to tackle challenging problems with ease.

Improving Speed and Accuracy

algebra nation section 4 test yourself answers

Enhancing both speed and accuracy in solving mathematical problems is essential for success. While accuracy ensures that your solutions are correct, speed allows you to solve problems more efficiently, making the most of your time. Achieving a balance between these two factors requires practice, focus, and the right strategies. With consistent effort, you can improve your performance and solve problems more quickly and correctly.

Strategies for Faster Problem Solving

Master fundamental techniques: The faster you can apply basic operations and formulas, the quicker you’ll be able to solve more complex problems. Focus on strengthening your foundational knowledge.
Practice mental math: Regularly practicing calculations in your head can significantly improve your speed and eliminate the need for paper and pencil in simpler problems.
Identify patterns: Recognizing common patterns in problems allows you to apply shortcuts or avoid unnecessary steps, which can save time.
Use time limits: Set time limits for yourself when practicing to encourage faster thinking and help you get used to working under pressure.

Ensuring Accuracy While Increasing Speed

Check your work periodically: Even when aiming to be faster, always make time for a quick review of your steps to avoid careless mistakes.
Understand the concepts: Having a solid understanding of the principles behind the problems you’re solving allows you to make fewer errors and solve them more confidently.
Work on reducing distractions: Focus is key for both speed and accuracy. Find a quiet, distraction-free environment where you can give your full attention to solving problems.
Practice regularly: The more problems you solve, the more efficient you’ll become. Set aside time each day to practice and challenge yourself with increasingly difficult tasks.

With focused practice and the right techniques, you can improve your ability to solve problems both quickly and accurately. Over time, these skills will become second nature, helping you to tackle even the most difficult problems with ease.

Time Management Techniques for Success

Effective time management is crucial for maximizing productivity and ensuring that tasks are completed efficiently. Whether you’re solving mathematical challenges or working on other projects, managing your time well can help you maintain focus, reduce stress, and improve overall performance. By implementing proven strategies, you can allocate your time wisely, avoid procrastination, and achieve better results in less time.

Essential Time Management Strategies

Set Clear Goals: Establishing clear, specific objectives helps prioritize tasks and ensures that your time is spent on what truly matters.
Break Tasks into Smaller Parts: Dividing complex tasks into smaller, more manageable segments makes them feel less overwhelming and allows you to track progress easily.
Use a Timer: Set a timer for each task to avoid spending too much time on any one problem or activity. This will help you stay focused and avoid distractions.
Avoid Multitasking: Multitasking can divide your attention and lower the quality of your work. Focus on one task at a time for better results.

Creating an Effective Schedule

One of the best ways to manage time effectively is by creating a structured schedule. By prioritizing tasks and allocating specific time slots for each one, you can prevent unnecessary delays and stay organized. Here’s a simple way to structure your time:

Task	Time Allotted	Priority
Study Key Concepts	30 minutes	High
Practice Problems	45 minutes	High
Review Mistakes	15 minutes	Medium
Relax and Recharge	20 minutes	Low

By following this structured approach, you’ll not only complete tasks more efficiently but also have time for breaks to recharge, which is essential for maintaining focus and preventing burnout.

With consistent practice of time management techniques, you’ll become more disciplined, productive, and confident in your ability to manage multiple tasks successfully, allowing you to achieve your goals more effectively.

Visualizing Algebraic Equations

Understanding mathematical relationships becomes easier when complex equations are visualized. Instead of relying solely on abstract symbols, graphical representations can provide a clearer understanding of how variables interact with each other. Visual tools, such as graphs, charts, and diagrams, help break down complex problems, making them more intuitive and accessible. By transforming equations into visual formats, you can gain insights into their behavior and identify patterns that may not be immediately apparent from the algebraic form alone.

The Power of Graphs

One of the most effective ways to visualize mathematical expressions is through graphing. A graph allows you to see the relationship between variables in a more concrete way. Here are some benefits of using graphs:

Identifying Trends: Graphs help you quickly identify whether a function is increasing, decreasing, or remains constant.
Solving Systems: Graphing systems of equations can provide an immediate visual solution by showing the point of intersection, which represents the solution to the system.
Understanding Shapes: Different types of equations produce different shapes on a graph, such as lines, parabolas, and circles, which can offer insight into their properties.

Using Diagrams for Problem-Solving

algebra nation section 4 test yourself answers

Another useful method is drawing diagrams or flowcharts to represent algebraic concepts. These can clarify steps in problem-solving and help visualize the relationships between different parts of the equation. For example:

Factorization: A diagram can help illustrate how a polynomial expression can be factored into simpler components, making it easier to solve.
Word Problems: Translating word problems into diagrams or visual models can help break down complex situations and make them easier to analyze.

By integrating visual tools into your approach, you not only simplify the process but also gain a deeper understanding of the relationships between numbers, which is essential for mastering mathematical concepts.

Using Graphs for Better Understanding

Graphs are powerful tools that transform abstract mathematical expressions into visual forms, making complex concepts easier to understand. By plotting equations on a graph, you can quickly observe how different variables interact with each other. Graphs provide a concrete representation of the relationships between numbers, helping to uncover patterns and trends that might otherwise be difficult to detect. This visual approach is particularly valuable for problem-solving and understanding the behavior of functions in various scenarios.

The Role of the Coordinate Plane

The coordinate plane serves as the foundation for graphing functions and equations. It allows you to map data points and visually track the relationship between input and output values. Key advantages of using the coordinate plane include:

Visualization of Solutions: Graphing equations helps identify solutions and intersections, making it easier to solve problems such as finding roots or solving systems of equations.
Instant Trend Recognition: A graph reveals whether a function is increasing, decreasing, or remains constant, providing immediate insight into its behavior.
Predicting Outcomes: By analyzing the graph of a function, you can predict future values or behaviors based on existing data.

Types of Graphs and Their Uses

Different types of graphs can be used to represent various kinds of equations. Some common types include:

Linear Graphs: These graphs represent straight lines and are used to show constant rates of change. They are useful for modeling relationships with a fixed slope.
Quadratic Graphs: Parabolic graphs are used to represent quadratic functions and are key for understanding motion, area problems, and more.
Circular and Exponential Graphs: These graphs help visualize more complex relationships, such as periodic behavior or exponential growth.

By incorporating graphs into your problem-solving toolkit, you gain a deeper understanding of mathematical relationships and can approach problems more effectively. Whether you are solving equations, analyzing data, or exploring new concepts, graphs provide an invaluable method for visualizing abstract ideas.

Understanding Algebraic Expressions

Mathematical expressions are the foundation of many complex problems and can represent a wide range of relationships. These expressions combine numbers, variables, and operations to form concise representations of mathematical ideas. Understanding how to read and manipulate these expressions is crucial for solving a variety of problems, from simple calculations to more advanced equations.

An expression typically includes variables (unknowns), constants (known values), and operators such as addition, subtraction, multiplication, or division. These components interact in specific ways according to the rules of mathematics, and understanding how to manipulate these elements is key to solving problems effectively.

Components of an Algebraic Expression

Algebraic expressions consist of several key components that work together to convey a specific relationship. These elements include:

Component	Description
Variables	Represent unknown values, often denoted by letters such as x, y, or z.
Constants	Fixed values that do not change, such as numbers (e.g., 3, -7, 5).
Operators	Mathematical symbols that indicate operations between terms, such as +, -, *, or /.
Terms	Parts of the expression separated by operators, which can include both constants and variables (e.g., 3x, -7).

How to Simplify Expressions

Simplifying algebraic expressions involves combining like terms and using the distributive property. Like terms are terms that share the same variable raised to the same power. By grouping like terms and performing the operations indicated by the expression, you can reduce the complexity of the expression.

Combining Like Terms: Add or subtract terms that have the same variable and exponent.
Distributive Property: Apply the distributive property to expand expressions, such as turning a(b + c) into ab + ac.
Factoring: Sometimes expressions can be factored into simpler terms for easier manipulation and solving.

Understanding how to break down and simplify these expressions is an essential skill in mathematics. By mastering the fundamental components and techniques of manipulating expressions, you will be able to approach more complex problems with confidence and clarity.

Simple Ways to Simplify Equations

Reducing the complexity of mathematical expressions is an essential step in solving equations efficiently. By applying basic principles and strategies, you can transform complicated equations into simpler forms, making it easier to find solutions. Whether you are dealing with linear equations or more advanced problems, these techniques are fundamental for streamlining the process.

Here are some effective methods to simplify equations:

1. Combine Like Terms

When an equation contains terms with the same variable and exponent, you can combine them to create a simpler expression. This process involves adding or subtracting the coefficients of the like terms.

Example: 3x + 2x = 5x
Example: 7y – 4y = 3y

2. Use the Distributive Property

To expand expressions or simplify ones that have parentheses, apply the distributive property. This involves multiplying each term inside the parentheses by the factor outside.

Example: 3(x + 4) = 3x + 12
Example: 2(5y – 3) = 10y – 6

3. Eliminate Fractions

algebra nation section 4 test yourself answers

Fractions can complicate solving an equation. You can eliminate them by multiplying both sides of the equation by the least common denominator (LCD) to make the equation easier to handle.

Example: (1/2)x = 4 becomes x = 8 when both sides are multiplied by 2.

4. Move Constants to One Side

For equations involving variables and constants, you can simplify by moving all constants to one side of the equation. This helps isolate the variable and makes the equation easier to solve.

Example: 3x + 5 = 11 becomes 3x = 6 after subtracting 5 from both sides.

5. Factor Common Terms

Factoring out the greatest common factor (GCF) from the terms in an equation can also make it simpler. This helps reduce the equation to a more manageable form.

Example: 6x + 9 can be factored as 3(2x + 3).

By using these basic strategies, you can significantly simplify equations, making them easier to solve and understand. Mastering these techniques will enhance your ability to approach more complex problems with confidence and efficiency.

Building a Strong Mathematical Foundation

Establishing a solid base in mathematics is key to mastering more advanced concepts. Whether you’re just beginning or refining your skills, understanding the fundamental principles provides the clarity and confidence needed to tackle complex problems. A strong foundation allows you to build on what you know, enabling you to approach more difficult topics with ease.

1. Mastering Basic Operations

The first step in mastering any field of mathematics is to become proficient in basic arithmetic operations. Addition, subtraction, multiplication, and division are the building blocks of all future work. These skills are essential for solving more intricate problems.

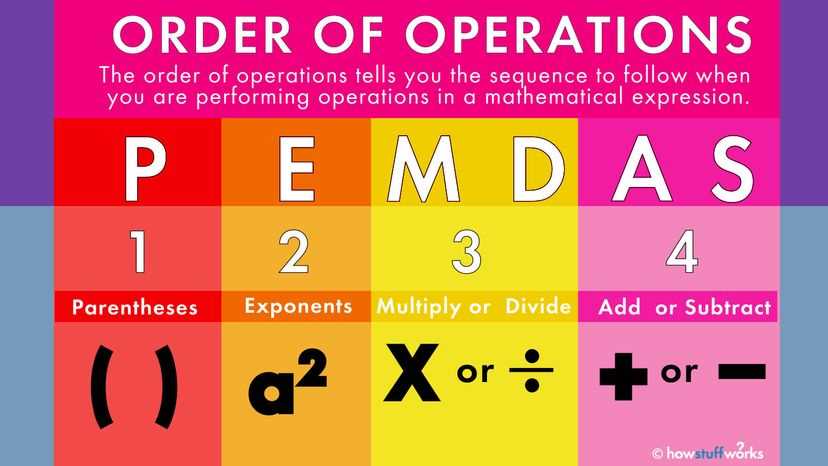
Understanding the order of operations (PEMDAS) helps ensure that calculations are done in the correct sequence.
Recognizing patterns in numbers and operations strengthens problem-solving abilities.

2. Developing Problem-Solving Strategies

Effective problem-solving involves breaking down complex problems into smaller, more manageable parts. Using systematic approaches to solve equations helps avoid errors and improve accuracy. Here’s a simple strategy to follow:

Step	Description
1	Understand the problem by carefully reading the instructions and identifying key information.
2	Plan the approach by deciding which methods or strategies to apply.
3	Execute the plan step by step, checking each stage for accuracy.
4	Review the solution and ensure it makes sense in the context of the problem.

Following these steps consistently improves efficiency and reduces mistakes, building confidence in mathematical reasoning.

By consistently practicing these foundational skills, you’ll not only gain fluency in solving problems but also develop the flexibility to approach various types of mathematical challenges. With a strong base, you’ll find yourself prepared to tackle more advanced topics with greater ease and success.

Essential Skills for Mastery

Achieving proficiency in mathematical problem-solving requires a combination of foundational knowledge and critical thinking skills. The ability to work through equations, recognize patterns, and apply logical reasoning are fundamental components of mathematical mastery. By honing these essential skills, learners can confidently approach more advanced topics and solve complex problems with ease.

The key to success lies in understanding the core principles and consistently practicing the techniques that will help solidify these concepts. Mastering the following skills is crucial for building a strong mathematical foundation:

Understanding Variables and Expressions: Recognizing how variables represent unknown quantities is the first step in translating real-world problems into solvable equations.
Simplifying Equations: Mastering the art of simplifying expressions by combining like terms and applying basic operations is essential for making problems more manageable.
Solving for Unknowns: The ability to isolate variables and solve for unknowns is the cornerstone of most mathematical problems, whether it’s through basic operations or more complex methods.
Recognizing Patterns: Identifying and understanding numerical patterns or relationships helps build strategies for solving problems faster and more accurately.

By developing these skills, learners can approach problems more strategically, break down complex tasks into simpler steps, and improve their accuracy in finding solutions. Mastery of these skills lays the groundwork for tackling more advanced mathematical challenges with confidence and precision.