Algebra Nation Workbook Answers Section 1

Understanding key mathematical principles is essential for building a strong foundation in problem-solving. This segment focuses on the core techniques and strategies that will help you confidently approach various challenges. By breaking down complex topics into manageable steps, you will gain a deeper understanding of the material.

The exercises in this part emphasize critical thinking and applying learned skills to solve practical problems. With clear explanations and step-by-step guidance, you’ll learn how to manipulate variables, simplify expressions, and find solutions efficiently. Mastering these fundamentals will not only enhance your mathematical abilities but also prepare you for more advanced topics in the future.

Whether you’re reviewing the material for the first time or reinforcing existing knowledge, this section offers valuable insights. Consistent practice and comprehension of these principles are the keys to becoming proficient in solving equations and tackling mathematical tasks with confidence.

Algebra Nation Workbook Section 1 Answers

This part of the material focuses on fundamental problem-solving techniques that serve as the basis for more complex challenges. It provides a solid understanding of how to approach different types of equations and expressions. The goal is to ensure mastery of essential skills that will support future learning in this subject.

Key Concepts to Master

• Understanding variables and constants
• Manipulating expressions with addition and subtraction
• Solving linear equations with one variable
• Applying the order of operations for correct results
• Identifying and avoiding common mistakes in calculations

Steps to Solve Problems Effectively

1. Start by identifying the given information in each problem.
2. Use the appropriate mathematical operations to simplify the equation.
3. Apply the correct steps to isolate the variable on one side of the equation.
4. Check your solution by substituting it back into the original equation.
5. Review the process to ensure no mistakes were made during calculations.

By following these methods, you will be able to tackle a variety of problems with ease, building confidence in your skills as you progress through the material.

Understanding the Basics of Algebra

Grasping the foundational principles of mathematics is crucial for solving a wide range of problems. This section introduces essential concepts that serve as the building blocks for more advanced topics. By mastering these basics, you’ll develop the skills needed to tackle more complex equations and functions with confidence.

Key Principles to Understand

• Variables represent unknown quantities in an equation.
• Constants are fixed values that do not change.
• Expressions combine numbers, variables, and operations.
• Equations show relationships between two expressions.
• Operations such as addition, subtraction, multiplication, and division form the core tools for solving problems.

Steps to Approach Mathematical Problems

1. Identify the variables and constants involved in the problem.
2. Analyze the equation or expression to determine the operation needed.
3. Isolate the variable by performing inverse operations.
4. Verify the solution by plugging it back into the original equation.

Once these basic concepts are clear, you can move on to solving more advanced problems with greater ease. The key is consistent practice and understanding how each element of a problem interacts with the others.

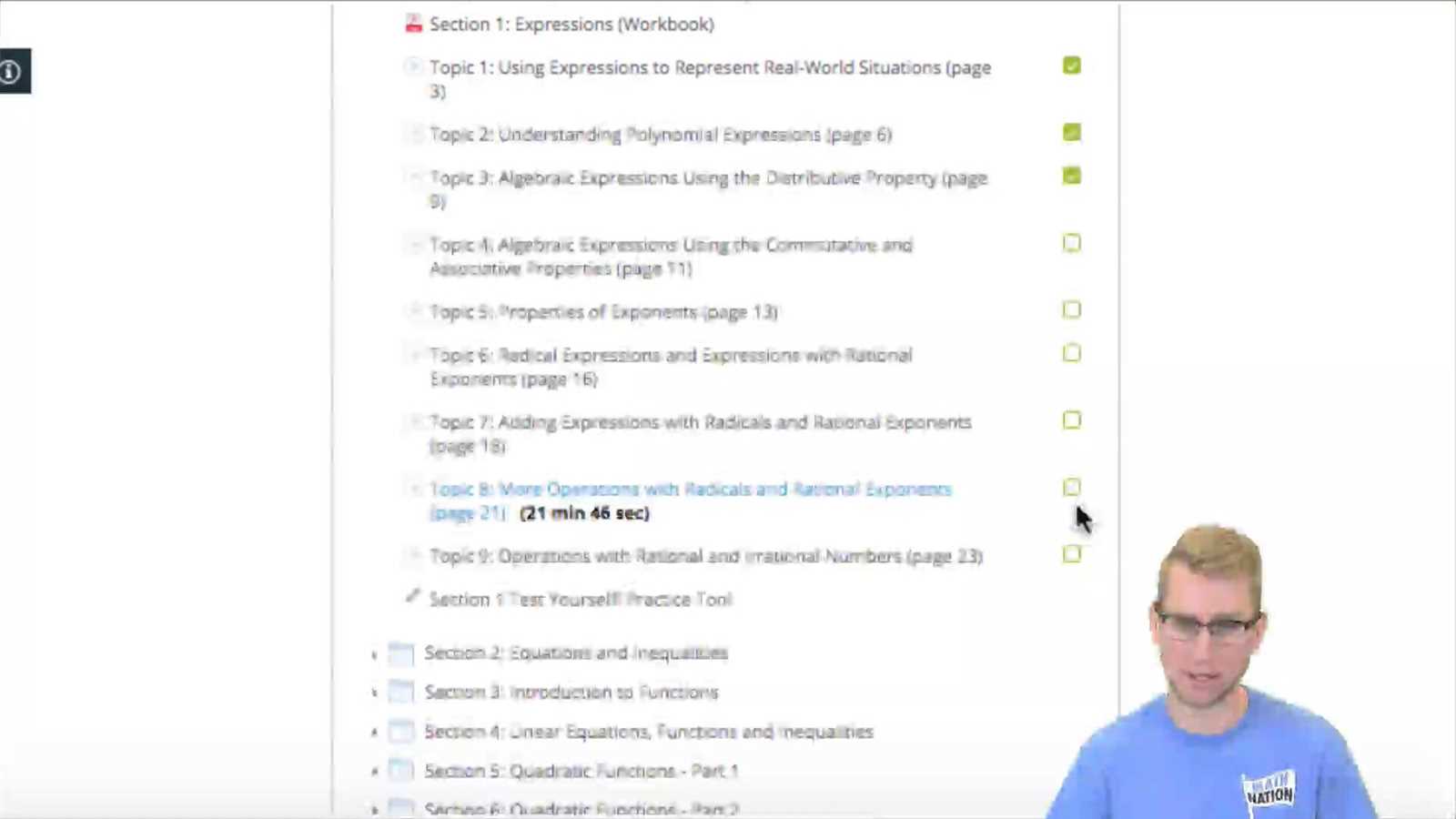
Key Concepts Covered in Section 1

This part of the material emphasizes the foundational concepts that are crucial for understanding more advanced topics. By focusing on these essential elements, you will gain a strong grasp of the basic techniques used to solve mathematical problems effectively. These concepts will serve as a stepping stone toward mastering more complex challenges.

Core Topics to Focus On

• Understanding the relationship between variables and constants
• Recognizing the importance of simplifying expressions
• Solving linear equations and isolating the unknown
• Using the correct order of operations to ensure accurate results
• Identifying different types of problems and applying appropriate methods

Techniques for Problem Solving

• Breaking down complex problems into simpler parts
• Performing operations systematically to find solutions
• Checking your work to avoid common mistakes
• Applying learned methods to a variety of problem types

Mastering these key concepts is essential for building a strong mathematical foundation. Once you have a solid understanding, you will be able to approach increasingly complex problems with confidence.

Step-by-Step Solutions for Exercises

This section provides clear, detailed instructions for solving a variety of mathematical problems. By breaking down each problem into manageable steps, you can develop a deeper understanding of the processes involved and learn how to apply these methods to similar challenges. The step-by-step approach ensures that no aspect of the problem is overlooked, giving you a comprehensive guide to finding the correct solution.

Breaking Down the Process

• Start by identifying the given information and what is being asked.
• Organize the equation or expression to clearly see the relationships between variables.
• Apply the appropriate mathematical operations in the correct order.
• Perform calculations methodically to avoid errors.
• Review each step to ensure accuracy and consistency.

Example Walkthroughs

Each exercise is accompanied by a detailed example that demonstrates how to approach and solve the problem from start to finish. These examples serve as a guide for replicating the steps on similar exercises, reinforcing key concepts along the way.

By practicing with these step-by-step solutions, you will build the confidence needed to handle more complex tasks and refine your problem-solving skills.

How to Approach Algebra Problems

When faced with mathematical challenges, having a clear strategy is essential for finding the correct solution. Approaching each problem with a step-by-step method helps ensure that nothing is overlooked and that you can work through even complex tasks efficiently. By breaking down problems into smaller parts and understanding each component, you can tackle them with confidence.

Steps for Solving Problems Effectively

1. Identify the unknowns and what is being asked.
2. Write down the given information and organize it clearly.
3. Look for relationships between variables and constants.
4. Determine the operations needed to simplify or solve the equation.
5. Apply the operations in the correct order to isolate the variable.
6. Check your final solution by substituting it back into the original equation.

Common Approaches to Use

• Start with basic operations like addition, subtraction, multiplication, or division.
• Use inverse operations to isolate unknowns.
• Simplify expressions step by step to avoid errors.
• Look for patterns or similar problems to apply known techniques.
• Stay organized and avoid skipping steps in the process.

By following these guidelines, you can approach any problem systematically and increase your chances of success. Consistent practice with these methods will help build your problem-solving skills and boost your confidence in tackling even more challenging tasks.

Common Mistakes to Avoid in Algebra

Even the most experienced learners can make mistakes when solving mathematical problems. However, understanding common errors can help you avoid them and improve your accuracy. Paying attention to details and following each step carefully is crucial to ensure you arrive at the correct solution.

Frequent Errors to Watch Out For

• Incorrectly applying the order of operations: Always follow the correct sequence (PEMDAS) to avoid mistakes in simplifying expressions.
• Forgetting to distribute: When dealing with parentheses, ensure that all terms are properly multiplied or divided.
• Mismanaging negative signs: Double-check your signs when adding or subtracting negative numbers.
• Skipping steps: Skipping steps can lead to small errors that compound and affect the final result.
• Not checking your solution: Always substitute your answer back into the original equation to verify that it works.

Tips for Avoiding Mistakes

• Double-check each step: After performing any operation, review it before moving on.
• Write everything down clearly: Keeping your work organized reduces the chance of errors.
• Take your time: Rushing through problems can lead to simple mistakes. Patience and precision are key.
• Practice regularly: The more problems you solve, the more familiar you become with common pitfalls.

By being mindful of these common mistakes and following the tips provided, you’ll be able to improve your problem-solving abilities and avoid errors that can affect your results.

Tips for Effective Algebra Learning

Mastering mathematical concepts requires not only understanding the theory but also practicing regularly and applying the techniques learned. To achieve proficiency, it is important to develop a systematic approach to learning and problem-solving. These tips will help you optimize your study habits and improve your overall performance.

Strategies for Successful Learning

• Practice consistently: Regular practice reinforces concepts and improves your ability to solve problems quickly and accurately.
• Understand the concepts: Focus on understanding the underlying principles rather than just memorizing formulas. This deeper understanding will make solving problems easier and more intuitive.
• Break down complex problems: Divide difficult problems into smaller, manageable parts. Solve each part step by step to avoid feeling overwhelmed.
• Ask for help when needed: Don’t hesitate to reach out to teachers, tutors, or classmates when you’re struggling. Clarifying doubts early on can save time in the long run.

Effective Study Habits

• Stay organized: Keep your notes and practice problems in order so you can easily review them when needed.
• Focus on understanding mistakes: Learn from the errors you make. Identify the reasons behind them and avoid repeating them in the future.
• Set achievable goals: Break your learning into smaller, achievable goals and celebrate each milestone to stay motivated.
• Use resources wisely: Utilize textbooks, online resources, and educational videos to enhance your learning.

By incorporating these strategies into your study routine, you’ll be able to strengthen your understanding and build confidence in solving various mathematical challenges.

How Algebra Nation Helps Students

This platform provides valuable resources that support students in mastering mathematical concepts. By offering interactive tools, practice exercises, and structured lessons, it helps learners of all levels gain a deeper understanding and improve their problem-solving abilities. The platform is designed to guide students through each topic systematically, ensuring that they can build their knowledge progressively.

Interactive Learning Tools

One of the key features of this platform is its interactive tools, which allow students to engage directly with the material. These tools offer real-time feedback, helping students identify areas of weakness and correct mistakes quickly. By practicing with interactive exercises, learners gain hands-on experience that enhances their understanding and retention of the concepts.

Structured Lessons and Support

The platform offers well-organized lessons that break down complex ideas into manageable steps. It provides clear explanations, examples, and tips, making learning accessible for every student. Additionally, students can review their progress and track improvements, which encourages continued learning and helps them stay motivated.

Overall, this resource is designed to make the learning process more engaging and effective, empowering students to confidently tackle mathematical challenges and achieve their academic goals.

Mastering Variables and Expressions

Understanding variables and expressions is a fundamental skill in solving mathematical problems. These components allow for the representation of unknown values and the relationships between them. Mastering this concept is crucial for progressing in various mathematical fields, as it forms the basis for more complex equations and problem-solving techniques.

Variables are symbols used to stand for unknown or changeable values, while expressions are combinations of variables, constants, and operations. To excel in this area, it’s important to learn how to manipulate these elements and understand how they interact in different contexts.

Key Concepts of Variables and Expressions

Term	Description	Example
Variable	A symbol that represents an unknown value	x, y, z
Constant	A fixed number that does not change	5, -3, 10
Expression	A combination of variables, constants, and operations	3x + 2
Coefficient	A number multiplied by a variable	4 in 4x

By learning how to recognize and work with these components, students can improve their ability to simplify expressions, solve for variables, and apply these concepts in real-world situations. The more practice you get, the more intuitive the process becomes.

Importance of Order of Operations

The order in which mathematical operations are performed plays a critical role in obtaining the correct result. Without a standard procedure, different people could interpret and solve the same problem in different ways, leading to inconsistent answers. Understanding and applying the correct sequence ensures that mathematical expressions are simplified properly and consistently.

In order to avoid errors and confusion, a specific hierarchy of operations has been established. This hierarchy dictates the order in which various operations–such as addition, subtraction, multiplication, division, and others–should be carried out. Mastery of this concept is essential for solving problems accurately, particularly when dealing with complex expressions.

The Correct Sequence to Follow

• Parentheses: Operations inside parentheses should always be performed first.
• Exponents: Next, calculate powers and roots.
• Multiplication and Division: These operations come next, performed from left to right.
• Addition and Subtraction: Finally, addition and subtraction are carried out, also from left to right.

Example: In the expression 2 + 3 × (4 + 5), the operations inside the parentheses are done first, followed by multiplication, and then addition. This results in 2 + 3 × 9 = 2 + 27 = 29, not 27.

By adhering to this order, students can ensure their calculations are accurate, avoiding mistakes that can arise from misinterpreting the sequence of operations.

Solving Linear Equations with Examples

Linear equations are among the most fundamental types of equations in mathematics. These equations involve variables that are raised to the first power and have constants added or subtracted. Solving these equations involves finding the value of the variable that makes the equation true. This is typically done by isolating the variable on one side of the equation through a series of logical steps.

The goal when solving such an equation is to manipulate it step by step until the variable is isolated. This process often includes operations like addition, subtraction, multiplication, and division, all while ensuring the equation remains balanced. Here, we will go through a few examples to demonstrate how to solve these types of problems effectively.

Example 1: Simple Linear Equation

Consider the equation 2x + 5 = 15. To solve for x, follow these steps:

• Step 1: Subtract 5 from both sides: 2x = 15 – 5 → 2x = 10.
• Step 2: Divide both sides by 2: x = 10 ÷ 2 → x = 5.

The solution to this equation is x = 5.

Example 2: Equation with Fractions

Now, let’s solve a more complex equation: 3/4x – 2 = 10. To solve this equation:

• Step 1: Add 2 to both sides: 3/4x = 10 + 2 → 3/4x = 12.
• Step 2: Multiply both sides by 4 to eliminate the fraction: 4 × 3/4x = 4 × 12 → 3x = 48.
• Step 3: Divide both sides by 3: x = 48 ÷ 3 → x = 16.

The solution to this equation is x = 16.

Through these examples, we see that solving linear equations involves clear, logical steps that gradually isolate the variable. With practice, these steps become second nature and the process becomes faster and more intuitive.

Graphing and Interpreting Algebraic Equations

Graphing equations is a visual way to represent mathematical relationships. By plotting points on a coordinate plane, we can easily observe how different variables relate to each other. This process allows for a deeper understanding of the equation’s behavior and makes solving and interpreting the relationship between variables more intuitive. Once an equation is graphed, it provides valuable insights into the slope, intercepts, and overall trends of the relationship.

Graphing helps transform abstract expressions into concrete, visual representations that can be analyzed and interpreted. It enables us to quickly identify key features of the equation, such as where the line crosses the axes, whether the slope is positive or negative, and how the variables interact over a range of values.

Example 1: Graphing a Simple Linear Equation

Consider the equation y = 2x + 3. This is a straight line equation, and its graph can be constructed by plotting points that satisfy the equation.

x	y
-1	1
0	3
1	5
2	7

Plotting these points on a graph and connecting them will yield a straight line. The slope of the line is 2, meaning for every unit increase in x, the value of y increases by 2. The y-intercept is 3, indicating the point where the line crosses the y-axis.

Interpreting the Graph

Once the graph is drawn, interpretation involves examining the slope and intercepts. In this case, the positive slope indicates an increasing relationship between x and y. The y-intercept tells us where the line crosses the y-axis, giving us information about the starting value of y when x is zero.

Graphing provides a powerful way to visualize and interpret relationships, making complex equations easier to understand and analyze. By learning how to graph and interpret these expressions, students can gain deeper insights into mathematical patterns and concepts.

Practice Problems for Skill Enhancement

To solidify understanding and improve proficiency, practicing a variety of problems is crucial. This process helps reinforce key concepts, boosts problem-solving abilities, and fosters greater confidence in tackling new challenges. Regular practice allows for identifying weaknesses and provides the opportunity to strengthen foundational skills.

Engaging with a range of exercises enhances both speed and accuracy, ensuring that essential techniques become second nature. Below are several practice problems designed to improve different aspects of mathematical skills:

Problem Set 1: Basic Operations with Variables

• Simplify the expression: 3x + 4x – 5
• Solve for x: 2x – 5 = 11
• Expand the expression: (x + 2)(x – 3)

Problem Set 2: Solving Linear Equations

• Solve for x: 4(x + 2) = 20
• Solve for y: 3y – 7 = 2y + 4
• Solve for x: 5x – 2 = 3x + 8

Problem Set 3: Graphing Linear Equations

• Graph the equation: y = 2x + 1
• Plot the points that satisfy: y = -x + 4
• Graph and interpret the equation: y = 3x – 5

These exercises focus on developing fundamental skills that are essential for mastering more complex concepts. By working through these problems, you’ll gain a deeper understanding of the principles and be better prepared for advanced topics.

For even greater improvement, try creating your own problems and challenge yourself with variations on the ones provided. The more practice you get, the more fluent you’ll become in handling similar tasks in the future.

Using Algebra Nation Resources Effectively

To maximize learning outcomes, it’s essential to utilize available resources in a strategic and focused manner. These tools are designed to support students in building strong mathematical foundations, helping them master core concepts and practice essential skills. Effective use of these resources ensures steady progress and a deeper understanding of the material.

Start with the Basics: Begin by exploring the introductory materials and foundational lessons. These resources break down complex topics into manageable sections, making it easier to grasp essential principles. Whether through video tutorials or practice problems, starting from the basics gives you a solid footing before advancing to more challenging content.

Use Interactive Features: Many platforms offer interactive quizzes and exercises that provide immediate feedback. This real-time assessment allows learners to identify areas that need improvement and focus their efforts accordingly. Regularly engaging with these activities helps reinforce understanding and correct mistakes as they arise.

Set a Routine: Consistency is key when using online resources. Establish a regular study schedule, dedicating specific times to working through problems and reviewing concepts. This discipline ensures steady progress and prevents the material from becoming overwhelming.

Focus on Weak Areas: Identify the topics that are most challenging and focus extra time on these areas. Some resources allow for targeted exercises based on your progress, helping you tackle difficult concepts head-on. Focusing on your weak points allows you to turn them into strengths.

Collaborate with Peers: If available, use discussion forums or collaborative tools to ask questions and share insights. Learning from others’ perspectives can provide valuable solutions to problems and deepen your understanding. Explaining concepts to peers also reinforces your own knowledge.

Track Your Progress: Many learning platforms offer progress tracking features. Regularly check your progress to ensure that you are staying on track and meeting your learning goals. Reflect on your performance and adjust your study methods as necessary to continue improving.

By combining these strategies, you can make the most out of the available resources, enhancing your ability to solve problems efficiently and confidently.

Review and Reinforce Key Algebra Skills

To solidify your understanding of key mathematical concepts, regular review and practice are essential. Reinforcing foundational skills ensures long-term retention and builds confidence in solving a variety of problems. By revisiting and strengthening these core skills, students are better prepared to tackle more complex challenges and advance their mathematical abilities.

Effective Strategies for Reinforcement

Several methods can be employed to review and reinforce essential skills. Here are some approaches that have proven to be effective:

• Practice with Purpose: Consistently work through exercises that focus on areas you find most challenging. Regular practice helps solidify understanding and improve problem-solving speed.
• Utilize Worked Examples: Reviewing solved examples can help you understand the problem-solving process and apply it to similar problems on your own.
• Break Down Complex Problems: Start by breaking down more complex problems into smaller, more manageable steps. This strategy helps prevent feeling overwhelmed and ensures you understand each stage of the process.
• Review Mistakes: Mistakes offer valuable learning opportunities. Analyzing errors helps identify common misunderstandings and gives you the chance to correct them before moving on.

Tracking Your Progress

It’s important to track your progress to see how well you’re reinforcing the concepts. Keep a record of exercises completed, note areas that require additional review, and celebrate your successes along the way. The following table can be used to track your reinforcement efforts:

Topic	Completed Exercises	Difficulty Level	Notes for Review
Linear Equations	10	Moderate	Review solving methods for fractions
Exponents	8	Easy	Need more practice with negative exponents
Word Problems	5	Challenging	Focus on translating word problems into equations

By employing these strategies and tracking your progress, you will continuously improve your skills and become more adept at solving mathematical problems with confidence and accuracy.

Preparing for Exams with Section 1

Effective preparation is key to mastering mathematical concepts and performing well on exams. Section 1 provides a solid foundation for understanding the basics, offering essential tools and strategies to help you succeed. By focusing on the material presented in this section, students can strengthen their problem-solving abilities and build confidence in their exam performance.

Identifying Key Topics for Review

Before diving into intense study sessions, it’s important to identify which concepts are most likely to appear on the exam. Focus your efforts on mastering the topics that form the core of the subject matter. Key areas often include:

• Equations and Variables: Understand how to manipulate variables and solve for unknowns.
• Operations and Functions: Be able to perform operations such as addition, subtraction, multiplication, and division on expressions.
• Graphing Techniques: Practice plotting graphs and interpreting data points.
• Word Problems: Learn how to translate real-world scenarios into mathematical equations.

Study Tips for Success

Maximize your study time by following these proven strategies:

• Focus on Understanding: Instead of memorizing formulas, focus on understanding the underlying concepts. This will help you solve problems more easily during the exam.
• Use Practice Exercises: Completing practice exercises from this section allows you to familiarize yourself with exam-style questions and identify areas that need improvement.
• Time Yourself: Simulate exam conditions by timing yourself while solving problems. This will help improve your speed and efficiency.
• Seek Help if Needed: Don’t hesitate to ask for clarification or additional resources if you’re struggling with specific concepts.

By focusing on these strategies and reviewing the core material in Section 1, students can effectively prepare for their exams, improve their problem-solving skills, and approach the test with confidence.