Algebra Nation Workbook Answers Section 2 Explained
In this part of the learning material, you will explore critical mathematical principles designed to strengthen your understanding of various topics. The exercises are aimed at helping you build a solid foundation by focusing on problem-solving techniques and logical reasoning. The key focus is on improving your ability to tackle different types of challenges with confidence and accuracy.
Throughout this section, you will encounter various types of problems that test your grasp of fundamental concepts. By engaging with these tasks, you will develop a better understanding of how to approach mathematical problems and sharpen your critical thinking skills. The solutions provided will guide you step by step, offering insights into the most effective strategies for solving complex exercises.
Mathematical Practice and Solutions
This part of the learning material focuses on strengthening essential mathematical skills through a series of well-structured problems. The goal is to enhance your ability to solve various challenges by applying logical reasoning and systematic methods. By working through these exercises, you will build confidence in tackling increasingly complex problems.
Each problem in this section is designed to help you understand and apply key principles, improving your problem-solving strategies. The detailed steps included in the solutions will guide you through each challenge, showing you the most efficient way to reach the correct answers. By following these processes, you’ll refine your skills and increase your accuracy.
- Problem-solving techniques for real-world applications
- Step-by-step guidance for each exercise
- Identifying common mistakes and how to avoid them
- Practical strategies for mastering mathematical tasks
As you progress through the exercises, remember to practice regularly. With consistent effort, you’ll notice improvement not only in your understanding of key topics but also in your ability to approach new challenges with ease.
Understanding Key Mathematical Concepts
Grasping the fundamental principles of mathematics is essential for solving complex problems with ease. This part of the learning process is dedicated to deepening your understanding of core concepts, providing you with the tools to approach various tasks confidently. Mastering these building blocks will make tackling advanced topics much more manageable and intuitive.
Core Principles for Problem Solving

By focusing on basic rules and methods, you will learn how to break down problems into smaller, more manageable steps. Understanding how to apply formulas, manipulate variables, and work through equations is the foundation of successful problem-solving. As you practice, you’ll develop a strong ability to analyze and solve various types of mathematical challenges.
Common Techniques and Strategies
To fully grasp mathematical ideas, it’s important to familiarize yourself with the most effective techniques. This includes methods for simplifying expressions, solving equations, and recognizing patterns that can lead to quicker solutions. By practicing these techniques regularly, you will increase both your speed and accuracy in solving problems.
Key strategies:
- Breaking down complex problems into smaller steps
- Applying formulas consistently
- Recognizing patterns and relationships between numbers
As you progress through exercises, remember that the more you practice these core concepts, the stronger your understanding will become. With time, these skills will become second nature, allowing you to approach problems with greater confidence and efficiency.
Solving Equations in Section 2
This section focuses on developing your skills in solving mathematical equations, a crucial aspect of mastering problem-solving. Understanding how to manipulate variables and balance equations is essential for progressing in more advanced topics. By practicing different types of equations, you will improve your ability to find solutions systematically and with greater ease.
Approach to Solving Linear Equations
Linear equations are one of the most fundamental types, and solving them requires a clear understanding of how to isolate variables. The process typically involves simplifying both sides of the equation and applying inverse operations to achieve the solution. As you practice, it’s important to check your work at each step to ensure accuracy.
Techniques for Solving Complex Equations
More complex equations may involve multiple variables or terms that need to be combined. In these cases, you may need to apply a series of steps such as factoring, using the distributive property, or simplifying expressions before solving. These techniques require practice but can significantly improve your efficiency in finding solutions.
Steps to solve equations:
- Simplify both sides of the equation
- Apply inverse operations to isolate variables
- Check your solution by substituting back into the equation
By consistently applying these techniques, you will gain a deeper understanding of how to approach various types of equations and become more confident in your problem-solving abilities.
Step-by-Step Solutions for Practice Problems
Breaking down practice problems into manageable steps is an essential strategy for understanding complex topics. By following a clear, systematic approach, you can gradually build confidence in solving different types of mathematical challenges. This method helps you see the logic behind each step and ensures that you reach accurate solutions without missing crucial details.
Understanding Each Step in the Process
Each problem in this section is designed to be solved by applying a series of well-defined steps. From simplifying expressions to isolating variables, breaking down the problem ensures that you don’t overlook important details. By following the step-by-step solutions, you will not only arrive at the correct answer but also understand the reasoning behind each action.
Example of Solving a Practice Problem
Here is an example of how to approach a typical problem using a step-by-step solution:
| Step | Action | Explanation |
|---|---|---|
| 1 | Simplify both sides of the equation | Combine like terms to reduce complexity |
| 2 | Apply inverse operations | Isolate the variable on one side |
| 3 | Check the solution | Substitute the value back into the original equation |
By repeating this process with various problems, you will improve your ability to solve them efficiently. Practicing these steps regularly helps reinforce your understanding and makes problem-solving feel more intuitive.
How to Approach Mathematical Word Problems
Word problems can often seem overwhelming at first glance, but breaking them down into manageable steps makes them easier to solve. The key is to translate the written information into mathematical expressions and equations. By identifying the relevant details and focusing on what is being asked, you can transform complex situations into solvable tasks.
The first step in solving word problems is to carefully read the entire problem to understand the context and identify the known and unknown quantities. Next, you should assign variables to represent these unknowns. Once the problem is set up mathematically, you can apply the appropriate methods to solve it.
After solving, always review your answer to ensure it makes sense within the context of the problem. This final check can prevent errors and reinforce your understanding of the material.
Common Mistakes in Mathematical Problem Solving
When solving mathematical problems, it’s easy to make errors that can lead to incorrect answers. These mistakes often stem from misunderstandings of key concepts, rushing through steps, or neglecting to double-check work. Recognizing these common pitfalls can help you avoid them and improve your overall problem-solving skills.
Some of the most frequent mistakes include incorrect operations, misunderstanding the problem’s requirements, and making calculation errors. Being aware of these issues and taking time to carefully review each step can prevent these mistakes and help you develop stronger mathematical abilities.
| Error | Cause | Solution |
|---|---|---|
| Incorrect application of operations | Forgetting to apply inverse operations or using the wrong operation | Double-check each step and ensure the correct operation is used |
| Skipping steps | Rushing through problems or skipping simplification | Take time to go through each step carefully and methodically |
| Misinterpreting word problems | Not understanding what the problem is asking for | Read the problem thoroughly, and write down what is known and unknown |
| Calculation errors | Simple arithmetic mistakes | Always verify calculations, especially in multi-step problems |
By being mindful of these common mistakes and practicing problem-solving with attention to detail, you can significantly reduce errors and improve your understanding of mathematical concepts.
Tips for Mastering Section 2 Topics
To master the concepts and skills in this part of the material, it’s important to approach each topic with focus and consistent practice. With the right strategies, you can deepen your understanding, reinforce your learning, and enhance your ability to solve problems effectively. The following tips will help guide you through the most important aspects of the material.
Effective Study Strategies
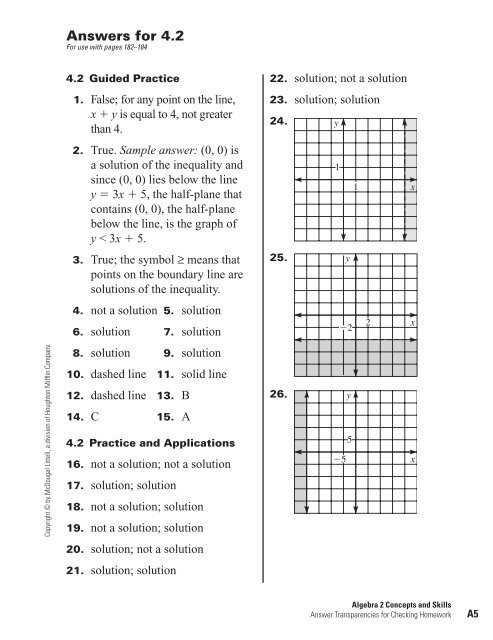
Adopting the right study techniques will make a significant difference in how quickly and effectively you master new topics. The key is to break down complex concepts into smaller, more manageable parts and focus on mastering each one before moving on to the next.
- Review key concepts regularly to reinforce your understanding.
- Work on practice problems to apply what you’ve learned and identify areas of improvement.
- Seek out additional resources, such as videos or online tutorials, to supplement your learning.
Mastering Problem-Solving Techniques
Building strong problem-solving skills is critical to succeeding in this part of the material. Learning how to break down each problem into logical steps will help you approach even the most challenging exercises with confidence.
- Read each problem carefully and identify the key information.
- Set up the problem step-by-step, making sure each step follows logically from the previous one.
- After solving, double-check your work to ensure the solution makes sense.
By implementing these strategies and focusing on consistent practice, you’ll be well on your way to mastering the topics in this section and achieving greater success in your mathematical learning journey.
Key Formulas and Rules to Remember
To succeed in solving problems, it’s essential to have a strong grasp of the key formulas and principles that form the foundation of problem-solving strategies. These formulas and rules provide the framework for tackling various mathematical challenges and help ensure that you approach problems with accuracy and confidence.
By memorizing and understanding these core concepts, you’ll be able to quickly recognize patterns and apply the right tools when faced with different types of problems. Below are some key formulas and rules to keep in mind.
Essential Formulas
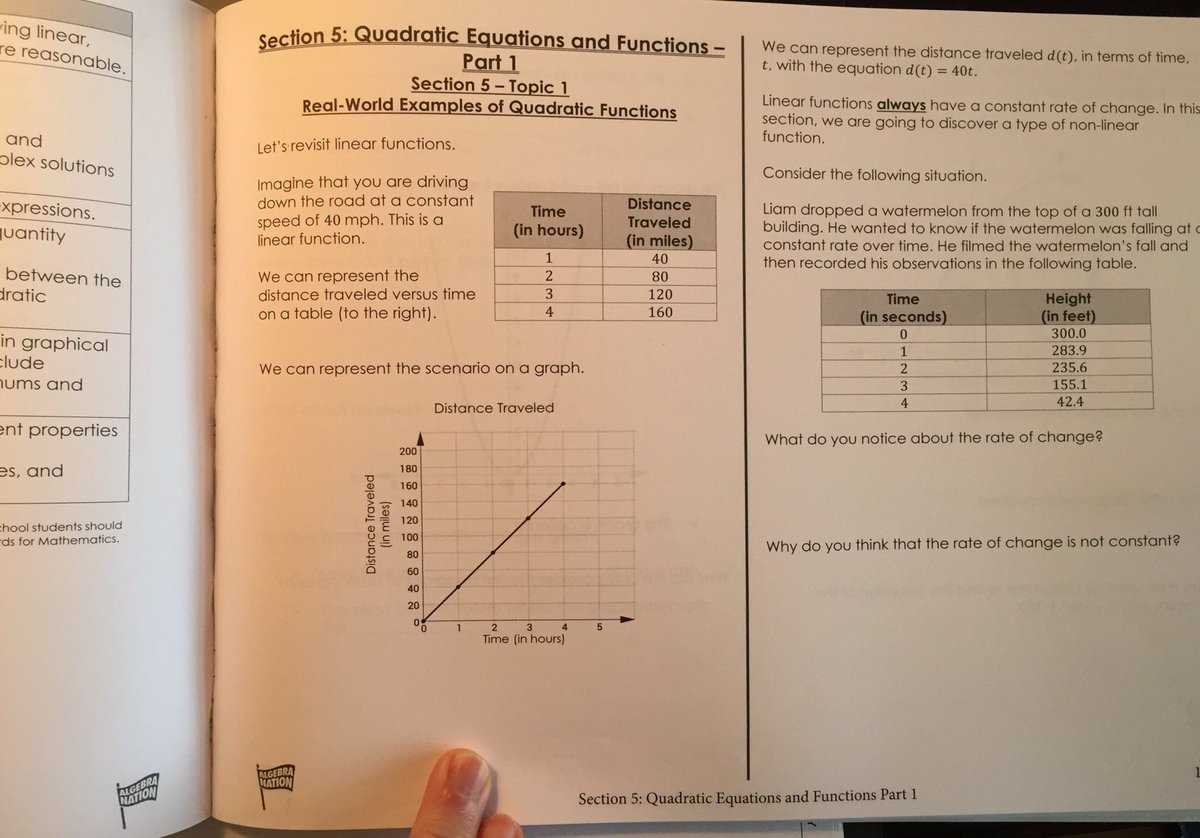
- Distributive Property: a(b + c) = ab + ac
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a
- Slope of a Line: m = (y₂ – y₁) / (x₂ – x₁)
- Area of a Rectangle: A = length × width
Important Rules to Follow
- Always simplify expressions before attempting to solve equations.
- When solving for variables, perform the same operation on both sides of the equation.
- Be aware of the order of operations: Parentheses, Exponents, Multiplication and Division, Addition and Subtraction (PEMDAS).
- For word problems, clearly define all variables and translate the problem into an equation before solving.
By committing these formulas and rules to memory and practicing their application, you will develop the skills needed to approach a wide range of problems with ease.
How to Check Your Answers Correctly
Once you’ve solved a problem, it’s crucial to verify that your solution is correct. This process helps you catch any mistakes made during calculations or in the interpretation of the problem. The goal is to ensure that the result makes sense and fits the conditions of the question.
Checking your work involves revisiting the steps you took to reach the solution, using alternate methods when possible, and comparing your results with the expected outcomes. This approach will help you identify any errors and reinforce your understanding of the process.
Steps for Correctly Verifying Your Solution
Here are a few effective techniques to check your solutions:
- Retrace each step in your solution to confirm every operation was performed correctly.
- If applicable, substitute your result back into the original equation to see if it satisfies the conditions.
- Cross-check with an alternative method or formula to see if the result matches.
- Look for logical consistency in your answer; does it fit within the context of the problem?
Common Methods of Verification
There are several common ways to verify solutions, depending on the type of problem you are solving:
| Problem Type | Verification Method |
|---|---|
| Linear Equations | Substitute the solution back into the original equation. |
| Quadratic Equations | Use the quadratic formula to check the results against each other. |
| Word Problems | Re-read the problem to ensure your solution matches the given conditions. |
By following these steps and methods, you can confidently verify your solutions and minimize the risk of making mistakes. This habit will improve both the accuracy of your work and your overall problem-solving skills.
Exploring Graphs and Functions in Section 2
Understanding the relationship between variables is crucial for solving problems efficiently. In this section, we delve into how different mathematical functions are represented visually and how to interpret these graphical representations. By studying graphs, you can gain a deeper understanding of the underlying mathematical concepts and their real-world applications.
Graphs allow us to see how one quantity changes in relation to another, offering valuable insights that can be difficult to grasp through equations alone. Through this exploration, you’ll learn how to construct and analyze various types of graphs, and use them to solve problems more effectively.
Key Graphing Techniques
To successfully interpret and create graphs, it’s important to master a few key techniques:
- Plotting Points: Start by marking key coordinates on the grid. Each point represents a specific relationship between two variables.
- Identifying Slope and Intercept: The slope shows how much one variable changes as the other changes, while the intercept represents where the graph crosses the axis.
- Understanding Function Behavior: Analyze the shape of the graph to understand the function’s behavior–whether it’s increasing, decreasing, or remaining constant.
Practical Applications of Graphs
Graphs are not just abstract concepts–they have many practical uses, especially when analyzing real-world data. For example, graphs can be used to represent trends in business, economics, and even daily life situations. They help visualize complex data and make it easier to draw conclusions and make informed decisions.
By mastering these techniques and understanding the significance of graphs and functions, you will be better equipped to solve a variety of problems and apply your knowledge in meaningful ways.
Strategies for Solving Complex Problems
When faced with challenging problems, having a clear strategy can make a significant difference in your approach and outcome. These problems often involve multiple steps or concepts, requiring careful thought and organization. By breaking down complex problems into manageable parts and applying systematic methods, you can tackle them more effectively.
The key to solving intricate problems is recognizing patterns, making logical connections, and approaching the problem from different angles. With the right strategies, you can simplify the process and avoid feeling overwhelmed by the complexity of the task.
Here are some strategies to help you solve complex problems with greater efficiency:
- Understand the Problem: Before attempting a solution, carefully read and analyze the problem. Identify all the given information and what needs to be solved.
- Break it Down: Divide the problem into smaller, simpler steps. This will make the task less daunting and help you focus on one piece at a time.
- Look for Patterns: Patterns often reveal themselves during the problem-solving process. Identifying these patterns can help you predict the next steps and lead to faster solutions.
- Check for Simpler Solutions: Before delving into complicated methods, see if there is a more straightforward way to solve the problem. Sometimes, simplifying the problem is all it takes.
- Work Backwards: In some cases, working backwards from the desired result can help you identify the correct steps or methods to reach the solution.
By practicing these strategies and developing a problem-solving mindset, you’ll become more adept at tackling complex challenges and building a deeper understanding of the concepts at hand.
What to Do if You’re Stuck
It’s natural to encounter obstacles when solving challenging problems, and sometimes it may feel like you’re completely stuck. However, the key to overcoming these moments is persistence and knowing the right steps to take. The first thing to remember is that getting stuck is a part of the learning process and can lead to greater understanding once you work through it.
There are several strategies you can use to get back on track and continue making progress. The important thing is to remain calm and approach the situation systematically, rather than feeling overwhelmed.
Step Back and Review the Problem
When you’re struggling, it’s often helpful to take a step back and carefully review the problem again. Sometimes, it’s easy to overlook key details or misinterpret the question. Rereading the problem slowly can help you gain new insights and identify any information you’ve missed.
Try Different Approaches
If the method you’re using isn’t working, consider trying a different approach. There are often multiple ways to solve a problem, and exploring new methods may help you find a path forward. For instance, if you’re stuck on an equation, try breaking it down into simpler steps or use a visual method like graphing to gain a different perspective.
Remember, it’s okay to ask for help when you’re stuck. Whether it’s a tutor, classmate, or online resource, seeking assistance can provide you with a new approach and help you understand the material better. The important thing is to keep moving forward, even if it means taking a brief pause to reset your thinking.
Real-Life Applications of Algebra Concepts
The concepts and techniques learned in mathematical studies are not only useful in classrooms but also have a wide range of practical applications in everyday life. These principles help solve problems, make decisions, and understand the world around us. Whether in business, technology, or personal finance, the ability to apply mathematical reasoning is invaluable.
Understanding how these mathematical methods are used in real-life scenarios can make the subject more relevant and engaging. By connecting theory with practice, you gain a deeper appreciation for how numbers and formulas are used to solve actual problems.
Applications in Business and Finance
One of the most common real-world applications of mathematical reasoning is in the world of business and finance. From budgeting to investment strategies, understanding relationships between variables helps make informed decisions.
- Budgeting: Knowing how to calculate expenses, profits, and savings can help individuals and businesses manage their finances effectively.
- Loans and Mortgages: Calculating interest rates, monthly payments, and loan amortization schedules involves using equations to determine the cost of borrowing money.
- Investment Analysis: Understanding how variables like interest rates, time, and capital influence investment returns is a crucial part of financial planning.
Applications in Technology and Engineering
Mathematical principles are foundational in fields like engineering and technology. Whether designing structures, developing software, or analyzing data, mathematical formulas are used to model, predict, and solve complex problems.
- Engineering Design: Engineers use equations to calculate forces, stresses, and material properties to design safe and efficient structures.
- Technology Development: Software developers rely on algorithms, which are based on mathematical concepts, to create applications and systems that function smoothly.
- Data Analysis: Understanding statistical relationships and trends is essential for interpreting data and making decisions in fields such as healthcare and marketing.
As you can see, the principles learned in math courses are not just abstract concepts; they are tools used to solve practical problems and make informed decisions. The more you understand how these ideas are applied in real life, the more useful and relevant they become.
Using the Workbook for Efficient Study
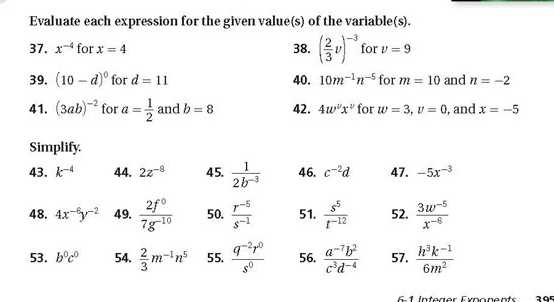
Using a structured study guide can significantly enhance your learning experience, helping you grasp essential concepts and solve problems more effectively. A good study guide provides exercises, examples, and step-by-step instructions that are designed to reinforce the material covered in your lessons. It serves as an excellent tool to test your understanding, identify areas for improvement, and solidify your knowledge.
By incorporating the guide into your study routine, you can maximize your productivity and ensure you’re well-prepared for exams or assignments. The key to effective study lies in consistent practice, understanding the underlying principles, and knowing how to approach different types of problems.
Organizing Your Study Sessions
To get the most out of your study sessions, it’s important to have a clear plan. A well-organized approach allows you to focus on specific areas, ensuring you’re building your skills progressively. Here are some tips for making the most of your study guide:
- Prioritize key concepts: Start with the foundational topics before moving on to more complex material. This ensures you have a solid understanding of the basics.
- Set specific goals: Break your study time into manageable chunks and set clear objectives for each session. This keeps you focused and prevents burnout.
- Review regularly: Revisit previous exercises and examples to reinforce your understanding and retain information more effectively.
Practicing with Examples
Working through examples is an essential part of mastering any subject. Practice problems help you apply what you’ve learned and build confidence in your problem-solving abilities. As you work through each problem, pay attention to the methods and strategies used to arrive at the solution. This will help you recognize patterns and improve your overall problem-solving skills.
- Analyze each step: Break down the steps involved in solving a problem to understand the logic behind each operation.
- Identify common mistakes: Take note of errors you make while solving problems, and focus on understanding why they occurred to avoid repeating them.
- Challenge yourself: As you become more comfortable, increase the difficulty of the problems to continue challenging yourself and expand your skill set.
By consistently using the study guide to reinforce and practice the material, you’ll be well on your way to mastering the content. The more effort you put into using the guide effectively, the more confident you’ll become in your ability to solve complex problems and apply what you’ve learned in real-life situations.
How Section 2 Prepares You for Advanced Topics
The second part of the learning material serves as a crucial foundation, ensuring that you have the necessary skills and knowledge to progress to more complex subjects. By focusing on core principles and essential techniques, this section helps to solidify your understanding of fundamental concepts, making it easier to grasp more advanced topics in the future.
Throughout this section, you are introduced to problem-solving methods, critical thinking skills, and various strategies that can be applied to more challenging material. Mastery of these skills will not only improve your ability to tackle more complex problems but also give you the confidence needed to approach higher-level topics with a deeper understanding.
Key areas that are covered in this section are often building blocks for advanced topics. For example, concepts involving equations, inequalities, and functions are frequently revisited in later studies, with greater depth and sophistication. Developing a strong grasp of these concepts now ensures that you will not only handle future material with ease but also make connections between topics that will enrich your learning experience.
In addition to providing a solid foundation in theory, this part of the course emphasizes practical application. By solving a range of problems and learning to interpret complex data, you will be better prepared for future subjects that require logical reasoning and problem-solving, such as calculus, data analysis, or even engineering principles.
By the time you complete this section, you will be equipped with the essential tools and confidence to move forward in your studies. The knowledge gained here serves as a springboard for future learning, allowing you to build on what you’ve mastered and tackle more advanced and specialized topics with success.
Reviewing and Reinforcing Key Algebra Skills
Mastering essential mathematical concepts requires continuous practice and review. Revisiting core techniques and skills ensures that foundational knowledge is solidified and applied correctly in future challenges. This section emphasizes the importance of revising key principles and reinforces your understanding by providing targeted exercises and examples that address common pitfalls.
One of the best ways to strengthen your understanding is through repetition and application. The more you practice core concepts, the more confident you will become in solving problems efficiently and accurately. By consistently reviewing topics, you are not only reinforcing your skills but also building a deeper understanding of how these principles interconnect and support more advanced concepts.
Effective Strategies for Reinforcement
- Practice Regularly: Consistent practice ensures that concepts remain fresh in your mind and helps to identify areas where improvement is needed.
- Work Through Examples: Take time to solve a variety of examples to reinforce understanding and adapt strategies to different problem types.
- Break Down Complex Problems: Start with simpler examples and gradually build up to more complex problems to develop a systematic approach to solving challenges.
- Identify Common Mistakes: Review common errors in problem-solving to understand where and why mistakes occur, helping to avoid them in the future.
- Test Your Understanding: Periodically test yourself on key concepts to ensure mastery and uncover any gaps in your knowledge.
Reinforcement Techniques
In addition to solving problems, using different approaches can help reinforce your skills more effectively. Visual aids like graphs and charts can provide a clearer understanding of abstract concepts, while explaining solutions to others helps deepen your own comprehension. Another useful strategy is to review previous exercises and try to solve them in different ways, which enhances flexibility in problem-solving.
By continuously reviewing and applying these key skills, you will build a strong foundation that makes more advanced mathematical topics easier to approach and understand.