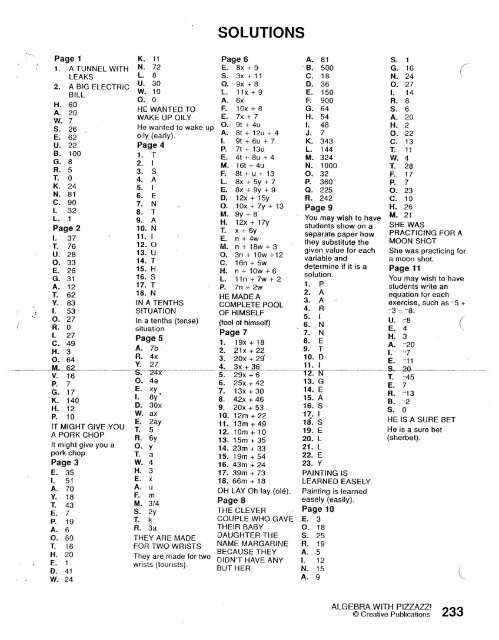
Algebra with Pizzazz Worksheet Answers Page 161

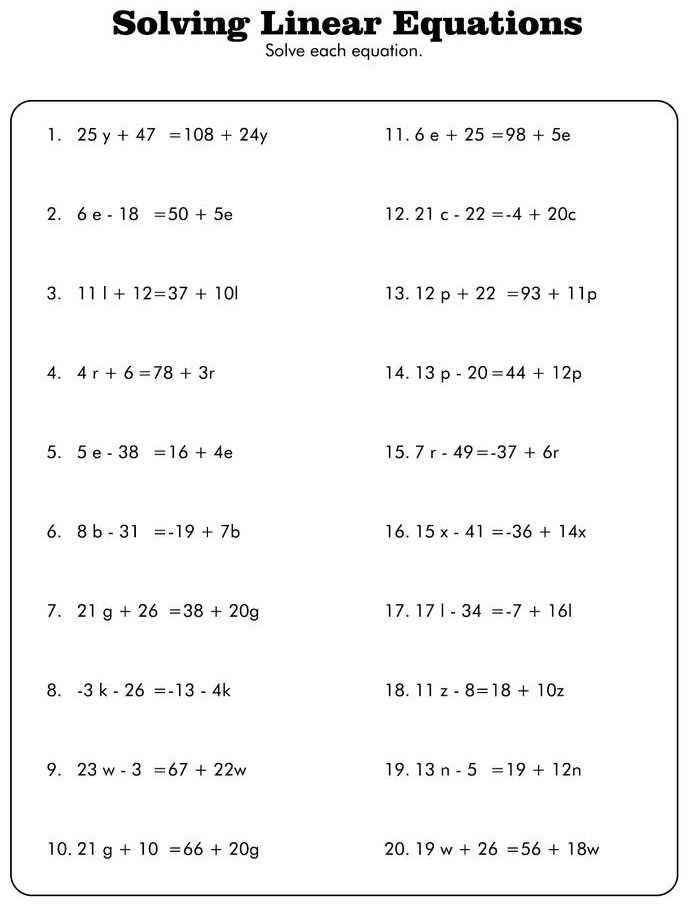
Mathematical exercises that involve variable manipulation and equation solving are key to developing critical thinking and problem-solving skills. These activities often require a structured approach, as they can range from simple to complex. Understanding the underlying principles is essential for effective learning and application. The ability to break down problems into manageable parts is a valuable skill that students can carry into other fields of study and everyday life.

By practicing regularly, learners can improve both their speed and accuracy when working through problems. This section provides valuable insights into the steps required to tackle a variety of challenges. Whether you’re reviewing solutions or exploring new concepts, these techniques help sharpen your approach. Mastering these methods makes it easier to navigate through complex scenarios.

Mathematical Exercises and Solution Techniques

When solving mathematical challenges that involve manipulating numbers and variables, it is important to follow a clear and logical method. Each problem presents unique elements that require different approaches. By practicing these exercises, learners can enhance their understanding of mathematical operations and improve their problem-solving abilities. These skills are crucial for tackling both academic and real-world problems effectively.

Breaking Down Problems for Better Clarity

One effective way to approach complex tasks is to break them down into smaller, more manageable steps. This method allows for a clearer understanding of the relationship between different elements within the problem. By addressing each part individually, it becomes easier to find a solution. Below is a table showcasing some common techniques for simplifying various mathematical problems:

Technique	Description
Identifying Key Variables	Focus on the most important elements that affect the solution, such as unknowns and constants.
Isolating Terms	Group similar terms together to simplify the process of solving equations.
Checking Work	Verify each step as you go to prevent errors and ensure accuracy.
Applying Real-World Examples	Use practical situations to better understand abstract concepts.

Improving Speed and Accuracy

As proficiency increases, so does speed. By practicing these techniques consistently, students can solve problems more quickly and accurately. This is especially important when dealing with timed tests or assignments. Regular practice and reviewing solutions can also help reinforce the concepts learned, leading to greater confidence in solving similar problems in the future.

Understanding the Worksheet’s Key Concepts

To successfully solve mathematical exercises, it’s essential to first grasp the core principles that govern the problems. These concepts provide a foundation for approaching challenges effectively. Whether the task involves equations, variables, or operations, understanding the underlying rules is crucial for finding the correct solutions. Breaking these concepts into smaller, understandable parts ensures that each step of the process is manageable and logical.

Identifying Fundamental Operations

One of the first steps in approaching any mathematical problem is to identify the operations involved. These could range from basic arithmetic functions to more advanced operations like factoring or simplifying expressions. Understanding how to perform each operation correctly is vital for progressing toward the final solution. It’s important to recognize the relationship between different operations and how they interact within a given problem.

Recognizing Patterns and Relationships

In many mathematical exercises, recognizing patterns and relationships between terms can provide shortcuts to finding solutions. Whether it’s recognizing a common factor, a distributive property, or a symmetrical structure, these patterns can simplify complex tasks. Identifying these patterns early on allows students to apply efficient strategies and reduce the chances of making errors.

Step-by-Step Problem Solving Process

Approaching mathematical problems in a structured way can make even the most complex tasks seem manageable. By following a clear, step-by-step method, you can break down each challenge into smaller parts, making it easier to identify the correct approach and solution. The process involves analyzing the problem, applying the appropriate strategies, and verifying the result. This method not only ensures accuracy but also helps build confidence in solving similar tasks in the future.

Breaking Down the Problem

The first step in solving any problem is to carefully read and understand what is being asked. This involves identifying key elements such as the variables, constants, and operations required. Once these components are identified, it becomes easier to figure out the path to the solution.

• Read the problem carefully and highlight important information.
• Identify known and unknown variables.
• Determine what operations or rules are necessary to solve the problem.

Executing the Solution

Once you have a clear understanding of the problem, the next step is to apply the necessary operations. This could involve simplifying terms, combining like terms, or using specific formulas. Throughout this stage, it’s important to work methodically and check each step as you progress to avoid errors.

1. Perform operations in a logical order (e.g., PEMDAS or other relevant rules).
2. Simplify expressions and isolate unknowns as needed.
3. Check each calculation to ensure accuracy.

By following these steps, you can confidently work through a variety of mathematical challenges, ensuring both precision and efficiency in the process.

Solving Algebraic Expressions with Ease

Solving mathematical expressions that involve variables and constants can seem intimidating at first, but with the right approach, it becomes a manageable task. The key is to understand the relationships between terms and know which operations to apply at each step. Once the basic rules and strategies are mastered, solving these problems becomes a more intuitive process. With practice, you can approach each expression confidently and efficiently.

One of the most important strategies is simplifying the expression step by step. Begin by combining like terms and applying the appropriate operations. Always work methodically, ensuring that each step follows logically from the previous one. This systematic approach reduces the chance of making errors and makes complex problems more approachable.

Another crucial tip is to use factoring techniques or distributive properties when necessary. These methods help simplify expressions more quickly, leading to faster and more accurate solutions. By familiarizing yourself with these techniques, you’ll find it easier to solve even more challenging tasks with greater ease.

Common Mistakes and How to Avoid Them

When working through mathematical exercises, it’s easy to make mistakes, especially when dealing with complex operations or multiple steps. These errors can often lead to incorrect results, but they are usually avoidable with careful attention and a systematic approach. By being aware of the most common pitfalls, you can take proactive steps to minimize mistakes and improve your problem-solving skills.

One common mistake is misinterpreting the problem’s requirements. This can happen when important details are overlooked or when the wrong operation is applied. Always read the problem carefully and make sure you understand what is being asked before starting the solution.

Another frequent error occurs during the simplification process, where terms are combined incorrectly or steps are skipped. This can lead to unnecessary complexity or incorrect final answers. To avoid this, double-check each step, especially when simplifying expressions or combining like terms.

Additionally, not following the correct order of operations can lead to errors. Whether it’s multiplying before adding or missing parentheses, the sequence in which operations are performed is crucial. Make sure to apply the order of operations correctly, such as PEMDAS, to maintain accuracy throughout the process.

Helpful Tips for Improving Speed and Accuracy

Improving both speed and accuracy when solving mathematical problems requires a combination of practice, strategy, and focus. By adopting effective techniques, you can become more efficient at solving problems while minimizing mistakes. These tips are designed to help you work through exercises more quickly and with greater precision, ensuring that you consistently reach the correct solutions.

One essential tip is to familiarize yourself with common patterns and formulas. The more you practice, the quicker you’ll recognize similar structures in problems. This recognition allows you to apply the correct approach more swiftly, reducing the time spent figuring out each step.

Another helpful strategy is to break down each problem into smaller parts. By isolating different elements of the task, you can tackle each one separately, making the overall process faster and less overwhelming. This method also helps reduce the risk of skipping crucial steps or making errors in complex calculations.

Finally, make it a habit to double-check your work as you go. While it may seem time-consuming, reviewing each step ensures that you’re on the right track and allows you to catch mistakes before they become bigger issues. Over time, this will help improve both your speed and accuracy in solving problems.

Mastering Techniques for Simplifying Equations

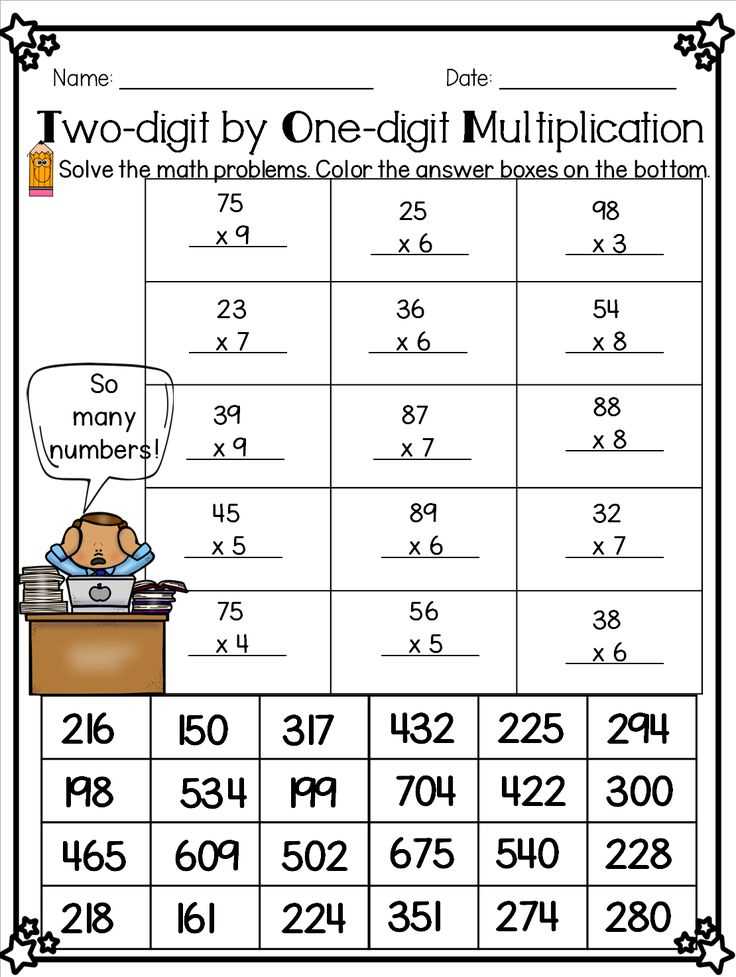
Simplifying equations is a fundamental skill that can greatly enhance your ability to solve mathematical problems efficiently. Whether you’re working with basic operations or more complex expressions, knowing the right techniques can make the process much smoother. By breaking down each equation step by step, you can eliminate unnecessary terms, group similar elements, and ultimately arrive at a simplified solution more quickly.

Key Methods for Streamlining Expressions

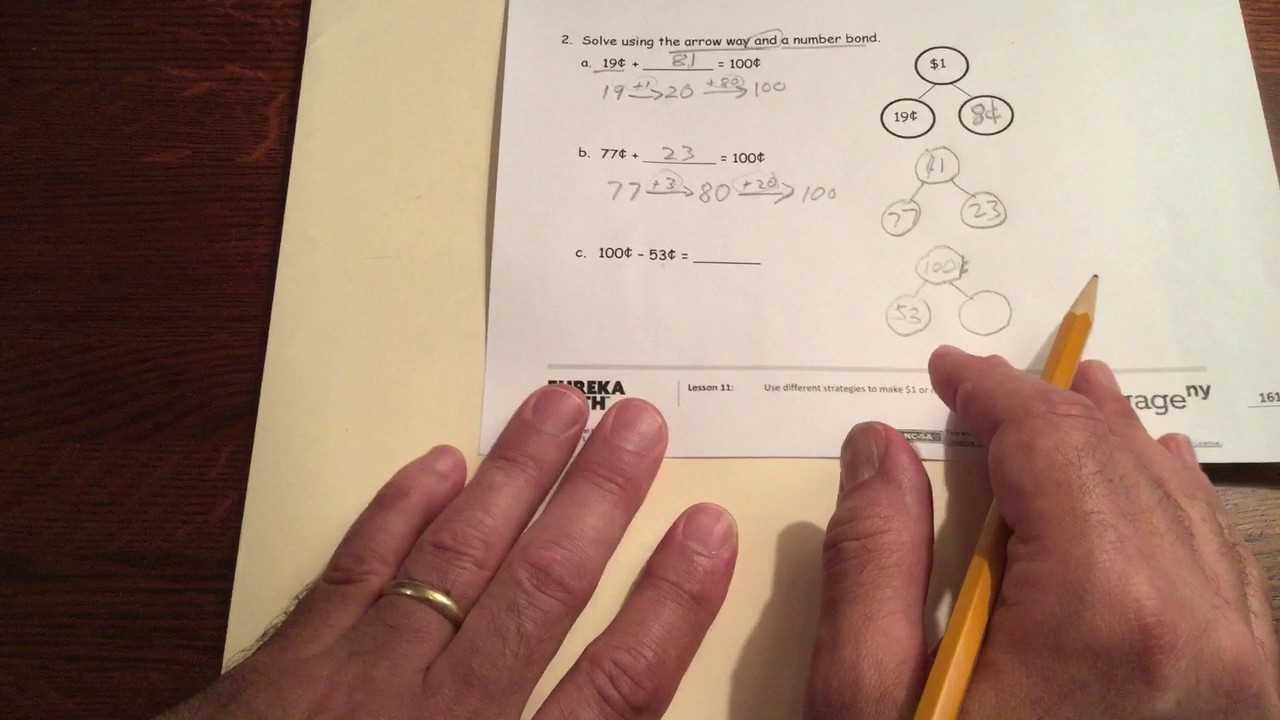
There are several approaches to simplifying equations, each suited to different types of problems. Common methods include combining like terms, factoring, and using the distributive property. Mastering these techniques allows you to solve problems faster and with greater accuracy. The table below highlights these methods and when to apply them:

Method	When to Use
Combining Like Terms	When you have terms that share the same variable or constant.
Factoring	When you can express an equation as a product of binomials or other expressions.
Distributive Property	When an expression involves parentheses that need to be expanded.

Practical Tips for Success

To become proficient at simplifying equations, practice is key. Start by applying these methods to simple equations and gradually work your way up to more complex problems. The more you work with these techniques, the more intuitive they will become, and your ability to simplify equations quickly will improve significantly.

Breaking Down Complex Algebraic Problems

When faced with a complex mathematical challenge, the key to solving it efficiently is to break it down into smaller, more manageable parts. Tackling each component individually allows you to focus on one element at a time, ensuring you don’t become overwhelmed. By applying logical steps and recognizing patterns, even the most complicated problems can be simplified and solved systematically.

Steps to Simplify Complicated Problems

To effectively manage difficult problems, it’s important to have a clear strategy in place. Start by identifying the main operations and elements of the equation. Then, apply appropriate techniques such as grouping like terms, using distributive properties, and eliminating unnecessary components. This step-by-step breakdown makes it easier to work through the problem and avoid errors along the way.

Step	Action
Step 1	Identify the core operations (addition, multiplication, etc.).
Step 2	Group like terms and simplify any constants.
Step 3	Apply necessary properties (distributive, associative, etc.).
Step 4	Check for errors and simplify further if needed.

Recognizing Patterns in Complex Problems

As you gain experience, you’ll start recognizing common patterns in more intricate problems. These patterns can guide you in applying the right methods more quickly. For example, identifying symmetry or repeated terms can help you streamline the solution process. The more you practice breaking down complex problems, the more intuitive these patterns will become, ultimately improving your problem-solving skills.

Strategies for Memorizing Algebra Formulas

Memorizing mathematical formulas can seem like a daunting task, but with the right strategies, it becomes much easier. A solid understanding of the formulas you encounter will not only speed up problem-solving but also increase your confidence when tackling different types of exercises. The key is repetition, association, and finding ways to relate the formulas to real-life examples or patterns you already know.

Here are several strategies that can help you effectively memorize essential formulas:

• Repetition and Practice: Write down each formula multiple times to reinforce your memory. Repeated exposure to the formula in different contexts helps solidify it in your mind.
• Use Mnemonics: Create simple memory aids or phrases that help you recall the structure of a formula. For example, a catchy phrase can make remembering a complex equation easier.
• Visual Aids: Draw diagrams or charts that represent the formulas. Associating a visual image with each formula can help make it more memorable.
• Chunking: Break down complex formulas into smaller, more manageable parts. Memorize these parts individually and then combine them to form the full formula.
• Real-Life Applications: Relate the formulas to practical examples you encounter in everyday life, such as calculating areas or distances. This helps you understand the formula’s purpose and reinforces your memory.

By applying these strategies consistently, you’ll find that remembering important formulas becomes less challenging, and you’ll be able to recall them quickly when solving problems.

Exploring Additional Practice Questions

Engaging with a variety of practice questions is one of the most effective ways to enhance your problem-solving abilities. By working through a diverse set of problems, you not only reinforce what you’ve already learned, but you also expose yourself to new challenges that help solidify your understanding of key concepts. The more questions you attempt, the more confident and skilled you will become in handling similar problems in the future.

Below are some strategies for making the most of additional practice questions:

• Start Simple: Begin with easier problems to build your confidence and ensure that you have a strong grasp of the basics before tackling more challenging ones.
• Work on Different Topics: Practice a range of problems across different concepts to get a comprehensive understanding of the subject. This will help you develop flexibility in your approach.
• Time Yourself: Set a time limit for each question to simulate real test conditions. This will help improve your speed and decision-making abilities during timed assessments.
• Review Mistakes: After completing a set of problems, go back and carefully review any mistakes you made. Understanding why you got a question wrong is crucial for avoiding similar errors in the future.
• Challenge Yourself: Once you’re comfortable with basic questions, challenge yourself with more difficult problems to expand your skills and push your limits.

Incorporating these strategies into your practice routine will help you tackle a wide range of problems with confidence and precision. Consistent practice is key to mastery.

How to Check Your Answers Effectively

Verifying your work is a crucial step in ensuring that your solutions are correct and that you haven’t made any mistakes. Effective checking not only helps catch errors but also reinforces your understanding of the steps involved in solving the problem. By following a systematic approach, you can be confident in the accuracy of your results and improve your problem-solving process over time.

Here are some strategies for checking your solutions effectively:

• Revisit the Problem: Before you begin checking, read the original question again. Make sure you understand what is being asked and confirm that you’ve addressed all parts of the problem.
• Reverse Your Operations: If possible, work backwards from your solution. This can help you identify whether your final answer is logically consistent with the initial conditions.
• Check for Common Mistakes: Review common errors such as miscalculations, sign mistakes, or incorrectly applied formulas. These are often simple to overlook during the initial solving process.
• Use Different Methods: If the problem allows, solve it using a different method or approach. If both methods lead to the same result, it’s a strong indication that your answer is correct.
• Double-Check Units and Signs: If your solution involves units of measurement or signed numbers, ensure that they are consistent and correctly applied throughout your work.

By incorporating these checking techniques into your routine, you’ll be able to identify errors early and improve your overall accuracy. Taking the time to double-check your work is an essential habit that will strengthen your mathematical skills.

How Algebra Builds Problem-Solving Skills

Engaging with mathematical concepts develops critical thinking and strengthens your ability to solve complex problems. By learning how to analyze patterns, make logical connections, and apply different strategies, you can approach any challenge with a methodical mindset. This process not only enhances your mathematical proficiency but also transfers to various real-world situations, improving overall problem-solving abilities.

Developing Logical Thinking

When tackling problems, the need to break down each step logically is essential. Mathematical exercises require you to identify relationships, simplify expressions, and draw conclusions based on established rules. This constant practice hones your ability to think critically and approach problems step by step, a skill that is invaluable in everyday life and professional environments.

Building Persistence and Patience

Complex problems often require perseverance. As you encounter challenging scenarios, learning to persist through trial and error helps cultivate patience. The process of refining your approach until you reach a solution teaches resilience and the value of learning from mistakes, both key components of effective problem-solving.

Through consistent practice, you develop the confidence and skills to tackle a wide range of problems, from academic challenges to practical, real-world situations. Strengthening these problem-solving skills benefits you far beyond the classroom.

Benefits of Using Algebra Worksheets Regularly

Practicing mathematical problems on a consistent basis offers numerous advantages for anyone looking to improve their problem-solving abilities. By dedicating time to solving exercises regularly, you not only reinforce previously learned concepts but also build new skills that will enhance your overall proficiency. Consistent practice helps sharpen both speed and accuracy, ensuring that you can solve problems more efficiently over time.

Improving Mathematical Skills

Regularly engaging with problems helps solidify key mathematical principles and strategies. It provides an opportunity to refine your skills, allowing you to tackle increasingly complex tasks with greater ease. As you continue practicing, the repetitive nature of solving problems strengthens your ability to recognize patterns, apply formulas, and follow logical steps effectively.

Boosting Confidence and Retention

When you regularly practice solving mathematical tasks, you gain confidence in your ability to approach any challenge. This consistency helps to cement the knowledge in your memory, ensuring that concepts and techniques remain fresh and accessible when needed. The more you practice, the more automatic your thinking becomes, leading to quicker and more reliable solutions.

Benefit	Impact
Improved Accuracy	By solving problems frequently, errors decrease, and precision improves.
Faster Problem Solving	Practice enables quicker identification of patterns and solutions.
Increased Understanding	Frequent engagement reinforces concepts and boosts comprehension.

By incorporating consistent practice into your routine, you can expect not only to improve your skills but also to gain a deeper understanding of the material. Over time, this approach leads to greater success and a more confident approach to solving complex problems.

Why Consistent Practice Leads to Mastery

Mastery in any field, particularly when it comes to problem-solving, requires consistent and focused practice. Repetition and regular engagement with challenging tasks help strengthen the brain’s ability to apply learned techniques. Over time, this continuous effort transforms knowledge from theoretical understanding to automatic skill, making even complex problems easier to tackle.

Building a Strong Foundation

Consistency is key to reinforcing the fundamentals. Each time you practice, you revisit essential concepts and improve your understanding of how they work together. This builds a strong foundation that allows for easier mastery of more advanced topics. The more you practice, the more natural the steps become, allowing you to handle complex problems effortlessly.

Enhancing Problem-Solving Speed

As you regularly solve problems, your ability to recognize patterns and solutions improves. What may have once been a slow and deliberate process becomes quicker and more intuitive. This increase in speed comes from familiarizing yourself with various problem types and learning the most efficient methods to solve them. Eventually, you will find yourself solving tasks faster and more accurately with each round of practice.

• Repetition reinforces knowledge, making it more accessible in future tasks.
• Regular practice helps identify weaknesses, allowing for targeted improvement.
• Building consistency strengthens both accuracy and speed over time.

Mastery isn’t achieved overnight. It requires dedication and the willingness to challenge yourself regularly. By committing to consistent practice, you create the foundation for success and continuous improvement, leading to an expert-level understanding of the material.

How to Apply Mathematical Concepts to Real-Life Situations

Mathematical concepts are not just abstract ideas; they have practical applications in everyday life. By understanding how to translate real-world problems into mathematical equations, you can simplify complex situations and make informed decisions. The key lies in recognizing patterns and relationships that allow you to model real scenarios with numbers and symbols.

Budgeting and Personal Finance

One of the most common applications of math is in managing personal finances. From calculating monthly expenses to planning savings goals, mathematical models help ensure you stay on track financially. For example, if you want to save a certain amount over a specific period, you can use simple equations to figure out how much to set aside each month. This is a straightforward application of solving for an unknown in an equation.

Cooking and Recipe Adjustments

In the kitchen, math plays a crucial role when adjusting recipes or managing portion sizes. If you need to alter the ingredients based on the number of servings, proportional reasoning helps make the necessary calculations. For instance, if a recipe calls for 2 cups of flour to serve 4 people and you need to adjust it for 6 people, basic ratios help you determine the right amounts of each ingredient.

• Managing budgets: Use equations to track income and expenses.
• Cooking: Apply ratios to adjust ingredient quantities for different serving sizes.
• Travel planning: Calculate distances, fuel needs, and time based on speed and routes.

By recognizing the mathematical patterns in everyday scenarios, you can apply these principles to make better choices, save time, and reduce errors. Whether you’re balancing a budget or cooking a meal, these concepts provide a powerful tool for solving practical problems.

Guidelines for Teaching Mathematical Concepts

Effective instruction in mathematical principles requires clear strategies that engage students and facilitate understanding. The goal is to build a strong foundation of concepts that students can apply to solve problems. By breaking down complex topics into manageable parts and using practical examples, educators can help learners grasp the core ideas and develop problem-solving skills.

1. Start with Concrete Examples

Begin by introducing students to basic, relatable examples. This helps bridge the gap between abstract concepts and real-world applications. For instance, use scenarios like calculating total costs or measuring quantities, where math is visibly involved. This approach makes the subject matter feel more relevant and less intimidating.

2. Break Down Complex Problems

Complex problems can overwhelm students if introduced too early or without sufficient background knowledge. Break down each step of the problem-solving process and explain the reasoning behind each step. Encouraging students to ask questions along the way ensures they understand each phase before moving on to the next.

3. Use Visual Aids and Tools

Visual aids such as graphs, charts, and diagrams can make abstract concepts easier to understand. Using tools like whiteboards or interactive software can allow students to see the problem and the solution as it develops. This helps to reinforce learning by engaging multiple senses and providing a clearer picture of the material.

4. Encourage Active Participation

Students learn best when they actively engage in the learning process. Allow them to participate in solving problems, either individually or in groups. Give them opportunities to present their solutions and reasoning to the class, fostering a sense of ownership over their learning.

• Use everyday examples to make concepts relatable.
• Provide step-by-step explanations to simplify difficult problems.
• Incorporate visual aids and interactive tools to enhance understanding.
• Promote student participation to increase engagement and retention.

By following these strategies, educators can create a positive learning environment that encourages mastery of mathematical concepts. With patience and practice, students will not only understand the material but also gain the confidence to apply it in various situations.