Core Connections Algebra Chapter 8 Answer Guide

In this section, we explore complex mathematical problems designed to enhance problem-solving skills and deepen understanding of fundamental concepts. The focus is on developing strategies for tackling intricate equations and applying mathematical principles effectively. Each problem presents a unique challenge, helping learners grasp essential skills needed for future coursework and real-world applications.

Mastering the process of solving problems involves breaking down each equation into manageable parts. By understanding the underlying rules and methodologies, students can approach each task with confidence. Practice with varied problem types allows for continuous improvement and mastery over time.

Through careful analysis and strategic thinking, learners can uncover patterns and connections that make solving problems more intuitive. The exercises in this section provide valuable insights into simplifying calculations and refining mathematical techniques, ensuring long-term success in mathematical studies.

Core Connections Algebra Chapter 8 Overview

This section focuses on key mathematical concepts designed to strengthen problem-solving abilities and enhance understanding of advanced topics. Through structured exercises, students will learn to identify patterns, work with complex equations, and apply critical thinking skills to various mathematical challenges. The goal is to provide a comprehensive understanding of these topics, allowing learners to approach future tasks with confidence and precision.

Throughout the exercises, students will encounter a variety of techniques for solving equations, managing variables, and simplifying expressions. The progression from basic to more advanced problems ensures that learners are gradually building their skills and solidifying their understanding of fundamental mathematical principles.

Topic	Description	Key Skills
Equations	Understanding the structure and solving different types of equations.	Simplification, substitution, solving for variables
Functions	Exploring relationships between variables and functions.	Graphing, interpreting function behaviors, transformations
Patterns	Recognizing patterns and using them to solve problems.	Pattern recognition, sequence solving, generalization
Problem-Solving Techniques	Applying strategies to efficiently tackle complex problems.	Critical thinking, strategy development, logical reasoning

By mastering these topics, students will not only improve their mathematical skills but also gain the confidence to tackle increasingly challenging exercises. With a focus on both theory and practice, this section provides a solid foundation for future academic and practical mathematical applications.

Understanding Key Algebraic Concepts

Grasping essential mathematical principles is crucial for solving complex problems and building a strong foundation in advanced topics. By focusing on core ideas, students develop the ability to approach various tasks with clarity and confidence. This section highlights fundamental concepts that are vital for mastering more intricate equations and understanding their applications.

The following key topics are central to building a strong mathematical framework:

• Equations and Expressions: Understanding the structure of mathematical statements and how to manipulate them.
• Variables: Learning how to represent unknowns and their relationships in mathematical formulas.
• Functions and Relationships: Recognizing how different quantities interact and using these connections to solve problems.
• Operations and Properties: Mastering the rules that govern addition, subtraction, multiplication, and division in equations.
• Patterns and Sequences: Identifying trends within numbers and variables that can simplify problem-solving.

These concepts serve as the building blocks for mastering more advanced material. A thorough understanding of each topic is necessary for moving on to more complicated subjects and for practical applications in the real world.

1. Start with Basic Operations: Master the basic mathematical operations before advancing to more challenging topics.
2. Apply Techniques to Problems: Use learned skills to solve real-world scenarios and mathematical puzzles.
3. Build on Each Concept: Continuously expand your understanding by linking new knowledge to what you’ve already mastered.

By developing proficiency in these areas, learners are better prepared to solve increasingly complex problems, ensuring a well-rounded understanding of mathematical reasoning and logic.

How to Approach Core Connections Problems

When faced with complex mathematical problems, it is essential to approach them with a systematic strategy. Breaking down the problem into smaller, manageable steps ensures that each part is understood and solved correctly. The key to success lies in being methodical, focused, and confident in applying learned techniques to each task.

Follow these steps to effectively tackle challenging mathematical exercises:

• Read the Problem Carefully: Ensure you understand the task before attempting to solve it. Look for important information such as variables, operations, and relationships.
• Identify the Objective: Know what the problem is asking. Are you solving for a specific variable? Are you simplifying an expression or finding a pattern?
• Break the Problem into Steps: Divide the problem into smaller parts. Solve each step methodically, ensuring you don’t miss any crucial details.
• Choose the Right Technique: Select an appropriate method based on the problem type. Whether it’s substitution, factoring, or graphing, use the technique that best fits the task at hand.
• Double-Check Your Work: After solving the problem, review each step to ensure there are no errors. Small mistakes can lead to incorrect results.

By applying these strategies, you can approach any mathematical problem with confidence and clarity. Practicing this method will improve your problem-solving skills and help you handle increasingly difficult challenges with ease.

1. Start with Simple Examples: Begin by solving easier problems to build a strong foundation before tackling more complex ones.
2. Use Visual Aids: Diagrams, graphs, and tables can help visualize relationships and simplify difficult concepts.
3. Stay Consistent: Consistent practice with various problems ensures that you become comfortable with different techniques and approaches.

With persistence and the right strategies, you’ll be well-equipped to solve even the most intricate problems effectively and efficiently.

Step-by-Step Solutions for Chapter 8

This section provides detailed, systematic solutions to the problems presented in the exercises. By breaking down each question into manageable steps, we aim to help you understand the logic behind each solution. These solutions offer a clear path to follow, ensuring that each concept is thoroughly explained and applied correctly.

Follow the steps outlined below to approach the problems effectively:

• Read and Analyze the Problem: Begin by carefully reviewing the given information. Identify the unknowns and the relationships between them.
• Formulate the Equation: Translate the problem into a mathematical expression. This could involve setting up an equation or a system of equations that represent the given situation.
• Apply Mathematical Techniques: Choose the appropriate methods such as substitution, elimination, or factoring to solve the equation.
• Simplify and Solve: Perform the necessary operations to simplify the equation and solve for the unknown variables.
• Verify the Solution: Once the solution is obtained, check it against the original problem to ensure it satisfies the conditions and is accurate.

By following this approach, each problem becomes easier to handle. Whether it’s a basic equation or a more complex challenge, this methodical process helps to clarify each step and avoid errors.

Consistent practice with this method will enhance your problem-solving skills and deepen your understanding of the concepts involved.

Common Mistakes in Algebra Chapter 8

When working through mathematical problems, it’s easy to make small errors that can lead to incorrect solutions. Understanding the most frequent mistakes can help you avoid them and strengthen your skills. By recognizing these common pitfalls, you can approach each problem more confidently and with greater accuracy.

Misinterpreting the Problem

One of the most common errors is misunderstanding the question or overlooking crucial details. Always ensure that you fully comprehend the given information before proceeding. Key details such as signs, variables, and conditions can significantly impact the solution.

Incorrect Simplification of Expressions

Another frequent mistake is simplifying expressions incorrectly. It’s easy to make errors when factoring, distributing, or combining like terms. Take the time to check each step and verify that each operation follows the correct order of operations.

By being mindful of these typical mistakes, you can reduce errors and improve your problem-solving process. Practicing these strategies will lead to more accurate results and a better grasp of the concepts involved.

Tips for Mastering Algebraic Techniques

To become proficient in solving mathematical problems, it’s essential to master the techniques used to manipulate equations, solve for variables, and simplify expressions. These skills form the foundation for handling more complex tasks and allow you to approach problems with greater efficiency and precision.

Developing Strong Problem-Solving Skills

Improving your ability to solve equations involves mastering specific techniques and applying them in various situations. Here are some tips to enhance your problem-solving approach:

• Practice Regularly: Consistent practice is key to improving. Solve a variety of problems to become familiar with different methods and techniques.
• Understand the Process: Don’t just memorize formulas; focus on understanding the reasoning behind each step. This will help you apply concepts to unfamiliar problems.
• Work on Mental Math: Developing your ability to perform calculations mentally will save time and improve accuracy during more complex problem-solving tasks.
• Use Visualization Tools: Diagrams and graphs can help you better understand the relationships between variables and simplify the solving process.

Breaking Down Complex Problems

When tackling difficult problems, breaking them into smaller, more manageable parts can make a big difference. Follow these steps to approach each problem effectively:

1. Identify Key Information: Look for important variables and relationships that will guide you in forming equations.
2. Apply Step-by-Step Methods: Use proven strategies, such as substitution or factoring, to simplify and solve the problem.
3. Check Your Work: After solving, always review each step to ensure no mistakes were made during the process.

By developing these techniques and incorporating them into your practice routine, you’ll increase your confidence and ability to tackle any mathematical challenge with ease.

Breaking Down Complex Algebra Problems

When faced with complicated mathematical tasks, breaking them into smaller, more manageable steps is essential for solving them efficiently. This approach allows you to focus on each part of the problem, ensuring that no details are overlooked. By organizing your work and tackling one step at a time, you can transform a daunting problem into a series of simpler challenges.

The first step is to thoroughly read and understand the problem. Identify the variables and relationships that are key to forming equations. Once you have a clear understanding of the problem, break it down into logical steps. Simplify complex expressions, isolate unknowns, and apply appropriate methods such as substitution or factoring.

As you work through each part, keep track of your progress and check your work to ensure accuracy. This method not only makes it easier to solve the problem but also helps build a deeper understanding of the underlying concepts.

Key Formulas and Theorems in Chapter 8

In this section, we explore the essential formulas and theorems that are crucial for solving the exercises. These mathematical principles serve as the foundation for a variety of problems, and understanding them thoroughly will enhance your ability to tackle complex tasks. Whether it’s simplifying expressions or solving for unknown variables, these formulas provide the necessary tools for success.

Important Equations to Remember

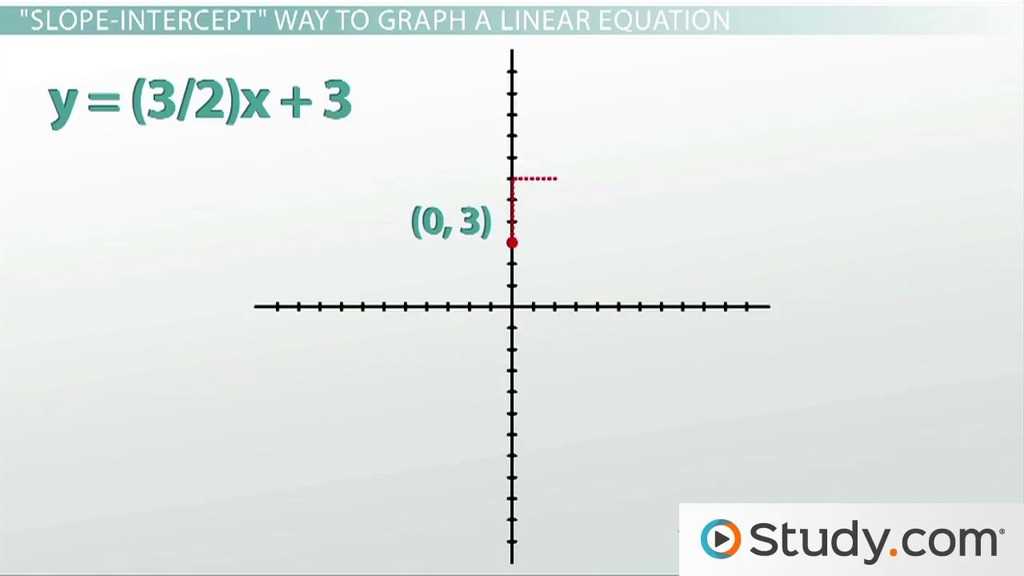
Equations are the backbone of many problems in this section. Here are a few key formulas to keep in mind:

• Quadratic Formula: The quadratic formula is used to find the roots of quadratic equations, expressed as x = (-b ± √(b² – 4ac)) / 2a.
• Factoring Formula: Factoring can be used to solve polynomial equations, breaking them into simpler binomial forms.
• Distributive Property: This principle states that a(b + c) = ab + ac, allowing you to expand expressions more easily.

Key Theorems for Problem Solving

Several theorems play a critical role in understanding how different mathematical elements interact. Some notable ones include:

• Zero Product Property: If the product of two factors is zero, then at least one of the factors must be zero.
• Remainder Theorem: This theorem helps determine the remainder when a polynomial is divided by a linear divisor.

By mastering these formulas and theorems, you’ll be equipped with the tools necessary to efficiently solve a variety of problems in this section.

Practical Applications of Algebra Concepts

The principles and techniques you learn in mathematics are not only abstract concepts but also have real-world applications that can be seen in various fields. Understanding how to apply these methods to solve everyday problems enhances both your problem-solving skills and your ability to navigate the world around you. From budgeting to engineering, these concepts are integral to making decisions and analyzing situations accurately.

One common application is in financial planning, where equations are used to calculate interest rates, loan payments, or investment growth. Another area is in construction, where mathematical principles help in determining dimensions, materials, and costs. Engineers, scientists, and even computer programmers rely heavily on mathematical reasoning to model systems, solve equations, and create algorithms that power technological advances.

By learning how to connect mathematical theories to real-life scenarios, you can not only improve your academic performance but also develop valuable skills that will be useful in a wide range of careers and everyday activities.

Review of Chapter 8 Practice Questions

In this section, we will review the practice problems that help reinforce the concepts covered in this section. These exercises are designed to test your understanding of key topics and provide an opportunity to apply what you’ve learned. By working through these questions, you can assess your strengths, identify areas for improvement, and build confidence in your problem-solving abilities.

Key Problem-Solving Strategies

As you approach these practice questions, it’s important to use effective strategies to simplify the process and find solutions efficiently. Here are a few tips:

• Identify the Known and Unknowns: Begin by clearly defining what information is given and what needs to be solved.
• Break Down Complex Problems: Divide complicated problems into smaller, manageable parts to make them easier to solve.
• Check Your Work: After solving a problem, review each step to ensure that all operations were performed correctly.

Types of Practice Questions

The practice problems in this section cover a range of topics, from simplifying expressions to solving equations. Here are some examples:

• Solving Linear Equations: Questions that focus on solving for an unknown variable in linear equations.
• Factoring Quadratics: Problems that involve factoring quadratic expressions or solving quadratic equations.
• Graphing Functions: Questions related to plotting functions on a coordinate plane and analyzing their behavior.

By reviewing and practicing these questions, you’ll reinforce your understanding and develop the skills needed to tackle more advanced problems in future lessons.

Helpful Resources for Algebra Study

To strengthen your understanding of mathematical concepts, it’s important to make use of various study materials and tools. These resources can help clarify difficult topics, offer practice opportunities, and guide you through challenging problems. Whether you’re a beginner or looking to sharpen your skills, there are plenty of resources available to support your learning process.

Online Learning Platforms

Websites and apps can provide interactive lessons and exercises to improve your skills. Some platforms offer step-by-step solutions, video tutorials, and quizzes to test your knowledge.

• Khan Academy: Free online lessons with practice exercises covering a wide range of mathematical topics.
• Wolfram Alpha: An advanced tool that allows you to compute solutions to complex problems and understand the steps involved.
• Coursera: Online courses from universities and institutions that dive deeper into specific mathematical concepts.

Books and Study Guides

Books provide comprehensive explanations and examples that are essential for thorough understanding. Many study guides also include practice questions and answers to reinforce what you’ve learned.

• “The Art of Problem Solving”: A series of books designed to challenge and enhance problem-solving abilities.
• “Pre-Algebra for Dummies”: A simple, straightforward guide that breaks down core concepts for beginners.
• Practice Workbooks: Printed or digital workbooks that offer a collection of problems to solve, often accompanied by detailed solutions.

By utilizing these resources, you can tailor your study routine to fit your needs, ensuring that you gain a strong grasp of key concepts and improve your problem-solving skills.

Using Visual Aids for Algebraic Problems

In mathematics, visual tools can greatly enhance your understanding and problem-solving ability. By transforming abstract equations and concepts into more tangible forms, visuals help to clarify complex relationships and make patterns more apparent. Using graphs, charts, and diagrams allows you to see solutions unfold, making it easier to approach difficult problems with confidence.

Here are some common visual aids that can support your learning process:

• Graphs: Plotting equations on a graph can help you understand their behavior, such as how they intersect, slope, or shift. Visualizing these elements provides a clearer perspective on how variables interact.
• Diagrams: Diagrams, such as number lines or geometric representations, can break down abstract concepts into simple, visual steps, aiding in the understanding of inequalities, linear functions, and more.
• Tables: Organizing data into tables can help illustrate relationships between variables. They provide a structured way to track values and detect patterns.
• Flowcharts: These can map out problem-solving steps, helping you follow a logical sequence when working through a complex problem.

By incorporating these tools into your study routine, you can transform challenging tasks into more manageable steps, helping you visualize solutions and improve your overall comprehension.

Test Your Knowledge with Chapter 8

It’s essential to assess your understanding of key concepts through practice. Testing your skills not only reinforces the material but also helps identify areas where further improvement is needed. By solving problems related to the topics covered, you can gauge how well you’ve internalized the content and make adjustments to your study plan accordingly.

Here’s a simple way to test your knowledge. Try answering the following practice questions to see how well you can apply the concepts you’ve learned:

Problem	Solution
1. Solve for x: 2x + 3 = 7	x = 2
2. Simplify: 3(2x – 4) = 12	x = 4
3. Evaluate the expression: 5y – 2 when y = 3	y = 13
4. Solve for x: x/4 = 5	x = 20

Use these questions to test your current understanding. If you’re able to solve them correctly, you’re on the right track! If not, revisit the relevant topics and practice more examples until you gain mastery.

Explaining Algebraic Expressions and Equations

Mathematical expressions and equations form the foundation of problem-solving in many areas of study. These forms represent relationships between variables and constants, and they allow us to model real-world situations. Understanding how to work with and manipulate these structures is key to advancing in mathematics and applying these concepts to practical problems.

An expression is a combination of numbers, variables, and operations (such as addition, subtraction, multiplication, or division). For example, the expression 2x + 5 involves the variable x and a constant, which can be evaluated for different values of x.

An equation, on the other hand, is a statement of equality between two expressions. It contains an equal sign (=) and is often used to solve for unknown variables. For instance, the equation 2x + 5 = 15 can be solved by isolating x, yielding a solution that satisfies the equality.

Understanding the difference between these two types of mathematical statements is essential for moving on to more advanced topics. By practicing how to simplify expressions and solve equations, you build the skills necessary to tackle a wide range of mathematical challenges.

Strategies for Efficient Problem Solving

Problem-solving in mathematics requires both critical thinking and a systematic approach. Whether tackling simple or complex questions, having effective strategies can help you solve problems more quickly and accurately. The key to success lies in breaking down each problem into manageable steps and applying the right techniques for each situation.

1. Understand the Problem Clearly

Before jumping into calculations, take a moment to carefully read and understand the problem. Identify the key information provided and determine what you are asked to find. This clarity will guide your approach and ensure that you do not overlook crucial details.

2. Break the Problem into Smaller Parts

Large or complex problems can often seem overwhelming. To avoid this, try to break the problem into smaller, more manageable parts. Solve each section individually, and then combine the results. This method makes the overall problem more approachable and allows for a more organized solution.

Here’s a simple example of breaking down a problem:

Step	Action
Step 1	Read the problem carefully and identify the given information.
Step 2	Write down the equation or expression to be solved.
Step 3	Apply relevant mathematical rules or formulas to simplify.
Step 4	Calculate the solution and check the result.

By following these steps, you can simplify the process and reach an accurate solution with confidence.

Understanding Functions in Algebra Chapter 8

In mathematics, functions play a fundamental role in understanding how different variables relate to each other. A function represents a relationship between inputs and outputs, where each input is mapped to exactly one output. Grasping this concept is essential for solving many types of problems and analyzing mathematical models. Functions allow us to express complex relationships in a more simplified form, making them easier to manipulate and solve.

The Concept of a Function

At its core, a function is a rule that assigns each element in a set of inputs (domain) to a single element in a set of possible outputs (range). This relationship can be expressed using equations, tables, or graphs. Understanding how to interpret and work with functions is crucial for solving problems that involve relationships between variables, such as in real-world scenarios involving physics, economics, and engineering.

Types of Functions

There are different types of functions, each with its own characteristics. Some common examples include:

• Linear Functions: These represent a constant rate of change between variables and are typically graphed as straight lines.
• Quadratic Functions: These involve squared terms and produce parabolic graphs, useful for modeling situations like projectile motion.
• Exponential Functions: These describe rapid growth or decay, commonly seen in population studies and finance.

Identifying which type of function is appropriate for a given problem is key to applying the correct mathematical tools for finding solutions.

Preparing for Chapter 8 Algebra Tests

Effective preparation is key to success when tackling any math assessment. When preparing for a test that covers various mathematical concepts and problem-solving techniques, it’s important to focus on understanding the core principles and practicing problem-solving strategies. This ensures that you can apply what you’ve learned efficiently and with confidence. Below are some strategies that can help you get ready for the test.

Key Preparation Strategies

Here are several steps you can follow to maximize your preparation and improve your chances of success:

• Review Key Concepts: Make sure you understand the major ideas covered, such as functions, equations, and transformations. Be able to recognize and solve different types of problems.
• Practice Regularly: The more problems you solve, the more confident you’ll become. Focus on both simple and complex problems to test your understanding of various techniques.
• Identify Weak Areas: Focus your efforts on areas where you feel less confident. Spend additional time on topics that are particularly challenging or where you’ve struggled in the past.
• Use Study Resources: Utilize textbooks, online tutorials, and practice problems to reinforce your understanding. Study guides and practice exams are great tools to simulate test conditions.
• Time Yourself: Practice solving problems within a set time limit to get accustomed to working under pressure. This will help improve your time management skills during the actual test.

Test-Taking Tips

On the day of the test, it’s important to stay calm and focused. Here are some strategies for managing the test itself:

• Read Questions Carefully: Make sure you understand what each question is asking before you begin solving it. Pay attention to specific details such as units or conditions.
• Start with Easy Questions: Begin by tackling the questions you are most confident about. This will help build momentum and give you a sense of accomplishment early on.
• Double-Check Your Work: After completing the test, go back and review your answers. Look for any mistakes or overlooked steps, especially in more complex problems.

By following these steps and approaching your study sessions methodically, you’ll be well-prepared to take on the test and perform your best.