Cpm Algebra 1 Answers and Solutions

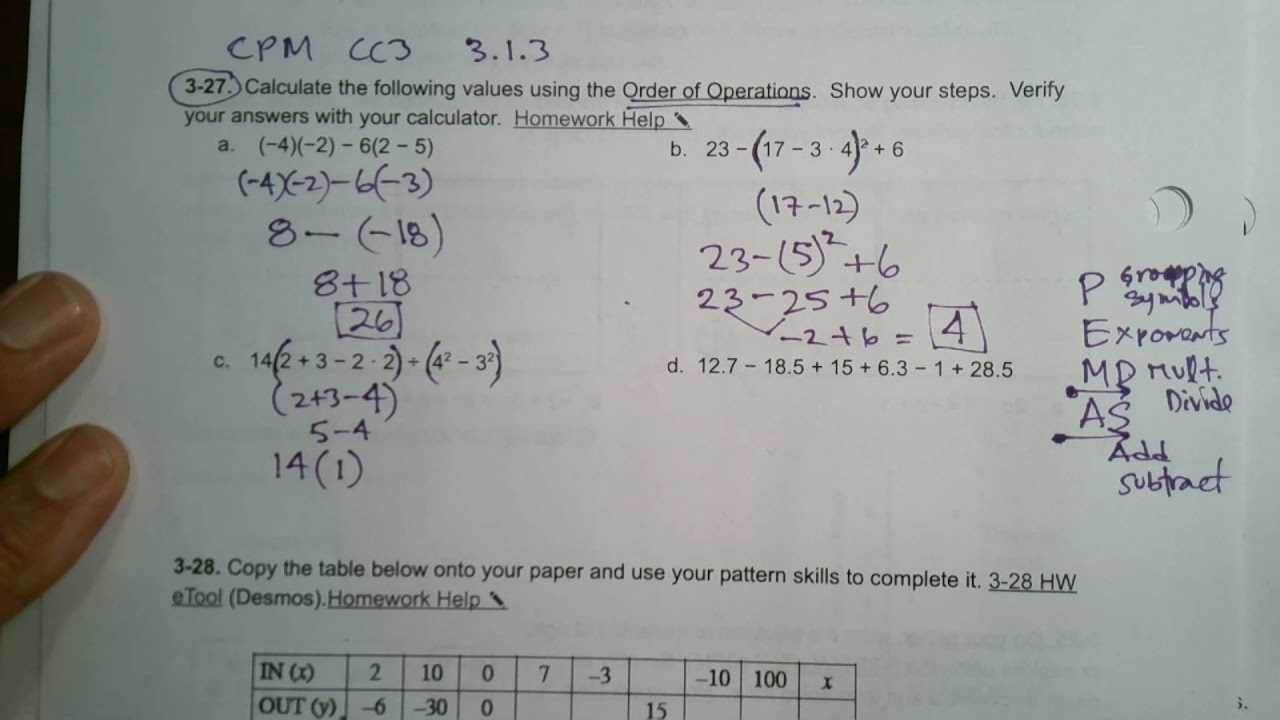
In this section, we explore various methods and techniques for solving mathematical equations and problems commonly encountered in early-level courses. Mastery of these foundational skills is crucial for building a strong understanding of more complex topics later on.

Students often face challenges in applying the correct steps to reach a solution. By breaking down each problem into smaller, manageable parts, learners can effectively navigate through the process. Understanding the principles behind each step is key to developing confidence in mathematical problem-solving.

Throughout this guide, you will find comprehensive solutions and explanations that help clarify tricky concepts. Whether you’re reviewing for an exam or tackling homework, these resources aim to provide a solid foundation to help you succeed in your studies.

Mathematical Problem Solving Solutions

This section provides comprehensive guidance on tackling mathematical problems typically found in introductory-level courses. It emphasizes step-by-step solutions that help clarify how to approach various types of equations and expressions. The goal is to break down each concept into manageable steps, allowing students to gain a deeper understanding and mastery of fundamental techniques.

Steps to Solve Common Problems

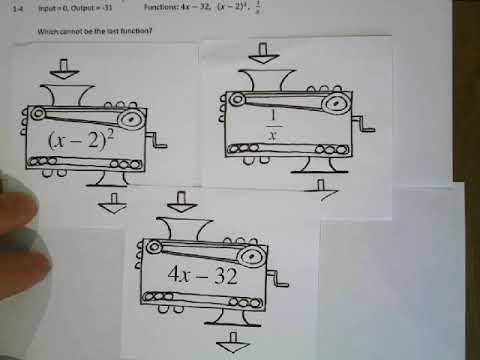
When faced with a mathematical challenge, it’s important to follow a structured approach. Here are the main steps that can help guide you to the correct solution:

1. Identify the type of problem and the necessary operations.
2. Write down the equation clearly and organize the given information.
3. Perform the operations in the correct order, paying attention to mathematical rules.
4. Double-check the final solution to ensure accuracy and consistency.

Key Techniques for Effective Problem Solving

To improve your problem-solving skills, it’s useful to familiarize yourself with the following techniques:

• Understanding Variables: Learn how to interpret and manipulate variables within equations.
• Applying Mathematical Properties: Utilize properties such as the distributive property or the associative property to simplify expressions.
• Recognizing Patterns: Spot recurring patterns in equations that can help simplify problem-solving.
• Reviewing Solutions: After solving, revisit the steps to ensure that the process aligns with mathematical principles.

With consistent practice and a clear approach, mastering problem-solving in mathematics becomes more intuitive. These strategies are essential for building a solid foundation for more advanced topics and ensuring success in future coursework.

Understanding Mathematical Curriculum Structure

This section explores the layout and objectives of the foundational mathematics course, designed to build strong problem-solving and reasoning skills. It emphasizes a step-by-step approach to mastering essential concepts, ensuring that students develop a thorough understanding of key mathematical principles. The curriculum is structured to guide learners through both theory and practical applications, enhancing their ability to solve complex problems.

Core Concepts Covered

The program focuses on several core topics that lay the groundwork for future mathematical success. These include:

• Equations and Expressions: How to work with linear equations and algebraic expressions.
• Functions and Relationships: Understanding how different mathematical quantities relate to one another.
• Problem Solving Strategies: Techniques for approaching word problems and abstract questions effectively.
• Data Analysis and Interpretation: Working with graphs, tables, and data sets to draw meaningful conclusions.

Key Features of the Curriculum

The curriculum is designed with several key features to maximize student engagement and understanding:

• Hands-on Learning: Emphasis on interactive problem-solving and real-world applications.
• Progressive Difficulty: Gradual increase in complexity, starting from basic concepts and advancing to more challenging problems.
• Collaborative Activities: Opportunities for students to work together, helping each other grasp difficult ideas.
• Continuous Assessment: Regular quizzes and assignments to track progress and identify areas for improvement.

By following this curriculum, students can expect to gain a solid foundation in mathematical reasoning, preparing them for more advanced studies and practical problem-solving scenarios in their academic careers.

Why Mathematics is Essential for Students

This subject plays a critical role in shaping a student’s academic and intellectual development. It provides the foundation for understanding abstract concepts, enhancing logical reasoning, and applying problem-solving strategies in various real-world situations. Mastery of basic mathematical principles is essential not only for future academic success but also for everyday life.

Benefits of Learning Core Mathematical Concepts

Understanding core mathematical concepts offers several advantages for students, including:

• Improved Analytical Skills: Students learn to break down complex problems into simpler, manageable parts.
• Enhanced Critical Thinking: Developing the ability to reason and make decisions based on structured analysis.
• Better Time Management: Mathematical thinking encourages students to organize their work systematically, leading to more efficient study habits.
• Boosted Confidence: Gaining mastery over challenging problems builds a student’s self-confidence and persistence.

Application in Everyday Life

Mathematics is not just confined to textbooks. The skills learned in this subject are applicable in many real-life scenarios:

• Financial Management: Understanding basic principles of budgeting, saving, and investing.
• Career Readiness: Many professions, from engineering to economics, require strong math skills for success.
• Logical Decision-Making: The ability to make informed decisions based on data and logical reasoning is valuable in everyday life.

By mastering fundamental mathematical concepts, students lay the groundwork for future academic achievements and gain valuable life skills that will help them in both their personal and professional endeavors.

Key Concepts Covered in Mathematics 1

This course introduces fundamental mathematical principles that serve as the building blocks for more advanced studies. It focuses on developing the skills needed to work with various types of equations, expressions, and relationships. Students will learn to manipulate mathematical structures and apply logical reasoning to solve problems efficiently.

The core topics include:

• Linear Equations: Techniques for solving and graphing equations involving variables, constants, and operations.
• Functions and Relations: Understanding how different values interact within a system and how to express these interactions mathematically.
• Inequalities: Methods for solving and graphing inequalities, helping to understand constraints within real-world problems.
• Systems of Equations: Approaches for solving multiple equations simultaneously, both graphically and algebraically.
• Polynomials: Introduction to expressions involving powers of variables, focusing on addition, subtraction, and multiplication techniques.
• Factoring: Methods for breaking down complex expressions into simpler, more manageable factors.
• Quadratic Equations: Solving equations that involve squared terms, using techniques like factoring, completing the square, and the quadratic formula.
• Rational Expressions: Working with fractions involving variables and learning how to simplify and manipulate them.

Mastering these concepts is essential for students to build a strong mathematical foundation that will be useful in further academic pursuits and practical problem-solving situations in various fields.

How This Approach Differs from Other Methods

This method of teaching mathematics emphasizes active problem-solving and collaborative learning, setting it apart from more traditional approaches. Instead of focusing solely on rote memorization and formula application, the approach encourages students to think critically, work through problems step by step, and engage with the material through real-world applications. This focus on understanding and reasoning helps students retain and apply concepts more effectively.

Focus on Conceptual Understanding

One key difference is the emphasis on conceptual understanding over simple procedural learning. While many traditional methods prioritize memorizing formulas and completing problems based on set procedures, this approach encourages students to grasp the “why” behind the steps. This deeper understanding allows students to apply their knowledge flexibly, even to unfamiliar problems.

Collaborative Learning and Peer Support

Another distinguishing feature is the strong emphasis on collaborative learning. Students are often encouraged to work together, discuss strategies, and help each other solve problems. This peer-driven learning environment fosters a sense of community and allows students to learn from diverse perspectives, strengthening their problem-solving skills.

By incorporating these strategies, this approach provides a more dynamic, student-centered learning experience that promotes critical thinking and long-term mathematical fluency, differentiating it from conventional methods that often prioritize individual performance and memorization over understanding.

Step-by-Step Solutions for Mathematics 1

This section provides detailed, step-by-step solutions to common problems encountered in introductory mathematics. By breaking down each problem into clear and manageable steps, students can follow the logical flow of the solution process, ensuring a deeper understanding of the concepts at hand. This method helps demystify complex equations and allows students to build confidence in their problem-solving abilities.

Example Problem 1: Solving a Linear Equation

Let’s go through an example of solving a basic linear equation:

Problem:	x + 5 = 12
Step 1:	Isolate the variable by subtracting 5 from both sides: x = 12 – 5
Step 2:	Simplify the right-hand side: x = 7
Solution:	x = 7

Example Problem 2: Solving a Quadratic Equation

Now, let’s solve a quadratic equation using factoring:

Problem:	x² – 5x + 6 = 0
Step 1:	Factor the equation: (x – 2)(x – 3) = 0
Step 2:	Set each factor equal to zero: x – 2 = 0 or x – 3 = 0
Step 3:	Solve each equation: x = 2 or x = 3
Solution:	x = 2 or x = 3

Following this process, students can solve a wide range of problems with clarity and accuracy, building a solid foundation in mathematical problem-solving techniques.

Common Mistakes and How to Avoid Them

In any mathematical discipline, there are common errors that students often make, especially when first encountering new concepts. These mistakes can lead to confusion and frustration, but understanding them and learning how to avoid them is key to mastering the material. In this section, we’ll explore some of the most frequent missteps and provide tips on how to overcome them effectively.

Misunderstanding the Order of Operations

One of the most frequent errors is neglecting the order of operations, often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction). Skipping steps or performing operations in the wrong order can result in incorrect answers. To avoid this:

• Always start with parentheses or brackets first.
• Handle exponents before multiplication or division.
• Multiplication and division should be done from left to right, as should addition and subtraction.

Sign Errors When Solving Equations

Another common mistake is mishandling negative signs, especially when distributing or solving equations. For instance, forgetting to apply the negative sign when distributing terms or solving for variables can lead to incorrect solutions. To prevent this mistake:

• Double-check your work when dealing with negative signs, particularly when distributing or factoring.
• Use parentheses to clearly indicate negative values.
• Work through the equation step-by-step, ensuring each sign is properly accounted for.

By being aware of these common pitfalls and practicing careful attention to detail, students can avoid many of the common mistakes and gain greater confidence in their mathematical skills.

Tips for Mastering Mathematics 1 Problems

Mastering mathematical problems requires a combination of strategy, practice, and understanding. Students often struggle with complex problems because they don’t approach them in the most effective way. By following a few helpful tips, you can improve your problem-solving skills and tackle challenges with confidence. In this section, we’ll explore key strategies for mastering the material.

Organize Your Work and Break Down Problems

One of the most effective ways to approach a problem is by breaking it down into smaller, manageable steps. Instead of attempting to solve everything at once, organize your work and handle each component methodically. This reduces mistakes and makes complex problems feel more approachable. Here’s a simple framework for tackling a problem:

Step 1:	Read the problem carefully and identify what is being asked.
Step 2:	Write down any known information and identify unknowns.
Step 3:	Decide on the method or formula to use based on the type of problem.
Step 4:	Perform calculations step-by-step, writing out all work clearly.
Step 5:	Double-check your work to ensure accuracy.

Practice Regularly and Seek Help When Needed

Consistent practice is essential for mastering mathematical concepts. The more problems you solve, the more familiar you become with various techniques. Additionally, don’t hesitate to seek help when necessary. Whether through online resources, study groups, or teachers, getting assistance can clear up any confusion and ensure you’re on the right track.

By incorporating these strategies into your study routine, you’ll be well-equipped to solve problems efficiently and develop a deeper understanding of the material.

Importance of Practice in Mathematics 1 Success

Success in mathematics is largely driven by consistent practice. While understanding concepts is essential, it is through repetition that students gain mastery and confidence. Regularly solving problems helps reinforce learning, exposes students to various problem-solving strategies, and improves their ability to tackle new challenges. In this section, we will explore why practice is crucial and how it contributes to long-term success.

Building Strong Foundations Through Repetition

One of the primary reasons practice is so important is that it solidifies foundational concepts. Each problem presents an opportunity to apply what you’ve learned, reinforcing the core principles. By practicing regularly, students ensure that the material becomes ingrained in their memory, making future lessons and problems easier to understand and solve. Repetition not only helps retain information but also enhances speed and accuracy in solving problems.

Developing Problem-Solving Skills

Another benefit of practice is the development of critical problem-solving skills. The more problems you work through, the more strategies and techniques you develop for handling different situations. By practicing a variety of problems, students learn to identify patterns, simplify complex situations, and use a systematic approach to reach solutions. This flexibility is key to succeeding in mathematics and beyond.

In conclusion, consistent practice is vital for mastering mathematical concepts. It helps students build strong foundations, improve their problem-solving skills, and gain the confidence needed to approach even the most challenging problems. The more you practice, the more capable you become of solving problems efficiently and accurately.

How to Use the Mathematics 1 Workbook

Workbooks are essential tools for reinforcing learning and providing additional practice outside of regular lessons. By working through problems in the workbook, students can improve their problem-solving abilities and better understand key concepts. This section will guide you on how to make the most of the exercises in the workbook to enhance your understanding of the material.

To use the workbook effectively, it’s important to follow a structured approach. This ensures you not only complete the exercises but also maximize the benefits of practicing regularly. Here is a simple strategy to follow when working through the workbook:

Step 1:	Begin by reviewing the chapter or concept in the textbook to understand the key ideas.
Step 2:	Start with easier problems to warm up, gradually progressing to more difficult ones.
Step 3:	For each problem, write down all work clearly, showing each step of your process.
Step 4:	If you get stuck, refer back to examples in the workbook or textbook for guidance.
Step 5:	After completing a section, check your answers (if available) and review any mistakes to understand where you went wrong.

By following these steps, you can ensure that you are using the workbook to its fullest potential. Consistent practice in the workbook not only helps with mastering concepts but also builds confidence in applying techniques to new problems.

Why Mathematics 1 Builds a Strong Foundation

Understanding mathematical concepts early on is crucial for success in higher-level courses and various real-world applications. The first steps in learning mathematical problem-solving provide the tools needed for tackling more complex topics later. This section explains how a solid grasp of foundational concepts is critical to both short-term academic success and long-term intellectual growth.

Essential Skills for Future Learning

The concepts learned in the initial stages of mathematics are not just about solving equations or memorizing formulas. They lay the groundwork for advanced topics such as calculus, statistics, and even fields like engineering and computer science. The skills developed–such as logical thinking, pattern recognition, and problem-solving techniques–are essential for tackling more challenging subjects. Without a firm foundation in these early lessons, progressing to advanced material can become significantly more difficult.

Real-World Applications and Everyday Use

In addition to its academic importance, the knowledge gained in early math courses has practical uses in everyday life. From managing finances to understanding trends in data, mathematical reasoning is an invaluable skill. By mastering basic mathematical principles, students develop the ability to think critically and apply knowledge to solve practical problems, making this foundational course essential for both academic and real-world success.

Ultimately, early mathematical education serves as the cornerstone for future learning, preparing students for a variety of challenges and opportunities that will arise in both academic and professional settings.

How to Solve Word Problems in Mathematics 1

Word problems can be one of the most challenging types of exercises in mathematics, but with the right approach, they can become manageable and even enjoyable. The key to solving these problems is breaking them down into smaller, more understandable steps. This section will walk you through the process of solving word problems and provide strategies to help you approach them confidently.

Steps to Solve Word Problems

To solve word problems effectively, follow these systematic steps:

1. Read the problem carefully: Understand what the problem is asking. Look for key information such as numbers, relationships, and operations.
2. Identify the variables: Assign variables to the unknown quantities in the problem. This will help you translate the problem into a mathematical expression.
3. Translate the problem into an equation: Use the information in the problem to form an equation that represents the situation. Make sure to include all relevant relationships between the quantities.
4. Solve the equation: Use appropriate mathematical techniques to solve for the unknown variable(s). This may involve basic operations such as addition, subtraction, multiplication, or division.
5. Check your solution: Once you’ve solved the equation, plug your answer back into the original problem to ensure it makes sense and satisfies the conditions given in the problem.

Tips for Success

• Draw diagrams: Visualizing the problem with a diagram can make it easier to understand and solve, especially for geometry-based word problems.
• Use units: Always include units in your answers (e.g., dollars, meters, hours). This will help you stay organized and ensure your solution is logical.
• Practice regularly: The more word problems you solve, the more familiar you will become with the strategies and techniques needed to tackle them.

By following these steps and applying these tips, you’ll be able to approach word problems with confidence and improve your problem-solving skills.

Helpful Resources for Mathematics 1 Solutions

When tackling mathematical challenges, having the right resources can make all the difference. There are various tools and platforms that provide valuable support for students seeking solutions and explanations to problems encountered in their studies. Whether you need step-by-step guides, interactive tools, or additional practice, these resources can help reinforce your understanding and improve your problem-solving skills.

Here are some helpful resources you can explore:

• Online Math Solvers: Websites like Wolfram Alpha or Mathway allow you to input problems and receive detailed solutions and explanations. These tools are great for checking your work and understanding the reasoning behind each step.
• Educational YouTube Channels: Channels such as Khan Academy or PatrickJMT offer video tutorials on a wide range of mathematical topics. These videos break down complex concepts into easy-to-understand lessons, perfect for visual learners.
• Math Textbooks and Workbooks: Many textbooks and workbooks come with practice problems and solutions that guide you through problem-solving strategies. These materials often include detailed examples and exercises to deepen your understanding.
• Online Forums and Study Groups: Platforms like Stack Exchange or Reddit’s Math Help forum provide a community of learners and experts who can answer questions and provide insights into tricky problems. These forums allow for interactive learning and collaboration with others.
• Practice Apps: Mobile apps like Photomath or Microsoft Math Solver allow you to scan problems and instantly get solutions along with detailed steps. These apps are especially handy for quick help when you’re on the go.

By utilizing these resources, students can enhance their learning experience and gain a deeper understanding of mathematical concepts. Whether it’s for additional practice, checking solutions, or learning new techniques, the right support is always just a click away.

Real-Life Applications of Mathematics 1

Mathematical concepts learned in foundational courses extend far beyond the classroom. They form the basis for understanding and solving everyday problems across various fields, from budgeting and construction to technology and medicine. This section explores how the skills gained in early mathematics can be applied to real-world scenarios, showing their relevance in both personal and professional life.

Applications in Everyday Life

Many everyday tasks require the use of mathematical principles, often without us realizing it. Here are a few examples:

• Personal Finance: Budgeting and managing expenses require an understanding of basic mathematical operations, such as addition, subtraction, multiplication, and division. Creating savings plans, calculating interest rates, and determining monthly payments all depend on these skills.
• Home Improvement: Whether you’re renovating a room, building furniture, or landscaping, math is used to calculate measurements, areas, and costs. For instance, determining the amount of paint needed for a wall or the number of tiles required for a floor involves applying mathematical formulas.
• Cooking and Recipes: Scaling recipes for different servings requires an understanding of ratios and proportions, which is an essential part of early mathematical learning.

Applications in Various Professions

Mathematics is also critical in numerous professional fields, where advanced problem-solving and analytical skills are required:

• Engineering: Engineers use mathematics to design structures, solve technical problems, and calculate measurements. Whether it’s for building bridges, designing software, or developing machinery, math plays a crucial role in engineering processes.
• Technology and Computer Science: From coding to creating algorithms, math is fundamental in the development of software, apps, and websites. Understanding mathematical patterns and logic is key to problem-solving in the tech industry.
• Healthcare: In fields such as medicine and pharmacology, professionals use mathematical formulas to calculate doses, track medical data, and analyze research results, making math an essential tool in ensuring accurate and effective treatment plans.

The practical applications of early mathematical concepts are vast and varied. By mastering the foundational skills, individuals can solve problems more efficiently, make informed decisions, and navigate a wide range of situations in both their personal and professional lives.

Preparing for Tests in Mathematics 1

Success in mathematics tests requires a blend of effective study strategies, time management, and a deep understanding of the core concepts. Proper preparation is key to performing well and building confidence for upcoming assessments. In this section, we will explore useful tips and techniques to help students excel in their math exams.

Effective Study Strategies

To prepare efficiently for a math test, it is important to adopt a structured approach. Here are some proven study methods:

• Practice Regularly: Consistent practice is essential for mastering mathematical concepts. Work on various problems to strengthen your understanding of different topics.
• Review Key Concepts: Identify the most important formulas, rules, and techniques. Make sure you understand their application thoroughly before the test.
• Use Study Guides: Study guides often highlight critical areas to focus on, offering concise explanations and sample problems that mirror test questions.

Time Management Tips

Managing your time during the study period can significantly impact your performance. Here are a few tips to help with effective time management:

• Set a Schedule: Plan specific study sessions for each topic. Make sure to allocate extra time for areas that you find more challenging.
• Take Breaks: Long study sessions can lead to fatigue. Incorporate short breaks to refresh your mind and maintain focus.
• Prioritize Difficult Topics: Focus on the most difficult or unfamiliar material first. This way, you can tackle the hardest topics when you’re most alert.

Mock Tests and Practice Questions

Practicing with mock tests and sample problems can help you simulate the exam environment and identify areas where you need more practice. Here’s how you can make the most of these tools:

• Take Timed Practice Tests: Set a timer to mimic the real test scenario. This will help you get comfortable with the time constraints and improve your test-taking speed.
• Review Mistakes: After completing practice problems, go over your mistakes and understand why you got them wrong. This is a crucial step in reinforcing concepts and avoiding similar errors during the actual test.
• Seek Additional Help: If you’re struggling with specific problems, don’t hesitate to ask a teacher, tutor, or peer for clarification.

Organizing Notes and Resources

Keeping organized study materials can save valuable time during test preparation. Here’s how to keep your notes and resources in order:

Resource	Purpose
Class Notes	Summarize key lessons, definitions, and explanations that are often referenced during the test.
Textbook	Contains examples, solved problems, and practice questions that can deepen your understanding.
Study Guides	Provide condensed versions of the material with additional practice problems and key insights.

By following these tips and dedicating enough time to your preparation, you can approach math tests with confidence and achieve better results.

Working with Math Study Groups

Collaborating with others in a study group can be a powerful method for mastering mathematical concepts and preparing for exams. When students come together to share their knowledge, solve problems, and discuss strategies, the learning process becomes more dynamic and effective. Study groups not only promote deeper understanding but also encourage accountability and provide a supportive environment for tackling challenging material.

Benefits of Study Groups

Participating in a study group offers numerous advantages that can enhance your learning experience:

• Varied Perspectives: Each member may have different approaches to solving problems, helping to expand your understanding of the material.
• Accountability: Regularly meeting with a group ensures that you stay on track with your studies and remain committed to preparing for exams.
• Motivation and Encouragement: Working with others fosters a sense of camaraderie and keeps you motivated, especially during difficult topics or challenging assignments.
• Clarification of Concepts: Explaining concepts to others can deepen your understanding, while group members can help clarify any confusion you may have.

How to Make the Most of Study Groups

To ensure that your study group is effective, it is important to approach it with a clear plan and goals. Here are some strategies to make the most of your study group sessions:

• Set Clear Goals: Before each session, agree on the topics you want to cover. This helps keep the group focused and ensures that everyone benefits from the meeting.
• Assign Roles: Assign roles to each group member, such as summarizer, problem solver, or question asker. This keeps everyone engaged and ensures that each person’s strengths are utilized.
• Prepare Ahead of Time: Each participant should review the material beforehand so they can actively contribute to the discussion and problem-solving process.
• Practice Together: Solve practice problems as a group. Collaborative problem-solving often leads to discovering new techniques and more efficient methods for tackling difficult questions.
• Respect Differences: Be open to different perspectives and techniques. Respectful discussion encourages learning and helps members grow in their understanding.

By following these guidelines, you can make your study group a valuable resource for success. Working together with others not only boosts your confidence but also strengthens your understanding of the material.

Advancing to the Next Level After Completion

Completing the initial level of mathematics paves the way for students to tackle more advanced concepts. The transition to a higher level of study can be both exciting and challenging. After mastering foundational skills, it is essential to build on this knowledge to prepare for more complex topics. This progression enables students to deepen their understanding and develop the problem-solving skills needed for success in future courses.

What to Expect in the Next Course

As you move on to the next level of mathematics, you will encounter new topics that build on the concepts you’ve already learned. These include:

• Advanced Equations: You’ll work with more complex equations that require deeper reasoning and understanding of relationships between variables.
• Functions and Graphing: Expect to study different types of functions and their graphs, which are fundamental for analyzing real-world problems.
• Polynomial Operations: Polynomial expressions and operations will become a major focus, requiring you to apply your knowledge of factoring and expanding.
• Rational Expressions: You’ll learn to work with expressions involving fractions, which introduces new strategies for simplification and manipulation.

How to Prepare for the Next Level

To successfully transition to more advanced material, it’s important to focus on solidifying your understanding of core concepts. Here are a few strategies to help you prepare:

• Review Key Concepts: Take the time to revisit fundamental principles such as solving equations, understanding inequalities, and working with basic functions.
• Practice Regularly: Consistent practice helps reinforce the skills you’ll need in more advanced topics. Work on problems that challenge your current understanding.
• Seek Additional Resources: Use textbooks, online tutorials, or study groups to fill in any gaps in your knowledge and gain more exposure to upcoming topics.
• Ask for Help When Needed: If any concepts seem unclear, don’t hesitate to seek help from a teacher or tutor. Addressing difficulties early will make the transition smoother.

By preparing in advance and continuing to build on your foundational knowledge, you will be well-equipped to succeed in the next stage of your mathematical journey.