Calculus AB Section 1 Part B Answers

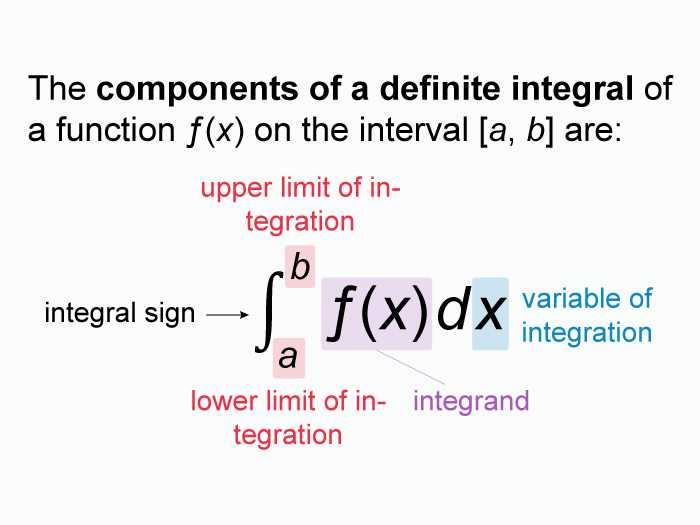
In this portion of the curriculum, students are introduced to some of the fundamental principles that shape the study of higher-level mathematics. These concepts form the building blocks for more complex topics and problem-solving techniques. Grasping the core ideas here is essential for advancing in mathematical studies and applying them to real-world scenarios.

As learners work through exercises and problems, they develop skills in analyzing and solving mathematical challenges. Mastering these methods is critical for success in both academic testing and practical applications. By focusing on the essential strategies, students gain the tools needed to approach more difficult problems with confidence and precision.

Understanding Advanced Mathematics Concepts

This section introduces crucial topics that form the foundation for deeper exploration in mathematical theory. By focusing on specific techniques and strategies, students learn how to approach complex problems and enhance their understanding of mathematical principles. The goal is to develop a solid grasp of essential tools that will serve as stepping stones to more advanced material.

Key Ideas Explored

• The application of limits and their significance in mathematical analysis.
• Strategies for solving derivative-based problems and their real-world uses.
• How continuity plays a critical role in understanding function behavior.

Critical Problem-Solving Techniques

• Applying the chain rule to simplify complex expressions.
• Optimizing solutions and understanding related rate scenarios.
• Exploring the fundamental theorem and its implications for integral work.

By mastering these key concepts, students build the necessary framework to approach more difficult problems with confidence and accuracy, ensuring readiness for future mathematical challenges.

Key Concepts of Advanced Mathematics 1

This section focuses on introducing foundational ideas that are essential for mastering higher-level mathematical reasoning. The material emphasizes understanding core principles that allow for a deeper analysis of functions, limits, and rates of change. These concepts provide a strong base for tackling more complex topics in the future.

At the heart of this material is the exploration of how different mathematical functions behave, with a particular focus on the relationships between variables. Understanding these relationships is crucial for both theoretical applications and real-world problem solving. By developing a solid understanding of these concepts, students are equipped to analyze and interpret various mathematical situations more effectively.

Step-by-Step Solutions for Section 1 Part B

In this section, we will guide you through solving key problems using a structured approach that simplifies complex mathematical tasks. By breaking down each problem into manageable steps, students can better understand the underlying principles and methods required to find the correct solution. This approach ensures clarity and helps build confidence in tackling similar challenges in the future.

Step 1: Understand the Problem

Begin by carefully reading the problem to identify the known and unknown elements. Determine what the question is asking and what information is provided. This is essential for selecting the right method or formula for solving the problem.

Step 2: Apply the Right Techniques

Once the problem is clearly understood, apply the relevant techniques, such as finding derivatives, using limits, or analyzing function behavior. For example, when solving rate of change problems, using the chain rule or product rule can simplify the process.

Step 3: Solve and Verify

Work through the calculations step by step, ensuring that each operation follows logically from the previous one. After reaching a solution, always verify the answer by checking if it satisfies the conditions of the problem or by substituting it back into the original equation.

Following this systematic approach helps ensure that problems are solved accurately and efficiently, allowing for a deeper understanding of the key concepts involved.

Mastering Limits in Advanced Mathematics

Understanding limits is fundamental for analyzing the behavior of functions as they approach specific points. This concept is crucial in determining how functions behave near certain values and plays a key role in many mathematical techniques. Mastery of limits allows students to confidently tackle problems involving continuity, derivatives, and integrals.

Understanding the Basic Concept

The idea behind limits is to examine the value a function approaches as the input gets closer to a certain point, without necessarily reaching it. This approach provides insights into the behavior of functions in areas where direct calculation might not be possible.

Common Techniques for Solving Limit Problems

• Direct Substitution: When possible, simply substitute the value into the function to determine the limit.
• Factoring: If direct substitution leads to an indeterminate form, factoring the function can help simplify the expression and allow for further evaluation.
• L’Hopital’s Rule: This rule is applied when indeterminate forms like 0/0 arise, providing a way to differentiate the numerator and denominator to resolve the limit.

By practicing these techniques, students can gain a deeper understanding of how functions behave under various conditions, which is essential for progressing in more advanced topics.

Techniques for Solving Derivative Problems

Derivatives are central to understanding how functions change and are essential for solving a wide range of problems. By determining the rate of change at any given point, students can gain valuable insights into the behavior of functions. Mastering the methods used to find derivatives enables effective problem solving in various contexts, from motion to optimization.

One of the most common methods for solving derivative problems is the power rule, which allows for the quick differentiation of polynomial functions. Another important technique is the product rule, used when differentiating the product of two functions. The quotient rule, on the other hand, is applied when dealing with the division of functions. Additionally, the chain rule is invaluable when differentiating composite functions, enabling students to break down complex expressions into simpler parts.

By familiarizing oneself with these techniques and practicing their application, learners can confidently solve a broad spectrum of derivative-related problems, ensuring accuracy and efficiency in their work.

Exploring Continuity in Mathematical Problems

Continuity is a crucial concept in understanding how functions behave across different intervals. A function is considered continuous if its graph has no breaks, jumps, or holes, meaning it can be drawn without lifting the pencil. This concept is fundamental when analyzing the behavior of functions and is particularly important in solving problems related to limits and derivatives.

Types of Discontinuity

• Removable Discontinuity: Occurs when a function has a hole at a specific point, but the limit exists at that point.
• Jump Discontinuity: Happens when the left-hand and right-hand limits at a point do not equal each other.
• Infinite Discontinuity: Occurs when the function approaches infinity as it gets closer to a particular point.

Testing for Continuity

To determine whether a function is continuous at a point, it must satisfy three conditions: the function must be defined at that point, the limit of the function must exist, and the limit must be equal to the function’s value at that point. These criteria help identify whether a function behaves smoothly or has any interruptions in its graph.

Understanding continuity is essential for solving advanced problems and applying key concepts in areas like optimization and curve analysis. By mastering this idea, students can more effectively approach real-world applications and theoretical challenges.

Understanding Chain Rule Applications

The chain rule is a fundamental technique used to differentiate composite functions. When a function is made up of two or more functions, the chain rule allows you to find the derivative by applying the rule to each part of the function. This method is essential for simplifying complex expressions and is widely used in problems that involve nested functions or transformations.

How the Chain Rule Works

• Step 1: Identify the outer and inner functions in the composite expression.
• Step 2: Differentiate the outer function, keeping the inner function unchanged.
• Step 3: Multiply the result by the derivative of the inner function.

Common Applications of the Chain Rule

• Trigonometric Functions: Applying the chain rule to functions like sin(x^2) or cos(3x) to differentiate them effectively.
• Exponential Functions: Differentiating expressions like e^(x^2) or a^(g(x)) using the chain rule.
• Logarithmic Functions: Deriving expressions involving composite logarithmic functions, such as ln(x^3 + 1).

By mastering the chain rule, students can handle a wide variety of complex differentiation problems with ease, ensuring that they are prepared for more advanced topics in mathematical analysis.

Common Mistakes in Advanced Mathematics Problems

When tackling complex mathematical problems, it’s easy to make errors that can lead to incorrect results. These mistakes often arise from misunderstandings of key concepts or overlooking important steps in the problem-solving process. Recognizing and addressing these common pitfalls is essential for improving accuracy and building confidence in solving more challenging problems.

One frequent mistake is misapplying differentiation rules, such as confusing the product rule with the chain rule. Another common issue occurs when simplifying expressions–students may incorrectly cancel terms or forget to apply parentheses when necessary. Additionally, failing to check whether a solution satisfies the given conditions can lead to false conclusions, especially when dealing with limits or continuity.

By being aware of these typical errors and taking the time to review each step carefully, students can avoid costly mistakes and develop a stronger foundation in problem-solving techniques.

How to Approach Optimization Questions

Optimization problems require finding the best possible solution, whether it’s maximizing or minimizing a given quantity. These types of problems often involve functions that describe real-world situations, and the goal is to determine the point at which the function achieves its optimal value. By following a structured approach, students can efficiently solve these problems and ensure they are finding the correct solutions.

Steps for Solving Optimization Problems

• Step 1: Identify the quantity to be optimized, such as area, volume, cost, or profit.
• Step 2: Express the objective function in terms of one variable by eliminating unnecessary variables. This often requires using constraints provided in the problem.
• Step 3: Differentiate the function and find the critical points by setting the derivative equal to zero. These points represent potential maxima or minima.
• Step 4: Use the second derivative test or analyze the behavior of the function to determine whether the critical points correspond to a maximum or minimum.
• Step 5: Check the endpoints of the domain if necessary, as the optimal value may occur at these points.

By carefully following these steps, students can efficiently solve optimization problems and gain a deeper understanding of how mathematical concepts apply to real-world situations.

Integrals and Their Role in Section 1

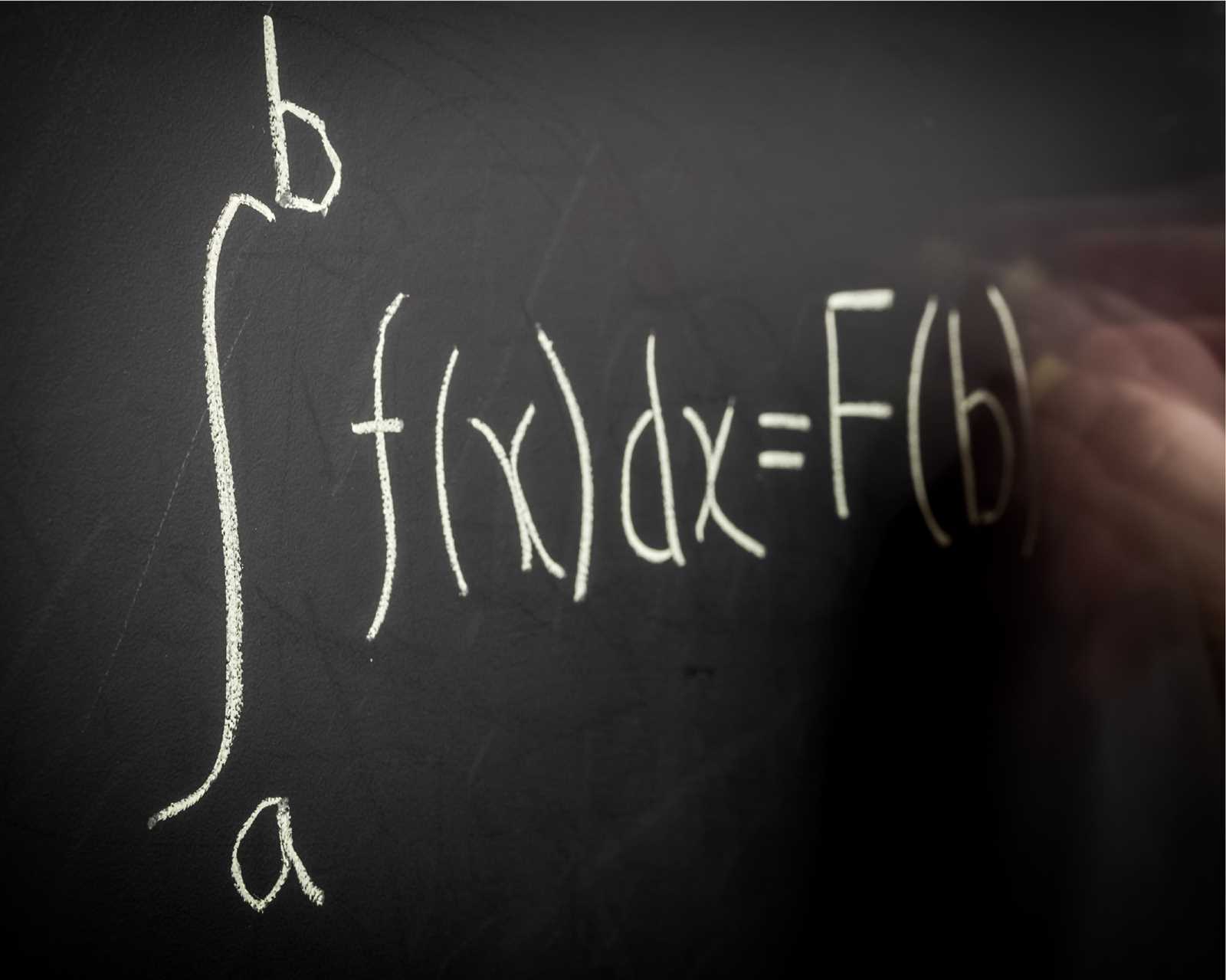
Integrals play a significant role in many mathematical problems, especially when analyzing areas, volumes, and accumulated quantities. In the context of the first part of this material, integrals provide the foundation for understanding how quantities change and accumulate over time or space. These concepts are crucial for solving problems related to accumulation, areas under curves, and even motion, making them indispensable for more advanced studies.

By mastering the process of integration, students can find the total value of quantities that are continuously changing. This includes solving for areas between curves or finding the total accumulation of a function’s value over a specified interval. The fundamental theorem of integration links integration with differentiation, creating a powerful tool for solving a wide range of problems.

In this section, students encounter various integral applications that allow them to calculate areas, volumes, and other quantities that accumulate as a function of time or space. Developing a strong understanding of these techniques is vital for approaching more complex mathematical and real-world scenarios.

Applications of Derivatives in Calculus

Derivatives are powerful tools that allow us to understand how a function behaves at any given point. They provide insights into the rate of change, slope, and behavior of quantities in various real-world contexts. From understanding motion to optimizing functions, derivatives are widely used to model and solve problems in a variety of fields such as physics, economics, biology, and engineering.

Key Uses of Derivatives

• Rate of Change: Derivatives help determine how a quantity changes with respect to another. For example, in physics, the derivative of position with respect to time gives velocity, indicating how fast an object moves.
• Optimization: Derivatives are essential in finding maximum or minimum values of functions. This is especially useful in economics for maximizing profit or minimizing cost.
• Motion and Kinematics: In motion problems, the derivative of position gives velocity, while the second derivative gives acceleration, helping to describe the movement of objects over time.

Real-World Applications

• Economics: Derivatives are used to determine marginal cost, marginal revenue, and other important metrics for business decision-making.
• Medicine: In biology and medicine, derivatives help model population growth rates, the spread of diseases, or changes in drug concentrations over time.
• Engineering: Derivatives are critical in engineering for designing systems that require optimization, such as minimizing energy consumption or maximizing efficiency in machines.

Understanding the applications of derivatives is essential for solving practical problems and making informed decisions in many disciplines. Whether it’s analyzing data, predicting outcomes, or optimizing systems, derivatives provide the mathematical foundation needed to navigate complex challenges.

Graphing Techniques for Calculus AB

Graphing is a crucial skill in understanding the behavior of mathematical functions and their applications. By analyzing the graph of a function, one can gain insights into its key properties, such as intervals of increase or decrease, concavity, and the location of critical points. In this context, mastering graphing techniques is essential for solving problems that involve optimization, rate of change, and other fundamental concepts.

When graphing functions, it is important to consider several factors, such as identifying key features like intercepts, asymptotes, and critical points. These elements provide valuable information about the function’s overall behavior. Additionally, understanding the relationship between a function and its derivative helps in sketching graphs with greater accuracy, particularly when analyzing slopes and concavity.

Steps for Graphing Functions

• Step 1: Identify and plot key points such as intercepts and critical points where the derivative is zero or undefined.
• Step 2: Determine the function’s intervals of increase or decrease by analyzing the sign of its derivative.
• Step 3: Investigate concavity by examining the second derivative. This helps identify inflection points where the curve changes direction.
• Step 4: Sketch asymptotes and any other boundaries that affect the function’s behavior.

By following these steps and understanding the relationships between a function, its derivative, and its graph, students can accurately represent functions and make informed decisions in solving related problems.

How to Tackle Related Rates Problems

Related rates problems involve finding the rate at which one quantity changes with respect to another, often when both quantities are related through a mathematical relationship. These problems are common in various fields, including physics, engineering, and economics, where understanding the connection between different rates is essential for making predictions or solving real-world problems.

The key to solving related rates problems is setting up an equation that relates the changing quantities, differentiating that equation with respect to time, and then solving for the unknown rate. It’s important to carefully interpret the problem, assign variables to the quantities, and use the chain rule to differentiate implicitly when necessary.

Steps to Solve Related Rates Problems

Step	Description
Step 1:	Identify all the given rates and quantities, and assign variables to them.
Step 2:	Write a relationship between the variables, based on the problem’s description (e.g., geometric relationships like volume, area, etc.).
Step 3:	Differentiate both sides of the equation with respect to time, applying the chain rule where needed.
Step 4:	Substitute the known values and solve for the unknown rate.

By following these steps systematically, you can effectively solve related rates problems and apply this technique to a wide range of practical situations. Understanding how different rates influence each other allows you to model complex systems more accurately and find the solutions you need.

Fundamental Theorem of Calculus Overview

The fundamental theorem provides a connection between two major concepts in mathematics: the process of finding areas under curves and the calculation of instantaneous rates of change. This theorem essentially bridges the gap between integration and differentiation, showing how they are related. Understanding this relationship is key to solving many problems in mathematics, especially those that involve both accumulation and rates of change.

In its simplest form, the fundamental theorem states that differentiation and integration are essentially inverse operations. That is, if you integrate a function and then differentiate the result, you essentially get back to the original function (under certain conditions). This concept is powerful, as it allows us to compute integrals more efficiently and understand the underlying structure of many physical and theoretical models.

Key Aspects of the Fundamental Theorem

• Part 1: It establishes the relationship between the derivative of the integral of a function and the function itself.
• Part 2: It shows how to compute definite integrals using antiderivatives, providing a practical method for calculating areas and other accumulations.

By understanding the fundamental theorem, you gain insight into how integration and differentiation work together to describe and solve real-world problems. Whether you are calculating areas, solving differential equations, or analyzing physical systems, this theorem serves as a crucial tool in mathematical analysis.

Real-World Applications of Calculus AB

Mathematical principles like rates of change, optimization, and accumulation are not confined to the classroom but are actively used in various industries. The tools you encounter in this area can be applied to real-world situations ranging from engineering to economics. By applying these concepts, professionals solve complex problems that affect everything from product design to financial forecasting.

For example, understanding how systems change over time allows engineers to design safer bridges, predict the behavior of materials under stress, and optimize energy usage in industrial processes. Similarly, in economics, these mathematical techniques help model market behaviors, determine the most cost-effective production strategies, and predict future financial trends.

Applications in Different Fields

Field	Application
Engineering	Designing structures, calculating stress on materials, optimizing fluid dynamics.
Physics	Modeling motion, understanding forces, predicting celestial mechanics.
Economics	Market analysis, forecasting, optimizing supply chain logistics.
Biology	Modeling population growth, understanding biological systems, calculating drug dosage rates.

By applying the principles learned, individuals in these fields can make more informed decisions, design more efficient systems, and better predict future outcomes. Mastery of these techniques leads to more effective solutions and deeper insights across multiple industries, showcasing the broad impact of these mathematical concepts in the real world.

Tips for Preparing for the Exam

Preparing for a challenging math exam requires both time and strategy. Understanding the concepts thoroughly and practicing problem-solving techniques are key steps to achieving success. Effective preparation goes beyond merely memorizing formulas; it involves building a deep understanding of the material and being able to apply it in different contexts. Here are some tips to help you approach your exam with confidence.

Study Strategies

• Understand the Fundamentals: Focus on grasping the core concepts before diving into complex problems. This will make solving advanced questions easier.
• Practice Regularly: The more problems you solve, the more comfortable you will become with the material. Try solving different types of questions to cover all areas of the subject.
• Review Mistakes: After completing practice problems, take time to review mistakes. Understand where you went wrong and how to correct it.
• Use Multiple Resources: Don’t rely on just one textbook or set of notes. Look for online tutorials, video lessons, or additional practice exams for varied perspectives on the material.

Time Management on the Exam

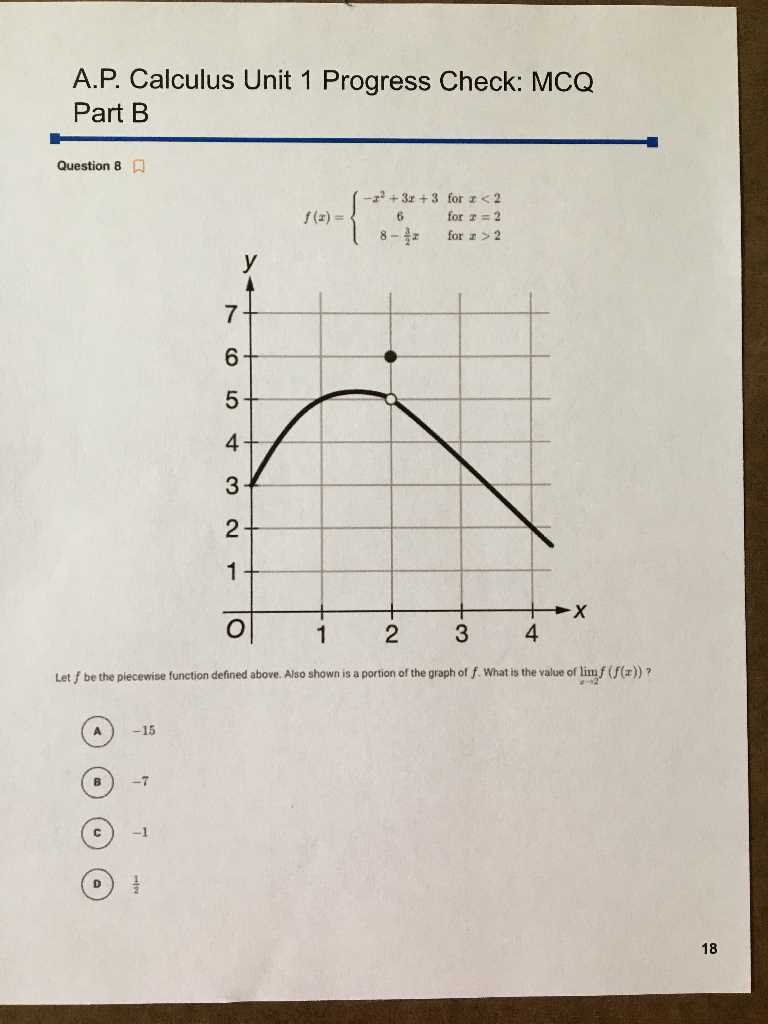
• Prioritize Questions: Start with the problems you are most confident about. This helps build momentum and saves time for more challenging questions.
• Read Carefully: Carefully read each question to ensure you understand what is being asked before jumping into calculations.
• Watch the Clock: Allocate a certain amount of time for each problem and stick to it. Avoid spending too much time on one question at the expense of others.

By following these strategies and focusing on consistent practice, you’ll be well-prepared for the exam. Remember, preparation is not only about knowing the content but also about building the confidence to apply your knowledge under pressure.