Cpm Geometry Chapter 2 Answers and Solutions

In this section, we explore fundamental principles that form the foundation of complex problem-solving in mathematics. With a focus on critical thinking and logical reasoning, this part helps students develop the skills needed to approach and solve challenging exercises effectively.

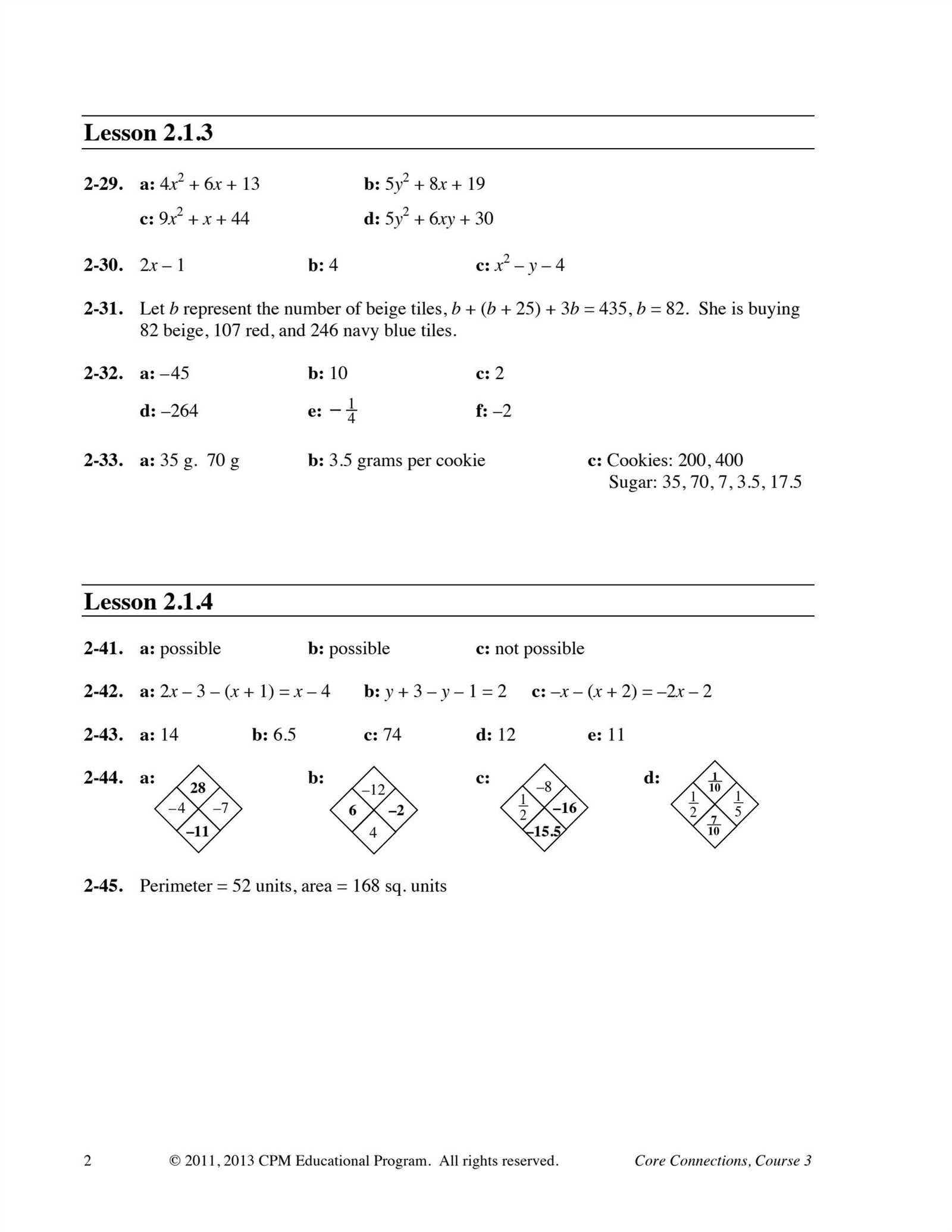
Key strategies for understanding essential formulas and theories are emphasized, ensuring a deeper comprehension of the material. By breaking down each problem into manageable steps, learners gain confidence in their ability to tackle even the most intricate questions.

Whether you are preparing for exams or simply looking to strengthen your mathematical abilities, this guide offers valuable insights and practical tips. The step-by-step approach outlined here is designed to simplify complex concepts and help you excel in your studies.

Cpm Geometry Chapter 2 Solutions

In this section, we focus on solving a range of mathematical problems that challenge your understanding of key concepts. The goal is to provide clear, step-by-step solutions that guide you through the reasoning process, ensuring that every step is understood and logically followed. By addressing various problem types, this part of the material offers practical insights into overcoming common obstacles faced by students.

Breaking Down Problem Solving Techniques

Effective problem-solving involves more than just memorizing formulas. It requires the ability to recognize patterns, apply the right methods, and check your work. Here, we emphasize strategies such as diagramming, identifying known variables, and simplifying expressions. With these techniques, you will be better equipped to tackle a wide variety of challenges.

Important Theorems and Their Applications

Understanding theorems and their applications is crucial for progressing through more advanced topics. By reviewing the core principles and their real-world uses, you can gain a deeper appreciation of the material. From geometric properties to algebraic relationships, mastering these concepts ensures a solid foundation for future learning.

Throughout this section, the focus is on providing comprehensive explanations that enhance understanding and build confidence. Each problem is presented with clarity, offering the support needed to excel in future exercises and tests.

Understanding Key Concepts in Chapter 2

This section dives into the essential ideas and principles that form the foundation of solving advanced mathematical problems. By mastering these core elements, students can approach more complex exercises with confidence. The focus is on developing a thorough understanding of the underlying methods and their practical applications in various scenarios.

Core Techniques for Problem Solving

Grasping the basic techniques is essential for progressing in mathematical studies. Here are some key approaches to focus on:

• Breaking down problems into smaller, manageable parts.
• Identifying relevant information and isolating unknowns.
• Using visual aids like diagrams and charts to simplify complex ideas.
• Checking work through verification and substitution.

Key Principles and Their Applications

The principles discussed here are central to solving a wide variety of problems. By understanding how they apply in different contexts, learners can develop the ability to think critically and solve problems efficiently. Some core concepts include:

1. Properties of shapes and angles that govern spatial relationships.
2. Formulas and equations that provide the framework for calculations.
3. Logical reasoning used to derive conclusions from given information.

By focusing on these fundamental concepts, students will not only improve their ability to solve exercises but also build a solid foundation for more advanced topics in mathematics.

How to Solve Problems Efficiently

Mastering problem-solving involves developing a clear approach to tackling challenges quickly and accurately. The key to efficiency lies in breaking down the process into manageable steps and applying logical reasoning to each part. With the right strategies, you can reduce errors and save time while solving complex problems.

Start by understanding the problem fully before attempting to find a solution. Carefully read through the given information and identify what is being asked. Organize the known variables and unknowns, which will guide the direction of your work.

Using visual tools such as diagrams or graphs can significantly speed up the problem-solving process. These tools help visualize relationships and simplify the process of finding solutions. Once you have a clear understanding of the problem, you can apply the most relevant formulas or principles to move forward efficiently.

Another critical aspect is to check your work regularly. Revisit each step of the process to ensure that calculations are correct and that logic is being applied consistently. This will help avoid mistakes and confirm the accuracy of your solution.

By following these steps and practicing regularly, you’ll be able to approach even the most challenging problems with confidence and efficiency.

Step-by-Step Guide to Solutions

This section provides a detailed, methodical approach to solving problems efficiently. By breaking down each problem into clear steps, it helps you understand the reasoning process and ensures that no detail is overlooked. This step-by-step guide aims to simplify complex tasks and provide the tools needed to reach the correct solution with confidence.

The first step is to carefully read the problem to understand what is given and what needs to be determined. Identify all known variables and unknowns, and think about the most appropriate approach for the problem at hand. This will allow you to formulate a plan before jumping into calculations.

Once you have a plan, the next step is to apply the relevant principles or formulas. Start by applying any known theorems or relationships that directly relate to the problem. If necessary, simplify the expression or use visual aids like diagrams to clarify the relationships between elements.

After performing the calculations or logical steps, review the solution to ensure accuracy. Double-check each step and verify that the answer aligns with the question’s requirements. If the result doesn’t seem right, retrace your steps to identify any errors in reasoning or calculation.

By following these steps and practicing regularly, you’ll develop the ability to solve problems with greater speed and accuracy, mastering even the most complex tasks with ease.

Common Mistakes and How to Avoid Them

When solving mathematical problems, it’s easy to make simple mistakes that can lead to incorrect solutions. Identifying these common errors and understanding how to avoid them is crucial for improving accuracy and efficiency. In this section, we’ll cover frequent pitfalls and provide tips to help you prevent them in your work.

Misinterpreting the Problem

One of the most common mistakes is misinterpreting the question or overlooking important details. To avoid this, always read the problem carefully and highlight key information. Ensure you understand exactly what is being asked before proceeding with any calculations or steps.

Incorrect Application of Formulas

Another frequent error is applying the wrong formula or incorrectly using a known equation. Double-check that you’re using the correct relationships and that you are substituting values into the formula properly. Take the time to review the formula and make sure it matches the problem at hand. This step can save you from unnecessary mistakes.

Tip: Always double-check your work after completing calculations to ensure that no steps were skipped or misapplied. A quick review can catch errors early and save time in the long run.

By being mindful of these common errors and following the steps outlined above, you can improve your problem-solving skills and achieve more consistent results.

Detailed Explanations for Theorems

Understanding the underlying principles that govern mathematical relationships is essential for solving complex problems. This section provides clear and comprehensive explanations of key theorems, breaking them down into understandable steps. By mastering these theorems, you will be better equipped to apply them in various scenarios and confidently tackle difficult exercises.

Theorem of Parallel Lines

One of the foundational principles in geometry involves the relationships between parallel lines and angles. The theorem states that when a transversal intersects two parallel lines, corresponding angles are congruent. This can be used to solve problems involving angle measures and distances. To apply this theorem, it’s crucial to identify parallel lines and transversals in the given figure, then use the properties of angles to find unknown values.

The Pythagorean Theorem

The Pythagorean theorem is another key concept that establishes the relationship between the sides of a right triangle. It states that the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides. This theorem is vital for solving problems related to distances, right-angle measurements, and various geometric shapes. When using this theorem, make sure you correctly identify the hypotenuse and apply the formula appropriately.

Tip: Always review the conditions under which each theorem applies. Understanding the specific requirements will ensure you apply them correctly and avoid common mistakes.

By breaking down these theorems and understanding their real-world applications, you can build a solid foundation for solving more complex problems in mathematics.

Tips for Mastering Problems

Successfully solving mathematical problems requires a combination of strategy, practice, and a deep understanding of the principles involved. To improve your ability to solve problems efficiently, it’s essential to adopt effective techniques and habits. This section offers practical tips to help you tackle challenging exercises with confidence and precision.

One of the most important strategies is to break down each problem into smaller, manageable steps. Approach each problem methodically, starting with identifying known variables, understanding the relationships between them, and determining what needs to be solved. Organizing the problem in this way will help clarify your approach and prevent errors.

Additionally, it’s crucial to practice regularly. The more problems you solve, the more familiar you’ll become with common patterns and problem types. Over time, you’ll develop the intuition needed to recognize shortcuts and apply efficient strategies. Below is a table of useful techniques to improve your problem-solving skills:

Tip	Description
Draw Diagrams	Visual aids like diagrams can help simplify complex relationships and make it easier to see connections between elements.
Check Your Work	Always review your solution to ensure accuracy and confirm that each step follows logically from the previous one.
Use Logical Reasoning	Apply critical thinking to assess the validity of each step. Use known theorems and properties to guide your reasoning.
Stay Organized	Keep your work neat and organized. This will help you follow your thought process and avoid missing important details.

By incorporating these tips into your practice, you’ll be able to tackle problems with greater efficiency and accuracy, ultimately mastering the skills needed for more advanced challenges.

How to Use Diagrams Effectively

Diagrams are powerful tools that can greatly enhance your problem-solving process. They help visualize relationships between elements, simplify complex concepts, and clarify the structure of the problem. By effectively utilizing diagrams, you can quickly identify important details and make the solution process much more manageable.

Start by carefully analyzing the problem to identify the key components that need to be represented visually. Whether it’s a shape, an angle, or a set of relationships, make sure your diagram accurately reflects all of the given information. Label all relevant points, lines, and angles clearly to ensure you don’t overlook any important elements.

Use the diagram to identify patterns and relationships that might not be immediately obvious from the written description. For example, parallel lines, equal angles, or congruent shapes often stand out more easily in a diagram than in a text-based problem. This can help you spot opportunities to apply known theorems or formulas.

Tip: Always check the diagram for accuracy before proceeding with calculations. A small mistake in drawing or labeling can lead to incorrect conclusions.

Lastly, remember that diagrams are not just for illustration; they are essential tools that help you think through the problem. Use them actively throughout the problem-solving process to guide your decisions and ensure that you are applying the correct reasoning at each step.

Practice Exercises for Better Understanding

One of the most effective ways to reinforce your understanding of mathematical concepts is through regular practice. By working through exercises, you not only familiarize yourself with different problem types but also enhance your ability to apply concepts in various contexts. This section provides practice exercises that will help you solidify your skills and build confidence.

Key Benefits of Practice

• Reinforces concepts: Repeatedly solving problems helps strengthen your grasp of core ideas and methods.
• Improves problem-solving speed: The more you practice, the quicker you’ll become at identifying the right strategies and solutions.
• Increases accuracy: Regular exercises help minimize errors and improve the precision of your work.
• Builds confidence: As you solve more problems, you’ll gain a greater sense of accomplishment and confidence in your skills.

Suggested Exercises

1. Solve problems involving basic shapes and their properties, focusing on calculating areas, perimeters, and angles.
2. Work through problems that require applying key theorems and formulas, such as those related to angles or parallel lines.
3. Practice with word problems that involve multiple steps, encouraging you to analyze and break down the information logically.
4. Challenge yourself with more complex problems that require creative application of multiple concepts to find the solution.

By regularly completing these exercises, you’ll not only improve your mathematical skills but also enhance your ability to approach new problems with a methodical and strategic mindset.

Breaking Down Complex Proofs

Solving complex proofs can initially seem overwhelming, but with the right approach, they become manageable and even intuitive. The key to tackling intricate problems lies in breaking them down into smaller, more approachable steps. This section explores strategies for simplifying complex reasoning and systematically working through proofs.

Start by identifying the given information and clearly defining what needs to be proven. Often, a proof will require a sequence of logical steps that build on one another. By organizing your thoughts and planning the sequence of steps, you can see how different pieces of information connect and how to proceed logically from one point to the next.

Another effective technique is to work backwards. Instead of starting from the given information, try to consider the desired conclusion and work in reverse. This can help you spot connections that may not be immediately obvious when approaching the proof from the beginning.

Additionally, use known theorems and properties to fill in the gaps. Proofs often rely on established rules, such as congruence, similarity, and parallelism, which can simplify the process. As you work through a proof, identify opportunities to apply these rules to make the reasoning clearer and more concise.

Finally, always review each step to ensure that each conclusion logically follows from the previous one. A small error or oversight can undermine the entire proof, so careful attention to detail is essential in reaching the correct solution.

Key Formulas from Chapter 2

In this section, we focus on essential formulas that are crucial for solving a wide range of problems. These formulas serve as the foundation for many types of calculations and provide the tools needed to approach complex tasks with confidence. Mastering these formulas will significantly improve your ability to solve problems efficiently and accurately.

Area of a Triangle: The area of a triangle can be calculated using the formula:

Area = 1/2 × base × height.

This formula is fundamental for finding the area of any triangle when the base and height are known.

Pythagorean Theorem: One of the most important principles in mathematics, it relates the lengths of the sides in a right triangle:

a² + b² = c²,

where a and b are the lengths of the legs, and c is the length of the hypotenuse. This theorem is used extensively in many geometric problems involving right angles.

Perimeter of a Rectangle: The perimeter of a rectangle can be determined by:

Perimeter = 2 × (length + width).

This formula is commonly used to calculate the total length of the boundary of rectangular shapes.

Sum of Interior Angles of a Polygon: The sum of the interior angles of a polygon with n sides is given by:

Sum of angles = (n – 2) × 180°.

This formula helps find the total measure of the angles in any polygon, useful for a variety of angle-related problems.

By familiarizing yourself with these key formulas and practicing their application, you can approach geometric problems with greater confidence and accuracy. Keep these formulas in mind as you work through problems to simplify your calculations and strengthen your understanding of the concepts.

How to Tackle Word Problems in Geometry

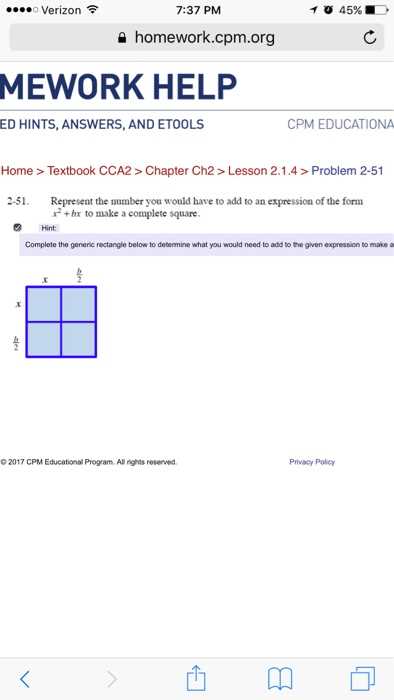
Word problems in mathematics can often feel overwhelming because they require you to translate real-world scenarios into mathematical expressions. However, by breaking down the problem step by step, you can simplify the process and make the solution more manageable. This section outlines effective strategies for solving word problems in geometry, helping you develop a methodical approach to tackle even the most complex questions.

The first step is to carefully read the problem. Identify the key information and determine what the question is asking. Often, word problems contain extra details that aren’t necessary for solving the problem, so focus on the relevant facts. Highlight or underline the important numbers, shapes, or relationships described in the problem.

Draw a diagram whenever possible. Visualizing the problem can help you better understand the relationships between different elements and make it easier to apply mathematical principles. Label all known quantities in your diagram, and include any important angles, lengths, or other details specified in the problem.

Next, set up an equation based on the relationships between the various parts of the problem. This could involve using basic formulas for area, perimeter, or other geometric properties. If the problem requires using multiple formulas, write each equation clearly to avoid confusion.

Lastly, solve the equation step by step, showing all your work to ensure accuracy. After you find the solution, check your answer by reviewing the problem and ensuring it makes sense in the context of the scenario provided.

Step	Action
1	Read the problem carefully and extract key information.
2	Draw a diagram to visualize the problem.
3	Set up the equation based on the given relationships.
4	Solve the equation step by step, showing your work.
5	Review your answer to ensure it makes sense.

By following these steps, you can approach word problems systematically and increase your chances of finding the correct solution. Practice and patience are key, and with time, you’ll become more confident in tackling these types of problems.

Reviewing Core Lessons

In this section, we will revisit the essential concepts from the previous lessons, ensuring a solid understanding of the fundamental principles that are key to solving problems effectively. Mastering these concepts is critical, as they form the foundation for more complex ideas and techniques. Let’s break down the core lessons you’ve learned so far and examine how to apply them in various scenarios.

Key Concepts to Remember

• Understanding Basic Shapes: Familiarize yourself with different shapes and their properties, such as triangles, circles, and quadrilaterals. Knowing the properties of these shapes will make it easier to solve problems involving them.
• Applying Theorems: Be comfortable using fundamental theorems, such as the Pythagorean theorem, to find relationships between sides, angles, and distances.
• Using Formulas: Remember the essential formulas for area, perimeter, and volume. These will frequently come up in various types of problems and need to be used correctly.
• Angles and Their Relationships: A strong understanding of angles, including complementary, supplementary, and vertical angles, is crucial for solving geometric problems.

Strategies for Reinforcement

1. Practice Regularly: Solving practice problems regularly helps reinforce the concepts and improve problem-solving speed.
2. Visualize Problems: Drawing diagrams or using models to visualize geometric relationships aids in understanding how different elements interact.
3. Work with Examples: Reviewing solved examples can help identify common problem types and familiarize you with the techniques used to solve them.
4. Review Mistakes: When mistakes occur, take the time to understand where the error happened and how to avoid it next time.

By revisiting these core concepts and applying them in various situations, you can ensure a strong foundation and improve your problem-solving skills. Consistent practice and a clear understanding of the key principles will help you approach more complex problems with confidence.

Helpful Resources for Students

Whether you’re just beginning to study or looking to deepen your understanding, there are several resources available that can support your learning process. These tools can help clarify concepts, provide additional practice, and offer different perspectives on the material. From textbooks to online platforms, the right resources can make a significant difference in mastering the subject.

Online Platforms and Tools

• Khan Academy: A free, comprehensive resource that offers lessons on various topics, including visual tutorials and practice exercises to help reinforce key concepts.
• Wolfram Alpha: An advanced computational tool that can help solve complex problems and provide step-by-step explanations.
• Desmos: A powerful graphing calculator that allows you to visualize geometric shapes, equations, and transformations, making it easier to understand spatial relationships.
• GeoGebra: An interactive tool for visualizing and solving problems related to geometry and algebra, ideal for both beginners and advanced learners.

Textbooks and Study Guides

• Study Guides: Many publishers offer supplemental study guides that break down key ideas in simpler terms, offering additional examples and exercises.
• Textbook Solutions: Many textbooks provide solutions to problems at the end of chapters or via online platforms, which can be helpful for reviewing and self-checking your progress.
• Practice Workbooks: These workbooks are designed for additional practice and focus on problem-solving skills, which are crucial for mastering the material.

By utilizing these resources, students can gain a deeper understanding of the material, reinforce their skills, and tackle more complex problems with confidence. Consistent use of these tools will contribute to better comprehension and success in the subject.

Strategies for Preparing for Tests

Preparing for a test requires more than just reviewing notes; it involves mastering the concepts, practicing problem-solving techniques, and managing your time effectively. A strategic approach can help reinforce your understanding and build confidence for the test day. The following methods focus on strengthening key skills and ensuring you’re fully prepared to tackle any challenge presented during the exam.

1. Review Key Concepts and Formulas

Start by revisiting the core principles and formulas that are central to the subject. These form the foundation of most problems, and understanding them thoroughly will allow you to apply them in various scenarios. Make sure you can:

• Recall important formulas without hesitation.
• Understand how to derive formulas if needed.
• Recognize when and how to use specific principles in different problems.

2. Practice Problem Solving

The best way to prepare for any test is through consistent practice. Try solving problems from different sources and difficulties to expose yourself to a variety of question types. This approach helps you:

• Identify common patterns in problems and their solutions.
• Increase speed and accuracy under timed conditions.
• Build confidence in applying concepts to unfamiliar situations.

By focusing on both concept review and active problem-solving, you ensure a comprehensive preparation. Regularly testing yourself with different problem types and under timed conditions can help reinforce your skills and prepare you for the exam with a clear and focused mindset.

Applying Concepts to Real Life

Understanding theoretical concepts is essential, but knowing how to apply them in everyday situations is what truly solidifies your knowledge. Real-world applications make abstract ideas more tangible and demonstrate their relevance. From architecture to art, and from engineering to nature, many professions and aspects of life rely on these fundamental principles. By connecting lessons to real-world scenarios, you can better appreciate the practical utility of what you’re learning.

1. Architecture and Design

Architects and designers use principles of shapes, sizes, and spatial reasoning in the creation of buildings, bridges, and public spaces. Here are a few examples of how these concepts are applied:

• Calculating angles and measurements to ensure structures are stable and balanced.
• Designing floor plans with precise dimensions to optimize space and function.
• Using symmetry and proportionality to create aesthetically pleasing designs.

2. Nature and the Environment

Natural patterns and structures are often governed by geometric principles. Understanding these relationships can help in fields like biology and environmental science:

• Recognizing the symmetry in flowers and leaves.
• Analyzing the shapes and patterns in animal shells and bones.
• Understanding the geometry behind natural forces like erosion or water flow.

By linking theoretical concepts to tangible outcomes in various fields, we gain a deeper understanding of how our knowledge can influence and improve the world around us.