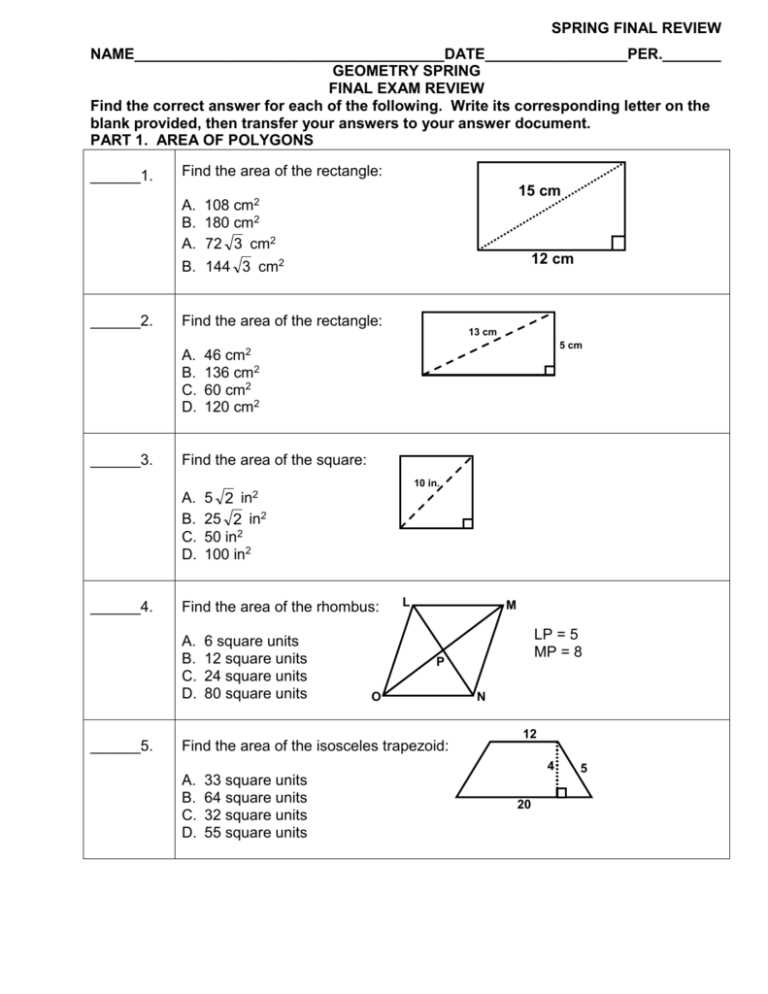
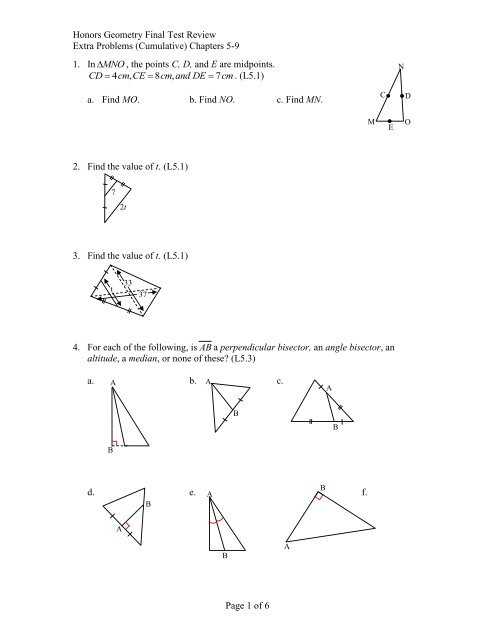
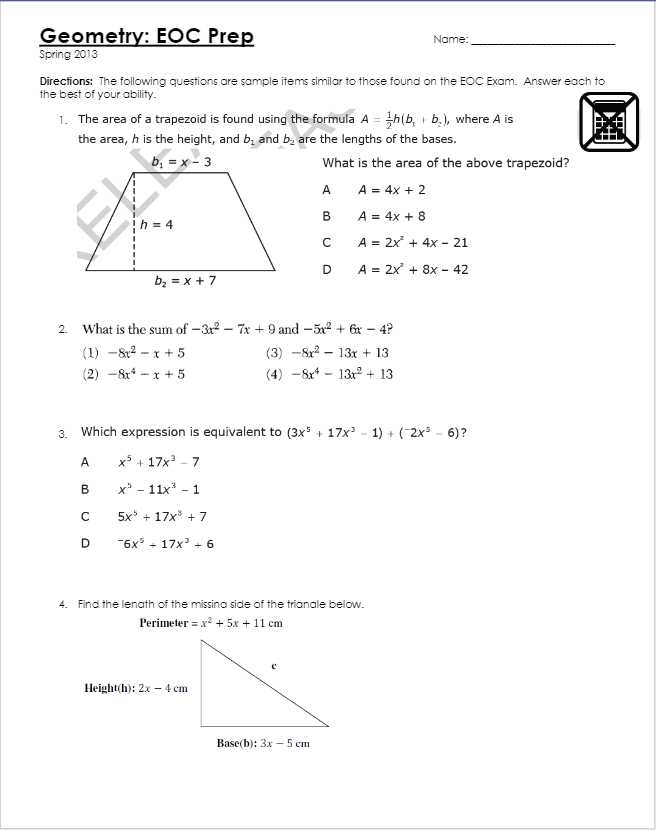
Geometry Spring Final Exam Answers and Study Tips

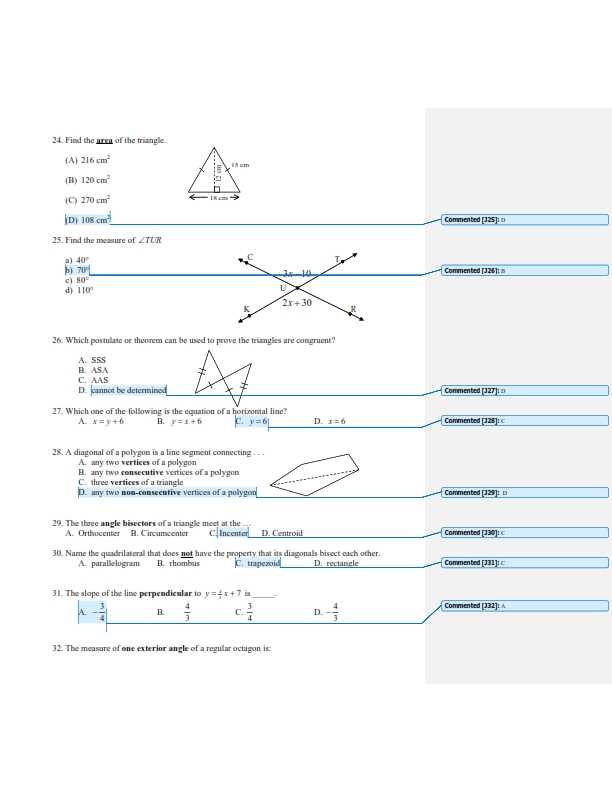
As you approach the upcoming assessment, it’s crucial to review key concepts and techniques that will help you perform at your best. Understanding the core principles and practicing problem-solving strategies are essential for success. This section offers a comprehensive guide to aid in your preparation, providing you with the tools needed to tackle a wide range of problems.

Mastering critical topics and refining your skills will allow you to navigate complex problems with confidence. By focusing on essential areas and reviewing relevant methods, you can improve your problem-solving abilities and enhance your overall performance. Whether it’s through visualizing concepts, applying formulas, or solving practical examples, this guide is designed to give you a solid foundation for achieving the results you aim for.

Assessment Solutions and Key Concepts

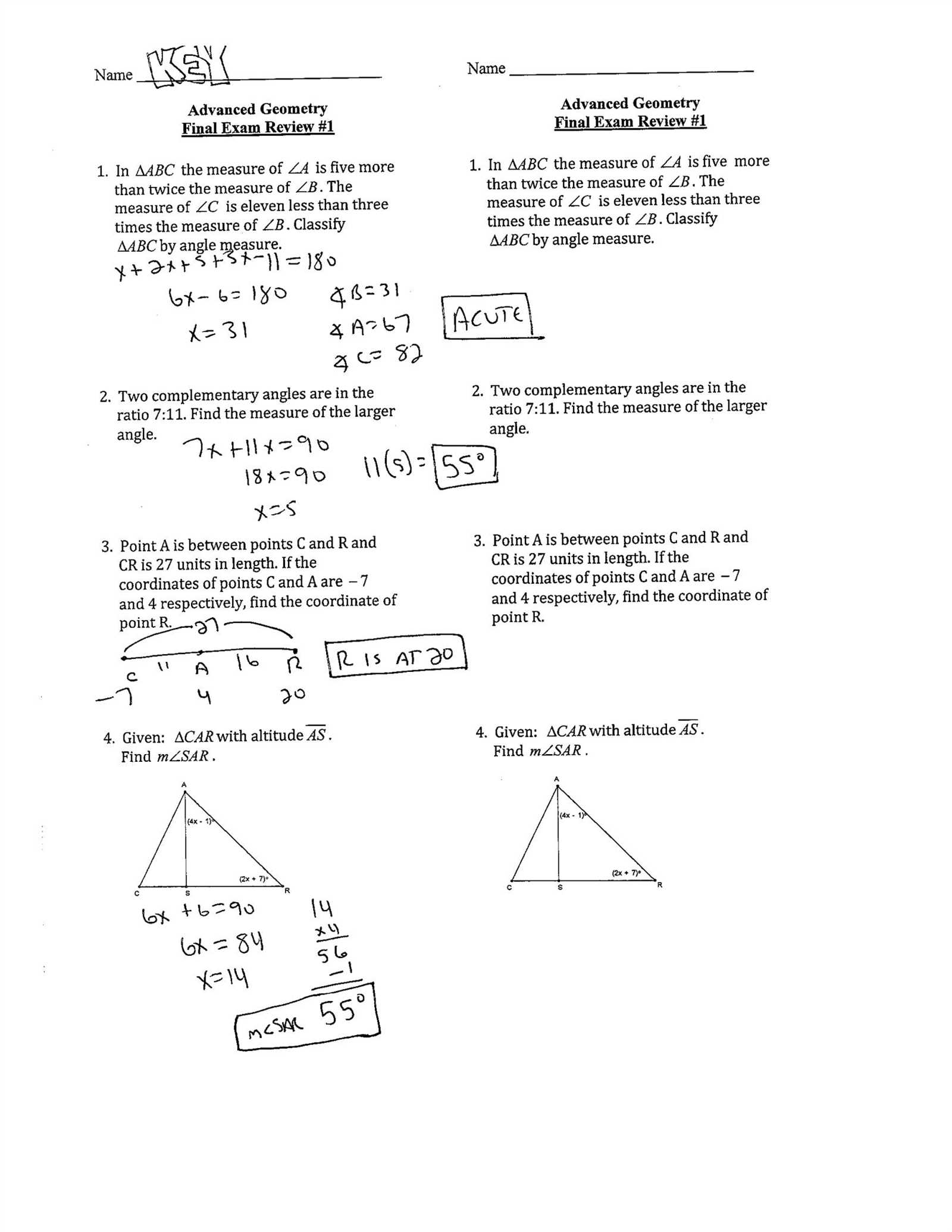
In this section, we delve into the critical solutions and strategies for success in your upcoming assessment. To excel, it’s essential to understand the fundamental principles and be able to apply them effectively to a range of problems. This guide provides the necessary steps and problem-solving techniques to help you approach the challenges with confidence.

Focus on the most important areas, such as calculating areas, solving equations, and working with different types of shapes and figures. By mastering these concepts, you will be prepared for any problem that may appear. Practice with relevant examples and learn how to manage your time effectively to ensure the best possible outcome on test day.

Key Topics Covered in Assessments

To succeed in any challenging assessment, it’s important to focus on a few core areas that often appear in various problem types. These topics form the foundation of the material and will allow you to tackle a wide range of questions confidently. Understanding the relationships between shapes, numbers, and their properties will guide your problem-solving approach.

Shapes, Angles, and Their Properties

One of the most crucial areas is the understanding of different shapes and their associated properties. This includes identifying types of triangles, quadrilaterals, and circles, as well as knowing how to calculate angles within these figures. Mastering these concepts enables quick identification of key information needed to solve problems efficiently.

Formulas and Measurements

Another important aspect is being familiar with the necessary formulas to calculate various measurements, such as area, perimeter, volume, and surface area. Knowing when and how to apply these formulas is vital in solving questions accurately. Consistent practice with these formulas ensures that you can approach calculations with speed and precision under pressure.

How to Approach Geometry Problems

When tackling complex problems, it’s essential to break them down into smaller, more manageable steps. Understanding the question and identifying what is being asked is the first step in finding a solution. Once you have a clear grasp of the problem, using a systematic approach will guide you toward the correct answer.

Start by identifying the key elements of the problem, such as the shapes involved, the given measurements, and any relationships between them. Next, look for patterns or known formulas that can simplify the task. Using visual aids, like diagrams, can often help in organizing your thoughts and ensuring you don’t miss crucial details. Step-by-step problem-solving ensures that no step is overlooked and helps you stay organized throughout the process.

Common Formulas You Need

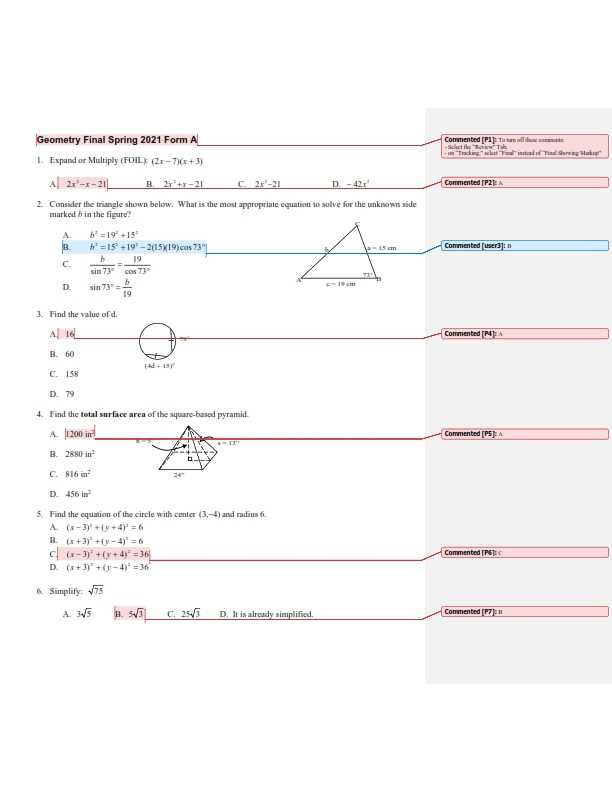
When solving problems involving shapes and measurements, there are a few key formulas that you’ll need to know. These formulas form the backbone of calculations involving areas, volumes, and other geometric properties. Having these at your fingertips ensures that you can quickly and accurately solve a variety of problems.

Area and Perimeter of Polygons

For basic polygons like squares, rectangles, and triangles, the formulas for calculating area and perimeter are essential. The area of a rectangle is calculated as length × width, while the perimeter is the sum of all sides. Similarly, for a triangle, the area is 1/2 × base × height, and the perimeter is the sum of the lengths of its sides. Mastering these basic formulas allows you to tackle a wide range of problems efficiently.

Volume and Surface Area of Solids

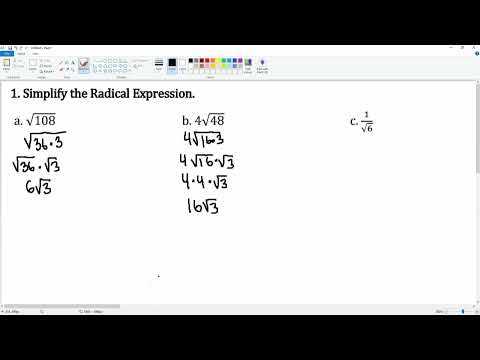
When dealing with three-dimensional objects, knowing the formulas for volume and surface area is crucial. For example, the volume of a rectangular prism is calculated as length × width × height, while the surface area involves adding up the areas of all faces. Understanding these calculations for shapes like cubes, spheres, and cylinders is key to solving more complex problems in spatial reasoning.

Tips for Solving Proofs Efficiently

Solving logical proofs requires a structured approach and a clear understanding of the relationships between different elements. The goal is to demonstrate the truth of a given statement by logically connecting known facts and principles. By following a systematic method, you can approach these problems with confidence and accuracy.

Start by carefully reading the statement you are asked to prove and identify the given information. Next, organize your thoughts and begin with what you know to be true. Use definitions, postulates, and previously proven theorems to support your steps. It’s helpful to work backward in some cases, starting from what you’re trying to prove and finding a path that leads to the given facts. Writing out each step clearly and justifying every move will not only help you stay organized but also ensure that your logic is sound.

Understanding Geometric Shapes and Properties

To solve problems effectively, it’s essential to have a clear understanding of the various shapes and their inherent properties. Each shape has specific characteristics that define how it behaves and how its measurements are calculated. Knowing these attributes will enable you to approach problems with a better grasp of the relationships between different figures.

For example, recognizing the differences between polygons like squares, triangles, and circles, and understanding their respective properties such as sides, angles, and symmetries, can make calculations easier. Additionally, knowing how to apply the properties of parallel lines, congruent shapes, and angles will help you solve more complex problems. Mastering these concepts ensures that you are equipped to work with a wide variety of challenges and can draw connections between different geometric elements.

Mastering Coordinate Geometry for Finals

Coordinate geometry is a powerful tool for solving problems involving points, lines, and shapes on a coordinate plane. By understanding the relationships between coordinates and applying key formulas, you can efficiently solve various types of problems. This section will help you build a strong foundation in this area, ensuring you are prepared to tackle any related questions.

Understanding the Basics

To begin, it’s important to be comfortable with the basic concepts, such as plotting points on a coordinate plane, calculating the distance between two points, and finding the slope of a line. These fundamental skills serve as the building blocks for more complex problems. The formulas used for these calculations are essential to understand, as they provide the means to solve a variety of geometry-related questions.

Key Formulas You Should Know

Here are some of the key formulas in coordinate geometry that you need to master:

Formula	Purpose
Distance Formula: √((x2 – x1)² + (y2 – y1)²)	Calculates the distance between two points
Slope Formula: (y2 – y1) / (x2 – x1)	Finds the slope of a line between two points
Midpoint Formula: ((x1 + x2)/2, (y1 + y2)/2)	Finds the midpoint of a line segment

By practicing these formulas and understanding their applications, you’ll be able to solve problems involving lines, shapes, and distances more confidently. Make sure to practice with different examples to get a firm grasp on using these formulas in various contexts.

Strategies for Tackling Word Problems

Word problems can often feel overwhelming due to the mix of textual information and mathematical concepts. However, by following a structured approach, you can break down the information into manageable steps and identify the key elements needed to find a solution. This section outlines effective strategies to approach these types of problems with confidence.

Read the Problem Carefully

The first step in solving any word problem is to read it thoroughly. Take note of what is being asked, the data provided, and any relationships or conditions mentioned in the text. Underlining or highlighting important numbers and keywords will help you focus on the relevant information. Sometimes, rephrasing the problem in your own words can make it easier to understand.

Identify the Right Approach

After understanding the problem, the next step is to determine which mathematical approach or formula to use. Often, word problems involve solving for unknown values using known relationships, such as ratios, areas, or distances. Start by identifying the type of problem and the equations that may apply. Once you’ve selected the appropriate method, carefully apply the formula or logic step by step.

Taking time to break down the problem into smaller, more manageable parts will help ensure that you don’t overlook key details. With practice, these strategies will allow you to tackle even the most complex word problems effectively.

Using Diagrams to Solve Problems

Visual aids are incredibly helpful when tackling complex problems, especially those involving shapes, distances, and angles. By drawing diagrams, you can make abstract concepts more tangible and better organize the information. A well-drawn diagram can clarify relationships between elements, helping you identify key details that may be easy to overlook in text alone.

Start by sketching a clear, labeled diagram based on the problem’s description. Mark the known quantities and angles, and look for any symmetries or geometric properties that might simplify the task. In many cases, seeing the problem visually will help you recognize patterns or apply formulas more effectively. Diagrams are particularly useful when you need to calculate areas, distances, or angles, as they provide a clear reference for your calculations.

Once the diagram is complete, use it to guide your logical reasoning. Often, the next steps in solving the problem will become more apparent when you can see the relationships between the different parts of the shape. With regular practice, you will find that using diagrams becomes an essential tool in your problem-solving toolkit.

Reviewing Angles and Their Relationships

Angles are fundamental components in solving many problems, as they define the orientation of lines and shapes. Understanding the different types of angles and their relationships with one another is essential for tackling a wide range of problems. Whether you’re working with parallel lines, intersecting lines, or various polygons, recognizing angle properties helps you apply the correct formulas and reasoning.

Types of Angles

There are several key types of angles that you need to be familiar with. Each type has its own properties and rules, making them important when analyzing geometric situations.

• Acute Angles: Angles less than 90°
• Right Angles: Angles exactly 90°
• Obtuse Angles: Angles greater than 90° but less than 180°
• Straight Angles: Angles exactly 180°
• Reflex Angles: Angles greater than 180° but less than 360°

Angle Relationships

Understanding how angles relate to each other can simplify the process of solving problems. Here are some key angle relationships that often come into play:

1. Complementary Angles: Two angles that add up to 90°.
2. Supplementary Angles: Two angles that add up to 180°.
3. Vertical Angles: When two lines intersect, the opposite angles are equal in measure.
4. Adjacent Angles: Two angles that share a common side and vertex.

By reviewing these key types and relationships, you will be better prepared to identify and work with angles in various geometric situations. Understanding these concepts is vital when proving theorems or solving problems involving shapes and lines.

How to Calculate Area and Volume

Understanding how to calculate the area and volume of various shapes is a crucial skill in many mathematical problems. These calculations help determine the space occupied by two-dimensional surfaces and three-dimensional objects. Knowing the appropriate formulas for different shapes will allow you to solve problems more efficiently and accurately.

Calculating Area

Area is the measure of the surface of a shape. The formula you use depends on the type of figure you’re working with. Below are some common shapes and their area formulas:

• Rectangle: Area = length × width
• Square: Area = side²
• Triangle: Area = ½ × base × height
• Circle: Area = π × radius²
• Parallelogram: Area = base × height

For irregular shapes, you might need to break them into smaller, manageable shapes and calculate each area individually before summing them up.

Calculating Volume

Volume measures the amount of space inside a three-dimensional object. The formula for volume varies depending on the shape. Here are some standard formulas:

• Cube: Volume = side³
• Rectangular Prism: Volume = length × width × height
• Cylinder: Volume = π × radius² × height
• Sphere: Volume = ⁴/₃ × π × radius³
• Pyramid: Volume = ⅓ × base area × height

When calculating volume, make sure to measure all dimensions accurately and use the correct units. If necessary, convert units to ensure consistency throughout the calculation process.

Practice Problems to Test Your Skills

Engaging in practice problems is one of the best ways to solidify your understanding and boost your problem-solving abilities. By applying the concepts you’ve learned to various exercises, you can improve your accuracy, speed, and confidence. Here are some problems to test your knowledge in a variety of topics related to shapes, measurements, and calculations.

Basic Shape Calculations

Start with these simple exercises to refresh your skills in calculating areas, perimeters, and volumes of common shapes:

Problem	Question	Solution
1	Find the area of a rectangle with a length of 8 cm and width of 5 cm.	Area = length × width = 8 × 5 = 40 cm²
2	Calculate the volume of a cube with a side length of 4 cm.	Volume = side³ = 4³ = 64 cm³
3	Determine the area of a circle with a radius of 7 cm (use π ≈ 3.14).	Area = π × radius² = 3.14 × 7² = 3.14 × 49 = 153.86 cm²

Intermediate Challenges

Once you’ve mastered the basics, try these more complex problems involving angles and relationships between different elements:

Problem	Question	Solution
1	If two angles are complementary, and one angle measures 35°, what is the other angle?	Complementary angles add up to 90°. 90° – 35° = 55°.
2	In a triangle, if one angle measures 45° and another measures 60°, what is the third angle?	The sum of angles in a triangle is 180°. 180° – (45° + 60°) = 75°.
3	Find the length of the hypotenuse in a right triangle with legs measuring 3 cm and 4 cm.	Using Pythagoras’ theorem: hypotenuse² = 3² + 4² = 9 + 16 = 25. Hypotenuse = √25 = 5 cm.

Working through these problems will help reinforce your understanding of important mathematical concepts. Make sure to review your solutions and identify any areas where you need further practice.

Time Management for Geometry Exams

Effectively managing your time during assessments is crucial to maximizing your performance. Proper time allocation allows you to complete all tasks within the given duration, while reducing stress and improving accuracy. Here are strategies to help you manage your time efficiently when tackling mathematical assessments.

Set a Time Limit for Each Section

Start by dividing your available time into smaller blocks for different sections of the assessment. This will ensure that you dedicate appropriate time to each type of problem, preventing you from spending too long on any one question.

• Allocate more time to complex problems, but don’t spend too much time on a single question.
• Set a time limit for multiple-choice or short-answer questions that are easier to solve.
• Reserve some buffer time for reviewing your work at the end.

Prioritize Questions Based on Difficulty

It’s often tempting to start with the easiest questions, but prioritizing based on difficulty can help you use your time more wisely.

• Start with the questions you feel most confident about to gain momentum.
• If you encounter a particularly challenging problem, move on and come back to it later, if time permits.
• Make sure to tackle the questions that carry the most points first.

Practice Under Timed Conditions

Before the actual test, simulate exam conditions by solving practice problems within a set time frame. This will help you get comfortable with managing time under pressure.

• Use a stopwatch or timer to track your progress while solving practice problems.
• Evaluate your speed and adjust your time allocation strategy as needed.

Stay Calm and Focused

Keeping your composure during the assessment is essential. When you stay calm, you’re less likely to waste time on nervous mistakes. Keep an eye on the clock, but don’t panic if you run out of time–prioritize accuracy over speed.

• Take deep breaths if you feel overwhelmed and refocus on the task at hand.
• Use every second wisely, but avoid rushing through questions in a way that leads to careless errors.

By following these strategies, you can approach the assessment with confidence and complete each section within the allotted time, ensuring that you’re able to demonstrate your full understanding of the material.

Dealing with Geometry Mistakes

Making mistakes is a natural part of the learning process, especially when solving complex problems. It’s important to view errors as opportunities for growth, as they provide valuable insights into where improvements can be made. Here are some strategies for managing mistakes effectively and learning from them during assessments.

Review Your Work Carefully

One of the best ways to avoid repeating mistakes is by reviewing your work thoroughly. Take time to check each step of your solution, even if you feel confident about your answer. Errors often stem from simple miscalculations or overlooked details, so carefully revisiting your process can help catch them.

• Start by verifying your final answer against the problem to ensure it makes sense.
• Look for common mistakes, such as incorrect signs, misinterpreted data, or skipped steps.
• If a solution doesn’t seem right, retrace your steps and rework the problem methodically.

Understand the Root Cause

Rather than simply fixing the immediate error, take time to understand why the mistake occurred in the first place. Identifying the root cause will help prevent similar mistakes in the future.

• Was it a lack of understanding of a specific concept or principle?
• Did you misinterpret the instructions or overlook key information?
• Was the error due to a misstep in your calculation process?

Learn from Mistakes and Practice More

Once you’ve identified the cause of the error, take the time to practice similar problems until you’re confident in your approach. Repeated practice will help reinforce the correct methods and reduce the likelihood of making the same mistake again.

• Find practice problems that challenge your weak points and focus on those areas.
• Rework the problems that caused mistakes to solidify your understanding of the correct approach.
• Don’t shy away from challenging problems; they’re opportunities to improve.

Stay Positive and Keep Going

It’s easy to get frustrated when mistakes occur, but maintaining a positive mindset is essential. Remember that errors are part of the journey toward mastery. Stay calm, keep a clear head, and continue working through challenges with confidence.

• Focus on learning from each mistake and improving over time.
• Stay persistent and avoid getting discouraged when faced with setbacks.

By adopting a methodical approach to identifying, understanding, and correcting mistakes, you can transform errors into valuable learning experiences that help improve your skills and increase your chances of success.

Why Geometry Requires Conceptual Understanding

To truly excel in solving problems related to shapes, sizes, and their properties, it’s essential to grasp the underlying principles rather than just memorizing formulas and procedures. A deep, conceptual understanding enables individuals to think critically and approach problems with flexibility, which is crucial for solving complex tasks effectively. Without this foundational knowledge, applying the correct methods becomes much harder, often leading to mistakes or inefficient solutions.

Understanding the core concepts allows you to visualize problems, recognize patterns, and make logical connections between different ideas. It’s not just about knowing the steps to take; it’s about understanding why those steps work. This kind of insight helps in tackling a wide variety of problems, as it equips you with the tools to approach each one with a fresh perspective, rather than relying on rote memorization.

For example, rather than simply knowing how to calculate the area of a triangle using a formula, a conceptual understanding helps you comprehend why this formula works and how it relates to the triangle’s dimensions. This deeper understanding not only enhances problem-solving ability but also fosters critical thinking, which is beneficial beyond just solving academic tasks.

In essence, mastering the concepts behind problems empowers you to think independently, apply knowledge across different contexts, and improve your problem-solving skills in more dynamic and efficient ways.

Final Review Resources and Tools

Preparing for a comprehensive assessment requires not only a solid understanding of key concepts but also access to the right tools and resources. These resources can provide practice, clarification, and efficient methods to enhance your study sessions. With the right approach, you can effectively review and reinforce the material, boosting both your confidence and performance on the test.

One of the most valuable resources is practice problems. They help reinforce concepts, familiarize you with the types of questions you may encounter, and allow you to track your progress. In addition to traditional textbooks, online platforms often offer interactive quizzes, video tutorials, and step-by-step solutions, making studying both engaging and effective.

Online Resources

Websites such as educational platforms and academic forums provide a wealth of materials, from practice questions to detailed explanations. These resources are often organized by topic, allowing you to target specific areas of weakness. Many platforms also offer forums where you can ask questions and discuss problems with peers and tutors.

Study Apps and Tools

In addition to websites, there are numerous apps designed to support studying. Tools like flashcard apps, equation solvers, and note-taking platforms allow for quick reviews and efficient organization of study materials. Many of these tools also include timers to help you improve your time management skills and simulate the pace of the actual test.

Using a combination of these resources can ensure that you are well-prepared, providing both practice and conceptual understanding in the days leading up to your assessment.

What to Expect on Assessment Day

The day of your comprehensive assessment is an important milestone in your academic journey. It can be both exciting and nerve-wracking, but with proper preparation, you can approach it with confidence. On the day of the test, you will need to be ready for various aspects that go beyond simply answering questions, from managing your time effectively to staying calm under pressure.

Here’s a brief overview of what you can expect and how to prepare:

• Arrival and Setup: Arrive early to ensure you have time to settle in and gather your materials. You will typically need a few essential items, such as pencils, an eraser, a calculator, and a ruler, depending on the guidelines provided by your instructor.
• Test Environment: The atmosphere in the room will be focused and quiet. Everyone will be working on their individual assessments, so it’s important to stay calm and avoid distractions. There may also be a strict no-talking policy during the test to maintain focus.
• Test Structure: The assessment will likely include a mix of different types of questions, such as multiple-choice, short answer, and problem-solving questions. Some problems may require you to show all your work, so it’s important to pay attention to the instructions carefully.
• Time Management: You will be given a set amount of time to complete the test. Make sure to pace yourself by quickly moving through easier questions and saving time for more challenging ones. Keep an eye on the clock to avoid spending too long on any one problem.
• Stay Focused: It’s normal to feel anxious, but maintaining focus will help you stay on track. If you encounter a difficult question, skip it and come back later if necessary. This will help you avoid wasting time and ensure you address every section of the test.

By knowing what to expect and preparing thoroughly, you can handle the day with confidence and maximize your performance. Stay calm, stay focused, and trust in the effort you’ve put into your studies.