Geometry Unit 2 Exam Answers and Solutions

Success in this section of mathematics relies on your ability to understand and apply essential principles. By grasping core theories, formulas, and problem-solving methods, you can approach each challenge with confidence. Building a strong foundation in these topics will not only prepare you for the upcoming challenges but also improve your problem-solving skills in general.

Preparation is key when it comes to tackling questions that require both critical thinking and technical knowledge. By practicing with similar exercises and reviewing relevant material, you can hone your skills and avoid common pitfalls. Understanding the underlying logic behind various problems will help you recognize patterns and make quicker, more accurate decisions during your study sessions.

Focus on clarity and precision while practicing. Each question will test your ability to analyze and apply concepts systematically. With proper preparation, the path to mastering these topics becomes clearer, and the fear of difficult tasks can be eliminated.

Geometry Unit 2 Exam Answers

This section provides detailed solutions and explanations for common problems faced in this part of the curriculum. It covers a variety of techniques that are essential for understanding the key topics and achieving success in assessments. By reviewing these examples, you can deepen your comprehension and apply the concepts effectively in different scenarios.

Below are some examples of problems you might encounter and their step-by-step solutions:

Problem	Solution
Find the area of a triangle with base 10 and height 5.	Area = 1/2 × base × height = 1/2 × 10 × 5 = 25
Calculate the perimeter of a rectangle with length 8 and width 4.	Perimeter = 2 × (length + width) = 2 × (8 + 4) = 24
Determine the volume of a cylinder with radius 3 and height 7.	Volume = π × radius² × height = 3.14 × 3² × 7 = 197.82
Solve for x in the equation 2x + 5 = 15.	x = (15 – 5) / 2 = 10 / 2 = 5

By working through these types of problems, you can build confidence and ensure that you are prepared to apply these techniques in future tasks. Understanding the logic behind each solution will help you approach unfamiliar problems with a clearer perspective.

Key Concepts to Master for the Exam

To excel in this section, it is crucial to focus on the foundational principles that form the basis of the problems you will face. Mastering these essential topics will allow you to approach each challenge with a structured mindset, increasing your ability to apply the appropriate strategies efficiently. A solid understanding of key concepts is the first step toward achieving success.

Critical Theorems and Formulas

One of the most important areas to focus on is mastering the theorems and formulas that are regularly applied. Knowing these by heart will save you time and effort during problem-solving. Be sure to practice using them in various contexts, as their correct application is essential for deriving accurate solutions.

Problem-Solving Techniques

Being able to break down a complex problem into manageable steps is a valuable skill. Work on developing a systematic approach to solving various types of questions. Whether it’s using algebraic methods or geometric visualization, the ability to think critically and organize your approach is key to finding the right solution.

Step-by-Step Solutions for Common Problems

Breaking down complex problems into simpler steps is a powerful method for understanding and solving them effectively. By following a structured approach, you can tackle various challenges more efficiently. Below are some common scenarios with detailed steps to guide you through the solution process.

Solving for Unknown Variables

Many problems require you to find an unknown variable. Follow these steps to approach such questions:

1. Identify the given information in the problem.
2. Set up an equation or system based on the relationships between the variables.
3. Use algebraic operations to isolate the unknown.
4. Check the solution by substituting the value back into the original equation.

Working with Figures and Shapes

When dealing with geometric shapes or figures, it is essential to approach the problem methodically:

• Identify the type of figure or shape and any relevant properties.
• Determine the necessary measurements (e.g., sides, angles, area, perimeter).
• Apply the appropriate formulas to calculate the required values.
• Double-check your calculations for accuracy.

By following these structured methods, you can ensure that you are taking the right steps toward solving the problem, minimizing the chances of making mistakes. Regular practice with these solutions will help reinforce your understanding and improve your problem-solving skills over time.

Important Theorems to Remember

To solve a wide range of problems efficiently, it is crucial to be familiar with key theorems that provide the foundation for logical reasoning and calculations. These principles help simplify complex tasks and provide a systematic way to approach challenges. Understanding and applying these theorems is essential for success.

Pythagorean Theorem: This fundamental theorem states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. It is written as c² = a² + b².

Sum of Angles in a Triangle: The interior angles of any triangle always add up to 180°. This theorem is vital for solving problems involving angles and can be applied to various types of triangles.

Parallel Line Theorem: When a transversal intersects two parallel lines, alternate interior angles are congruent. This principle is crucial for proving various angle relationships in geometric figures.

Area of a Circle: The area of a circle can be found using the formula A = πr², where r is the radius. This formula is essential for calculating areas in problems involving circular shapes.

Mastering these theorems and knowing when and how to apply them is key to solving many mathematical problems effectively. They provide the necessary tools for understanding relationships between different elements in various shapes and figures.

Practice Questions for Geometry Unit 2

Practicing a variety of problems is one of the best ways to reinforce your understanding of the core principles. By working through different scenarios, you can sharpen your skills and become more confident in solving problems under time constraints. Below are some sample questions designed to test your knowledge and prepare you for upcoming challenges.

Algebraic and Numerical Problems

These questions focus on applying mathematical concepts to solve for unknown values and work with equations:

• Find the length of the hypotenuse of a right triangle with legs measuring 6 and 8 units.
• Calculate the perimeter of a rectangle with a length of 12 units and a width of 5 units.
• Solve for x: 3x + 7 = 22.

Spatial and Visual Problems

These problems involve working with geometric shapes and understanding their properties:

• Determine the area of a triangle with a base of 10 units and a height of 4 units.
• Find the volume of a cylinder with a radius of 5 units and a height of 10 units.
• What is the sum of the interior angles of a hexagon?

By practicing these types of problems, you will become more comfortable with using the relevant formulas and applying the correct methods to find solutions. Regular practice will also help you recognize patterns and improve your problem-solving efficiency.

Common Mistakes to Avoid in Geometry

When solving problems in this area of mathematics, it’s easy to make small mistakes that can lead to incorrect solutions. Being aware of these common errors can help you avoid them and approach problems with greater accuracy. Below are some of the most frequent mistakes students make and tips on how to avoid them.

Misunderstanding Concepts

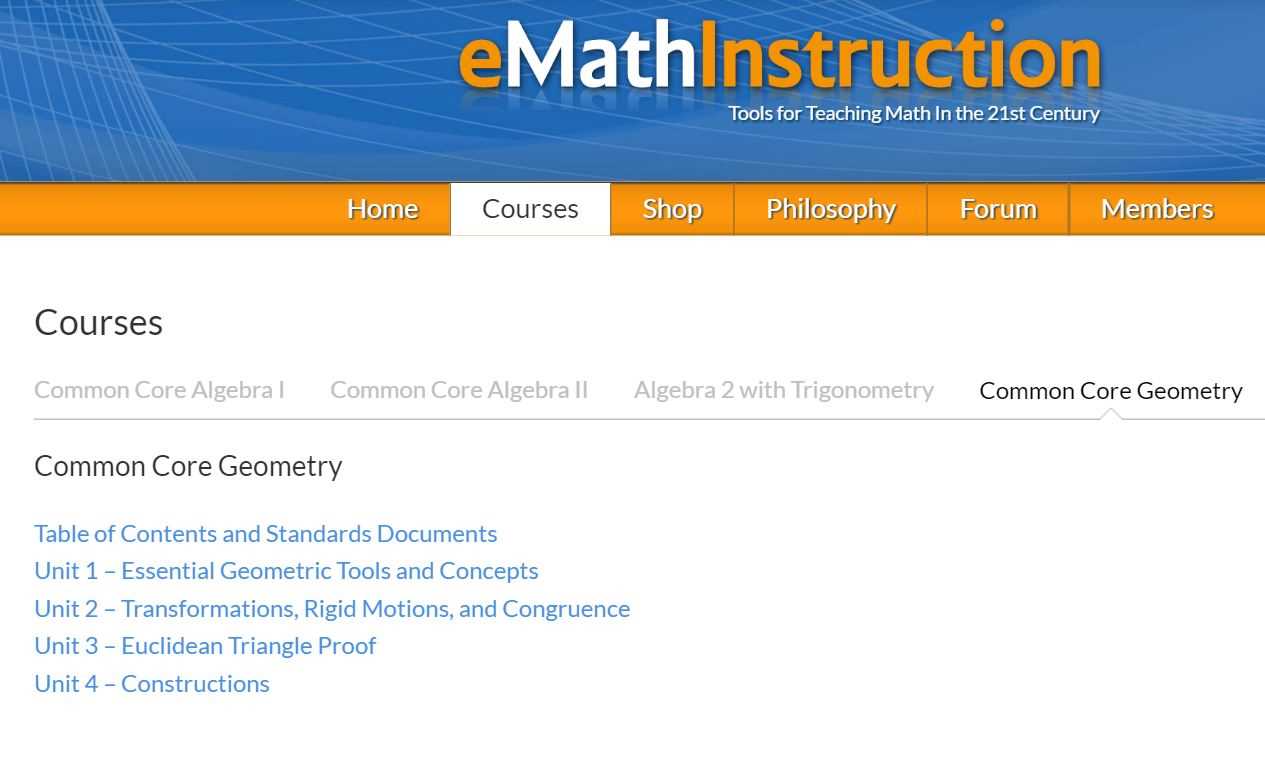
A common issue arises when basic concepts are misunderstood or overlooked. Here are some typical mistakes:

• Confusing the properties of different shapes, such as the difference between squares and rectangles.
• Forgetting key properties of parallel lines, such as equal angles formed by a transversal.
• Misinterpreting the relationship between angles in polygons or triangles.

Calculation Errors

Mathematical calculations are another area where mistakes often occur. Be mindful of these errors:

• Incorrectly applying formulas, such as using the wrong formula for area or volume.
• Forgetting to square numbers or apply the proper order of operations when solving equations.
• Making arithmetic errors when adding or multiplying numbers.

By reviewing the material thoroughly and double-checking your calculations, you can reduce the likelihood of these mistakes. Regular practice and a careful, methodical approach will help you avoid these common pitfalls and improve your problem-solving abilities.

How to Approach Word Problems

Word problems can often feel overwhelming, but with a structured approach, you can break them down into manageable steps. The key to solving these types of problems is to carefully analyze the information provided and determine the correct method for finding the solution. Below are some strategies to help you tackle word problems effectively.

Step-by-Step Strategy

Follow these steps to approach word problems with clarity:

1. Read the Problem Carefully: Take your time to read through the entire problem, making sure you understand what is being asked and what information is provided.
2. Identify Key Information: Highlight or underline important data such as numbers, relationships between shapes, and conditions that will guide your solution.
3. Translate Words into Math: Convert the verbal descriptions into mathematical equations or expressions. This may involve identifying variables, applying formulas, or setting up relationships between quantities.
4. Perform the Calculations: Once you have a clear equation or method, proceed with the necessary calculations. Ensure that you follow the correct order of operations.
5. Double-Check Your Work: Review your steps and ensure the solution makes sense in the context of the problem. Verify your answer by substituting values back into the equation if applicable.

Common Pitfalls to Avoid

While approaching word problems, be mindful of these common mistakes:

• Skipping over important details or misinterpreting the question.
• Forgetting to apply the correct formula or method for the specific type of problem.
• Making errors in arithmetic or failing to check your work after solving.

By following these steps and being aware of potential errors, you will develop the confidence and skills needed to solve word problems effectively and accurately.

Tips for Solving Geometry Proofs

Solving proofs requires a logical approach and a deep understanding of relationships between various elements. It can seem challenging at first, but with the right strategies, you can develop a systematic method for constructing solid arguments and demonstrating your reasoning. Below are some essential tips to help guide you through the process of solving proofs effectively.

1. Understand the Given Information: Carefully read the problem to identify the information provided. Make sure you know what is given and what you are trying to prove. This will help you focus on the key elements that will guide your proof.

2. Break Down the Problem: Before jumping into the solution, take time to visualize the situation. Draw diagrams where necessary and label all known elements. This will give you a clearer picture of the relationships and help you organize your thoughts.

3. Use Logical Steps: Proofs are built on logical steps. Start from the given facts and use deductive reasoning to move towards the conclusion. Each step should follow naturally from the previous one, using established properties, theorems, or definitions.

4. Justify Your Reasoning: In a proof, it’s important to explain why each step is valid. This means citing relevant theorems, definitions, or properties to support your arguments. Whether you’re using the Pythagorean theorem, angle relationships, or congruence rules, make sure to reference the rules you’re applying.

5. Work Backwards (When Needed): If you’re struggling to get started, try working backwards. Begin with the conclusion you’re trying to prove and see if you can identify a path that leads from it to the given information. This approach can sometimes reveal the steps that are most helpful to use.

6. Stay Organized: Organize your proof clearly, with each step numbered and supported by the appropriate reasoning. Keep your work neat to avoid confusion and to ensure that the logic of your argument is easy to follow.

By following these tips and practicing regularly, you’ll become more comfortable with constructing proofs and develop a better understanding of how to approach logical problems in a structured way. Patience and attention to detail are key to mastering the art of proving geometric relationships.

Understanding Coordinate Geometry Techniques

Coordinate-based methods are essential tools for solving problems that involve the positions and relationships of points in space. These techniques allow you to represent geometric shapes and analyze their properties using a numerical system. Whether you’re working with straight lines, curves, or complex figures, mastering these strategies can significantly simplify the process of finding solutions.

Plotting and Analyzing Points

The first step in coordinate-based problems is often plotting points on a plane. Understanding how to place points accurately is crucial for solving problems involving distances, midpoints, and slopes. Here are some common approaches:

• Identifying coordinates: Each point is defined by a pair of numbers, often referred to as x and y, which represent its position relative to the horizontal (x-axis) and vertical (y-axis) lines.
• Distance formula: This technique allows you to calculate the distance between two points. The formula is √[(x₂ – x₁)² + (y₂ – y₁)²].
• Midpoint formula: To find the midpoint between two points, use the formula ((x₁ + x₂)/2, (y₁ + y₂)/2).

Working with Lines and Slopes

Understanding the relationship between points on a line is essential for many types of problems. The slope of a line, which measures its steepness, can be determined using the formula:

• Slope formula: m = (y₂ – y₁) / (x₂ – x₁), where m represents the slope and (x₁, y₁) and (x₂, y₂) are the coordinates of two points on the line.
• Equation of a line: Once you know the slope, you can write the equation of a line using the point-slope form: y – y₁ = m(x – x₁).

These techniques form the foundation of solving a wide range of problems that require an understanding of spatial relationships between points and lines. By mastering these methods, you can approach complex geometric challenges with confidence and precision.

Essential Formulas for Geometry Unit 2

To solve many types of problems, it’s important to remember key formulas that govern the relationships between different shapes and measurements. These formulas allow you to quickly compute areas, perimeters, volumes, and other important properties. Below, we cover some of the most essential equations to keep in mind as you prepare for your studies and assessments.

Key Area and Perimeter Formulas

These formulas are fundamental for calculating the dimensions of various shapes. Whether dealing with polygons, circles, or other figures, they serve as the foundation for many problems.

Shape	Area Formula	Perimeter Formula
Rectangle	Area = length × width	Perimeter = 2(length + width)
Triangle	Area = 1/2 × base × height	Perimeter = side₁ + side₂ + side₃
Circle	Area = π × radius²	Perimeter (Circumference) = 2 × π × radius

Volume and Surface Area Formulas

For three-dimensional shapes, volume and surface area are key measurements. These formulas are essential when working with solids such as cubes, spheres, and cylinders.

Shape	Volume Formula	Surface Area Formula
Cube	Volume = side³	Surface Area = 6 × side²
Cylinder	Volume = π × radius² × height	Surface Area = 2π × radius × (radius + height)
Sphere	Volume = 4/3 × π × radius³	Surface Area = 4π × radius²

By memorizing these formulas and understanding how to apply them, you’ll be better equipped to tackle a wide range of problems that involve measurements and properties of various figures.

Time Management Strategies for the Exam

Effective time management is crucial when preparing for any assessment. Developing a clear plan and understanding how to allocate your time during the test can greatly impact your performance. By organizing your approach and managing your pace, you can ensure that each section of the test receives the attention it deserves, allowing you to work through problems efficiently and confidently.

Preparation Before the Test

Time management begins well before the day of the assessment. Proper preparation allows you to approach the test with a sense of readiness and confidence. Consider the following tips:

• Create a study schedule: Break down your study material into manageable chunks and set aside time for review. Make sure to focus on your weaker areas and leave time for practice tests.
• Prioritize important topics: Focus on the concepts most likely to appear on the test or those that have historically been emphasized. Ensure that you are familiar with key formulas and methods.
• Avoid last-minute cramming: Spacing out your study sessions helps with retention. Trying to cram the night before often leads to unnecessary stress and a lack of focus.

During the Test

Once you’re sitting for the test, managing your time becomes essential to completing all questions thoroughly. Here’s how to optimize your performance:

• Read instructions carefully: Take a moment at the start to fully understand the instructions. Rushing through them can lead to mistakes that waste valuable time.
• Allocate time per section: Divide the total time by the number of sections or questions. This gives you a rough idea of how long you should spend on each part, helping you stay on track.
• Don’t linger too long on one question: If you find yourself stuck, move on to the next question and return to difficult ones later. This will prevent wasting time on one problem while missing others.

By applying these strategies, you can manage your time effectively during both preparation and the actual assessment, ensuring you maximize your performance and reduce stress.

Visualizing Geometric Figures Effectively

Being able to visualize shapes and their properties is a key skill in solving many problems. A clear mental image of figures and their relationships can greatly enhance understanding and problem-solving ability. Whether it’s a complex polygon, a circle, or a three-dimensional shape, being able to mentally manipulate these objects makes it easier to apply the right formulas and reasoning to find solutions.

To visualize figures effectively, it’s important to break down each shape into simpler parts and recognize their key attributes. Drawing diagrams is an essential tool in this process. Even a rough sketch can clarify the relationships between angles, sides, and other elements. By practicing with various figures and experimenting with different perspectives, you can improve your ability to interpret and solve geometric problems with greater confidence.

Additionally, using color coding or labeling different parts of a figure can help highlight important aspects such as angles, side lengths, and relationships between components. This simple technique can turn a confusing diagram into a much clearer visual aid, aiding both understanding and accuracy during problem solving.

Reviewing Key Geometric Definitions

Understanding the fundamental terms and concepts is crucial to solving problems involving shapes and spatial reasoning. Each concept forms the foundation for more complex problem-solving techniques, and mastering these definitions allows for quicker identification of patterns and solutions. Whether it’s the properties of angles, lines, or specific shapes, a solid grasp of the terminology helps to build a clear mental framework for approaching questions.

When reviewing, focus on the key elements that define different figures, as well as the relationships between them. For instance, knowing the difference between parallel and perpendicular lines or understanding how different types of angles interact can significantly aid in your problem-solving. Pay attention to definitions that apply to both two-dimensional and three-dimensional shapes, as this knowledge is often interconnected.

By regularly reviewing and practicing these essential terms, you will enhance your ability to quickly identify and apply the correct principles when tackling problems.

Using Graphs to Solve Geometry Problems

Graphs are powerful tools for solving spatial problems and visualizing relationships between different elements. By plotting points, lines, and curves on a coordinate plane, you can translate complex geometric concepts into more manageable visual representations. This method helps identify key properties, such as distance, slopes, and intersections, which are often crucial in finding solutions.

Plotting Key Points and Lines

One of the first steps in solving a problem using graphs is to accurately plot the given points and lines. Each point represents a specific location on the plane, and by connecting points or identifying line equations, you can gain valuable insights into the spatial relationships between them. For example, plotting a set of points can help you recognize geometric shapes such as triangles, rectangles, or circles, which you can then analyze further.

Analyzing Relationships and Distances

Graphs also allow you to easily calculate distances and slopes between points, which is essential for many types of problems. Using the distance formula or slope formula on a graph can give you the exact measurements needed to solve for unknowns in problems involving parallel lines, perpendicularity, or area. Visualizing the problem on a graph often simplifies the process of finding solutions and understanding the connections between different components.

By regularly using graphs in problem-solving, you can build a deeper understanding of spatial concepts and improve your ability to work through complex problems efficiently.

How to Check Your Work Efficiently

Verifying the accuracy of your solutions is an essential part of the problem-solving process. Efficient checking involves reviewing each step systematically to ensure that all calculations, logical steps, and interpretations are correct. Rather than redoing the entire problem, focus on key areas where errors are most likely to occur, such as arithmetic operations or assumptions about the relationships between elements.

One useful approach is to retrace your steps by starting from the final answer and working backward to the initial conditions. This allows you to spot any inconsistencies or mistakes that may have been made along the way. Another strategy is to check for logical consistency: confirm that the relationships between variables make sense and that the result aligns with known properties or expected outcomes.

Additionally, it’s helpful to use alternative methods or formulas to verify your results. If the answer can be obtained in more than one way, compare the outcomes to ensure they match. This dual verification adds an extra layer of confidence in the correctness of your solution.

Efficient checking not only saves time but also helps to prevent small mistakes from undermining the overall accuracy of your work. By practicing these techniques, you can improve both the speed and reliability of your problem-solving process.

Preparing for Multiple-Choice Questions

When tackling multiple-choice questions, it’s essential to approach them with a strategic mindset. These questions often present several plausible options, making it crucial to focus on eliminating incorrect answers and identifying the most accurate choice. The key to success lies in preparation, understanding core concepts, and practicing problem-solving techniques.

To effectively prepare, consider the following strategies:

• Review Key Concepts: Familiarize yourself with the main principles and definitions relevant to the topics. This will allow you to quickly identify the right approach for each question.
• Practice with Sample Questions: Work through practice problems to familiarize yourself with the types of questions that may be asked. This will help you identify patterns and common themes in the material.
• Understand the Structure: Multiple-choice questions often include distractors designed to test your understanding. Pay attention to subtle differences between options to avoid being misled.
• Eliminate Obvious Errors: If an answer choice seems obviously incorrect, eliminate it immediately to improve your chances of choosing the correct one from the remaining options.

Additionally, make sure to pace yourself during the assessment. Don’t get stuck on a difficult question–move on and come back to it later if necessary. Time management is key when answering multiple-choice questions efficiently.

With the right preparation and mindset, you can approach multiple-choice questions with confidence and improve your chances of selecting the correct answers quickly.

What to Do Before the Test

Preparation plays a significant role in achieving success when facing any assessment. A few focused steps taken in the days leading up to the test can make all the difference. It’s important to go beyond mere content review and incorporate strategies that enhance your performance under timed conditions. By organizing your review sessions, managing your time, and prioritizing key areas, you can enter the assessment feeling confident and prepared.

1. Review Key Concepts and Techniques

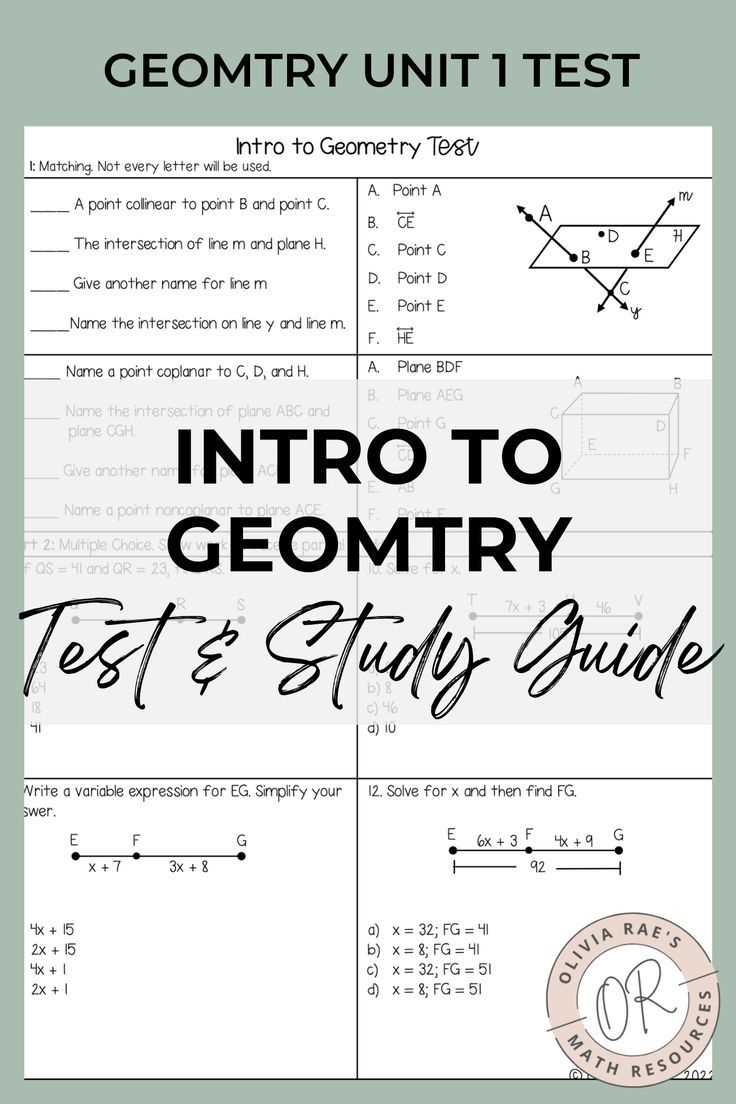
Revisiting important principles is essential for reinforcing your understanding. Focus on the most frequently tested ideas and techniques, and ensure you can apply them in different contexts. Break down complex ideas into simpler steps, and don’t hesitate to revisit challenging problems to ensure full comprehension.

2. Create a Study Plan

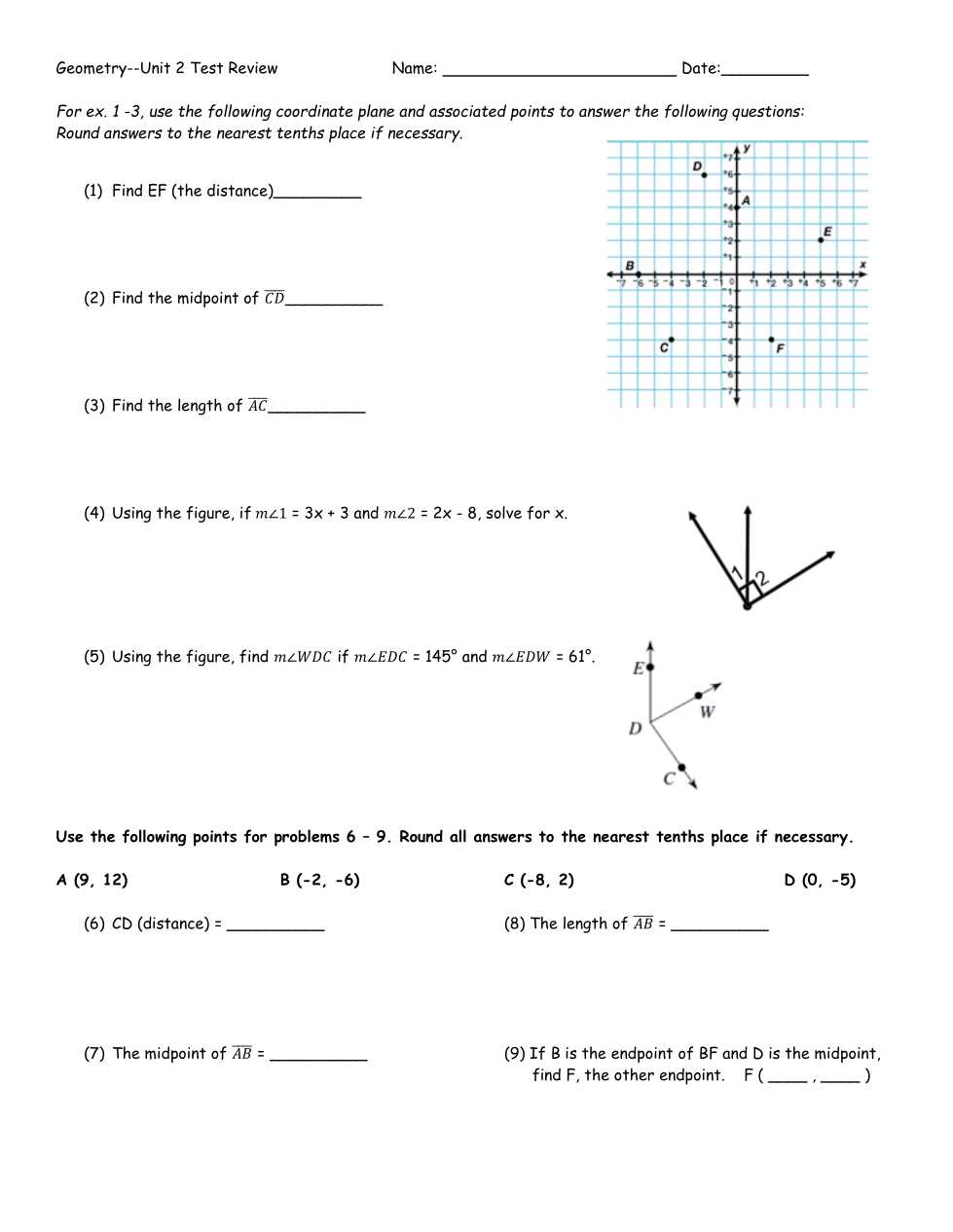
Design a realistic study schedule to ensure adequate time for each topic. Prioritize areas where you may need additional practice. Don’t overload yourself the night before the test; aim to study in manageable blocks to avoid burnout.

Take time to relax as well. Overloading your brain can be counterproductive. Instead, focus on getting a good night’s sleep before the test. This helps maintain mental clarity and focus during the assessment.

By following these simple but effective steps, you’ll be better prepared and more confident when it comes time to face the test.