Honors Geometry Final Exam Review Guide

Preparing for an advanced level of mathematical challenges requires a clear understanding of various principles and strategies. To excel, it is essential to strengthen your grasp of key theories, formulas, and problem-solving techniques. This section will guide you through critical concepts that form the foundation of your studies, ensuring you’re well-equipped to tackle complex questions with confidence.

Solidifying your understanding of geometric shapes, their properties, and their relationships is vital for success. Whether you’re working with angles, curves, or spatial figures, it’s important to approach each problem systematically. Additionally, practice with real-world applications of these principles will significantly enhance your ability to think critically and solve problems quickly.

By focusing on the core components and applying proven methods for tackling questions, you can improve both your speed and accuracy. With the right preparation, you’ll be ready to handle any challenge and approach your studies with greater ease.

Honors Geometry Final Exam Review

Preparing for a challenging mathematical assessment requires focusing on key concepts, problem-solving techniques, and strategies to approach complex questions. This section will help you build a strong foundation and ensure you’re ready to tackle any problem that comes your way.

Understanding Essential Concepts

In order to succeed, it’s critical to have a clear understanding of the core principles that will appear in the assessment. Focus on:

• Properties of different shapes
• Relationship between angles, lines, and figures
• Key formulas and their applications
• Methods for proving geometric theorems

Key Problem-Solving Strategies

Efficiency and accuracy are crucial when solving complex problems. Here are some strategies to keep in mind:

1. Break down the problem step by step
2. Use diagrams to visualize the relationships between elements
3. Apply appropriate theorems or formulas based on the problem’s context
4. Double-check your work to avoid simple errors

With thorough practice and a focused review of these concepts, you’ll feel more prepared and confident when tackling mathematical challenges.

Understanding Key Geometry Concepts

To excel in mathematics, it’s essential to grasp the foundational ideas that govern shapes, angles, and spatial relationships. Mastering these principles will not only enhance your problem-solving skills but also make complex tasks more manageable. This section focuses on the core concepts you need to understand and apply in a variety of situations.

Basic Properties of Shapes

Each geometric figure has its own set of rules and properties that define its characteristics. A solid understanding of these properties allows you to apply the right methods for solving problems. Here are some important aspects to consider:

Shape	Key Properties	Formula/Rule
Triangle	Sum of angles is 180°	Area = 1/2 × base × height
Circle	All points equidistant from center	Area = π × r², Circumference = 2π × r
Rectangle	Opposite sides are equal, angles are 90°	Area = length × width

Relationships Between Angles and Figures

Angles are critical in solving many problems, especially when combined with lines and shapes. Understanding how angles interact with parallel lines, transversals, and other geometric figures will help you solve complex problems more efficiently. Focus on:

• Complementary and supplementary angles
• Vertical and adjacent angles
• Angle pairs formed by parallel lines and transversals

These fundamental concepts are the building blocks for more advanced topics and will provide a solid foundation for success in future challenges.

Important Theorems to Memorize

Having a strong command of key mathematical principles and theorems is crucial for solving complex problems. These rules provide the foundation for many geometric calculations and proofs, allowing you to tackle a wide range of questions with confidence. This section highlights some of the most essential theorems that you should commit to memory.

Fundamental Theorems for Shapes and Angles

Understanding relationships between lines, angles, and figures is essential for solving many problems. Here are some fundamental theorems to remember:

• Pythagorean Theorem: In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides (a² + b² = c²).
• Angle Sum Theorem: The sum of the interior angles of a triangle is always 180°.
• Isosceles Triangle Theorem: In an isosceles triangle, the angles opposite the equal sides are congruent.

Key Theorems for Parallel Lines and Circles

Parallel lines and circular figures present unique challenges, but theorems related to them can simplify problem-solving. Focus on the following:

• Alternate Interior Angles Theorem: When a transversal crosses two parallel lines, each pair of alternate interior angles is congruent.
• Tangent-Secant Theorem: The square of the length of the tangent segment from a point to a circle is equal to the product of the lengths of the secant segment and its external segment.
• Circle Angle Theorem: The angle subtended by a chord at the center of a circle is twice the angle subtended at any point on the circumference.

By memorizing these essential theorems, you’ll be better equipped to solve a variety of problems and apply these principles in different contexts.

Mastering Geometric Proofs

Developing the ability to construct logical arguments and prove relationships between figures is an essential skill in advanced mathematics. Proofs allow you to justify claims based on established principles and theorems, transforming assumptions into verified truths. Mastering this skill will enhance your ability to reason through problems and demonstrate understanding.

Approaching Proofs with Logic

Successful proofs rely on a clear, step-by-step logical approach. Each statement made in a proof must be supported by a previously established fact or rule. Here’s an overview of common types of reasoning used in proofs:

Type of Reasoning	Description	Example
Direct Proof	Start with known facts and build up to the conclusion.	Prove that the sum of two even numbers is even.
Proof by Contradiction	Assume the opposite of what you want to prove, then show a contradiction.	Prove that √2 is irrational.
Proof by Induction	Prove a statement for a base case, then assume it’s true for an arbitrary case to prove it for the next case.	Prove the sum of the first n odd numbers is n².

Common Proof Strategies

Proofs often require combining various strategies to reach the desired conclusion. Here are some useful techniques to incorporate into your approach:

• Constructing auxiliary lines: Drawing extra lines or segments can simplify the relationships between figures.
• Using symmetry: Identifying symmetric properties of shapes can reduce the complexity of the problem.
• Applying known theorems: Always make use of established principles, like the Pythagorean theorem, when they are relevant.

By practicing different proof methods and recognizing when each strategy is most effective, you’ll build confidence and improve your ability to reason through complex geometric situations.

Solving Triangle Properties Efficiently

Triangles are fundamental shapes in mathematics, and understanding their properties is key to solving a wide range of problems. Efficiently applying the relationships between the sides and angles of triangles can save time and reduce errors in your calculations. This section focuses on methods to quickly and accurately solve for unknowns in various types of triangles.

Key Properties to Remember

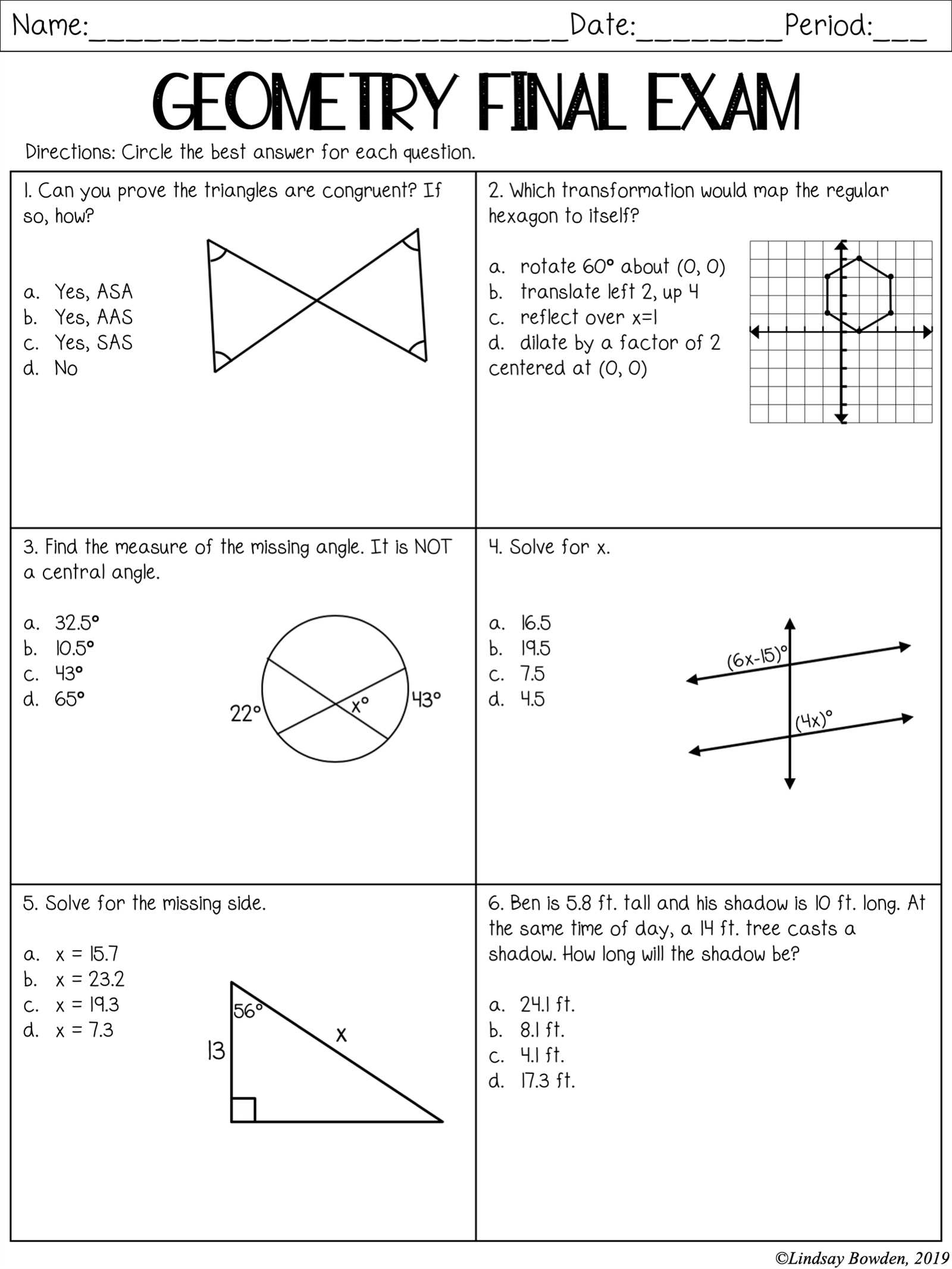
To tackle triangle-related problems effectively, make sure to remember these essential properties:

• Sum of Angles: The sum of the interior angles in any triangle is always 180°.
• Congruent Triangles: If two triangles are congruent, their corresponding sides and angles are equal.
• Right Triangle Hypotenuse: In a right triangle, the hypotenuse is opposite the right angle and is the longest side.
• Isosceles Triangle Angles: In an isosceles triangle, the two base angles are congruent.

Efficient Techniques for Solving Problems

Use these methods to solve triangle problems more effectively:

• Apply the Pythagorean Theorem: For right triangles, use the relationship a² + b² = c² to find the missing side when two sides are known.
• Use Trigonometric Ratios: When dealing with right triangles, sine, cosine, and tangent ratios can help solve for unknown angles or sides.
• Use the Law of Sines and Cosines: For non-right triangles, these laws allow you to find unknown sides or angles based on known information.

By understanding these properties and applying efficient techniques, you’ll be able to solve triangle-related problems more quickly and accurately, enhancing your ability to tackle complex geometric tasks.

Analyzing Circles and Their Equations

Understanding the properties of circular shapes and their mathematical descriptions is essential for solving many problems in spatial reasoning. The equation of a circle provides a powerful tool for analyzing its position, size, and other key features in a coordinate plane. This section will guide you through the fundamental aspects of circles and how to work with their equations efficiently.

Standard Equation of a Circle

The standard equation for a circle in a coordinate plane is derived from the general relationship between the points on the circle and its center. The equation is:

Equation	Meaning
(x – h)² + (y – k)² = r²	Where (h, k) is the center of the circle and r is its radius.

By understanding this equation, you can quickly identify key elements such as the center and radius of any circle, which are critical when solving related problems.

Transforming and Manipulating Circle Equations

Sometimes, you may need to rewrite or transform the equation of a circle into a different form to better understand its properties or solve specific problems. Key techniques include:

• Completing the Square: Used to convert a general equation into the standard form.
• Radius and Center Identification: Once in standard form, easily identify the circle’s radius and center by comparing with the standard equation.
• Finding Intersections: When working with other geometric figures, you may need to solve systems of equations to find where the circle intersects other shapes.

By mastering these strategies, you’ll be well-equipped to analyze and manipulate circle equations, allowing you to solve a wide variety of problems more effectively.

Coordinate Geometry and Its Applications

The study of points, lines, and shapes within a coordinate plane enables a more analytical approach to solving spatial problems. By translating geometric concepts into algebraic equations, we can apply powerful tools to solve for distances, slopes, midpoints, and other key characteristics. This section explores how to use coordinate-based methods to tackle a variety of mathematical challenges.

Key Concepts in Coordinate Systems

To work effectively with coordinate systems, it’s important to understand the following concepts:

• Distance Formula: Used to calculate the distance between two points in the plane: d = √[(x₂ - x₁)² + (y₂ - y₁)²]
• Slope Formula: Helps determine the steepness of a line: m = (y₂ - y₁) / (x₂ - x₁)
• Midpoint Formula: Finds the center point between two points: Midpoint = ((x₁ + x₂)/2, (y₁ + y₂)/2)

Applications of Coordinate Geometry

Coordinate geometry is widely applicable in various fields of study and real-world situations. Some common applications include:

• Line Equations: Determine the equation of a line given two points or a point and a slope. This is useful for analyzing relationships between variables and graphing linear functions.
• Circle Equations: Using the standard equation of a circle to find the center and radius, or to solve problems involving circles in coordinate planes.
• Intersection Points: Solving systems of equations to find where two lines or curves intersect, which is critical in both geometry and physics.

By mastering coordinate-based techniques, you’ll be equipped to handle a wide variety of geometric problems, from calculating lengths and angles to determining the positions and relationships of different shapes in the coordinate plane.

Working with Angles and Parallel Lines

Understanding the relationships between angles and parallel lines is crucial for solving various geometric problems. These relationships are governed by specific rules and properties that help determine the measures of unknown angles, identify parallelism, and analyze the interaction of different lines in the plane. Mastering these concepts allows you to solve a wide range of problems efficiently.

Properties of Parallel Lines

Parallel lines, by definition, never intersect and remain equidistant from each other. When a transversal intersects parallel lines, several angle relationships come into play. Some key properties to remember are:

• Corresponding Angles: Angles that are in the same position relative to the parallel lines and the transversal are equal.
• Alternate Interior Angles: Angles that lie on opposite sides of the transversal and inside the parallel lines are congruent.
• Alternate Exterior Angles: Angles on opposite sides of the transversal and outside the parallel lines are also congruent.
• Consecutive Interior Angles: Angles on the same side of the transversal and inside the parallel lines are supplementary (add up to 180°).

Solving Problems Involving Angles and Parallel Lines

By applying these properties, you can solve problems involving parallel lines and angle measures more effectively. Here are some common techniques:

• Using Angle Relationships: When you encounter a transversal intersecting parallel lines, identify angle pairs (corresponding, alternate interior, etc.) to set up equations and solve for unknown angles.
• Finding Missing Angles: In many problems, you will need to find missing angles by recognizing supplementary or complementary angle relationships, especially when working with parallel lines.
• Proving Lines Are Parallel: If certain angle conditions are met (e.g., corresponding angles are equal or alternate interior angles are congruent), you can prove that two lines are parallel.

By mastering these angle relationships and properties of parallel lines, you’ll be able to approach complex geometric problems with confidence and precision.

Strategies for Solving Word Problems

Word problems can often seem intimidating, but with the right approach, they become more manageable. The key to solving these types of questions is to break down the information provided, translate it into mathematical terms, and apply the appropriate concepts. By following a systematic process, you can solve complex scenarios step by step.

Understanding the Problem

Before jumping into calculations, take time to fully understand what the problem is asking. Here are some steps to help you clarify the problem:

• Read Carefully: Make sure you read the problem at least twice to ensure that all the important details are clear.
• Identify What Is Given: Highlight or underline the information that is provided. This includes numbers, shapes, or relationships between elements.
• Determine What Is Being Asked: Clearly identify the final question or goal. What exactly are you solving for?

Translating Words Into Equations

Once you have a solid understanding of the problem, the next step is to translate the words into a mathematical equation or expression. Here’s how you can do this effectively:

• Define Variables: Assign variables to unknowns. For example, let x represent the unknown length or value.
• Use Known Relationships: Identify any formulas or geometric properties that relate to the problem, such as area formulas or the Pythagorean theorem.
• Set Up Equations: Write equations based on the relationships described in the problem. Use the given information to form a solvable equation.

By following these strategies, you can transform word problems into solvable equations. This structured approach helps simplify the problem-solving process and ensures you don’t miss any important steps along the way.

Using Pythagorean Theorem Effectively

The Pythagorean theorem is a powerful tool for solving problems involving right triangles. It establishes a relationship between the lengths of the three sides of a right triangle, allowing you to calculate an unknown side when the other two are known. By understanding how to apply this theorem, you can solve various real-world and mathematical problems with ease.

Key Concepts and Formula

The Pythagorean theorem states that in any right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. The formula is:

• a² + b² = c²

Where:

• a and b are the lengths of the legs of the triangle, and
• c is the length of the hypotenuse (the side opposite the right angle).

Practical Applications

The Pythagorean theorem is not just a theoretical concept; it has numerous applications in various fields. Here’s how you can use it effectively:

• Finding an Unknown Side: If you know the lengths of two sides of a right triangle, you can easily calculate the third side by rearranging the formula.
• Checking for a Right Triangle: You can use the theorem to verify if a given set of three lengths forms a right triangle. If a² + b² = c² holds true, the triangle is a right triangle.
• Real-World Problems: In construction, navigation, and even art, the Pythagorean theorem is used to calculate distances, angles, and other geometric properties. For example, determining the shortest path between two points or ensuring the corners of a building are square involves applying this concept.

By mastering this fundamental theorem, you can approach a wide variety of problems confidently and accurately, whether in academic exercises or practical situations.

Reviewing Area and Perimeter Formulas

Understanding the formulas for calculating area and perimeter is crucial for solving many problems involving shapes. These fundamental concepts allow you to find the size of a space or the length around a boundary, both of which are common tasks in various mathematical and real-world situations. Mastery of these formulas helps streamline problem-solving and increases confidence when dealing with complex shapes.

Basic Formulas for Common Shapes

Here are some of the most essential formulas for finding the area and perimeter of common shapes:

• Rectangle:
• Area = length × width
• Perimeter = 2 × (length + width)
• Square:
• Area = side²
• Perimeter = 4 × side
• Triangle:
• Area = 1/2 × base × height
• Perimeter = sum of all three sides
• Circle:
• Area = π × radius²
• Perimeter (Circumference) = 2 × π × radius

Applying the Formulas

Once you are familiar with the basic formulas, applying them becomes much easier. Here’s how you can use these formulas effectively:

• Identify the Shape: Determine the type of shape you are working with so you can choose the correct formula.
• Substitute the Known Values: Plug in the given dimensions into the formula. If necessary, convert units to ensure consistency (e.g., converting feet to inches).
• Check for Additional Information: Some problems may require extra steps, such as calculating the diagonal of a rectangle or finding the height of a triangle using trigonometry. Always look for clues in the problem statement.

By regularly practicing these formulas, you’ll develop a strong understanding of how to calculate area and perimeter efficiently. These skills are not only vital for academic purposes but also for everyday applications such as planning spaces, construction, and even interior design.

Volume and Surface Area Problems

When working with three-dimensional shapes, calculating volume and surface area are essential skills. Volume helps measure the amount of space a solid occupies, while surface area gives the total area covering the surface of the object. Mastery of these concepts is crucial for solving a variety of practical and theoretical problems in both mathematics and real-life situations.

Key Formulas for Common Solids

Below are the formulas you need to calculate the volume and surface area for some of the most common three-dimensional shapes:

• Cube:
• Volume = side³
• Surface Area = 6 × side²
• Rectangular Prism:
• Volume = length × width × height
• Surface Area = 2 × (length × width + width × height + height × length)
• Cylinder:
• Volume = π × radius² × height
• Surface Area = 2 × π × radius × (radius + height)
• Sphere:
• Volume = 4/3 × π × radius³
• Surface Area = 4 × π × radius²
• Volume = 1/3 × π × radius² × height
• Surface Area = π × radius × (radius + slant height)

Approaching Volume and Surface Area Problems

Solving problems involving volume and surface area requires a step-by-step approach to ensure accurate calculations:

• Understand the Shape: Identify the three-dimensional object you’re working with and determine which formula applies.
• Substitute Known Values: Insert the given measurements into the appropriate formula. Pay attention to units and convert them if necessary to maintain consistency.
• Use Accurate Measurements: Ensure all measurements, such as radius, height, and side lengths, are correctly identified and used in the formulas.
• Check Your Work: Double-check the values you’ve used and the steps taken to confirm accuracy, especially when working with complex shapes or non-standard dimensions.

Whether you’re calculating the volume of a swimming pool, determining the surface area of a gift box, or tackling more complex theoretical problems, understanding how to apply these formulas efficiently will significantly improve your problem-solving ability. Practice with a variety of shapes to strengthen your skills and gain confidence in handling volume and surface area calculations.

Transformations and Symmetry in Geometry

In the study of shapes and figures, transformations and symmetry play a vital role in understanding their properties and relationships. These concepts help describe how objects can be moved, rotated, reflected, or resized while maintaining certain characteristics. Mastering transformations and symmetry allows for a deeper understanding of the structure and behavior of figures in space.

Types of Transformations

Transformations describe how a figure can change its position or orientation on a plane. The main types include:

• Translation: A slide where every point of a figure moves the same distance and in the same direction.
• Rotation: Turning a figure around a fixed point by a certain angle.
• Reflection: A flip where a figure is mirrored over a line, creating a mirror image.
• Dilation: A resizing transformation that either enlarges or reduces a figure while maintaining its shape.

Understanding Symmetry

Symmetry refers to the balance and proportionality found in shapes and figures. There are two primary types of symmetry that are crucial for analyzing figures:

• Reflectional Symmetry: A figure has reflectional symmetry if it can be reflected over a line, creating two identical halves.
• Rotational Symmetry: A figure exhibits rotational symmetry if it can be rotated around a point and still look the same after a certain angle.

These concepts are essential for solving many problems, from simple shape analysis to more advanced applications involving spatial relationships. By understanding how figures can be transformed and the types of symmetries they possess, you can gain insight into the structure and properties of complex geometric systems.

Understanding Congruence and Similarity

In the study of shapes, two key concepts that frequently arise are congruence and similarity. These terms help us compare figures and understand how their sizes, shapes, and angles relate to each other. While both concepts involve comparing figures, they describe different relationships between them. By mastering these ideas, you can better analyze how shapes can be transformed or scaled without losing their essential characteristics.

Congruence: Identical in Shape and Size

Congruent figures are exactly the same in both size and shape. When two figures are congruent, their corresponding sides and angles are equal. This means that one figure can be superimposed on the other without any distortion. To determine congruence, the following conditions must be met:

• Corresponding Sides: All corresponding sides of the two figures must be of equal length.
• Corresponding Angles: All corresponding angles of the two figures must be equal in measure.
• Rigid Transformations: Congruence can be proven through transformations such as translations, rotations, and reflections that do not change the size or shape of the figure.

Similarity: Same Shape, Different Size

Similar figures, on the other hand, have the same shape but may differ in size. While their corresponding angles are equal, their corresponding sides are proportional, meaning they have the same ratio. For two figures to be similar, the following conditions apply:

• Equal Angles: All corresponding angles between the figures must be congruent.
• Proportional Sides: The corresponding sides of the figures must be proportional, meaning the ratio of their lengths remains constant.
• Dilations: Similarity is often achieved through a dilation, which scales a figure up or down while preserving its shape.

Understanding the distinction between congruence and similarity is essential for solving many types of problems involving figures. By knowing when shapes are congruent or similar, you can use geometric properties to find unknown lengths, angles, and areas more efficiently.

Trigonometry Basics for Geometry

Trigonometry plays a crucial role in understanding the relationships between the sides and angles of triangles. This branch of mathematics helps us solve problems that involve right-angled triangles by using functions and ratios that connect angles to side lengths. Grasping these basic principles allows for the analysis of various geometric shapes and situations, providing solutions to complex problems with relative ease.

The Fundamental Trigonometric Ratios

In right triangles, there are three primary ratios that form the foundation of trigonometry:

• Sine (sin): The ratio of the opposite side to the hypotenuse.
• Cosine (cos): The ratio of the adjacent side to the hypotenuse.
• Tangent (tan): The ratio of the opposite side to the adjacent side.

These functions can be used to find unknown side lengths or angles in a right triangle. The key to using these ratios effectively is remembering which sides are referred to in relation to the angle of interest. For example, the “opposite” side is opposite the angle, while the “adjacent” side is next to it.

Using the Pythagorean Theorem

Another essential tool in trigonometry is the Pythagorean theorem, which applies specifically to right triangles. This theorem states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. In formula form, this is:

a² + b² = c²

Where a and b are the legs of the triangle, and c is the hypotenuse. This relationship is particularly useful when you know the lengths of two sides and need to find the third side.

By understanding and applying these fundamental concepts, you can confidently approach problems involving angles and side lengths in triangles, allowing you to solve a variety of geometric challenges with ease.

Time-Saving Tips for Final Exam

When preparing for an important assessment, managing your time effectively can make a significant difference in how well you perform. Efficiently navigating through the test ensures you have ample time to answer each question thoughtfully, while avoiding unnecessary stress. By applying specific strategies, you can maximize your focus and speed, allowing you to tackle even the most challenging problems with confidence.

Prioritize the Easy Questions

Start by identifying the questions that you can answer quickly and confidently. These are typically the ones that don’t require extensive calculations or complex reasoning. By tackling them first, you gain a sense of accomplishment and free up time for the more challenging sections of the test. This approach also helps you avoid getting stuck on difficult questions early on.

Use Process of Elimination

When faced with multiple-choice questions, don’t hesitate to eliminate obviously incorrect answers. Even if you are unsure about the correct choice, narrowing down the options increases your chances of guessing correctly. This technique saves time by reducing the number of choices you need to evaluate.

In addition, ensure you don’t dwell on any single question for too long. If you find yourself stuck, move on and return to it later if time permits. This way, you maximize your overall time allocation across the test.

How to Stay Calm During the Test

Maintaining a clear and composed mindset is key to performing well under pressure. Tests can be stressful, but staying calm allows you to think more clearly and solve problems efficiently. Developing techniques to manage your anxiety and stay focused will not only help you complete the task but also improve the quality of your responses.

Breathing Techniques

One of the simplest ways to reduce stress is through controlled breathing. Deep, slow breaths help activate the body’s relaxation response, lowering heart rate and calming the mind. Try the following technique:

Step	Description
1	Inhale slowly through your nose for 4 seconds.
2	Hold your breath for 4 seconds.
3	Exhale slowly through your mouth for 4 seconds.
4	Repeat for several minutes until you feel calmer.

Break Down the Test

Instead of focusing on the entire test at once, break it down into smaller, more manageable sections. Tackle one part at a time, and take short breaks if needed. This approach will help you stay organized and reduce feelings of being overwhelmed.