Pre Calc Final Exam Study Guide and Tips

As you approach your upcoming assessment in advanced mathematics, it’s crucial to have a solid understanding of the core principles and techniques that will be tested. With the right approach, you can confidently tackle complex problems and showcase your skills. This section will provide you with essential insights and strategies to enhance your performance.

Focusing on key concepts and practicing regularly is the best way to ensure you’re well-prepared. From understanding the fundamentals to mastering more intricate problem-solving methods, every element of the subject plays a vital role. Taking a structured approach to review will allow you to identify areas where you need improvement and boost your confidence.

In addition, using the right resources and adopting effective study techniques can make a significant difference in how well you perform. Whether you’re reviewing formulas, practicing equations, or solving word problems, your preparation will be more efficient with the right tools and mindset.

Essential Math Concepts to Master

To perform well in an advanced mathematics assessment, it’s vital to have a strong grasp of several core principles. These concepts form the foundation for solving a wide range of problems. Understanding them deeply will not only help you solve individual problems more effectively but also enhance your overall problem-solving skills.

Functions and Their Applications

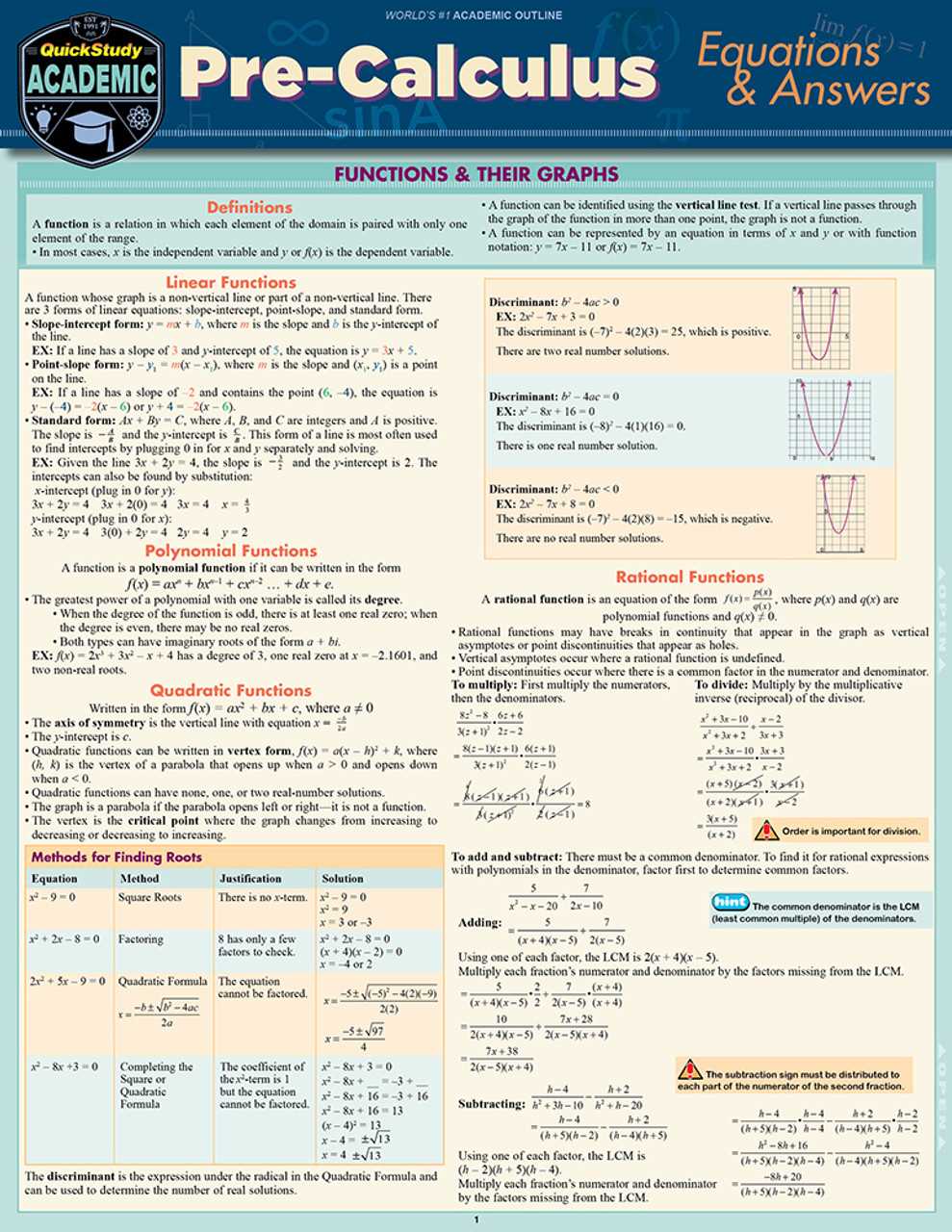
Mastering the concept of functions is crucial, as they are central to many problems you’ll encounter. Functions describe the relationship between two quantities, where one depends on the other. Understanding different types of functions, such as linear, quadratic, and exponential, will help you solve equations and interpret real-world problems. Additionally, being able to graph these functions and identify key characteristics, such as intercepts and asymptotes, is equally important.

Solving Equations and Inequalities

Another key area is the ability to solve equations and inequalities. These are foundational skills for tackling various problem types, from algebraic expressions to more complex expressions involving multiple variables. It’s important to know how to manipulate equations using algebraic rules and techniques such as factoring, substitution, and elimination. Understanding how to work with inequalities and their graphical representations will also be vital for success in problem-solving scenarios.

Effective Time Management for Assessment Preparation

One of the most important factors in preparing for a challenging math test is managing your time effectively. Without a clear plan, it can be easy to fall behind or feel overwhelmed. Proper time management helps you allocate enough time for each topic, stay on track, and avoid last-minute cramming. A strategic approach ensures that you cover all necessary material and are well-prepared for any type of question.

Creating a Study Schedule

Start by establishing a realistic timetable. Break down your study sessions into manageable blocks, focusing on one concept at a time. Consider how much time you have available and prioritize the most difficult or unfamiliar topics. Be sure to include breaks to prevent burnout and maintain focus throughout the session. Consistency is key, so try to stick to your schedule as closely as possible.

Using Practice and Review Sessions

Equally important is setting aside time for practice. Working through past problems and reviewing solutions helps reinforce what you’ve learned. Aim to simulate real test conditions during these sessions to build both speed and confidence. Afterward, review your mistakes and identify areas that need further attention. This type of active learning is crucial for retaining information and improving performance.

Top Resources for Mastering Advanced Math

Having access to the right resources can make a significant difference in your preparation. Whether you’re looking for online tools, textbooks, or video tutorials, the best materials will help you deepen your understanding and improve your problem-solving skills. Here are some of the top resources to consider as you prepare for your upcoming assessment.

Online Tools and Websites

The internet offers a wealth of platforms that cater specifically to mathematics. These resources often provide interactive exercises, practice problems, and video explanations. Some of the best websites include:

• Khan Academy – Offers free video lessons and exercises on a wide range of math topics.
• Wolfram Alpha – A powerful tool for solving complex problems and visualizing graphs.
• Desmos – An online graphing calculator that helps you explore functions and their properties.
• PatrickJMT – Features step-by-step explanations for various math problems.

Textbooks and Workbooks

Textbooks remain a reliable resource for comprehensive learning. Many math textbooks provide detailed explanations and practice problems with solutions. Workbooks, on the other hand, focus on providing hands-on practice. Some widely recommended books are:

• “Algebra and Trigonometry” by Robert F. Blitzer – Covers essential topics with plenty of practice exercises.
• “College Algebra” by Michael Sullivan – A great resource for both explanations and practice problems.
• “Precalculus” by James Stewart – Comprehensive guide for understanding advanced math concepts.

Video Tutorials and Lessons

If you prefer visual learning, video tutorials are an excellent way to understand complex concepts. Platforms such as YouTube have many channels dedicated to explaining mathematics in a clear, engaging way. Popular channels include:

• Professor Leonard – Offers in-depth, high-quality math lectures on a variety of topics.
• 3Blue1Brown – Known for visually explaining mathematical concepts with animations.
• PatrickJMT – Provides concise, easy-to-follow videos on various math problems.

Understanding Functions and Their Types

Functions are fundamental concepts in advanced mathematics that describe the relationship between two sets of values. Each input is associated with exactly one output, and understanding how to manipulate and analyze functions is key to solving a variety of problems. Mastering different types of functions will enable you to approach more complex mathematical challenges with confidence.

Linear and Quadratic Functions

Linear functions are the simplest type of function, represented by straight lines on a graph. These functions have a constant rate of change and can be expressed in the form y = mx + b, where m is the slope and b is the y-intercept. Quadratic functions, on the other hand, produce parabolic graphs and are represented by y = ax² + bx + c. These functions describe situations with variable rates of change, such as projectile motion or the path of an object under gravity.

Exponential and Logarithmic Functions

Exponential functions, written as y = a * b^x, describe situations where the rate of growth or decay is proportional to the current value, such as population growth or radioactive decay. Logarithmic functions, the inverse of exponential functions, are expressed as y = log_b(x), and are used to solve problems involving exponential growth or decay when the time is unknown. Both types of functions play an essential role in solving complex real-world problems.

Key Strategies for Solving Equations

Solving equations requires a combination of critical thinking, systematic steps, and familiarity with various techniques. By mastering a few key strategies, you can approach any equation confidently and efficiently. These methods help simplify complex problems, ensuring that you can isolate the unknown and find the correct solution.

Step-by-Step Approach

One of the most effective strategies is to break down the problem into smaller, manageable steps. Follow a consistent order of operations to simplify the equation. Start by eliminating any parentheses, then combine like terms, and finally isolate the variable. This systematic approach ensures that no steps are missed.

Using Substitution and Elimination

For equations with multiple variables, substitution and elimination methods are valuable tools. Substitution involves solving one equation for a variable and replacing that variable in another equation. Elimination, on the other hand, involves adding or subtracting equations to eliminate one variable, making it easier to solve for the remaining variable.

Step	Method	Example
1	Eliminate parentheses	2(x + 3) = 14 → 2x + 6 = 14
2	Combine like terms	3x + 5x = 16 → 8x = 16
3	Isolate the variable	8x = 16 → x = 2

How to Tackle Word Problems

Word problems often present a challenge because they require translating real-world scenarios into mathematical expressions. The key to solving these problems is to carefully extract relevant information, organize it, and then apply appropriate mathematical methods. By following a systematic approach, you can break down complex problems into manageable steps and find the solution with confidence.

Start by reading the problem carefully to understand what is being asked. Identify the given information and make note of any quantities or relationships mentioned. Next, translate the words into mathematical symbols and expressions. This may involve setting up equations or recognizing patterns. Once the problem is in a mathematical form, apply the relevant concepts, such as algebraic manipulation or geometry, to find the answer.

Finally, after solving the equation or performing the necessary operations, check your work. Ensure that the solution makes sense in the context of the problem. This step helps avoid errors and ensures that the solution is both mathematically correct and logically valid.

Reviewing Graphing Techniques

Graphing is an essential skill in mathematics, as it allows you to visually interpret relationships between variables. Whether you’re working with linear, quadratic, or more complex functions, understanding the techniques for graphing will help you better understand the behavior of these equations. By mastering key concepts and methods, you can confidently plot graphs and analyze their properties.

One of the first techniques to master is plotting points. This involves selecting specific values for the independent variable, substituting them into the equation, and then plotting the corresponding points on a coordinate plane. For more complex equations, it’s important to understand key features of the graph, such as intercepts, slopes, and asymptotes. These elements help to sketch the overall shape of the graph before refining it with more points.

Another key aspect is recognizing the transformations that affect the graph’s position or shape. These include vertical and horizontal shifts, stretches, and reflections. For example, adding or subtracting constants to the equation will shift the graph up or down, while multiplying by a factor will stretch or compress the graph. Understanding these transformations allows you to quickly graph more complex equations without plotting every individual point.

Common Mistakes to Avoid on the Test

When preparing for any major assessment, it’s essential to not only focus on the correct methods but also to be aware of common errors that can easily slip by. These mistakes can often be the difference between getting the right answer and making unnecessary errors. Understanding what to look out for and how to avoid these pitfalls will help you approach the test with greater confidence.

Misinterpreting the Question

One of the most frequent mistakes students make is misinterpreting the question. It’s easy to jump into solving without fully understanding what is being asked. Always take time to read the problem carefully and make sure you know exactly what you need to find. If there are multiple parts to the problem, break it down and tackle them one step at a time. Ensure you’re solving for the correct variable and applying the right concepts to the specific situation presented.

Neglecting to Check Work

Another common mistake is not reviewing your answers. It’s tempting to submit your work as soon as you’ve solved the problem, but skipping this step increases the chances of missing small errors. After completing a problem, go back and verify that all steps are correct and that you’ve followed the proper procedure. Checking calculations, signs, and units can help prevent simple mistakes that might affect your final answer.

Tips for Memorizing Formulas Quickly

Memorizing formulas is an essential skill when preparing for mathematical assessments. However, with numerous equations to remember, it can feel overwhelming. Fortunately, there are several effective strategies that can help you commit important formulas to memory quickly and efficiently. By applying these techniques, you can improve recall and ensure you have the formulas at your fingertips when needed.

• Break it Down: Start by breaking the formula into smaller, manageable parts. Understand the components and how they relate to each other before attempting to memorize the entire formula. This makes it easier to retain.
• Use Mnemonics: Create memorable phrases or shortcuts to help you remember the formula. Mnemonics can be a powerful tool for encoding complex ideas into simple, easily recallable associations.
• Write and Rewrite: Repetition is key to memorization. Write out the formula multiple times to reinforce the pattern. By physically writing it, you’re engaging both visual and motor memory, improving retention.
• Visualize the Formula: Draw diagrams or graphs related to the formula. Visualizing the equation in a real-world context can help make the formula more meaningful and easier to recall.
• Practice Regularly: Regular review is crucial. Set aside time to revisit formulas each day, even if it’s just for a few minutes. The more frequently you encounter the formula, the easier it will be to remember.

By employing these techniques consistently, you’ll be able to memorize formulas more effectively and recall them under pressure when it matters most.

Using Practice Exams to Improve Skills

One of the most effective ways to refine your problem-solving abilities and boost your confidence is through regular practice. Simulating real testing conditions by completing practice assessments allows you to identify areas where you need improvement, track your progress, and become more familiar with the types of questions you may encounter. This approach provides valuable experience, helping you perform better when it matters most.

• Simulate Real Conditions: Treat practice tests like the actual assessment by timing yourself and working in a distraction-free environment. This will help you build the stamina and focus needed to complete tasks under pressure.
• Review Mistakes: After completing a practice test, take time to thoroughly review your errors. Understand why you made each mistake and figure out how to avoid it in the future. This reflection process is key to improving your understanding of the material.
• Identify Patterns: Regular practice allows you to identify common themes or question types. Once you become familiar with recurring concepts, you can focus your efforts on mastering those areas.
• Build Time Management Skills: Practice exams not only test your knowledge but also help you develop efficient time management. By working through problems at a steady pace, you’ll learn how to allocate your time wisely during the actual test.
• Increase Confidence: The more you practice, the more confident you will become. Familiarity with the format and content of the questions can reduce test anxiety and allow you to approach the assessment with a calm mindset.

Consistent practice will help solidify your skills, boost your performance, and ultimately prepare you to excel in any mathematical challenge you face.

Breaking Down Trigonometric Functions

Trigonometric functions are fundamental tools in mathematics, widely used to describe relationships between angles and side lengths in triangles, as well as periodic phenomena. These functions can initially seem complex, but once you break them down, understanding them becomes much easier. By grasping their properties, behavior, and the key concepts behind them, you can tackle problems with greater confidence.

At the core of trigonometry are six primary functions: sine, cosine, tangent, cosecant, secant, and cotangent. Each of these functions is tied to a specific relationship between the angles and sides of a right triangle. For instance, the sine of an angle represents the ratio of the length of the opposite side to the hypotenuse, while the cosine represents the ratio of the adjacent side to the hypotenuse. Understanding these ratios is crucial for solving a wide range of problems, from calculating distances to analyzing waveforms.

To simplify the process of mastering trigonometric functions, it’s important to become familiar with their key characteristics, such as periodicity, amplitude, and phase shifts. These properties allow you to predict the behavior of the functions in various contexts, such as graphing or solving equations. Additionally, knowing how to use the unit circle as a tool for evaluating the functions at various angles can significantly streamline the problem-solving process.

With consistent practice and a clear understanding of these concepts, trigonometric functions will become an intuitive part of your mathematical toolkit, enabling you to solve complex problems with ease.

Mastering Limits and Continuity

Understanding the concepts of limits and continuity is essential for navigating advanced mathematical topics. These foundational principles describe the behavior of functions as they approach certain points or values. By mastering these concepts, you can gain deeper insight into how functions behave and apply this knowledge to solve a variety of problems, from evaluating complex expressions to analyzing real-world phenomena.

Limits: Understanding Function Behavior

At its core, a limit represents the value that a function approaches as its input gets closer to a particular point. This idea helps to explain how functions behave near certain values, even if the function doesn’t actually reach that value. Limits are used to determine whether a function has a specific value at a given point, or whether it approaches infinity. Mastering limits involves learning various techniques, such as factoring, rationalizing, or applying L’Hôpital’s Rule, to calculate the limit of a function in different situations.

Continuity: Connecting the Dots

Continuity ensures that a function behaves in a smooth, unbroken manner at a given point. A function is continuous if there are no jumps, gaps, or holes in its graph at that point. To check for continuity, it’s important to evaluate the function at the point in question, and ensure that the limit from both sides matches the function’s value. Understanding continuity allows you to determine the stability and smoothness of functions, which is especially important in areas like physics and engineering, where sudden changes can have significant consequences.

With regular practice and application of these concepts, you’ll develop a strong grasp of how functions behave near critical points, equipping you with the tools to solve complex mathematical problems and advance your understanding of calculus.

Handling Polynomials and Rational Expressions

Polynomials and rational expressions are fundamental components in algebra, and mastering them is crucial for solving complex mathematical problems. These expressions involve variables raised to powers, and understanding how to manipulate them is key to unlocking a wide range of mathematical techniques. Whether you’re simplifying, factoring, or performing operations, knowing how to handle these expressions effectively can simplify many problems.

Polynomials are expressions that consist of variables raised to integer powers, combined with coefficients. They can range from simple linear expressions to more complex quadratic or cubic forms. Mastering how to simplify and factor polynomials allows you to break them down into more manageable parts. Techniques like factoring by grouping, using the distributive property, and applying the difference of squares are essential for solving polynomial equations.

Rational expressions, on the other hand, are fractions where the numerator and denominator are both polynomials. To work with these expressions, it’s important to understand how to simplify them by factoring both the numerator and denominator. Common operations include finding common denominators, adding and subtracting rational expressions, and solving rational equations. Understanding restrictions on the domain of these expressions, especially when the denominator equals zero, is also critical for ensuring the validity of your solutions.

By practicing these techniques and refining your skills, you’ll develop the ability to handle both polynomials and rational expressions with ease, allowing you to approach a variety of algebraic problems with confidence.

Exam Day Tips for Maximum Performance

The day of a significant assessment can be stressful, but with the right mindset and preparation, you can maximize your performance. Knowing how to manage your time, stay calm, and approach each question methodically can make a substantial difference. These practical strategies will help you navigate the assessment confidently and effectively, ensuring that you’re ready to showcase your knowledge.

Effective Time Management

One of the most important aspects of a successful assessment is managing your time wisely. Knowing how long to spend on each section can prevent you from rushing through difficult questions or spending too much time on simpler ones. Here’s a suggested approach to time allocation:

Section	Suggested Time Allocation
Multiple Choice	30% of the total time
Short Answer	40% of the total time
Problem Solving	30% of the total time

Stay Calm and Focused

Keeping a clear head is crucial during the assessment. Stress can cloud your judgment and make even simple problems appear more complex than they are. Practice deep breathing exercises, take short breaks if needed, and avoid panicking when faced with challenging questions. The key is to stay calm and approach the task one step at a time.

By applying these strategies, you’ll be well-equipped to tackle your assessment with focus and efficiency, maximizing your performance on the big day.

Dealing with Test Anxiety Effectively

For many individuals, the pressure of an important assessment can lead to anxiety, which may affect performance. Learning how to manage this stress is essential for maintaining focus and performing at your best. This section explores practical methods to overcome nervousness and stay calm during an evaluation, helping you to approach the task with confidence and clarity.

Understanding the Root Causes

Anxiety often stems from fear of failure, self-doubt, or the overwhelming pressure to perform perfectly. Recognizing that these feelings are common can help you take the first step toward addressing them. Acknowledging your emotions and reframing negative thoughts can make a significant difference in how you approach the situation. The goal is to shift from a mindset of fear to one of control.

Practical Techniques to Calm Nerves

There are several effective strategies that can help reduce anxiety before and during the assessment:

• Breathing Exercises: Practice deep, slow breathing to relax your body and mind. This can reduce the physical symptoms of anxiety, such as rapid heartbeat and shallow breathing.
• Visualization: Picture yourself succeeding and handling the assessment confidently. Positive visualization can improve your self-assurance.
• Breaks and Movement: Take short breaks to stand, stretch, or walk around briefly. Physical movement can release tension and help reset your mental focus.

Incorporating these techniques into your preparation routine can help you build resilience against test-related stress, ensuring you are better equipped to handle the situation calmly and effectively.

What to Do After the Exam

Once the assessment is complete, it’s important to shift focus to recovery and reflection. Taking care of yourself and reviewing your performance can help you gain valuable insights and ensure you’re prepared for future challenges. The post-assessment period is an opportunity to relax, reflect, and plan your next steps.

Relax and Recharge

Immediately after completing a significant task, it’s essential to give yourself a break. Here are some activities to consider for relaxation:

• Engage in a hobby: Take some time to do something you enjoy, whether it’s reading, listening to music, or spending time outdoors.
• Physical activity: A quick walk, yoga, or exercise can help release tension and improve your mood after the pressure of the task.
• Socialize: Catch up with friends or family to share your experience and disconnect from the stress of the assessment.

Reflect on Your Performance

While it’s important to relax, reflecting on your performance can help you understand areas of strength and opportunities for growth. Here’s how you can approach this:

• Review your approach: Think about how you approached the task. Were there strategies that worked well, or could you improve your methods for next time?
• Assess emotional management: Consider how you handled stress and time management. Were you able to stay calm, or did anxiety impact your performance?
• Set new goals: Based on your reflections, identify areas to focus on for the future. Use what you’ve learned to guide your next steps in your learning journey.

By taking these steps after the assessment, you can ensure that you stay balanced, learn from the experience, and set yourself up for success in the next challenge.