8th Grade Math Practice Test with Answer Key

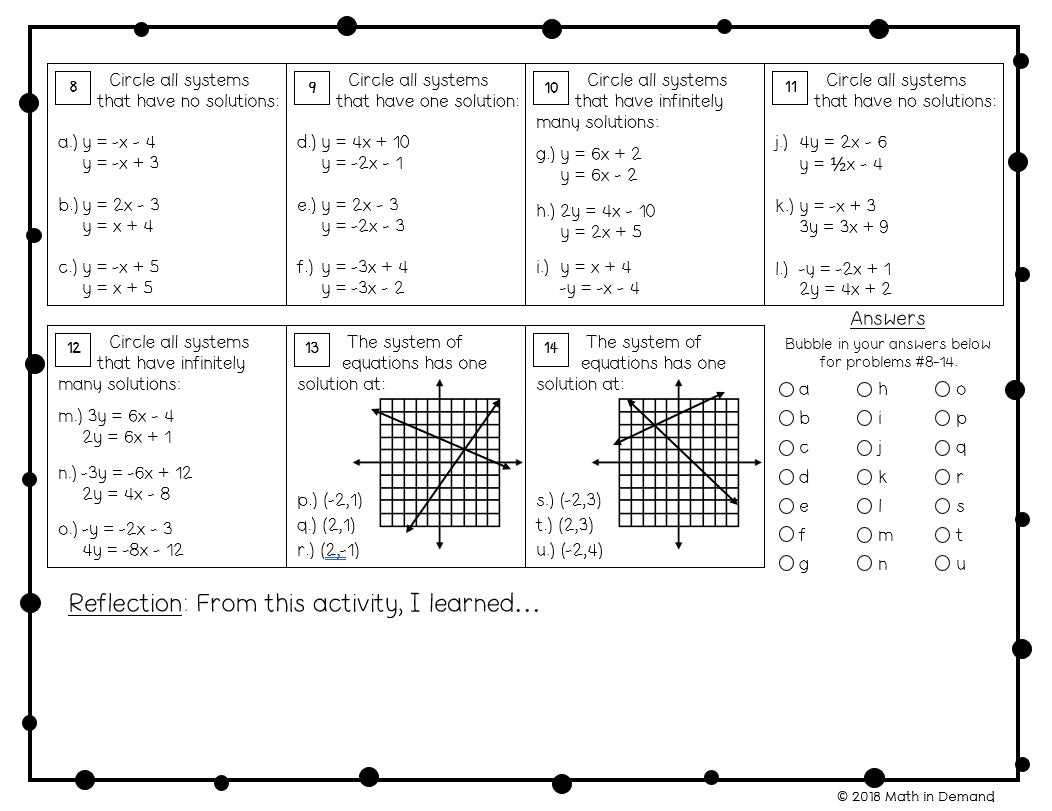
As students approach important assessments, it becomes crucial to sharpen their skills and review key concepts. Mastering these areas can lead to improved performance and a deeper understanding of the subject matter. This section is designed to help students identify their strengths and areas for improvement through a series of focused exercises.

Effective preparation involves not only practicing problems but also reflecting on the solutions and recognizing patterns. By revisiting solved examples, learners can gain clarity on the methods used and solidify their grasp of essential techniques. This approach fosters confidence and better retention of information.

Whether preparing for a specific evaluation or simply reinforcing foundational knowledge, practicing various problem types is an essential strategy. Utilizing resources that offer immediate feedback can accelerate the learning process, helping students stay on track and reach their full potential.

8th Grade Math Practice Test Overview

To excel in any academic evaluation, it’s important to be familiar with the types of questions that will be asked. A well-structured series of exercises can guide learners through the essential topics, helping them build both skill and confidence. By engaging with a variety of problems, students can focus on areas that require further attention and improve their overall performance.

This section provides an outline of the key areas covered in such assessments. The following categories are typically included:

• Basic operations and number theory
• Algebraic expressions and equations
• Geometrical concepts and shapes
• Data analysis and interpretation
• Word problems and critical thinking tasks

Each topic focuses on a specific set of skills that are essential for mastering the subject. Understanding these concepts will help students approach each challenge more effectively and develop a stronger grasp of core principles. Practicing these areas in detail can also reveal any gaps in understanding, providing an opportunity for targeted review.

By engaging with the exercises, learners can refine their ability to solve problems quickly and accurately, ensuring they are prepared for the actual evaluation. Immediate feedback, when available, allows students to check their progress and adjust their strategies accordingly.

Key Concepts Covered in the Test

To ensure success in any examination, it is essential to grasp the core principles and methods that are regularly assessed. The evaluation typically focuses on several important topics that are foundational for further learning. Understanding these concepts is crucial, as they form the basis for solving a variety of problems that may arise.

Basic Arithmetic and Number Theory

This category includes essential calculations such as addition, subtraction, multiplication, and division. A strong command of number properties, factors, multiples, and prime numbers is necessary for solving more complex problems. Learners will encounter questions that require quick mental math as well as those that involve step-by-step problem-solving.

Algebraic Equations and Functions

Algebra is a central focus in many assessments. Students need to understand how to manipulate variables, solve linear equations, and work with inequalities. Topics like simplifying expressions, solving for unknowns, and understanding functions are key areas that frequently appear. These concepts help in developing critical thinking and problem-solving abilities.

These concepts are not only important for passing exams but also for building a deeper understanding of more advanced topics in the future. Mastering these areas ensures that students have the skills needed to tackle real-world challenges and apply their knowledge in practical situations.

How to Prepare for the Exam

Effective preparation is crucial for performing well in any assessment. It involves more than just reviewing materials–it’s about understanding the key concepts, refining your skills, and building confidence. A systematic approach to preparation will help you stay focused and organized throughout the process.

Here are some strategies to consider:

Preparation Step	Description
Review Key Topics	Go over all the essential concepts that are likely to appear on the assessment. This includes operations, equations, geometry, and data analysis.
Practice Regularly	Consistent practice helps solidify understanding and improves speed. Work through various problems to gain confidence in solving different types of questions.
Work on Weak Areas	Identify areas where you’re struggling and focus on improving them. This might involve revisiting specific topics or seeking additional resources for better understanding.
Use Feedback Wisely	Review solved problems and understand where you went wrong. Use any available feedback to adjust your approach and avoid similar mistakes in the future.
Simulate Exam Conditions	Practice under timed conditions to simulate the pressure of the actual exam. This will help you manage time effectively and reduce anxiety.

By following these steps, you can ensure you’re thoroughly prepared and ready to tackle any challenge that comes your way during the assessment.

Understanding Common Math Challenges

When preparing for any examination involving numerical reasoning, it’s essential to be aware of the common difficulties many students face. Recognizing these challenges early allows learners to focus on overcoming them, improving their problem-solving ability, and building greater confidence. Understanding where mistakes are likely to occur can help prevent them in the future.

Some of the most frequent obstacles include:

• Complex Problem Solving: Many students struggle with multi-step problems that require the application of different concepts simultaneously.
• Algebraic Manipulations: Simplifying equations, working with variables, and solving for unknowns can be confusing, especially when the rules are not fully understood.
• Word Problems: Translating real-world scenarios into mathematical operations often leads to misunderstandings of the question’s core requirements.
• Time Management: Under time pressure, it can be difficult to balance accuracy and speed, leading to rushed calculations and avoidable mistakes.
• Attention to Detail: Missing small details or making careless errors during calculations is a common issue, especially when working under pressure.

By identifying these challenges early in the preparation process, students can adopt strategies to address them, turning weaknesses into strengths. Practicing these types of problems regularly will help increase familiarity and make tackling them more manageable during the actual evaluation.

Step-by-Step Solutions for Practice Problems

Breaking down complex problems into manageable steps is an effective way to build understanding and gain confidence. By carefully analyzing each problem and working through it systematically, students can learn to apply techniques consistently, ensuring they can solve similar questions with ease in the future. This method not only improves accuracy but also helps develop a clear approach to problem-solving.

Here’s how to approach any problem step by step:

• Step 1: Understand the problem. Carefully read the question to identify what is being asked. Highlight key information and terms.
• Step 2: Identify relevant concepts. Determine which mathematical rules or formulas will be needed to solve the problem.
• Step 3: Break the problem into smaller parts. If the problem requires multiple steps, divide it into manageable chunks to solve each part individually.
• Step 4: Perform calculations. Carefully follow each step, ensuring all operations are performed correctly and in the right order.
• Step 5: Check the solution. Once the problem is solved, review the result to make sure it makes sense in the context of the question.

Following these steps allows students to approach each problem logically and methodically. With consistent practice, this approach will help solidify understanding and improve overall performance in any assessment.

Importance of Answer Keys for Learning

Having access to solutions after completing a set of problems is a valuable tool for understanding and improvement. It provides learners the opportunity to verify their work, identify any mistakes, and see the correct approach to solving a problem. This immediate feedback enhances the learning process by making students aware of where they went wrong and how to fix their errors in the future.

One of the key benefits of reviewing solutions is that it allows students to learn from their mistakes. Rather than simply moving on to the next set of problems, they can take the time to analyze the steps that led to the incorrect answer. This reflection process helps reinforce correct methods and solidify concepts in their minds.

Moreover, by comparing their own methods to the provided solutions, learners can discover more efficient or alternative ways of solving problems. This promotes critical thinking and encourages students to approach challenges from different angles, ultimately improving their problem-solving skills.

Tips for Improving Math Skills

Improving your problem-solving abilities requires consistent effort, focus, and a strategic approach. There are several effective ways to enhance your skills and gain confidence in your ability to tackle various challenges. By incorporating these strategies into your study routine, you can build a strong foundation and become more proficient in handling different types of questions.

• Review Fundamental Concepts: Mastering the basics is essential. Make sure you understand foundational principles before moving on to more complex problems. This provides a solid base for tackling harder concepts.
• Practice Regularly: Consistency is key. The more you practice, the more comfortable you will become with solving different types of problems. Regular practice helps to reinforce concepts and improve retention.
• Break Down Problems: When faced with a difficult challenge, break it into smaller, manageable parts. Solve each part step by step to avoid feeling overwhelmed.
• Learn from Mistakes: Don’t be discouraged by errors. Review where you went wrong and understand the correct method. Learning from mistakes is an essential part of the process.
• Seek Help When Needed: If you’re struggling with a specific topic, don’t hesitate to ask for assistance. Whether from a teacher, tutor, or online resource, getting help can clarify difficult concepts.

By adopting these practices, you will steadily build your skills and feel more confident in your abilities to solve a variety of challenges effectively. Improvement comes over time with persistence and the right strategies in place.

Time Management During the Test

Effective time management is crucial when facing any timed evaluation. Without careful planning, it’s easy to spend too much time on certain problems, leaving less time for others. By learning how to allocate time wisely and stay on track, you can maximize your performance and ensure that all questions are answered thoroughly.

Here are some tips for managing time efficiently during an assessment:

Tip	Description
Read Instructions Carefully	Start by reading the instructions to avoid wasting time later. Clear understanding of what’s expected will prevent mistakes and confusion.
Plan Your Time	Divide the total time by the number of questions. Allocate an appropriate amount of time for each section based on its difficulty and point value.
Start with Easier Questions	Answer the simpler questions first to build confidence and ensure that you secure easy points before tackling more challenging ones.
Don’t Get Stuck	If you find a question too difficult, move on and come back to it later. Spending too much time on one problem can hurt your overall score.
Check Your Work	If time allows, review your answers. A quick check can catch mistakes you may have overlooked initially.

By applying these strategies, you can ensure that you manage your time wisely, allowing you to complete the entire evaluation while maintaining accuracy and focus.

Using Practice Tests Effectively

Simulated assessments are a powerful tool for reinforcing learning and preparing for actual evaluations. By completing these exercises, students can gauge their understanding, identify areas for improvement, and refine their problem-solving strategies. However, simply taking these exercises isn’t enough – it’s essential to approach them strategically to maximize their value.

To use practice sessions effectively, consider the following tips:

• Replicate Exam Conditions: Complete the practice exercises under timed conditions to simulate the real evaluation experience. This will help you get accustomed to working under pressure and managing your time effectively.
• Review Mistakes Thoroughly: After finishing the exercises, go through every mistake carefully. Understand where you went wrong and why. This process is essential for learning and preventing similar errors in the future.
• Focus on Weak Areas: Pay extra attention to the sections or topics where you struggled the most. Revisit those concepts and reinforce your understanding through additional exercises or review.
• Track Progress: Monitor your performance over time. If you see improvement in certain areas, it means your strategies are working. If not, adjust your approach and focus more on those challenging topics.
• Practice Regularly: Consistent practice is key to mastering any subject. Try to complete practice exercises frequently, not just when a test is approaching.

By incorporating these techniques into your study routine, practice sessions will become an invaluable tool in mastering new concepts and achieving better results on actual assessments.

Types of Questions on the 8th Grade Math Test

The assessment is designed to cover a wide range of topics and question types to evaluate a student’s overall understanding and problem-solving abilities. These questions test both basic knowledge and the ability to apply concepts to more complex scenarios. Understanding the different types of questions can help students prepare effectively by recognizing patterns and anticipating the kinds of problems they will face.

Multiple Choice Questions

Multiple-choice questions are common and require students to select the correct answer from a list of options. These questions typically assess a student’s ability to recognize the right solution among several possible choices. They can test a variety of topics, from basic calculations to more complex problem-solving scenarios.

Short Answer and Computation Problems

These questions require students to work through a problem and provide a written answer. This type of question tests a student’s ability to execute calculations and show their work. Often, these problems require step-by-step solutions, allowing students to demonstrate their understanding of the methods and processes involved.

In addition to these types, students may encounter questions that require the application of concepts in real-world contexts, such as word problems, graph interpretation, and reasoning questions. Each question type provides valuable insight into different aspects of a student’s mathematical abilities and challenges them to think critically and systematically.

Focus Areas in Algebra and Geometry

Algebra and geometry form two of the most essential areas in the assessment. Mastering these topics is key to achieving success, as they involve both theoretical understanding and practical application. The questions often combine abstract concepts with real-world scenarios, requiring students to think critically and solve problems step by step. Below are the key focus areas within these two subjects that students should pay special attention to while preparing.

Algebra Focus Areas

• Solving Equations: Students should be able to solve linear and quadratic equations, including those with one variable.
• Understanding Functions: Knowing how to interpret and manipulate functions, including working with slope and intercepts, is crucial.
• Operations with Polynomials: Simplifying, adding, subtracting, and multiplying polynomials are fundamental algebraic skills.
• Factoring: Factoring expressions, such as quadratic trinomials, is an important skill to master for solving more complex problems.
• Systems of Equations: Solving systems of equations using substitution or elimination methods is another area of focus.

Geometry Focus Areas

• Properties of Shapes: Understanding the properties of various geometric shapes, including triangles, quadrilaterals, and circles, is essential.
• Measurement and Perimeter: Calculating the area, perimeter, and volume of 2D and 3D shapes, as well as using the Pythagorean theorem, are key skills.
• Angles and Lines: Knowledge of parallel and perpendicular lines, as well as calculating angles formed by intersecting lines, is tested frequently.
• Transformations: Identifying and performing transformations such as rotations, reflections, and translations is another critical concept.

By honing skills in these areas, students will be well-prepared to tackle questions in both algebra and geometry, providing a solid foundation for more advanced concepts in mathematics.

Mastering Word Problems in Math

Word problems often present a unique challenge, as they require students to translate real-world situations into mathematical equations. These types of problems assess not only a student’s ability to perform calculations, but also their comprehension and problem-solving skills. To excel in this area, it’s crucial to understand the structure of word problems and apply the right strategies to solve them effectively.

The key to mastering word problems lies in breaking them down into manageable steps. Begin by carefully reading the problem to identify important information and what is being asked. Then, translate the situation into an equation or set of equations that can be solved. Finally, review the solution to ensure it answers the original question and makes sense in the context of the problem.

• Read and Understand the Problem: Pay attention to key details such as numbers, relationships, and operations indicated in the problem.
• Identify the Goal: Clearly define what you are being asked to find–whether it’s a value, a ratio, or a comparison.
• Translate Words into Math: Use appropriate symbols and operations to convert the word problem into a mathematical expression.
• Solve Step by Step: Carefully perform each calculation, ensuring each step is logical and correct before moving forward.
• Check Your Work: Review the solution to verify that it answers the question posed and fits the context of the problem.

By practicing these strategies, students can develop a systematic approach to word problems and improve their ability to tackle even the most complex scenarios confidently and efficiently.

Common Mistakes to Avoid in Math

Even the most proficient students can fall victim to simple errors during calculations or problem-solving. Recognizing and avoiding common mistakes is a crucial part of improving accuracy and confidence. Many of these errors are preventable with careful attention to detail and a systematic approach. Below are some of the most frequent missteps that students encounter and strategies for avoiding them.

• Misinterpreting the Problem: Often, students rush through word problems and fail to fully understand what is being asked. It’s important to read the problem carefully, paying attention to keywords and units of measurement.
• Skipping Steps: Jumping straight into solving without properly setting up the equation or identifying the correct approach can lead to mistakes. Always write out the necessary steps and check your work as you go.
• Overlooking Negative Signs: One of the most common errors is forgetting to account for negative signs when performing operations. Always be mindful of both positive and negative numbers, especially during subtraction or when dealing with equations.
• Incorrectly Applying Formulas: Using the wrong formula or applying it incorrectly is a frequent issue. Before starting any calculations, make sure you are using the right equation and understand how each variable is defined.
• Forgetting to Simplify: After solving an equation, students sometimes forget to simplify the result or check for any further reductions. Always double-check for simplifications to ensure your final answer is in its simplest form.
• Making Calculation Errors: Simple arithmetic mistakes, like incorrect addition or multiplication, can skew the entire solution. It’s always a good idea to review your calculations, especially after performing multiple operations.

By being mindful of these common errors and adopting a more methodical approach to problem-solving, students can significantly improve their accuracy and efficiency, leading to better results and a deeper understanding of the material.

Building Confidence for the Exam

Confidence is essential when approaching any assessment. Feeling self-assured allows you to think clearly, approach each problem methodically, and manage stress effectively. One of the most effective ways to build confidence before an exam is through consistent preparation and understanding your strengths and weaknesses. The more familiar you become with the types of questions and concepts covered, the more relaxed and capable you’ll feel when it’s time to sit down and start solving problems.

Strategies for Building Confidence

There are several approaches that can help students feel more confident and prepared for their upcoming exam. The key is to combine practice, positive thinking, and thorough review. Below are some strategies that can make a big difference:

Strategy	Description
Consistent Practice	Regularly practicing problems is one of the best ways to build familiarity and reduce anxiety. The more problems you solve, the easier it becomes to identify patterns and apply the right strategies.
Identify Weak Areas	Take note of the areas where you struggle the most and focus extra time on improving those. Tackling difficult concepts head-on builds competence and reduces uncertainty during the exam.
Simulate Exam Conditions	Recreating the exam environment by timing yourself and working in a quiet setting helps train your mind to focus and perform under pressure.
Positive Affirmation	Adopt a positive mindset before and during your preparation. Remind yourself of past successes and trust in your ability to perform well.
Review Mistakes	Rather than just practicing problems, make sure to review your mistakes. Understanding why something went wrong helps prevent similar errors in the future.

Overcoming Exam Anxiety

Aside from technical preparation, managing anxiety plays a huge role in boosting confidence. Many students experience nervousness, but recognizing that it’s a natural part of the process can help. By employing relaxation techniques such as deep breathing or visualizing success, you can calm your nerves and approach the exam with a clearer, more focused mind. A confident mindset can be the difference between a stressful and successful exam experience.

Reviewing Solutions and Identifying Gaps

After completing a set of questions or exercises, it’s crucial to spend time reviewing the solutions in detail. This process not only helps in confirming the correctness of your answers but also in understanding the reasoning behind each step. By thoroughly analyzing your responses, you can pinpoint areas where mistakes were made and identify gaps in your knowledge that need to be addressed. This reflective approach is key to improving both accuracy and overall understanding.

When reviewing, focus on the following steps:

• Check for calculation errors: Look for simple mistakes such as arithmetic errors, misplacing decimal points, or incorrect signs. These small errors can add up, impacting the accuracy of your answers.
• Understand the problem-solving approach: Evaluate whether the strategy used to solve the problem was the most efficient or appropriate. If another method could have yielded faster results, take note of it for future reference.
• Identify patterns: Often, problems of similar types appear across different sections or exams. Recognizing these patterns can help you predict and prepare for similar challenges in the future.
• Analyze misunderstood concepts: If certain topics or concepts continue to pose challenges, it’s essential to revisit the underlying principles. Going back to the basics and working through examples can help solidify your understanding.

Identifying knowledge gaps early allows you to focus your efforts on strengthening those areas. This proactive approach not only improves your ability to tackle similar questions in the future but also boosts your confidence when faced with new material.

Benefits of Regular Practice

Consistent engagement with exercises and problem-solving activities plays a critical role in improving both speed and accuracy. Regularly working through a variety of challenges allows individuals to reinforce their understanding of key concepts while building confidence. Over time, this habit leads to a deeper grasp of the subject matter and enhances problem-solving abilities, ensuring better performance in assessments.

Here are some key advantages of staying consistent with your practice:

• Improved Retention: Repetition strengthens neural pathways and helps retain information for longer periods. This leads to greater ease when recalling methods and formulas during real challenges.
• Faster Problem-Solving: The more familiar you are with different types of problems, the quicker you can identify solutions. Regular exposure to a range of exercises helps you develop shortcuts and more efficient strategies.
• Increased Confidence: Each time you successfully solve a problem, no matter how complex, your belief in your abilities grows. This mental boost makes it easier to tackle new, unfamiliar questions without hesitation.
• Better Error Identification: The more you practice, the more attuned you become to common mistakes. This ability to quickly identify and correct errors is a crucial skill in improving your accuracy.
• Enhanced Analytical Thinking: Working through various types of problems encourages logical thinking and helps you break down challenges into manageable steps, a skill that can be applied to other areas as well.

Incorporating regular exercises into your routine leads to steady progress, making future challenges less intimidating and helping you achieve a higher level of proficiency in your skills.

How to Use the Answer Key for Review

When reviewing completed exercises, it’s essential to approach the provided solutions strategically. Simply looking at the correct responses is not enough; it is more beneficial to understand the reasoning behind each answer. This method of active review helps you not only identify mistakes but also improve your problem-solving techniques for the future.

Step 1: Check Your Work Thoroughly

Start by comparing your responses to the given solutions. Rather than just focusing on whether your answers are correct or not, examine the process you followed. Did you use the appropriate steps? Were there any shortcuts you missed? This reflective practice allows you to spot where your approach may have been flawed and helps reinforce correct methods.

Step 2: Analyze Mistakes and Learn from Them

Pay special attention to any discrepancies between your solution and the correct one. Mistakes are invaluable learning opportunities. When you make an error, try to understand why it happened–did you misinterpret the question, skip a crucial step, or make a calculation error? Once identified, you can focus on improving that specific area in your future exercises.

• Identify Patterns: If you make similar mistakes in multiple problems, it’s a signal that you need to review that particular concept more thoroughly.
• Clarify Confusing Concepts: Use external resources, such as textbooks or online tutorials, to better understand concepts that caused confusion during your review.
• Seek Alternative Methods: If your method for solving a problem was inefficient or overly complicated, look at the solution and see if there’s a simpler approach you can adopt.

Step 3: Reinforce Correct Strategies

Once you’ve analyzed your errors, focus on the correct strategies that led to accurate results. Try to apply these approaches to new problems to ensure that they become second nature. By consistently reinforcing correct methods, you improve your ability to approach similar challenges in the future.

By using the provided solutions thoughtfully, you can turn mistakes into growth opportunities and refine your problem-solving skills, paving the way for greater success in future assessments.