Algebra 1 Practice Final Exam with Answer Key

algebra 1 practice final exam with answer key

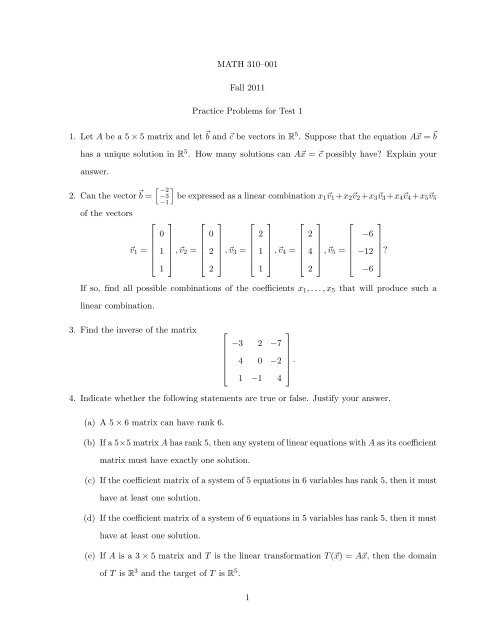
Understanding Algebra 1 Key Concepts

Importance of Practice Exams in Learning

Common Topics in Algebra 1 Finals

How to Approach Your Algebra 1 Exam

Mastering Equations and Inequalities

Solving Systems of Linear Equations

Working with Polynomials and Factoring

Tips for Tackling Word Problems

Understanding Functions and Graphing

Exponents and Radicals Simplified

Quadratic Equations: Key Techniques

Algebraic Expressions and Operations

Time Management During the Exam

How to Use the Answer Key Effectively

Identifying Common Mistakes in Algebra

Boosting Confidence Before the Exam

Reviewing Your Final Exam Results

Algebra 1 Practice Final Exam with Answer Key

Preparing for a mathematics assessment requires a solid understanding of key principles. This section will help strengthen your grasp on essential topics, offering a comprehensive review and solutions to common problem types. The focus is on reinforcing knowledge and boosting problem-solving confidence.

To improve your skills, it’s important to focus on specific areas that often appear in these types of tests. Consider working through a variety of problems that test your ability to manipulate variables, solve equations, and interpret graphs. Here are some key areas to concentrate on:

Solving linear and quadratic expressions
Understanding systems of equations and their solutions
Working with inequalities and their graphical representations
Factoring and simplifying algebraic expressions
Interpreting functions and their behaviors

Utilizing the provided solutions to check your work is crucial for understanding your mistakes and preventing them in the future. This will guide you in refining your strategies and enhancing your ability to solve similar problems more efficiently.

After going through the exercises, reviewing the solutions will help clarify any uncertainties. Pay special attention to the steps involved in arriving at the solution and make sure you understand why each choice is made during the process.

Regular practice in these areas will lead to greater accuracy and faster problem-solving abilities, ensuring you’re prepared for any challenging problem types that may appear during your assessment.

Understanding Algebra 1 Key Concepts

Mastering the fundamentals of mathematical reasoning is essential for solving a wide variety of problems. A deep understanding of core principles allows you to approach and solve complex challenges with confidence. The following concepts form the foundation of the subject and should be thoroughly reviewed to build a strong skill set.

Some of the most important areas to focus on include:

Solving and simplifying expressions
Manipulating variables and constants
Understanding and graphing linear equations
Working with systems of equations
Identifying and factoring polynomials
Applying exponent rules and properties
Recognizing relationships between functions and their graphs

Each of these topics plays a critical role in solving more complex problems. The ability to work through them step by step not only strengthens problem-solving skills but also helps build a logical approach to addressing challenges in various areas of mathematics.

Focusing on these key concepts will not only improve accuracy but also help develop a clearer understanding of how different areas of mathematics are connected, preparing you for more advanced material and applications.

Importance of Practice Exams in Learning

Engaging in mock assessments plays a crucial role in reinforcing learning and preparing for real-world challenges. These exercises allow students to test their understanding, identify weak areas, and build confidence. By simulating actual testing conditions, learners become more familiar with the format, expectations, and time management necessary for success.

Strengthening Retention Through Repetition

Repetition helps solidify concepts and techniques in long-term memory. When students work through multiple questions on similar topics, they reinforce their understanding and increase retention. This iterative process aids in transforming theoretical knowledge into practical skills.

Boosting Confidence and Reducing Anxiety

Frequent exposure to mock problems can significantly reduce stress levels before important assessments. By practicing in a low-pressure setting, students become more comfortable with the material and the testing format, making them less anxious and more prepared for actual performance.

Increased familiarity with common problem types and test-taking strategies makes the learning experience more effective, ultimately leading to improved results in any formal evaluation.

Importance of Practice Exams in Learning

Strengthening Retention Through Repetition

Boosting Confidence and Reducing Anxiety

Increased familiarity with common problem types and test-taking strategies makes the learning experience more effective, ultimately leading to improved results in any formal evaluation.

How to Approach Your Algebra 1 Exam

When preparing for a significant evaluation, having a clear strategy is essential for success. Knowing how to effectively manage your time, review concepts, and approach each problem will help reduce stress and improve your performance. Proper preparation involves more than just knowing the material; it also includes being mentally and physically ready for the test.

One of the most effective methods is to break the time into manageable sections, allowing you to focus on specific areas without feeling overwhelmed. The following table provides a suggested approach to organize your preparation:

Time Period	Focus Area	Action Steps
1-2 Weeks Before	Review Key Concepts	Identify strengths and weaknesses. Focus on core areas like equations, inequalities, and graphing.
1 Week Before	Practice Problem Solving	Work through practice problems and timed exercises. Focus on applying techniques under time constraints.
1-2 Days Before	Final Review	Go over any remaining areas of uncertainty. Review any notes, mistakes from practice sessions, and important formulas.
Day of the Test	Test-Taking Strategies	Read instructions carefully. Manage your time well, and tackle easier problems first to build confidence.

By following this structured approach, you can maximize your chances of performing well. Focusing on targeted practice, time management, and a calm mindset will help you approach the assessment confidently and efficiently.

Mastering Equations and Inequalities

To succeed in solving mathematical problems, a strong understanding of equations and inequalities is essential. These concepts form the backbone of problem-solving skills and are used across various problem types. Mastering the techniques to manipulate variables, isolate unknowns, and graph solutions will give you a solid foundation for tackling more complex challenges.

Key strategies to focus on when working with these concepts include:

Identifying the type of equation or inequality you’re dealing with (linear, quadratic, etc.)
Using proper techniques to isolate the variable, such as adding, subtracting, multiplying, or dividing both sides
Understanding how to deal with inequalities, including flipping signs when multiplying or dividing by negative numbers
Graphing solutions on a number line or coordinate plane for visual clarity
Checking solutions by substituting values back into the original equation or inequality

By practicing these methods consistently, you’ll develop a deeper understanding of how to approach a wide variety of equations and inequalities. Whether you’re solving simple linear equations or more complex systems, these foundational skills will make the process more manageable and efficient.

Solving Systems of Linear Equations

When dealing with multiple variables, solving systems of linear equations allows you to find values that satisfy all given equations simultaneously. This method is essential in various fields, from business to engineering, where multiple constraints must be considered at once. There are several methods available for solving these systems, each suited to different types of problems.

Common techniques include:

Substitution Method: Solve one equation for one variable and substitute that expression into the other equation.
Elimination Method: Add or subtract equations to eliminate one variable, simplifying the system to a single equation.
Graphical Method: Plot both equations on a coordinate plane and identify the point where they intersect, which represents the solution.

Mastering these methods requires practice and a clear understanding of how each technique works. By focusing on the relationships between variables and using the appropriate method, solving systems of equations becomes a systematic and manageable process.

Working with Polynomials and Factoring

Understanding how to manipulate expressions involving multiple terms is a crucial skill in mathematics. By breaking down complex expressions into simpler components, you can solve problems more efficiently and uncover deeper insights. Factoring is a key tool for simplifying and solving polynomial expressions, making it easier to find solutions to a variety of equations.

Expanding and Simplifying Polynomials

Before factoring, it’s important to be able to expand and simplify polynomial expressions. This involves applying distributive properties and combining like terms. Mastering this skill allows you to rewrite polynomials in a more manageable form, preparing them for factoring.

Factoring Techniques

There are several methods for factoring polynomials, each useful for different situations. Some common techniques include:

Factoring by grouping: Group terms in pairs and factor out common factors.
Factoring trinomials: Look for two numbers that multiply to the constant term and add to the middle coefficient.
Difference of squares: Recognize when a polynomial is a difference of two squares and apply the formula.

By practicing these methods, you can quickly break down complicated polynomials into factors, making it easier to solve equations or simplify expressions further.

Tips for Tackling Word Problems

Word problems can often seem daunting, as they require both mathematical understanding and the ability to translate a written scenario into a solvable equation. However, with the right approach, these problems become more manageable. The key is breaking the problem down step-by-step, identifying what information is given, and determining what needs to be found.

Here are some strategies to help approach word problems effectively:

Read Carefully: Understand the problem by reading it more than once. Highlight important details such as numbers, relationships, and keywords like “total,” “difference,” or “rate.”
Define Variables: Assign variables to unknown quantities. This helps to organize your thoughts and creates a clear path toward solving the problem.
Set Up an Equation: Translate the word problem into a mathematical equation or inequality based on the relationships described.
Look for Clues: Words like “sum,” “product,” or “difference” indicate which mathematical operations to use (addition, multiplication, etc.).
Double-Check Your Work: After solving, plug your solution back into the original context to verify that it makes sense.

By systematically following these steps, word problems become less intimidating, and the process of finding a solution becomes clearer and more straightforward.

Understanding Functions and Graphing

Grasping the concept of relationships between variables and how they are represented visually is essential for solving many mathematical problems. A function defines how one quantity depends on another, and graphing helps to visualize this dependency. Understanding how to interpret and manipulate these relationships forms the basis for solving various types of problems efficiently.

The Concept of Functions

In simple terms, a function describes a rule that assigns each input exactly one output. This relationship can be represented in multiple ways, such as equations, tables, or graphs. Being able to identify functions and understand their behavior is critical for tackling more complex problems.

Graphing Techniques

Graphing provides a visual representation of a function’s behavior. Here are key aspects to keep in mind when plotting graphs:

Plotting Points: Start by plotting individual points that satisfy the function. These points are derived from substituting different values of the input variable into the function.
Identifying Intercepts: Determine where the graph crosses the axes. The x-intercept occurs when the output is zero, and the y-intercept is where the input is zero.
Understanding Slope: The slope indicates how the output changes in relation to the input. A positive slope shows an increasing trend, while a negative slope represents a decrease.

By mastering these graphing techniques, you can more easily analyze and interpret the relationships between variables, making complex problems much more approachable.

Exponents and Radicals Simplified

Working with powers and roots may initially seem complicated, but once you understand the basic rules and techniques, they become much more manageable. Simplifying expressions involving exponents and radicals allows you to solve problems more efficiently, especially when dealing with complex expressions. The key is recognizing patterns and applying the appropriate operations to simplify these terms.

Understanding Exponents

algebra 1 practice final exam with answer key

Exponents represent repeated multiplication, and simplifying expressions with exponents follows a set of rules. The following table highlights some important exponent properties:

Rule	Example	Explanation
Product of powers	a^m × aⁿ = a^m+n	Add the exponents when multiplying like bases.
Power of a power	(a^m)ⁿ = a^m×n	Multiply the exponents when raising a power to another power.
Power of a product	(ab)ⁿ = aⁿ × bⁿ	Distribute the exponent across the product of terms.

Understanding Radicals

Radicals, often used to express roots, such as square roots or cube roots, are another important concept. The process of simplifying radical expressions involves factoring the number under the radical symbol and identifying perfect squares or cubes. Here are some helpful tips:

Square Root Simplification: Look for perfect square factors under the radical and simplify accordingly.
Rationalizing Denominators: When a radical appears in the denominator, multiply both the numerator and denominator by an appropriate expression to eliminate the radical.
Combining Radicals: You can combine radicals with the same index (e.g., square roots) by adding or subtracting their coefficients.

By understanding and applying these simplification techniques, you can make working with exponents and radicals much simpler and more efficient.

Quadratic Equations: Key Techniques

Solving quadratic equations is a fundamental skill in mathematics, involving equations where the highest degree of the variable is 2. These equations can often be solved through several different techniques, depending on the specific form of the equation and the information available. Mastering these methods is essential for finding the roots or solutions of quadratic problems efficiently.

Factoring Method

One of the simplest methods for solving quadratic equations is factoring. When the equation is in the standard form ( ax^2 + bx + c = 0 ), factoring allows you to express the quadratic as a product of two binomials. Here’s how to approach it:

Rewrite the quadratic equation in standard form.
Look for two numbers that multiply to give you the constant term (c) and add up to the coefficient of the middle term (b).
Factor the quadratic into two binomials using these numbers.
Set each binomial equal to zero and solve for x.

Quadratic Formula

If factoring is difficult or not possible, the quadratic formula is a reliable method for finding the roots of any quadratic equation. The formula is:

x = (-b ± √(b² – 4ac)) / 2a

This formula works for all quadratic equations and provides both solutions at once. It’s particularly useful when the equation cannot be easily factored or when the discriminant (the expression under the square root) is not a perfect square.

Both factoring and the quadratic formula are valuable tools for solving quadratic equations. By recognizing which method is most efficient for a given problem, you can solve these equations more quickly and accurately.

Quadratic Equations: Key Techniques

Factoring Method

Rewrite the quadratic equation in standard form.
Look for two numbers that multiply to give you the constant term (c) and add up to the coefficient of the middle term (b).
Factor the quadratic into two binomials using these numbers.
Set each binomial equal to zero and solve for x.

Quadratic Formula

If factoring is difficult or not possible, the quadratic formula is a reliable method for finding the roots of any quadratic equation. The formula is:

x = (-b ± √(b² – 4ac)) / 2a

Time Management During the Exam

Effectively managing time during a test is essential to ensure all questions are answered and to reduce stress. Proper time allocation helps you stay focused, prioritize tasks, and avoid rushing through difficult sections. By planning your approach, you can maximize your performance and ensure a thorough completion of the assessment.

One strategy for managing time is to first glance over the entire test, noting the number of questions and their respective point values. This allows you to estimate how much time to spend on each section. If a particular question seems complex, it’s best to move on temporarily and return to it later if time allows. This prevents getting stuck on one problem and losing valuable time for others.

Another useful technique is to set time limits for each section. For instance, you could decide to spend 20 minutes on the first portion and 15 minutes on the second. Keeping track of time during the test ensures that you don’t spend too long on one part, which could compromise your ability to complete the rest of the assessment.

Ultimately, effective time management is a key factor in achieving success. By staying organized, practicing pacing, and remaining calm, you can efficiently navigate the test and ensure a strong performance from start to finish.

How to Use the Answer Key Effectively

Using a solution guide effectively can significantly improve your understanding of complex topics and help you identify areas that need further attention. Instead of just copying the solutions, it’s important to analyze each step and understand the reasoning behind it. This method will allow you to learn from mistakes and reinforce your problem-solving skills.

Steps to Take When Reviewing the Solutions

When going over the provided solutions, start by attempting to solve the problem on your own. After you’ve completed your work, compare your method and result to the guide. If you found any discrepancies, try to identify where you went wrong and focus on understanding the correct approach.

Using the Solution Guide for Practice

Instead of relying on the solution guide right away, consider using it as a reference when you get stuck. This strategy allows you to work through the problem independently, while still having a resource to fall back on if needed. It’s also helpful to revisit the guide after you’ve practiced similar problems to reinforce what you’ve learned.

Steps	Benefits
Attempt problem on your own first	Encourages independent problem-solving and critical thinking
Review the solution guide	Helps to identify mistakes and correct methods
Understand each step of the solution	Strengthens understanding of problem-solving techniques

By using the solution guide in a reflective and methodical way, you’ll not only improve your ability to solve problems but also deepen your overall understanding of the material. This approach ensures that the solution guide serves as an effective learning tool rather than just a shortcut to the correct answer.

Identifying Common Mistakes in Algebra

Recognizing and correcting errors is a crucial step in mastering mathematical concepts. Often, students make similar mistakes, which can hinder progress and lead to misunderstandings of core principles. By identifying these frequent errors, learners can refine their problem-solving techniques and avoid repeating the same missteps.

Common Errors to Watch Out For

Misapplying the Order of Operations: Failing to follow the correct sequence of operations can lead to incorrect results. Always remember to perform operations in the correct order: parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right).
Sign Errors: Incorrectly handling positive and negative signs is a frequent issue. Be especially cautious when subtracting negative numbers or when distributing negative signs across terms.
Forgetting to Simplify: Some problems require simplifying expressions before solving. Forgetting this step can result in incomplete or incorrect answers.
Incorrectly Combining Like Terms: It’s essential to combine terms that have the same variable and exponent. Failing to do so leads to errors in the final solution.
Overlooking the Distributive Property: Not distributing terms properly when simplifying expressions can lead to incorrect results, especially when dealing with parentheses.

How to Avoid These Mistakes

Double-check your work: Before finalizing an answer, review each step to ensure that you followed the correct procedures and didn’t overlook any small details.
Practice consistently: The more you work on problems, the more familiar you’ll become with the common pitfalls and how to avoid them.
Ask for clarification: If a step doesn’t make sense, seek guidance or look for resources to explain the concept in a different way.
Use tools like checklists: When solving problems, create a checklist of steps to follow, ensuring that you don’t miss any important operations or simplifications.

By being aware of these typical mistakes and implementing strategies to avoid them, learners can approach problems more confidently and improve their overall understanding of mathematical concepts.

Boosting Confidence Before the Exam

Approaching an assessment with confidence is crucial to performing well. Often, self-doubt can cloud your judgment and hinder your ability to recall concepts during the test. To ensure you are mentally prepared and confident, it’s important to establish a solid foundation in the material and adopt strategies to calm any nerves before the day of the test.

Effective Techniques to Build Confidence

Review Past Work: Going over previous assignments, quizzes, and tests allows you to identify your strengths and weaknesses. Focus on areas where you have improved and reinforce concepts that still feel uncertain.
Simulate Test Conditions: Try practicing under timed conditions to get used to the pressure. Completing a practice session helps build mental endurance and gives you a better sense of timing during the real test.
Stay Positive: Maintain a positive mindset by visualizing success. Remind yourself of your past achievements and how far you’ve come. A positive outlook can shift your focus from fear to determination.
Stay Organized: Keep your study materials well-organized and make a study schedule. Knowing exactly what to review and when can reduce feelings of being overwhelmed.

How to Manage Stress Effectively

Practice Deep Breathing: Taking slow, deep breaths can help calm nerves and reduce anxiety before the test. Practice this technique regularly to make it easier when you need it the most.
Get Enough Rest: A good night’s sleep before the test is essential for mental clarity and alertness. Avoid cramming the night before and aim for at least 7–8 hours of sleep.
Stay Hydrated and Eat Well: Eating a balanced meal and drinking plenty of water before the test will keep your energy levels high and help you stay focused.

By preparing effectively and adopting strategies to calm your nerves, you can walk into your assessment feeling confident and ready to tackle any challenge that comes your way.

Reviewing Your Final Results

After completing an assessment, it’s important to take the time to review your performance. Analyzing your results helps you understand where you did well and where improvement is needed. This process not only identifies areas of strength but also highlights concepts that may need further attention before moving on to the next stage of learning.

Steps to Effectively Review Your Performance

Review Mistakes Carefully: Focus on the questions you got wrong. Determine whether you misunderstood the concept, made a calculation error, or missed a step in your reasoning. Understanding the root cause of each mistake is key to preventing it in the future.
Analyze Patterns: Are there specific types of problems you struggled with more than others? Identifying patterns in your mistakes can reveal areas where you need additional practice or a deeper understanding.
Seek Clarification: If any errors are unclear, don’t hesitate to ask your instructor or peers for clarification. Sometimes a different explanation can make a complex concept more understandable.

Using the Results to Improve

Focus on Weak Areas: Once you’ve identified your weaknesses, dedicate more study time to those topics. Practice similar problems to build confidence and reinforce your understanding.
Use Available Resources: Utilize textbooks, online tutorials, or study groups to improve your grasp on the material. Sometimes practicing in different ways or seeing problems explained from a new angle can help solidify your knowledge.
Track Progress: Keep track of your improvements over time. Regularly reviewing both past mistakes and new successes will help you track your progress and stay motivated.

By carefully reviewing your results, you not only improve your understanding but also prepare yourself for future challenges. Embrace the learning process, and view every mistake as an opportunity to grow.