Algebra Nation Answer Key Section 5 Solutions and Guide

The fifth segment of this math course provides essential tools and detailed solutions designed to help students grasp complex concepts. Whether you’re struggling with specific problems or looking for ways to strengthen your understanding, this part offers guidance for tackling key challenges. The focus is on honing skills needed to solve difficult equations and navigate through various exercises with confidence.

Step-by-step explanations accompany each solution, offering clear insights into the methods required to reach the correct results. This approach allows learners to develop a deeper comprehension of mathematical principles while improving problem-solving strategies. By following the provided instructions, students can better navigate similar challenges in future lessons.

Utilizing the provided solutions can significantly accelerate progress. With practice and application, mastering the content becomes achievable, setting a strong foundation for more advanced topics. Understanding the steps involved in each problem is crucial for building the skills necessary to excel in mathematics.

Algebra Nation Answer Key Section 5 Overview

This part of the learning material focuses on providing students with a comprehensive set of solutions aimed at enhancing problem-solving skills. It covers essential mathematical topics, offering an in-depth look at how to approach and solve challenging exercises. By breaking down complex problems into understandable steps, this section helps learners strengthen their grasp on key concepts.

What You Will Learn

• Step-by-step solutions for various mathematical exercises.
• Strategies for tackling equations and understanding their structure.
• Methods to improve accuracy and efficiency in solving problems.

Why This Section is Important

• It provides clarity on how to approach different types of problems.
• Each solution is explained in detail, helping students see the process behind the final result.
• This approach ensures a solid foundation for progressing to more advanced topics.

By working through the material, students can not only improve their current understanding but also gain the confidence needed to tackle future challenges in the subject.

Understanding Section 5 Problem Types

This portion of the study material focuses on various types of problems that require different approaches and strategies for effective resolution. The exercises within this segment are designed to test a range of skills, from basic operations to more complex calculations. Understanding the nature of these problems is crucial for developing a systematic method to tackle them efficiently.

The problems are categorized into several types, each with its own unique challenge. Below is an overview of the common types of problems you will encounter:

Problem Type	Description	Example
Linear Equations	Equations involving variables with exponents of one.	2x + 3 = 7
Quadratic Equations	Problems that include variables with exponents of two.	x² – 5x + 6 = 0
Systems of Equations	Multiple equations solved simultaneously.	3x + y = 7 and 2x – y = 3
Word Problems	Real-world scenarios requiring mathematical interpretation.	A farmer has 12 apples, and he buys 8 more. How many apples does he have now?

Each problem type requires specific techniques to solve, and recognizing the pattern is key to success. Once the problem type is identified, you can apply the correct method to find the solution with greater ease and accuracy.

How to Use the Answer Key

To maximize the benefit of the provided solutions, it’s important to understand how to use the resources effectively. This guide will help you navigate the provided explanations and make the most of each example. Rather than simply checking the final answers, it’s essential to focus on the process behind them, as this will enhance your problem-solving skills for future challenges.

Step-by-Step Learning Approach

Focus on the process: When reviewing the provided solutions, pay attention to the steps used to reach the final result. Understand the logic behind each action, as this will allow you to replicate the process in your own work.

Don’t skip over explanations: Even if you can find the final answer quickly, read through the explanation to ensure you understand the reasoning behind it. This deeper understanding is crucial for solving similar problems in the future.

Common Mistakes to Avoid

• Relying only on the final answers without checking the method.
• Skipping over any intermediate steps that seem trivial but may hold important insights.
• Not revisiting problems you find difficult to fully grasp – practice is key to improvement.

By carefully reviewing both the solutions and their corresponding steps, you can deepen your understanding of the material and become more confident in your ability to solve similar problems independently.

Key Concepts Covered in Section 5

This portion of the material focuses on several fundamental principles designed to enhance problem-solving abilities. The topics covered are essential for mastering the subject and progressing to more advanced concepts. By understanding and applying these principles, students will develop the skills needed to tackle more complex challenges with confidence.

Core Topics Explored

• Understanding the relationship between variables and constants in mathematical expressions.
• Working with different types of equations, including linear and quadratic forms.
• Mastering the art of simplifying and solving expressions involving multiple terms.
• Strategies for manipulating equations and finding unknown values.

Skills Developed

• Improving the ability to recognize patterns and apply appropriate techniques.
• Enhancing critical thinking through the application of step-by-step methods.
• Building a deeper comprehension of mathematical relationships and operations.

By focusing on these core concepts, students will gain a solid foundation for advancing in the subject, improving both their skills and confidence in solving a wide variety of problems.

Common Mistakes in Algebra Section 5

When working through complex mathematical exercises, it’s easy to make small errors that can have a significant impact on the final result. Recognizing these common mistakes can help improve accuracy and avoid frustration. In this section, we’ll explore some of the most frequent missteps students make and provide tips for correcting them.

Frequently Made Errors

Error	Explanation	How to Avoid It
Misapplying Operations	Confusing addition and subtraction with multiplication and division.	Double-check the order of operations and ensure correct application.
Skipping Steps	Omitting intermediate steps, leading to inaccurate conclusions.	Always write out each step to avoid skipping important parts.
Overlooking Negative Signs	Forgetting to account for negative signs during calculations.	Pay close attention to negative numbers, especially when distributing or factoring.
Incorrectly Simplifying Expressions	Incorrectly combining like terms or simplifying too early.	Carefully check each term and simplify step by step.

How to Overcome These Challenges

• Focus on understanding the logic behind each operation rather than rushing through problems.
• Work through example problems slowly and make sure each step is understood before moving on.
• Use visual aids like diagrams or checklists to keep track of each calculation.

By being aware of these common mistakes and taking the time to correct them, you’ll improve your problem-solving skills and gain greater confidence in your ability to handle more complex topics.

Step-by-Step Problem Solving Approach

Solving mathematical problems can often seem overwhelming, but by breaking them down into manageable steps, the process becomes more systematic and less intimidating. Adopting a clear, step-by-step approach allows you to tackle each problem with confidence and ensures you don’t miss any important details. This method not only helps in reaching the correct solution but also improves overall understanding of the material.

Essential Steps for Solving Problems

1. Read the Problem Carefully: Understand exactly what is being asked before attempting any calculations. Identify the given information and what you need to find.
2. Plan Your Approach: Determine which techniques or methods are required based on the problem type. Whether it’s simplifying, factoring, or solving an equation, have a strategy in mind.
3. Perform Calculations: Work through the problem step by step. Always check your calculations to ensure accuracy at each stage.
4. Double Check Your Work: Once you’ve arrived at a solution, go over your steps again to make sure no mistakes were made along the way. Verify that the answer makes sense in the context of the problem.
5. Final Answer: Once confident that all steps have been followed correctly, write down the final result clearly.

Helpful Tips for Success

• Always take the time to write out every step–don’t skip ahead, even if the solution seems obvious.
• Use diagrams or charts if they help to visualize the problem more clearly.
• If stuck, revisit previous examples or similar problems to refresh your approach.

By following this methodical approach, students can not only solve individual problems with greater ease but also build a strong foundation for tackling more advanced topics in the future.

Tips for Improving Algebra Skills

Enhancing your mathematical abilities requires a mix of practice, strategy, and understanding. By focusing on key areas and applying consistent effort, you can strengthen your problem-solving techniques and gain more confidence in handling complex tasks. Below are several practical tips that can guide you in improving your overall skill set and performance.

Effective Study Habits

• Practice Regularly: Consistent practice is one of the most effective ways to improve. Set aside time each day to work on problems, even if it’s just for a short period.
• Review Mistakes: Instead of moving past errors, take the time to understand why a mistake was made and how to avoid it in the future.
• Break Down Complex Problems: Large or complicated problems can be overwhelming. Break them into smaller, more manageable steps to make the solution clearer.

Building Conceptual Understanding

• Focus on Fundamentals: Make sure you have a strong grasp of basic concepts, as they serve as the foundation for solving more difficult problems.
• Use Visual Aids: Diagrams, graphs, and charts can help visualize problems and make abstract concepts more tangible.
• Learn from Examples: Study worked-out examples to understand the methods and reasoning behind each solution. Try solving similar problems on your own to reinforce your understanding.

Improving your skills is an ongoing process, but with dedication and the right strategies, you can progressively become more proficient. Don’t hesitate to seek out additional resources or assistance when needed, as continuous learning is key to mastering the subject.

Practice Problems for Section 5

Practicing with a variety of problems is an essential part of mastering any mathematical concept. The following exercises are designed to reinforce the key skills and techniques introduced in this portion of the material. By tackling these problems, you can improve both your understanding and speed in solving similar tasks.

Basic Practice Problems

• Solve for x: 3x + 5 = 20
• Simplify the expression: 2(4x – 3) + 7x
• Find the value of y when 5y – 9 = 16
• Factor the equation: x² + 5x + 6

Advanced Practice Problems

• Solve the system of equations:
  • x + y = 7
  • 2x – y = 3
• Simplify the expression: (3x + 2)(x – 4) – x²
• Find the roots of the quadratic equation: x² – 4x + 3 = 0
• Graph the equation: y = 2x + 3

By practicing these problems, you can reinforce your problem-solving abilities and develop a deeper understanding of the concepts covered. Be sure to revisit any problems you find difficult, as repeated practice will help solidify your knowledge and improve your overall performance.

Understanding Algebraic Equations Better

Grasping the fundamentals of mathematical equations is essential for solving more complex problems. By understanding the structure and components of an equation, you can learn to approach solutions more confidently and efficiently. This section will explore the key concepts behind equations and provide tips for improving your ability to work with them.

Breaking Down an Equation

At its core, an equation is a statement of equality between two expressions. The challenge lies in finding the value of the unknown variable that makes both sides of the equation equal. Here are some steps to help you understand and solve equations more effectively:

• Identify the variable: The unknown value in the equation is typically represented by a letter such as x or y.
• Simplify both sides: Combine like terms and perform any basic operations (addition, subtraction, multiplication, division) to simplify the expressions on both sides of the equation.
• Isolate the variable: Use inverse operations to move terms around and get the variable by itself on one side of the equation.
• Check your solution: Once you’ve solved for the variable, substitute it back into the original equation to verify that both sides are equal.

Common Types of Equations

• Linear Equations: These equations involve the first power of the variable and are often in the form of ax + b = c.
• Quadratic Equations: These equations involve the square of the variable and typically appear in the form of ax² + bx + c = 0.
• Rational Equations: These equations include fractions where the variable is present in the denominator.
• Exponential Equations: In these, the variable appears as an exponent, such as in expressions like a^x = b.

With practice and a solid understanding of the underlying principles, you can gain greater confidence in solving equations and apply these skills to more advanced mathematical topics.

How to Check Your Answers

Verifying your solutions is a crucial part of problem-solving. It helps ensure that the work you’ve done is correct and that you’ve followed the right steps. By double-checking your calculations and reasoning, you can catch any potential errors and strengthen your understanding of the concepts.

Steps to Verify Your Solutions

• Substitute Back into the Original Problem: The most reliable way to check your solution is to substitute the value you found back into the original equation. If both sides are equal, your solution is correct.
• Review Your Work: Go through each step carefully to ensure that you haven’t made any mistakes in your operations. Pay close attention to signs, coefficients, and variables.
• Check for Consistency: Compare your result with any related problems or example solutions. If your answer is significantly different from expected patterns, rework the problem.
• Use Estimation: For some problems, especially when dealing with large numbers or complex expressions, estimate the solution beforehand. This can give you a rough idea of what the answer should be, making it easier to spot mistakes.

Additional Tips for Accurate Checking

• Work in Stages: Break down the problem into smaller sections and verify each part before moving on to the next. This reduces the likelihood of making errors in long calculations.
• Utilize Tools: For more complex problems, consider using calculators, graphing tools, or online checkers to verify numerical solutions or graphical results.
• Cross-check with Peers: If you’re unsure about your solution, ask a classmate or tutor to check your work. Sometimes a fresh perspective can identify overlooked errors.

By regularly checking your work, you’ll not only improve your accuracy but also develop a deeper understanding of the problem-solving process, which is essential for mastering more advanced topics.

Strategies for Time Management in Algebra

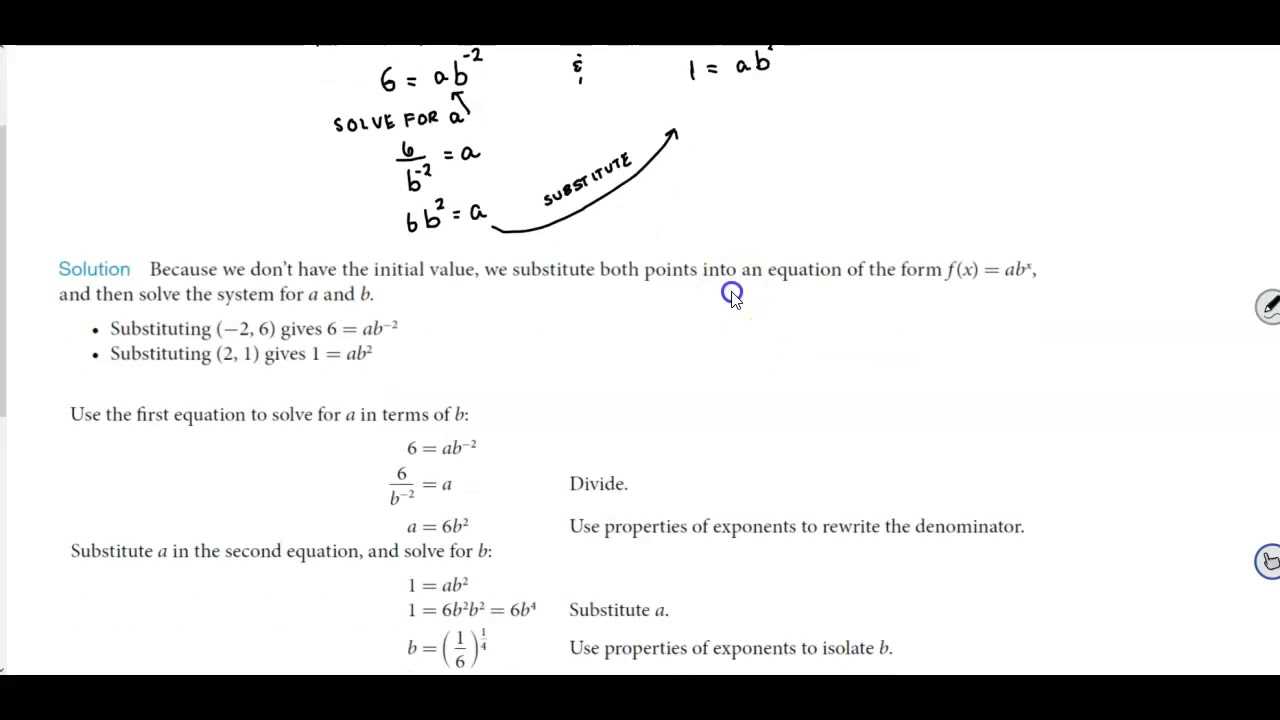
Effective time management is essential for success in mathematics, especially when tackling complex problems. Properly managing your time not only ensures you complete tasks on time but also helps you approach problems with a clearer mind. By applying a few strategic methods, you can increase your efficiency and improve your overall performance in problem-solving exercises.

One key aspect of time management is breaking down tasks into smaller, manageable parts. Start by reviewing the problem and identifying the steps involved. Prioritize the most important steps and allocate sufficient time to each part. Avoid rushing through calculations; taking your time at each stage ensures that you won’t make careless mistakes.

Another helpful strategy is practicing timed exercises. This helps you get a feel for how long you should spend on each problem and improves your ability to work efficiently under pressure. By simulating real test conditions, you can build confidence in your ability to manage time during assessments.

Additionally, setting clear goals and deadlines can help you stay on track. For example, aim to solve a certain number of problems within a set time frame or master specific concepts by a particular date. Regularly reviewing your progress and adjusting your schedule as needed will ensure you stay focused and make steady progress.

Lastly, avoid multitasking. While it might seem like a good idea, jumping between different problems can waste time and lead to confusion. Instead, focus on one problem at a time, giving it your full attention before moving on to the next one. This approach not only improves your efficiency but also ensures that you fully understand each concept before progressing.

How to Prepare for Tests

Preparation is crucial when it comes to performing well in mathematical assessments. The key to success lies in understanding the core concepts, practicing problems, and managing your time effectively. By adopting a structured approach, you can reduce test anxiety and approach each question with confidence.

Review Key Concepts and Techniques

Before diving into practice problems, it’s essential to review the foundational concepts. Revisit any formulas, rules, or strategies that are frequently used in the problems you’ll face. Make sure you understand the logic behind each method and not just the steps involved. This deeper understanding will help you solve problems more effectively during the test.

Practice with Sample Problems

Solving sample questions is one of the most effective ways to prepare. By working through various problems, you become familiar with the different types of questions and learn to apply the concepts you’ve reviewed. Start with easier problems to build confidence and gradually work up to more challenging ones. Additionally, try to time yourself to simulate test conditions.

It’s also helpful to review previous tests or exercises, if available. This gives you insight into the types of problems commonly asked and any recurring patterns in question format. Understanding what to expect can reduce surprises and make you feel more prepared.

Stay Organized and Plan Ahead

Don’t wait until the last minute to start studying. Set aside regular time for review, ensuring you cover all necessary material well in advance. Create a study schedule that includes breaks and focuses on different topics each day. Consistent preparation over time is more effective than cramming all at once.

Additionally, avoid distractions during study sessions. Turn off your phone, find a quiet space, and focus solely on the material at hand. This focused effort will help you retain information more effectively and increase your chances of success.

Learning from Solution Explanations

One of the most valuable resources in mastering mathematical problems is the explanation provided alongside the solutions. Instead of just looking at the final answer, understanding the steps involved helps reinforce the problem-solving process and ensures that the method is clear. Analyzing these detailed solutions allows you to see where you might have gone wrong and how to correct mistakes in the future.

Why Solution Explanations Matter

Solution explanations offer more than just the correct result. They show you the thought process behind each step, which is essential for improving your problem-solving skills. By carefully reviewing each stage of a solution, you can gain insight into different techniques that you might not have considered. This knowledge helps to deepen your understanding of the topic and apply it to similar problems in the future.

Steps for Effective Learning from Explanations

• Read Each Step Carefully: Pay attention to every part of the solution, especially the logic behind the steps.
• Identify Key Concepts: Take note of the strategies or rules used and how they are applied in the solution.
• Compare Your Approach: Reflect on how your method differs from the one shown and understand why certain steps work better.
• Practice Similar Problems: After reviewing the solution, try solving similar problems to reinforce the technique.
• Ask Questions: If something is unclear, ask for clarification or review the theory behind the method used in the solution.

By actively engaging with solution explanations, you can improve your approach to problem-solving, develop a stronger grasp of mathematical concepts, and increase your confidence when tackling future problems.

Mastering Mathematical Techniques Efficiently

Mastering complex mathematical techniques requires focused practice and a strategic approach. Understanding the core principles and learning to apply them in various scenarios is key to improving problem-solving skills. By breaking down the process into manageable steps and practicing consistently, you can enhance your proficiency and tackle more challenging problems with confidence.

Efficiency in learning comes from not just memorizing formulas, but understanding when and why to use them. Practice with varied examples and seek to refine your approach after each mistake. Over time, the goal is to reduce the time spent on problem-solving by recognizing patterns and applying the most effective strategies quickly.

Techniques for Effective Mastery

Here are some key techniques to help you master mathematical concepts efficiently:

Technique	Description
Practice Consistently	Regular practice with different problem types reinforces understanding and builds speed.
Understand Concepts	Rather than memorizing, focus on fully grasping the underlying ideas and their applications.
Identify Patterns	Recognizing recurring structures in problems can save time and guide you to the solution faster.
Work in Small Steps	Break down complicated problems into smaller, more manageable parts to avoid overwhelm.
Learn from Mistakes	Review incorrect solutions to understand where mistakes occurred and avoid repeating them.

By focusing on these strategies and staying consistent in your efforts, you will be able to master the techniques required for success in mathematical problem-solving more efficiently and effectively.

What to Focus on in Section 5

To effectively tackle the material covered in this part of the course, it’s important to understand the key concepts and strategies needed to solve the problems. Focus on the areas that challenge your current understanding and strengthen your grasp of the fundamental techniques. With the right approach, you can build confidence and improve your problem-solving abilities.

Key topics in this section often require you to apply a combination of techniques, such as identifying patterns, simplifying expressions, and carefully analyzing each problem step-by-step. Pay close attention to the common problem types presented and practice solving them with increasing complexity. This will help you identify patterns and approaches that are reusable in various scenarios.

To maximize your learning, it’s essential to break down each problem into manageable parts and focus on one concept at a time. This focused approach will allow you to deepen your understanding and make connections between different ideas, improving your overall skill set.

How Answer Keys Help in Learning

Using solutions to problems plays a crucial role in enhancing your understanding of complex topics. They provide a roadmap for how to approach each problem and ensure you can follow the correct process. By reviewing solutions, you not only check the accuracy of your work but also gain insight into more efficient or alternative methods of solving similar challenges.

One of the primary benefits of having access to detailed explanations is the opportunity to learn from mistakes. Understanding why a particular approach doesn’t work allows you to identify areas where your knowledge might be lacking, and helps reinforce the correct methods. By analyzing each step in the solution process, you can gain a deeper understanding of the underlying principles at play.

Benefits of Reviewing Solutions

• Clarifying Misunderstandings: Solutions highlight areas where you may have gone wrong, allowing for better understanding of the correct approach.
• Reinforcing Techniques: By seeing multiple methods to solve a problem, you expand your toolbox of strategies.
• Identifying Patterns: Solutions can help reveal patterns and tricks that can be applied to a variety of problems.

Effective Use of Solutions for Practice

When using solutions as a learning tool, it’s important not to just memorize the answers. Instead, focus on the reasoning behind each step and attempt to apply these techniques independently. This active approach fosters better retention and enhances problem-solving skills in the long term.

Exploring Algebra Nation Resources

Educational platforms often offer a wide array of tools that help students deepen their understanding and strengthen their problem-solving skills. These resources typically include guided lessons, practice problems, and explanations that can assist learners at various stages of their studies. By utilizing these materials effectively, students can build confidence and mastery in their subject area.

One of the key features of such platforms is the variety of content available, catering to different learning styles. Whether you prefer step-by-step walkthroughs, video tutorials, or practice exercises, these resources allow you to tailor your study approach to your individual needs. The accessibility of these materials ensures that learners can review concepts at their own pace, revisiting any areas that need improvement.

Types of Educational Resources Available

• Interactive Lessons: These often include detailed instructions, visual aids, and exercises that provide a hands-on learning experience.
• Practice Exercises: A wide range of problems help solidify understanding and test knowledge on various topics.
• Explanatory Videos: These videos break down complex concepts into digestible segments, often including examples and tips for solving problems efficiently.

Maximizing the Benefits of Online Resources

To get the most out of these platforms, it’s important to actively engage with the materials. Instead of passively reading or watching, take notes, solve problems, and attempt to teach the concepts to others. This active involvement strengthens comprehension and enhances retention of information.

Improving Problem Solving Confidence

Building confidence in tackling challenges requires consistent practice and understanding of the concepts involved. One of the most effective ways to enhance your problem-solving skills is through active engagement with a variety of exercises, allowing you to gradually increase your proficiency. As you encounter and solve more problems, your ability to approach unfamiliar questions with confidence improves, making it easier to handle more complex tasks in the future.

It’s essential to embrace the learning process and accept mistakes as part of growth. Each error offers valuable insight into areas that need more attention, providing opportunities to refine your approach. By reviewing solutions, identifying mistakes, and adjusting your methods, you can deepen your understanding and become more adept at solving similar problems moving forward.

Strategies for Boosting Confidence

• Start with Simpler Problems: Begin with exercises that you are comfortable with, gradually working your way up to more challenging ones.
• Track Your Progress: Keep a record of the problems you’ve solved, noting improvements and areas that require more focus.
• Understand the “Why”: Instead of just memorizing steps, ensure that you understand the reasoning behind each solution, which will give you more flexibility when faced with new types of problems.

Overcoming Mental Blocks

Sometimes, the hardest part is getting started. Mental blocks can arise when facing a particularly difficult problem or when you feel unsure about your abilities. To overcome these obstacles, try taking short breaks, approaching the problem from a different angle, or discussing it with someone else. This can help reset your thinking and provide fresh perspectives, ultimately boosting your confidence in tackling the next challenge.