Algebra with Pizzazz Answer Key for Page 149

In the world of mathematics, solving complex problems can often be challenging, but with the right guidance, these tasks become much more manageable. Providing step-by-step solutions helps students gain confidence in their problem-solving abilities, allowing them to tackle even the most difficult questions. By offering detailed explanations, learners are able to follow logical processes and understand the reasoning behind each answer.

Mastering mathematical concepts involves breaking down complicated exercises into simple, understandable steps. The process of reviewing solutions not only reinforces learning but also improves critical thinking skills. With clear examples and easy-to-follow methods, students can apply the learned techniques to new problems with greater ease and accuracy.

Whether you’re revisiting previous lessons or working on fresh challenges, having a structured approach to math exercises is essential for long-term success. With the right resources at hand, students can develop a solid foundation that supports their academic growth and builds essential problem-solving skills.

Answer Solution Overview

This section provides a comprehensive look at the solutions provided for a set of mathematical problems. It serves as a guide to help students understand how each step of the problem is solved, ensuring a clear path from start to finish. By breaking down each solution, learners can grasp the underlying principles and apply similar methods to new challenges.

How the Solutions Are Organized

The solutions are presented in a structured format, starting from basic concepts and progressing to more complex steps. This approach allows students to gradually build their understanding, ensuring they can follow the logical flow of each problem. The answers are accompanied by detailed explanations to clarify each stage of the process, emphasizing the importance of correct reasoning and methodology.

Benefits of Using Detailed Solutions

Using these solutions not only enhances comprehension but also improves critical thinking and problem-solving abilities. By reviewing each step, students can better identify where mistakes might occur and learn from them, ultimately boosting their confidence in handling similar tasks independently in the future.

Step	Explanation	Outcome
Step 1	Identify the given information and understand the problem context.	Clear understanding of the task to be completed.
Step 2	Break the problem into smaller, manageable parts.	Organized approach for easier solving.
Step 3	Apply mathematical operations to each part logically.	Accurate results based on the operations performed.

Understanding the Importance of Answer Keys

Access to correct solutions plays a crucial role in the learning process, helping students identify mistakes and comprehend the correct approach to problem-solving. These resources not only provide the right answers but also offer valuable insights into the methodology behind each step. By reviewing solutions, learners can better understand how to tackle similar challenges and develop stronger problem-solving skills.

Having a reliable resource to compare results is essential for reinforcing concepts and building confidence. When students know how to verify their work, they gain a deeper understanding of the material and are more likely to retain the knowledge. The ability to independently check one’s progress fosters both self-reliance and a clearer grasp of how different methods are applied to solve problems.

How Algebra with Pizzazz Simplifies Learning

This resource is designed to make the process of learning mathematics more accessible and engaging. It breaks down complex concepts into manageable sections, using clear explanations and creative examples that help students grasp challenging topics. By incorporating visual aids and interactive elements, it encourages learners to actively engage with the material, making the learning experience both enjoyable and effective.

Key Features of the Resource

• Clear, step-by-step instructions for each problem.
• Illustrative examples that simplify complex ideas.
• Interactive exercises that keep students engaged.
• Gradual difficulty progression to build confidence.

How It Enhances Student Understanding

This resource makes difficult topics easier to understand by presenting them in a format that students can follow at their own pace. The clear structure helps to demystify the subject and encourages learners to think critically about each problem. The addition of fun, creative exercises also allows students to apply what they’ve learned in a hands-on way, reinforcing their understanding and keeping them motivated throughout the process.

Step-by-Step Solutions for Page 149

Breaking down complex exercises into manageable steps is essential for mastering any topic. By following a clear sequence of actions, learners can navigate through the process and build a deeper understanding of each concept. Each step serves as a building block, helping students connect theory with practice and strengthening their problem-solving skills along the way.

These solutions are structured to guide learners through every stage of the task, ensuring they comprehend not only the “how” but also the “why” behind each step. This approach allows for a more thorough grasp of the material, making it easier to apply the learned techniques to new and more challenging problems.

Key Mathematical Concepts Covered in Page 149

This section introduces several fundamental mathematical principles that are essential for solving a range of problems. The exercises focus on core techniques, helping learners deepen their understanding and apply these methods to new situations. By reinforcing key concepts, students can improve their ability to approach complex tasks confidently and accurately.

Core Techniques Explained

The section highlights critical methods for manipulating equations, identifying patterns, and applying operations effectively. These skills are foundational in progressing toward more advanced topics, offering a strong basis for further study in mathematics.

Building a Strong Foundation

As learners work through the material, they gain proficiency in managing numbers, variables, and expressions. By mastering these basic concepts, students can approach future challenges with the confidence to solve problems using logical steps and sound reasoning.

Common Mistakes in Mathematical Problems

When working through mathematical problems, many learners encounter recurring errors that can hinder their progress. These mistakes often stem from misunderstandings of concepts, improper application of rules, or simple calculation errors. Identifying and addressing these issues early can prevent frustration and improve accuracy in solving problems.

Misunderstanding Key Concepts

One of the most common issues is a lack of understanding of core principles. Whether it’s incorrectly applying the order of operations or failing to recognize the relationship between different variables, these misunderstandings can lead to incorrect results. Reviewing fundamental concepts thoroughly can help avoid this mistake and build a stronger foundation for tackling more complex problems.

Simple Calculation Errors

Even when the correct method is followed, small mistakes in arithmetic can lead to incorrect answers. These errors may include misplacing decimal points, adding or subtracting incorrectly, or making simple typographical mistakes when transferring numbers. Double-checking each calculation can help catch these issues and ensure more reliable results.

Why Use This Learning Resource?

This educational tool is designed to make the learning process more engaging and effective. By providing a structured approach to mathematical exercises, it encourages students to actively participate in solving problems while developing essential skills. The resource not only simplifies complex topics but also makes learning fun, which can significantly enhance a student’s motivation and confidence.

One of the key reasons to use this resource is its ability to break down intricate concepts into smaller, more manageable parts. This makes it easier for learners to understand and apply mathematical principles step by step. Additionally, the inclusion of interactive examples and clear explanations ensures that students stay engaged and retain the material more effectively.

Efficiently Navigating the Solution Guide

To get the most out of a solution guide, it’s essential to approach it with a clear strategy. Knowing where to look for specific solutions and understanding how to follow the steps will save time and reduce frustration. A well-organized resource allows learners to quickly reference the correct methods and results, ensuring they stay focused and efficient throughout their study sessions.

The key to efficient navigation is familiarity with the structure of the guide. Most solution resources are organized in a way that helps students find relevant information quickly. By focusing on the steps and explanations provided, learners can easily pinpoint where they might have gone wrong and make adjustments without wasting time searching for answers.

Detailed Breakdown of Problem Solving

Effective problem-solving requires a step-by-step approach that breaks down complex tasks into manageable parts. By following a structured process, learners can address each component of the problem systematically. This method not only makes solving easier but also ensures that each stage is thoroughly understood before moving on to the next one.

Step-by-Step Approach

The first step is always to read the problem carefully and understand what is being asked. Next, identify the relevant information and organize it in a way that makes it easier to manipulate. After that, apply the appropriate methods to solve the problem, checking for accuracy as each step is completed. Finally, review the solution to ensure that it answers the question fully and logically.

Key Strategies for Success

• Clarify the Goal: Understand exactly what the problem requires before beginning.
• Break It Down: Divide the problem into smaller, more manageable parts.
• Check Your Work: Ensure each step is completed correctly before moving forward.
• Review the Final Solution: Double-check the result to confirm it makes sense.

Mathematical Techniques Explained in Page 149

This section covers essential methods for solving mathematical problems, focusing on the most commonly used techniques to simplify complex tasks. These strategies are designed to guide students through various operations and help them develop the skills needed to approach new problems with confidence. By mastering these methods, learners can enhance their problem-solving abilities and build a solid foundation for more advanced concepts.

Each technique is explained step by step, allowing learners to follow a logical progression from start to finish. Whether it’s simplifying expressions, solving equations, or factoring, the resource ensures that students understand not only the “how” but also the “why” behind each process. This comprehensive approach makes it easier to retain information and apply it in future challenges.

Key Methods Covered

• Solving Linear Equations: A systematic approach to isolating variables.
• Factoring Quadratic Expressions: Breaking down complex polynomials into simpler factors.
• Simplifying Rational Expressions: Techniques for reducing fractions and simplifying terms.
• Identifying Patterns: Using patterns to solve problems faster and more accurately.

Benefits of Using a Solution Guide

Using a solution guide can significantly enhance the learning process, offering students a clear path to understanding complex concepts. It serves as a helpful reference tool, providing both answers and explanations that help learners grasp the correct methods for solving problems. By using this resource, students can quickly identify mistakes, understand the logic behind each solution, and reinforce their learning.

One of the major advantages of relying on a solution guide is that it promotes self-assessment and independent learning. With access to the correct answers and step-by-step explanations, students can check their work, identify areas for improvement, and apply the correct approach moving forward. This fosters a sense of confidence and mastery over the material.

Key Benefits

• Instant Feedback: Students can quickly verify their answers and identify errors.
• Clear Explanations: Detailed breakdowns of problem-solving methods help deepen understanding.
• Encourages Self-Study: Learners can work independently, boosting problem-solving skills.
• Reinforces Concepts: Repeated exposure to correct methods strengthens retention.

Understanding the Solution Guide Format

The format of a solution guide is designed to help learners easily navigate through the material and understand the problem-solving process. It typically provides a structured layout, ensuring that each solution is presented in a clear, step-by-step manner. By following this format, students can gain a deeper understanding of how each problem is approached and solved, while also identifying the key strategies used in the process.

One of the main elements of a well-structured solution guide is consistency. The problems are typically numbered or listed in order, with each corresponding solution following the same layout. This consistency makes it easy for learners to find answers and explanations quickly, whether they are reviewing a specific problem or revisiting the material for further practice.

Real-World Applications of Mathematics

Mathematical principles are not just theoretical concepts; they have practical applications in a variety of fields. Understanding how these concepts work in real-world scenarios can enhance problem-solving skills and open doors to career opportunities. From budgeting personal finances to designing complex structures, mathematical techniques are crucial for tackling everyday challenges and driving innovation across industries.

In particular, mathematical methods are used extensively in engineering, economics, technology, and even healthcare. By applying these principles, professionals can make informed decisions, optimize processes, and solve complex problems in an efficient and effective way. Students who grasp these concepts early on are better equipped to use them in their academic and professional careers.

Common Uses in Various Fields

Industry	Application	Examples
Engineering	Designing and building structures	Calculating load-bearing capacity, material strength
Economics	Financial modeling and analysis	Budgeting, investment calculations, market predictions
Technology	Software development, data analysis	Algorithms, encryption, machine learning
Healthcare	Medical diagnostics and treatments	Analyzing data for patient care, medical imaging

Impact of Mathematics on Innovation

As technology continues to advance, the role of mathematical principles becomes even more critical. Professionals in cutting-edge fields like artificial intelligence and quantum computing rely heavily on these methods to drive progress and develop new solutions. By learning how to apply these concepts, individuals can contribute to breakthroughs that shape the future of society and the economy.

Solving Complex Problems with Confidence

Tackling challenging problems can often seem intimidating, but breaking them down into smaller, manageable steps can make the process much more approachable. Gaining confidence in solving difficult tasks comes from understanding the core principles and practicing problem-solving techniques regularly. By mastering the fundamentals, individuals can approach even the most intricate issues with a structured mindset, leading to effective solutions.

It’s important to stay focused and systematic when faced with complexity. One key approach is to carefully analyze the problem, identify known variables, and then use logical reasoning to determine the best path forward. With each successful solution, confidence builds, reinforcing the ability to take on more complex challenges in the future. This method not only strengthens problem-solving skills but also encourages a positive mindset toward learning and growth.

Learning from Mistakes in Exercises

Errors are an inevitable part of the learning process, and they play a vital role in developing a deeper understanding of mathematical concepts. Instead of viewing mistakes as setbacks, they should be embraced as opportunities for growth. Recognizing where things went wrong and understanding why an approach was incorrect can lead to more effective problem-solving strategies in the future.

Common Mistakes and Their Lessons

When working through problems, some errors occur more frequently than others. Understanding these common mistakes can help learners avoid them in the future and reinforce the correct methods. Below are a few typical errors:

• Misinterpreting the Problem: Often, misreading a question can lead to incorrect steps. Carefully reviewing the problem before starting can help ensure clarity.
• Incorrect Operations: Simple arithmetic errors can change the entire outcome. Double-checking calculations is crucial to avoid this mistake.
• Skipping Steps: Jumping ahead too quickly can result in missed steps or faulty reasoning. It’s important to break down each problem into manageable parts.

How to Turn Mistakes Into Learning Opportunities

Rather than just correcting an error, it’s valuable to spend time understanding why the mistake happened. This reflective practice helps in building a stronger foundation for tackling future challenges. Below are some strategies for learning from mistakes:

1. Review the Solution: Go over each step to identify where the problem arose. Was it a misunderstanding of the concept or a simple calculation error?
2. Ask for Help: If you’re struggling to identify the mistake, discussing it with a peer, teacher, or tutor can provide new insights.
3. Practice Similar Problems: After correcting a mistake, try similar problems to ensure you’ve fully understood the process and can apply the correct approach.

Ultimately, the key to mastering any skill, especially in mathematics, is persistence and learning from past errors. Each mistake is a stepping stone toward improvement, and embracing them is essential for success in more complex exercises.

Tips for Mastering Mathematical Exercises

Mastering any set of mathematical exercises requires a strategic approach, focused practice, and a deep understanding of the underlying concepts. It’s important to work through problems step by step, remain patient, and utilize effective methods to improve accuracy and speed. Whether you’re just starting or working through advanced problems, the following tips will help you excel in solving complex tasks with confidence.

Focus on Understanding, Not Just Memorizing

One of the biggest mistakes in learning mathematics is relying solely on memorizing formulas and techniques without truly understanding how they work. To build a solid foundation, focus on grasping the reasoning behind each concept:

• Comprehend the logic: Before diving into solving problems, make sure you understand the ‘why’ behind the method. This ensures you can apply the technique in different contexts.
• Relate concepts: Try connecting new information to what you already know. This creates a more comprehensive understanding and helps to retain knowledge over time.
• Break down complex ideas: If a concept feels too complicated, break it into smaller, manageable pieces and solve them individually.

Practice Regularly and Strategically

Practice is essential for mastering any skill, and math is no exception. However, the quality of practice matters just as much as the quantity:

• Start simple: Begin with basic problems to ensure that you have a strong grasp of fundamental principles. As you gain confidence, gradually tackle more challenging tasks.
• Vary the problems: Don’t limit yourself to just one type of problem. A diverse set of exercises will help reinforce concepts and improve problem-solving flexibility.
• Track your progress: Keep a record of the problems you’ve solved, marking those that were difficult or required extra attention. Revisit these problems to reinforce your understanding.

By following these strategies, you’ll improve your skills and develop a stronger, more intuitive grasp of the subject, preparing you for increasingly difficult challenges ahead. Consistency, understanding, and practice will help you master exercises efficiently and effectively.

Improving Your Mathematical Skills with Practice

Building proficiency in mathematics is not a quick process, but rather one that thrives on consistent practice and problem-solving. Whether you are working through simple equations or more complex challenges, repeated engagement with different types of problems will gradually enhance your understanding and boost your confidence. Here are some effective strategies for improving your skills through targeted exercises.

Start with the Basics

A solid foundation is essential for mastering more advanced topics. Focus on understanding and mastering the fundamental principles before moving on to more complex problems:

• Review core concepts: Make sure you have a clear grasp of essential operations such as addition, subtraction, multiplication, and division, as these will form the basis of solving more intricate problems.
• Practice simple exercises: Begin with straightforward tasks to solidify your understanding, then progressively tackle more complicated challenges.

Consistency is Key

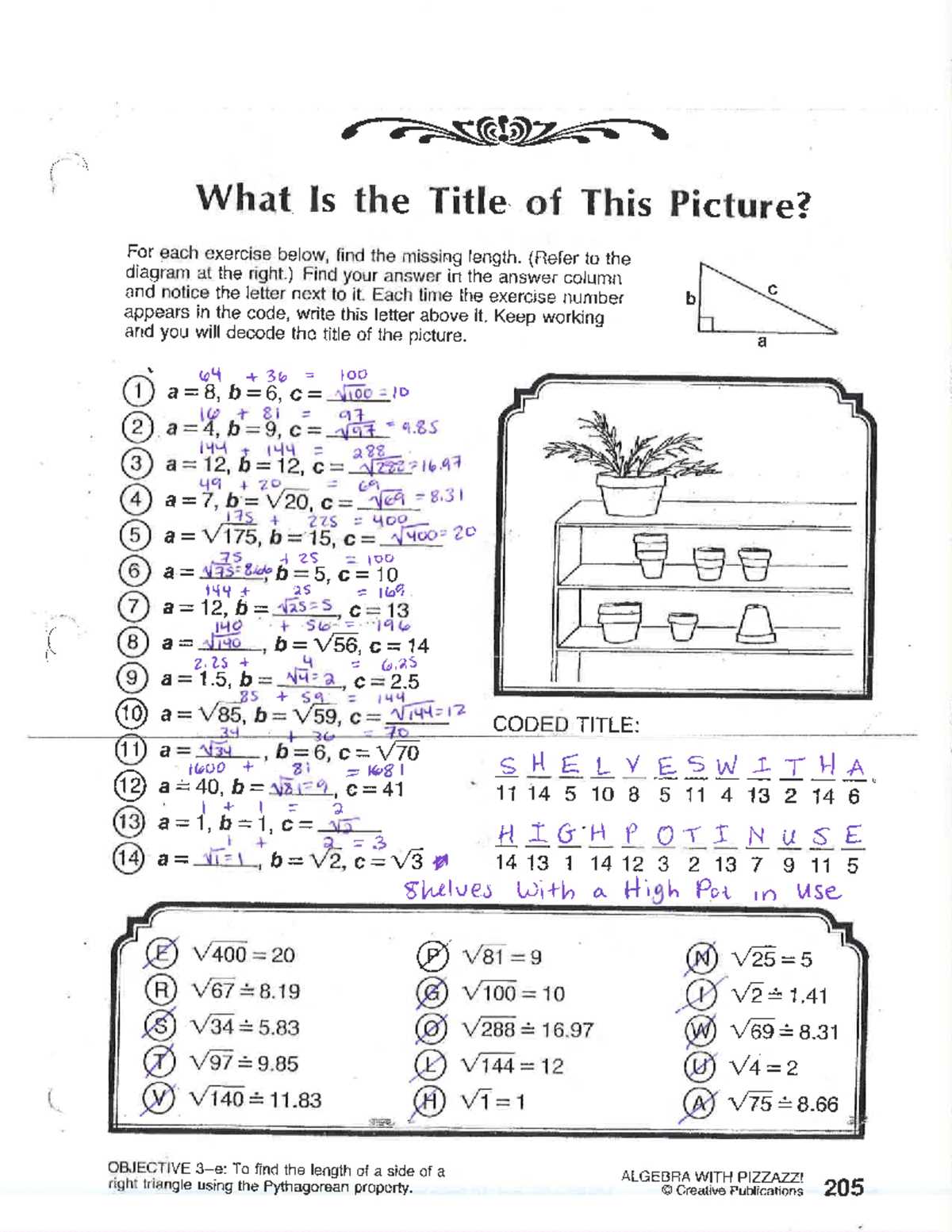
One of the most effective ways to improve is to practice regularly. Set aside time each day or week to work on math exercises, ensuring that you are constantly reinforcing what you’ve learned:

• Daily exercises: A short, focused session every day can help reinforce concepts and prevent forgetting key ideas.
• Practice varied problems: Work on a range of problem types to keep your skills sharp and adaptable to different challenges.
• Track progress: Keep a log of problems solved, noting areas where you struggled or succeeded, and revisit those areas to ensure continuous improvement.

Engage with Challenging Problems

To improve further, it’s important to step out of your comfort zone and attempt problems that are slightly more difficult than what you’re used to:

• Push your limits: Gradually introduce more challenging exercises as you become comfortable with easier ones. This helps to expand your abilities and fosters problem-solving growth.
• Understand mistakes: Whenever you make an error, take time to analyze why it happened and learn from it. This will help you avoid making the same mistakes in the future.

By dedicating time and effort to regular practice, focusing on mastering the fundamentals, and challenging yourself with increasingly difficult problems, you can significantly improve your mathematical abilities. The key is consistency, patience, and a willingness to learn from both successes and setbacks.

Using Solutions to Boost Understanding

Having access to solutions for exercises can be a valuable tool in enhancing comprehension. Rather than simply checking whether your responses are correct, reviewing solutions provides insight into the problem-solving process. This can deepen your understanding of the methods used and offer clarity on how to approach similar tasks in the future. The process of self-correction and learning from mistakes is an essential part of mastering any subject.

Understanding the Problem-Solving Process

When you refer to a solution, it’s crucial not just to look at the final answer, but also to focus on the steps taken to arrive at it:

• Step-by-step breakdown: By following each step of the solution, you can learn the logical sequence and methods used to solve the problem, which is often more beneficial than just knowing the answer.
• Identify techniques: Pay attention to any techniques or strategies employed to simplify the task, such as factoring, substitution, or pattern recognition. These methods can be applied to similar problems in the future.

Learning from Mistakes

One of the greatest benefits of using solutions is the opportunity to identify and understand your mistakes:

• Analyze errors: When you encounter a mistake, use the solution as a guide to pinpoint where things went wrong. This can help you avoid similar errors in future exercises.
• Revisit concepts: If mistakes stem from a lack of understanding of a particular concept, revisit the underlying material to strengthen that area and prevent recurring issues.

By using solutions not only to verify your answers but also to understand the reasoning behind each step, you can enhance your learning process. This approach leads to a deeper mastery of the subject, turning each error into an opportunity for growth.