Big Ideas Math Book Answer Key and Solutions

In any subject, having access to comprehensive solutions plays a vital role in understanding complex concepts. These resources are designed to help learners verify their work, identify areas for improvement, and reinforce their knowledge. Whether you’re a student or an educator, using a well-structured guide for problem-solving can make a significant difference in mastering difficult topics.

Mastering the process of problem-solving involves more than just knowing the right answer. It’s about understanding the steps taken to reach that solution. This resource provides detailed explanations, helping individuals break down each challenge and grasp the underlying principles.

With a well-organized structure, these guides support not only quick reference but also deeper learning. They offer clear and accurate methods to solve problems, ensuring that every learner can enhance their skills and gain confidence in their abilities.

Big Ideas Math Book Answer Key Guide

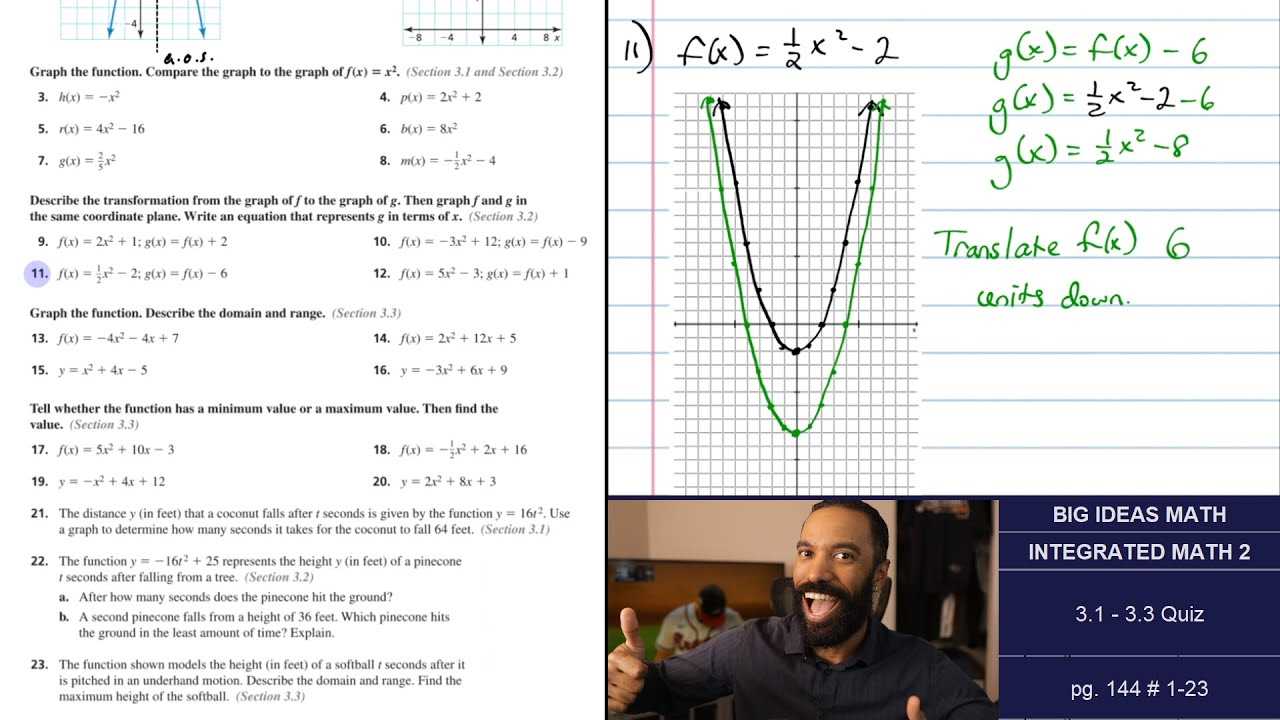
Having a detailed guide that provides solutions to problems is essential for reinforcing learning and ensuring understanding. These resources offer explanations to complex exercises, allowing individuals to check their work, spot errors, and build a stronger foundation in the subject. They serve as a valuable tool for students, educators, and anyone seeking to enhance their problem-solving abilities.

To make the most of a solution guide, it’s important to approach it methodically. Here are a few tips for effectively using this type of resource:

• Review each solution carefully: Focus not only on the final result but on the steps leading up to it. Understanding the process is key to mastering similar problems in the future.
• Use it for self-assessment: After solving a problem, cross-check your method with the guide to see if you followed the correct procedure.
• Identify common mistakes: If the solution doesn’t match your own, carefully analyze where you may have gone wrong. This helps in avoiding similar errors in the future.

While solutions provide a clear path to the correct result, they also offer deeper insights into the problem-solving process. These guides can be particularly useful in preparing for exams or revising challenging topics.

By using these resources wisely, you can improve your comprehension, speed, and accuracy in solving exercises, and ultimately strengthen your grasp of core concepts.

How to Use the Answer Key Effectively

Accessing a comprehensive solution guide can significantly enhance learning by providing clarity on problem-solving techniques. However, it is important to use such resources thoughtfully to maximize their benefits. Simply looking at the final result is not enough; understanding the steps involved is essential for deepening your knowledge and avoiding future mistakes.

Review the Process Step by Step

Focus on the methodology: Rather than rushing to check if your answer matches, take the time to understand each step in the solution. This will give you a clearer picture of how to approach similar problems in the future. Pay attention to the logic behind each calculation or decision.

Self-Assessment and Reflection

Evaluate your approach: After attempting a problem, compare your method with the guide to assess whether you followed the correct steps. If there are discrepancies, reflect on where you might have made errors and consider alternative strategies for solving the problem. This practice strengthens your overall problem-solving skills.

By approaching a solution guide with this level of engagement, you can make the most of the resource and gain a deeper understanding of the concepts involved. It turns the guide from just a tool for checking results into an invaluable learning asset.

Step-by-Step Solutions for Practice Problems

Breaking down complex exercises into smaller, manageable steps is crucial for mastering any subject. A structured approach to solving problems not only leads to correct solutions but also deepens understanding of the underlying principles. By following a clear sequence, learners can build confidence and improve their ability to tackle similar challenges in the future.

Understanding Each Step

Don’t rush through the solution: It’s important to fully understand the reasoning behind each step, even if the answer seems obvious. Carefully follow the logic behind each operation and ask yourself why each step is necessary. This will help solidify your comprehension of the concepts being applied.

Practice with Purpose

Focus on problem types: By practicing different problem types, you will start to recognize patterns in the solutions. Take time to compare how the steps vary between different problems, and how similar strategies can be applied across multiple exercises. This will increase your flexibility and readiness when facing new challenges.

Using a step-by-step approach to problem-solving encourages a deeper understanding and better retention of concepts. It ensures that learners not only reach the right answers but also gain valuable insights into the process of solving similar problems in the future.

Common Challenges in Big Ideas Math

In any educational resource, students often encounter obstacles that can hinder their progress. Understanding these challenges is key to overcoming them and developing a stronger grasp of the subject matter. Identifying the root causes of difficulties, whether they are conceptual, procedural, or based on individual learning styles, is essential for making consistent improvements.

Here are some common challenges learners face when working through complex exercises:

Challenge	Potential Cause	Solution
Difficulty Understanding Concepts	Lack of foundational knowledge or unclear explanations	Review earlier topics, seek clarification, use visual aids
Misinterpreting Problems	Failure to fully read and comprehend instructions	Take time to analyze each question before attempting a solution
Inconsistent Problem-Solving Approach	Jumping into problems without a clear strategy	Develop a systematic approach and break problems into steps
Errors in Calculation	Overlooking small details or rushing through steps	Double-check each step and review solutions carefully

Recognizing these challenges is the first step toward improving your problem-solving skills. By adopting a methodical approach and focusing on specific areas of difficulty, learners can build confidence and ensure steady progress.

Benefits of Understanding Each Answer

Simply knowing the correct solution to a problem is not enough for long-term success. Gaining a deep understanding of the process behind each solution helps reinforce the concepts and strengthens overall problem-solving skills. This approach allows learners to apply their knowledge to a wide variety of challenges, leading to greater confidence and mastery of the material.

Here are some key benefits of thoroughly understanding the solution process:

Benefit	Description
Enhanced Problem-Solving Skills	By understanding the steps involved, you can apply the same methods to different types of problems, improving your adaptability.
Improved Retention	When you grasp the reasoning behind each solution, you’re more likely to remember the steps and strategies in the future.
Increased Confidence	A deep understanding eliminates confusion and doubt, enabling you to approach new problems with assurance.
Identifying Weak Areas	Analyzing each step helps pinpoint where you may have misunderstood a concept, allowing you to focus on improving specific skills.

By taking the time to understand the reasoning behind each solution, learners not only improve their current performance but also develop the ability to tackle future challenges with greater ease and efficiency.

Exploring the Key Concepts in Math

Understanding the foundational principles behind any subject is crucial for effective learning. In this case, mastering the core concepts empowers learners to build a solid base of knowledge, enabling them to tackle more complex challenges with ease. A strong grasp of these ideas not only helps in solving individual problems but also aids in developing critical thinking and analytical skills.

Core concepts are the building blocks that students need to understand fully before moving on to more advanced topics. They include fundamental ideas that recur across various problems and scenarios, which are essential for mastering the subject. By exploring and deeply understanding these principles, learners can enhance their ability to solve problems more efficiently.

Key concepts often include topics such as number relationships, patterns, logical reasoning, and the application of formulas. Recognizing how these elements connect and support each other is essential for long-term success. Without understanding these basics, learners may struggle with more intricate concepts that rely heavily on this foundational knowledge.

Tips for Solving Complex Math Questions

Approaching difficult questions requires a clear strategy and a methodical approach. Complex problems often involve multiple steps and various concepts, which can make them challenging to solve. By following a systematic process, learners can break down the problem into manageable parts and find the solution more effectively.

Here are some valuable tips to help you solve intricate questions:

• Understand the problem: Carefully read the question and make sure you fully understand what is being asked before attempting to solve it.
• Break it down: Divide the problem into smaller, more manageable parts. Tackle each part separately to avoid feeling overwhelmed.
• Identify known and unknown variables: Clearly identify what information is given and what needs to be determined. This will help guide your approach.
• Use diagrams or visual aids: If applicable, drawing a diagram or chart can help you better visualize the problem and understand the relationships between different elements.
• Double-check your work: After solving the problem, review each step to ensure no errors were made, especially in calculations or logic.

By applying these strategies, you can approach difficult problems with confidence and improve your ability to solve them accurately and efficiently.

How the Answer Key Supports Learning

Accessing a solution guide can be a powerful tool in the learning process. Rather than merely offering the correct results, it serves as a detailed resource for understanding the thought process behind each problem. By reviewing step-by-step explanations, students can gain clarity on how to approach similar challenges and improve their overall problem-solving skills.

Clarification of Concepts: A solution guide helps to clarify difficult concepts by showing the exact methods used to arrive at a correct answer. This allows learners to see where they may have misunderstood a step or concept, providing a clearer path forward in their studies.

Filling Knowledge Gaps: By comparing their approach with the provided solutions, students can identify areas where they might lack understanding. This insight encourages them to review specific concepts, fill knowledge gaps, and strengthen their foundational skills.

Ultimately, the solution guide supports the learning process by serving as a reflective tool–enabling students to improve not only their understanding of individual problems but also their overall approach to tackling challenges in the future.

Improving Your Math Skills with Answers

Reviewing solutions is an essential part of strengthening your ability to tackle mathematical challenges. When you examine the reasoning behind a correct result, you gain valuable insight into the steps and strategies needed to solve similar problems in the future. This practice helps you recognize patterns, improve your approach, and build confidence.

By carefully analyzing each solution, you can identify areas where your understanding might be lacking and adjust your methods accordingly. This not only deepens your grasp of individual problems but also helps refine your overall problem-solving techniques. The more you practice and learn from the provided solutions, the more proficient you become in handling new and more complex tasks.

Understanding Different Problem Types

Every mathematical challenge presents its own unique set of requirements and approaches. Understanding the different categories of problems is crucial for applying the right techniques and strategies. Each type has specific characteristics that dictate how it should be tackled, from simple calculations to more complex multi-step processes.

By recognizing the structure and demands of various problem types, you can develop a more efficient approach to solving them. Whether dealing with equations, geometric figures, or data analysis, knowing how to break down a problem based on its category allows for better focus and improved accuracy in reaching the solution.

How to Check Your Work Using the Key

Reviewing your solutions is a critical step in the problem-solving process. After completing a task, checking your work ensures that you didn’t overlook any steps or make calculation errors. Using a solution guide can be an effective way to verify the accuracy of your answers and understand where you might have gone wrong.

Steps to Effectively Check Your Work

• Compare your results: Look at your final solution and compare it to the one provided in the guide. Ensure that the final answer matches the expected result.
• Review each step: Go through each part of your solution carefully. Check that the steps you took to reach the final answer align with the process used in the provided solution.
• Identify mistakes: If your solution differs from the guide, try to identify where the mistake occurred. Was it in the initial setup, a calculation, or a misapplication of a concept?

Tips for Improvement

• Understand the process: Simply checking the final result is not enough. Make sure you understand the reasoning behind each step in the solution guide.
• Practice regularly: The more you check your work and analyze the reasoning behind the solutions, the better you will become at recognizing and avoiding mistakes in the future.

By following these steps, you can confidently verify your work and improve your problem-solving skills over time. Understanding the process is key to mastering new concepts and refining your approach to future tasks.

Helping Students Prepare for Exams

Effective exam preparation involves more than just reviewing concepts–it requires a deep understanding of the material and the ability to apply it under pressure. Providing students with the right resources and strategies can significantly enhance their performance during assessments. One of the most valuable tools in this process is having access to solutions, which helps them practice and perfect their skills ahead of the test.

Strategies for Effective Exam Preparation

• Review key concepts: Ensure that students understand the foundational concepts. This can be done by revisiting problems, breaking them down into manageable parts, and analyzing the solutions provided.
• Practice regularly: Encourage regular practice with a variety of problems to reinforce learning and increase familiarity with different types of questions they may encounter.
• Focus on problem-solving methods: Teach students not only to reach the correct answers but also to focus on the methods used to solve the problems. This will help them approach new problems with confidence.

Using Solutions to Strengthen Understanding

• Analyze each solution: Guide students to go through each step of the provided solutions to ensure they understand the reasoning behind it. This helps to solidify their understanding of key techniques.
• Identify patterns: Regular exposure to different problem types and solutions helps students recognize common patterns, which aids in faster problem-solving during exams.

By incorporating these strategies and utilizing solution guides as a resource, students can enter their exams feeling confident and prepared to tackle any challenge that comes their way.

Common Mistakes and How to Avoid Them

When solving problems, it’s easy to fall into certain pitfalls that can lead to incorrect results. Many of these mistakes stem from misunderstandings or hasty steps, but with careful attention, they can be avoided. Recognizing these common errors and learning strategies to prevent them is crucial for improving accuracy and efficiency in problem-solving.

One frequent mistake is misinterpreting the problem itself. Sometimes, students rush through the question, not fully understanding the task at hand. This can lead to applying incorrect formulas or methods. To avoid this, take time to read the problem thoroughly and identify exactly what is being asked before proceeding.

Another common error is overlooking small details, such as signs or units of measurement. A minor sign change or missed unit conversion can drastically affect the final answer. To reduce this risk, double-check each step, paying close attention to these details, and always make sure the units are consistent throughout the process.

Lastly, calculation errors are also prevalent, especially in multi-step problems. These can be as simple as adding numbers incorrectly or misapplying arithmetic rules. A solid strategy is to work slowly and methodically, checking each intermediate step. Using a calculator when appropriate can help minimize this type of mistake.

By practicing patience and being mindful of these common mistakes, you can significantly improve the accuracy of your solutions and build more confidence in your problem-solving abilities.

Using the Answer Key for Group Study

Group study can be a highly effective way to reinforce learning, and using a solutions guide can make the experience even more productive. By working through problems together, students can share different approaches, clarify concepts, and help each other overcome difficulties. When combined with access to detailed solutions, this collaborative effort can deepen understanding and improve problem-solving skills.

Here are some strategies for using a solutions guide effectively in group study:

• Divide the work: Break down the set of problems into smaller sections and assign them to different group members. Each member can then work through their assigned section and share their solutions with the group.
• Discuss each step: Rather than just checking the final answer, work through each solution step by step. This will help everyone understand the reasoning behind the process and prevent errors from going unnoticed.
• Encourage active participation: Ensure everyone in the group is actively engaged. Rotate the role of explaining the solution to encourage deeper understanding and improve communication skills.
• Identify common mistakes: Use the solutions to highlight common errors or misconceptions. Discuss these mistakes as a group and work together to understand why they happened and how to avoid them in the future.
• Review difficult concepts: For more complex problems, take extra time to break down the steps and concepts involved. Use the solutions as a guide to clarify areas where the group is struggling.

Using a solutions guide in group study not only helps students get correct answers but also strengthens their understanding of problem-solving methods. Working together allows them to gain multiple perspectives, learn from each other, and enhance their overall grasp of the material.

How to Find Answers in the Book

Locating solutions within a study guide is a valuable skill that can greatly enhance your learning process. Understanding where to look and how to use provided resources effectively can save time and help you stay on track. This section outlines several methods to efficiently find the necessary information in a guide or reference material.

Steps to Find Solutions Quickly

• Use the Table of Contents: Start by checking the table of contents for a clear overview of the topics covered. This will allow you to pinpoint the chapter or section that matches the material you’re studying.
• Utilize Indexes: The index can be a helpful tool for locating specific terms, formulas, or concepts. Simply look up keywords related to the problem you’re trying to solve.
• Check for Detailed Explanations: Many guides include explanations or examples after the solutions. Use these to understand the reasoning behind the answers provided and learn how to approach similar problems.
• Look for Solution Sections: Some guides offer solutions in separate sections at the end of the chapter or in dedicated appendices. These sections are specifically designed to help you verify your work.

Tips for Efficient Use

• Search for Patterns: As you review the solutions, take note of recurring methods or strategies that can be applied to various problems. Recognizing these patterns will help you solve future questions more easily.
• Cross-reference with Examples: Compare the steps provided in examples with your own work. This cross-referencing technique can help you identify any errors or gaps in your understanding.
• Work Through Problems Independently First: Try to solve problems on your own before consulting the solution. This promotes critical thinking and helps you identify areas where you need further clarification.

By effectively navigating the guide, you can enhance your problem-solving abilities and gain a deeper understanding of the material. Proper use of the available resources will contribute to both your immediate success and long-term learning outcomes.

Explaining Answers for Better Understanding

Merely finding solutions is not always enough; understanding the reasoning behind them is crucial for deeper learning. Explaining how and why a particular method or result is correct can significantly enhance your grasp of the material. This section focuses on the importance of articulating solutions in detail, which helps solidify your comprehension and prepares you for future challenges.

When you break down each step involved in solving a problem, you reinforce the concepts in your mind. By explaining the logic behind each move, you can connect different ideas and uncover any misunderstandings you may have. Articulation forces you to think critically about each decision, ensuring that you aren’t just memorizing steps, but actually understanding the underlying principles.

Additionally, explaining solutions allows you to identify any patterns or strategies that may apply to other problems. This process of verbalizing your understanding can make abstract concepts more tangible and easier to recall later. Whether you explain it to yourself or others, the act of teaching is one of the most effective ways to reinforce knowledge.

Why Answer Keys Are Essential for Math

In the learning process, immediate feedback is crucial to ensuring that students understand the material correctly. Having a reliable reference for checking results is fundamental in helping learners confirm whether they are on the right track. This reference not only provides the correct solutions but also helps in identifying mistakes and improving problem-solving strategies.

Access to solutions supports students in gaining confidence, especially when faced with complex problems. It allows learners to evaluate their thought process and determine where errors might have occurred. Furthermore, this tool serves as a guide for understanding how to approach similar problems in the future, promoting a deeper understanding of the concepts involved.

Promotes Independent Learning

When students can verify their work, they become more independent in their studies. They can self-assess their understanding and progress without needing constant supervision. This sense of autonomy fosters self-discipline and allows students to take ownership of their learning process.

Clarifies Complex Concepts

By comparing their work to the provided solutions, students can better understand intricate procedures and techniques. This comparison helps bridge gaps in their knowledge, offering clarity on methods that might seem confusing at first glance. Ultimately, having a solution guide makes learning more accessible and effective.

How to Use Answer Keys for Self-Assessment

Self-assessment is an important part of the learning process, allowing students to monitor their progress and identify areas where improvement is needed. One of the most effective tools for this is having access to a guide that provides the correct solutions to problems. Using this guide thoughtfully can enhance self-awareness and lead to more targeted practice. It encourages reflection on one’s understanding and the identification of gaps in knowledge.

Step-by-Step Review

To effectively use a guide for self-assessment, follow a structured approach to compare your solutions with the provided ones. This can help highlight both strengths and weaknesses in your problem-solving approach.

• Work through each problem: Attempt each problem without any assistance, ensuring you rely solely on your understanding of the material.
• Check your results: After solving a problem, compare your solution with the correct one to see if you arrived at the same conclusion.
• Identify mistakes: If your answer differs, analyze the steps you took to find the mistake and correct it. This process helps reinforce understanding.
• Understand the solution process: Even if you get the correct answer, take time to understand the steps involved in the solution to ensure you can apply the same logic in future problems.

Table for Tracking Progress

Creating a table to track common errors can help in focusing your review efforts on recurring mistakes. This allows for more efficient learning and ensures that similar mistakes are not repeated.

Problem Number	Your Solution	Correct Solution	Mistake Identified	Action to Correct
1	15	16	Sign error	Review sign rules and retry
2	12	12	No mistakes	Continue practicing

By systematically using this approach, learners can improve their accuracy, understanding, and overall performance. Regular self-assessment fosters a deeper connection with the material and increases confidence in tackling new challenges.