Core Connections Algebra 2 Answer Key

Mastering advanced math concepts often requires access to reliable resources that can guide students through complex exercises. Whether you’re studying for exams or trying to better understand difficult topics, a detailed resource can make a significant difference in grasping challenging material. This article is designed to provide valuable support for anyone working through higher-level math problems, offering insights into solving equations and understanding the underlying principles.

By exploring various methods and solutions, learners can build confidence and improve their problem-solving abilities. From step-by-step explanations to practical examples, this guide aims to equip you with the tools needed for success. With consistent practice and access to comprehensive materials, any student can sharpen their skills and achieve greater mastery of mathematical concepts.

Core Connections Algebra 2 Answer Key

Accessing detailed solutions to mathematical exercises is crucial for students aiming to fully understand the material. Providing clear, structured solutions allows learners to recognize the logic behind each step and reinforce their knowledge. This section offers comprehensive guidance on solving challenging problems, enabling individuals to approach similar tasks with greater confidence and precision.

Each solution is carefully designed to illustrate the thought process behind problem-solving techniques, highlighting important concepts and methods. By examining these solutions, students can develop a deeper understanding of the material and improve their ability to solve problems independently. With the right support, complex mathematical topics become more approachable, and mastery becomes attainable.

Understanding the Algebra 2 Curriculum

The study of advanced mathematical concepts requires a structured approach that builds upon foundational skills. The curriculum for more advanced courses in mathematics is designed to challenge students with a variety of problem types, encouraging the development of analytical thinking and problem-solving abilities. Understanding the core objectives of this curriculum can help students navigate the complexity of the material and prepare for future coursework.

Key Topics Covered

• Linear equations and inequalities
• Quadratic functions and their properties
• Polynomials and rational expressions
• Exponential and logarithmic functions
• Systems of equations and matrices
• Sequences and series
• Probability and statistics

Approaching Difficult Topics

Some topics within this course can be challenging, particularly when dealing with abstract concepts like functions or advanced problem-solving strategies. However, breaking these topics down into smaller, manageable parts can make them easier to understand. Consistent practice and applying learned techniques to various examples can also reinforce comprehension and make the material more accessible.

Why Answer Keys Are Important

Having access to correct solutions is essential for students who are working through complex mathematical problems. Solution guides not only help confirm the accuracy of the work but also provide an opportunity to learn the correct methods and steps involved. By reviewing the reasoning behind each solution, students can identify mistakes and understand where they may have gone wrong, which is crucial for mastering the material.

Benefits of Using Solution Guides

• Helps verify accuracy and understanding
• Clarifies complex problem-solving steps
• Improves self-assessment and correction skills
• Encourages independent learning and confidence

How to Use Solution Guides Effectively

It’s important to use solution guides as a tool for learning rather than just as a means to check answers. By working through the problems on your own first, then comparing your solutions with the provided ones, you can identify areas for improvement and better understand the reasoning behind each step.

Step	What to Look for in the Solution	How It Helps
Step 1: Identify the problem	Ensure you understand the question and its requirements	Clarifies the goal of the problem-solving process
Step 2: Work through the solution	Compare each step with your own work	Reveals if any steps are skipped or misunderstood
Step 3: Analyze errors	Identify where your solution diverges	Allows you to correct mistakes and avoid them in the future

How to Use Core Connections Effectively

Utilizing educational resources properly can significantly enhance the learning experience. When working through challenging mathematical content, it’s crucial to approach the material in a structured and thoughtful way. A well-organized study plan can help students engage with the material more deeply and retain information more effectively, especially when using comprehensive study guides.

Developing a Structured Approach

Begin by reviewing the concepts presented in each section before diving into exercises. This initial overview helps you identify key topics and the overall structure of the material. Don’t rush through the problems; take your time to work through each step, applying the methods learned. Consistently reviewing solutions afterward allows you to check your understanding and pinpoint areas that need further attention.

Maximizing the Learning Experience

To get the most out of your study materials, make sure to practice regularly. Applying concepts to a variety of problems is the best way to reinforce understanding. Take advantage of practice sets that challenge you to use different strategies and problem-solving techniques. By revisiting difficult exercises, you can build stronger skills and improve your overall proficiency.

Step-by-Step Approach to Algebra 2 Problems

Solving complex mathematical problems becomes more manageable when approached methodically. Breaking down each problem into smaller, more manageable parts helps to clarify the process and ensures that no steps are overlooked. By following a structured approach, students can build confidence in their problem-solving abilities and avoid common mistakes.

Understanding the Problem

The first step in solving any problem is to fully understand what is being asked. Carefully read through the problem and highlight key information such as variables, constants, and operations. Take note of what you need to find–whether it’s an unknown variable, a graph, or a solution to an equation. This step sets the foundation for your approach and helps to keep you focused on the end goal.

Breaking Down the Steps

Once you understand the problem, start by identifying the most appropriate method to solve it. Look for patterns that can simplify the process, such as factoring, substitution, or graphing techniques. Next, work through each part of the solution one step at a time. Don’t rush–ensure that each calculation is accurate before moving on to the next one. Finally, once a solution is reached, double-check the results by substituting them back into the original equation to verify their correctness.

Common Algebra 2 Challenges and Solutions

Mathematics at an advanced level often presents a variety of challenges that can be difficult to navigate. These challenges may stem from complex concepts or unfamiliar problem-solving techniques. Understanding where students commonly struggle and learning strategies to overcome these hurdles can significantly improve both comprehension and performance in the subject.

Understanding Complex Functions

One of the most common struggles in higher-level math is dealing with complicated functions. Whether it’s exponential, logarithmic, or rational functions, the complexity of their properties can confuse students. Solution: Break down these functions step-by-step, starting with their basic forms and gradually exploring how transformations affect their graphs. Use graphical representations to visualize the behavior of these functions and understand their behavior in different contexts.

Solving Systems of Equations

Another frequent difficulty arises when solving systems of equations, particularly when multiple variables are involved. Without a solid understanding of solving one-variable equations, progressing to systems can be overwhelming. Solution: First, practice solving simpler equations. Then, apply methods like substitution or elimination to systematically reduce the system to simpler parts. Visual aids like graphing the systems can also help in better understanding their solutions.

Exploring the Structure of Core Connections

Understanding the structure of educational resources is essential for making the most of the material. A well-organized system not only ensures that the content is presented logically but also helps students build upon their existing knowledge. By exploring the layout and approach, learners can better navigate through complex topics and approach each lesson with confidence.

Key Features of the Structure

• Introduction to Key Concepts: Each section starts with an overview of the main ideas, helping to build a strong foundation.
• Step-by-Step Guidance: Lessons are broken down into manageable steps, making it easier to understand difficult material.
• Practice Exercises: A variety of exercises are included to reinforce learning and provide opportunities for applying new concepts.
• Real-World Applications: The curriculum often includes practical examples to show how abstract concepts are used in everyday scenarios.

How the Structure Supports Learning

This structured approach helps students progress at their own pace, ensuring that each concept is fully understood before moving on. By organizing the material in this way, learners can track their progress, identify areas that need more focus, and build confidence as they advance through the course.

Key Concepts Covered in Algebra 2

Advanced mathematical studies involve mastering a range of important ideas that build on foundational skills. The topics covered in these courses are designed to deepen understanding of mathematical relationships and prepare students for further academic pursuits. By focusing on both theory and application, students gain a solid grasp of the concepts necessary for solving more complex problems.

Key Areas of Study

Topic	Description	Application
Quadratic Functions	Study of second-degree polynomial functions and their graphs	Used to model parabolic relationships in real-life situations like projectile motion
Systems of Equations	Solving sets of linear equations with multiple variables	Application in finding solutions for problems involving constraints, such as budgeting or optimization
Exponential and Logarithmic Functions	Understanding growth and decay models, and inverse functions	Important for modeling exponential growth in populations or financial investments
Polynomials and Rational Functions	Exploration of functions with multiple terms and rational expressions	Used in engineering and physics for complex modeling tasks
Sequences and Series	Study of ordered sets of numbers and their sums	Helps in calculating compound interest, financial planning, and predicting future patterns

These concepts lay the groundwork for solving increasingly sophisticated mathematical problems, with each topic contributing to a broader understanding of how numbers and relationships behave. Mastering these areas is essential for those planning to pursue further studies in mathematics, science, or economics.

How to Verify Your Algebra Answers

Ensuring that your solutions to mathematical problems are correct is a critical part of mastering the subject. Verifying your results helps identify any errors that may have occurred during the problem-solving process. By using various methods to double-check your work, you can gain more confidence in your understanding and accuracy.

Methods for Verifying Solutions

• Substitution: One of the most straightforward ways to check your work is to substitute your solution back into the original equation. If the equation holds true, your solution is correct.
• Graphing: For equations involving variables, graphing the functions can visually confirm the accuracy of your solutions. If the point of intersection matches your solution, you can be confident in your answer.
• Using Inverse Operations: Solving for an unknown often involves a series of operations. By reversing these operations, you can confirm that the steps you took to find the solution were correct.
• Estimation: If the problem allows, you can estimate an approximate solution. This helps ensure that your result is within a reasonable range, especially for more complex problems.

By applying these techniques, you can consistently verify the correctness of your solutions and improve your problem-solving skills over time. Verification not only helps catch mistakes but also reinforces your understanding of key concepts.

Using Practice Exercises for Better Learning

Engaging in regular practice is one of the most effective ways to reinforce your understanding of mathematical concepts. By working through a variety of problems, you not only solidify your knowledge but also develop the critical thinking skills necessary to approach new and more complex challenges. Practice exercises offer a structured way to test your skills, track progress, and identify areas that need improvement.

Why Practice is Essential

Repetition is key when it comes to mastering new concepts. The more you practice, the more familiar you become with different types of problems and the strategies needed to solve them. By consistently applying what you’ve learned, you strengthen both your memory and problem-solving abilities, making future lessons easier to comprehend.

How to Use Practice Exercises Effectively

Approach	Description	Benefit
Start Simple	Begin with basic problems to build your confidence before moving to more complex ones.	Helps establish a solid foundation and reduces the risk of frustration.
Review Mistakes	After completing exercises, carefully review any mistakes to understand what went wrong.	Identifying errors enhances learning and prevents repeating them in the future.
Mix Problem Types	Work on different types of problems in each session to broaden your understanding of the topic.	Prepares you for diverse challenges and ensures a well-rounded grasp of concepts.
Set Time Limits	Challenge yourself by setting time limits to complete exercises.	Improves efficiency and helps you manage time during tests and exams.

By adopting these strategies, you maximize the value of practice exercises, making your study time more effective and efficient. Continuous practice not only boosts your performance but also deepens your understanding of complex mathematical principles.

Tips for Mastering Algebra 2 Topics

Achieving mastery in advanced mathematical topics requires a combination of consistent practice, strategic learning, and the right approach to problem-solving. Whether you’re tackling complex functions or working through intricate equations, having effective strategies can significantly improve your understanding and performance. Below are several tips to help you succeed and strengthen your skills in this challenging subject.

Effective Strategies for Mastery

• Break Down Complex Problems: Large problems can seem overwhelming. Break them down into smaller, manageable parts. Solve each part step by step to maintain focus and reduce errors.
• Focus on Concepts, Not Just Formulas: Understanding the underlying principles behind each formula is more important than memorizing them. Grasping the “why” behind a method helps you apply it more effectively.
• Practice Regularly: Repetition is key to retaining new concepts. Set aside dedicated time to solve practice problems every day to reinforce your knowledge.
• Seek Help When Stuck: If you find yourself struggling with a particular topic, don’t hesitate to ask for help. Whether it’s from a tutor, a classmate, or online resources, getting clarification can save you time and frustration.
• Work on Word Problems: These problems often require more critical thinking and the application of multiple concepts. Practicing word problems can help you understand how abstract concepts work in real-life situations.

Additional Tips for Success

• Stay Organized: Keep your notes, assignments, and practice problems well-organized. This allows you to easily review key concepts and track your progress.
• Use Visual Aids: Diagrams, graphs, and charts can help you visualize complex relationships and make abstract concepts easier to understand.
• Review Mistakes: When you make a mistake, take the time to review and understand why it happened. This helps you avoid similar errors in the future.
• Apply Real-World Examples: Try to relate mathematical concepts to real-world scenarios. This can deepen your understanding and make the subject more engaging.

By incorporating these strategies into your study routine, you’ll be better equipped to handle the challenges presented by advanced mathematical topics. Mastery comes with time, patience, and a focused approach to learning, so stay committed and keep practicing!

Resources for Algebra 2 Study Support

When tackling advanced mathematical concepts, having access to the right resources can make all the difference in understanding and mastering the material. Whether you’re looking for online tools, textbooks, or community support, there are numerous options available to help reinforce your learning. Utilizing these resources effectively can boost your confidence and improve your problem-solving abilities.

Online Tools and Platforms

• Khan Academy: A popular platform offering free lessons and practice exercises on a wide range of topics, including advanced mathematics. Their step-by-step tutorials are a great way to reinforce difficult concepts.
• Wolfram Alpha: A powerful computational tool that allows you to solve equations, visualize graphs, and explore detailed solutions to various math problems.
• Mathway: A problem-solving app that provides instant answers and explanations for a variety of math problems, making it a great tool for quick learning.
• Desmos: An interactive graphing calculator that helps visualize functions, equations, and their solutions. This tool is especially useful for understanding the relationships between variables.

Additional Resources for Success

• Textbooks and Workbooks: Standard math textbooks often contain detailed examples and practice problems that can help solidify your understanding. Workbooks also offer additional exercises for practice and review.
• Study Groups: Joining or forming a study group can help you tackle difficult problems collaboratively. Explaining concepts to others can reinforce your understanding and offer new perspectives on a topic.
• Tutoring Services: If you’re struggling with specific topics, one-on-one tutoring can provide personalized attention and tailored explanations to help you grasp challenging material.
• Online Forums: Websites like Stack Exchange and Reddit host math communities where students can ask questions, share solutions, and discuss difficult topics with others.

By taking advantage of these resources, you can find the support you need to succeed in your studies. Whether you’re learning independently or seeking help from others, there are countless tools available to enhance your comprehension and mastery of advanced topics.

Core Connections vs Traditional Algebra Books

When it comes to studying advanced mathematics, different educational approaches can significantly impact how well students grasp complex topics. Two of the most common methods involve using progressive, inquiry-based resources versus more traditional, step-by-step textbooks. Each method offers its own advantages and challenges, and understanding the differences can help students choose the right materials for their learning style.

Progressive learning methods often focus on encouraging critical thinking and problem-solving from the start, while traditional textbooks provide clear, structured guidance with step-by-step explanations. This section will explore the key differences between these two approaches and help students understand which might be more effective for their needs.

Aspect	Progressive Approach	Traditional Approach
Structure	Flexible, open-ended problems that encourage exploration.	Clear, step-by-step explanations with a defined sequence.
Learning Style	Designed for self-discovery and collaborative learning.	More suitable for students who prefer clear instructions and repetition.
Focus	Emphasizes understanding concepts and applying them in various contexts.	Focuses on mastering techniques and formulas before applying them.
Examples	Real-world applications and open-ended problems that require creative solutions.	Numerical problems with defined solutions that demonstrate specific methods.
Support	Students often need external help or peer discussion to fully grasp the concepts.	Guided practice problems with detailed explanations help reinforce learning.

Both methods have their strengths, and choosing between them often depends on the student’s learning preferences and academic goals. Some may prefer the structured approach of traditional textbooks, while others might thrive in a more explorative, concept-driven environment. The key is to identify which approach suits one’s personal learning style and to use available resources effectively to maximize understanding.

Understanding Problem Types in Algebra 2

In advanced mathematical studies, different types of problems require varied approaches and strategies. Understanding the structure and format of each problem type can significantly enhance problem-solving skills and lead to more efficient solutions. This section provides an overview of the most common problem types found in higher-level mathematics and offers insight into how to tackle each one effectively.

Recognizing the problem’s nature is the first step toward applying the right techniques. Whether it involves solving for unknown variables, working with functions, or simplifying complex expressions, each type of problem follows a specific pattern and requires a unique approach.

Common Problem Types

• Linear Equations – Problems involving equations where variables are raised to the first power. These are often solved through simple algebraic manipulation like addition, subtraction, multiplication, and division.
• Quadratic Equations – Problems that require factoring, completing the square, or using the quadratic formula to find solutions. These equations often have two solutions, either real or complex.
• Rational Expressions – Problems that involve fractions with variables in the numerator or denominator. Techniques such as finding a common denominator or simplifying fractions are key here.
• Exponential Functions – Problems involving growth or decay patterns, where variables appear in the exponent. These can be solved using logarithms or by applying specific exponential properties.
• Systems of Equations – These problems involve solving multiple equations with multiple variables. Methods such as substitution, elimination, or matrix operations are often used to find solutions.
• Inequalities – Problems where the goal is to find the range of values that satisfy a condition. These require understanding of operations with inequalities and graphing on a number line.

Approaches to Solving These Problems

• Step-by-step methods – Breaking down complex problems into simpler steps makes them easier to solve. This technique is useful for linear, quadratic, and rational problems.
• Graphical solutions – Sometimes, solving problems graphically can provide insights or confirm algebraic solutions. This method is particularly useful for inequalities and functions.
• Substitution and elimination – For systems of equations, using substitution or elimination methods can simplify the process of finding the variable values.
• Factoring – Factoring expressions or equations can be the quickest way to find solutions, especially with quadratics or rational expressions.

Mastering these problem types and approaches will help you develop a strong foundation in mathematical reasoning and problem-solving. Each type of problem builds on the previous one, so understanding the basics thoroughly is essential for tackling more advanced topics.

The Role of Answer Keys in Self-Study

When studying independently, having a reliable way to verify your progress is essential for improving understanding and identifying areas that need further work. Tools that provide correct solutions to exercises play a crucial role in this process. By comparing your results with the correct ones, you can assess your grasp of the material and adjust your learning strategies accordingly.

One of the most significant benefits of using solution guides is the opportunity for immediate feedback. This allows you to identify mistakes and learn from them rather than continuing with misunderstandings. Moreover, these resources help in building confidence as you can track your improvement over time.

Immediate Feedback and Error Correction

• Identifying mistakes – When working through problems, it’s easy to overlook errors. A solution guide lets you quickly spot these and understand why certain approaches did not work.
• Learning from errors – Instead of just fixing mistakes, solution guides provide insights into why a particular method is correct, helping to deepen your understanding of the subject matter.
• Avoiding repeated mistakes – By reviewing the solutions thoroughly, you can avoid making the same errors in future exercises.

Building Confidence Through Self-Assessment

• Tracking progress – Regularly checking your solutions against a reliable guide lets you monitor your improvement, which is motivating and helps reinforce your learning.
• Gaining independence – Having access to solutions fosters self-reliance. Over time, you’ll be able to tackle more complex problems on your own without needing external help.
• Filling gaps in knowledge – If you consistently struggle with specific problem types, solutions can highlight these gaps, allowing you to focus on the areas that need the most attention.

In summary, using solution guides in self-study provides essential feedback, promotes error correction, and boosts confidence. It helps learners develop both the skills and the autonomy needed to master the material efficiently and effectively.

Common Mistakes to Avoid in Algebra 2

When studying advanced mathematical topics, certain mistakes are frequently made that can hinder progress. Recognizing and avoiding these errors is key to mastering complex concepts. Many learners struggle with similar pitfalls, such as misinterpreting instructions, skipping steps, or not fully understanding the underlying principles of a problem. By addressing these common mistakes, students can improve their understanding and confidence in solving mathematical problems.

Misunderstanding Problem Instructions

• Skipping important details – It’s easy to overlook specific instructions or constraints mentioned in a problem. Carefully reading the question and identifying all the given information is crucial before starting to solve the problem.
• Incorrectly applying formulas – Using formulas without fully understanding their purpose or when they should be applied can lead to errors. Make sure to review the context in which a formula is valid.
• Assuming the wrong operation – Sometimes, a problem may involve several operations, but the wrong one may be assumed at the start. Always double-check the operations that are being asked for in the problem.

Skipping Steps and Rushing Through Solutions

• Omitting intermediate steps – When solving complex problems, skipping intermediate steps in favor of quick shortcuts can lead to mistakes. Take the time to break down each part of the problem to ensure accuracy.
• Mismanaging time – Rushing through problems or becoming too focused on speed can lead to errors. It’s important to balance efficiency with accuracy, especially when tackling multi-step problems.
• Overcomplicating simple steps – Sometimes, problems are simpler than they first appear, but overthinking can make them more complicated. Try to approach each problem with a clear, logical thought process.

By recognizing these common pitfalls and working to avoid them, learners can gain a clearer understanding of the material and improve their problem-solving abilities. Patience, attention to detail, and a step-by-step approach are essential when tackling advanced mathematical challenges.

How to Prepare for Algebra 2 Exams

Preparing for an exam in advanced mathematics requires a structured approach and dedication. Whether you’re tackling complex equations, inequalities, or functions, effective preparation can significantly improve your performance. To master the content and boost your confidence, it’s essential to focus on key areas, review essential concepts, and practice problem-solving strategies. A well-organized study plan can make a big difference in how well you grasp the material and apply it during the exam.

Review Key Topics and Concepts

• Identify critical areas – Focus on the most important topics that are frequently tested, such as polynomials, quadratic equations, rational expressions, and functions. Review class notes, textbooks, and any provided resources to ensure you cover these areas thoroughly.
• Understand the fundamentals – Make sure you have a solid grasp of foundational concepts. Understanding the underlying principles will help you approach problems logically and avoid confusion during the test.
• Organize formulas – Create a cheat sheet with essential formulas and methods for quick reference. This can help you recall important information during the exam and ensure that you’re applying the right technique to each problem.

Practice and Problem-Solving Strategies

• Work through sample problems – Practice is crucial for mastering mathematical techniques. Solve various problems from textbooks, practice tests, or online resources to familiarize yourself with different problem types and develop efficient strategies.
• Understand solution steps – It’s not just about finding the correct answer; it’s also about understanding the steps involved in reaching that solution. Practice solving problems step-by-step to ensure clarity in your thought process.
• Simulate exam conditions – Set up timed practice sessions to replicate the pressure of the actual exam. This will help you manage your time effectively and stay calm under pressure.

By systematically reviewing the material, practicing problem-solving, and staying organized, you’ll be better equipped to handle the challenges that arise during your exam. Consistency and focus in your preparation are the keys to success.

Improving Problem-Solving Skills in Algebra

Enhancing your ability to solve mathematical problems involves more than just understanding formulas. It’s about developing a methodical approach to tackling challenges, refining your reasoning skills, and learning how to break down complex problems into manageable steps. With practice and the right techniques, anyone can improve their problem-solving abilities and approach even the most difficult problems with confidence.

Strategies to Enhance Problem-Solving

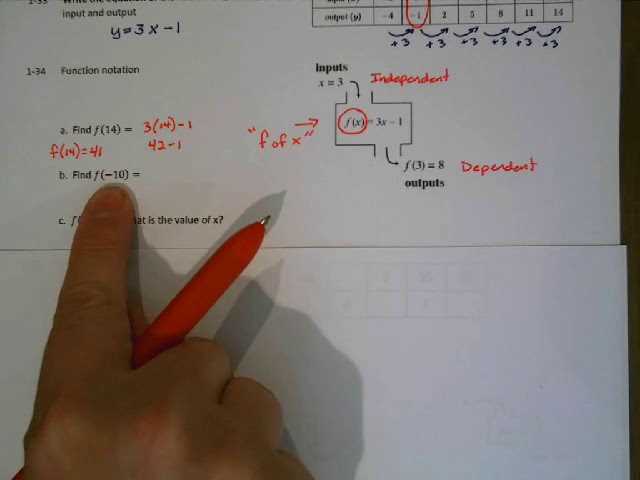
• Understand the problem – Start by carefully reading the problem to ensure you fully understand what is being asked. Identify the given information and what needs to be determined. This initial step is crucial for forming a clear plan of action.
• Break the problem down – Complex problems can be intimidating, but they are often easier to solve when broken into smaller, more manageable pieces. Work through the problem step-by-step, focusing on one part at a time.
• Identify patterns and relationships – Many problems have underlying patterns or relationships that, once identified, can make the solution process much quicker. Look for patterns in numbers, variables, or operations that might simplify the task.
• Choose the right technique – Different types of problems require different methods. Whether it’s factoring, graphing, or using the quadratic formula, knowing which technique to apply is vital. Practice using a variety of methods so you can choose the most appropriate one for each situation.

Practicing Regularly

• Solve a variety of problems – The more you practice, the better you’ll become. Challenge yourself with a range of problems, including both simple and advanced examples, to build your confidence and reinforce different skills.
• Review your mistakes – Learning from mistakes is one of the best ways to improve. After solving a problem, take the time to review your work and identify any errors. Understanding why a mistake occurred helps prevent it in the future.
• Use resources effectively – Don’t hesitate to use study guides, textbooks, or online resources when you’re stuck. Sometimes, a different explanation or approach can help clarify the problem and guide you toward the solution.

By consistently practicing these strategies, you’ll build a strong foundation in problem-solving that will allow you to approach mathematical challenges with confidence and ease. The key is to stay persistent, patient, and proactive in your learning process.