Core Connections Course 3 Chapter 1 Answer Key

In the first section of this learning material, a variety of essential mathematical concepts are introduced to build a strong foundation for the upcoming lessons. The problems presented in this segment are designed to challenge your understanding and help you develop a deeper grasp of key mathematical principles. With a focus on critical thinking and problem-solving, this section encourages active engagement and hands-on practice.

Effective learning involves not only understanding the material but also reviewing and verifying your solutions. By exploring the provided solutions, you gain insight into different approaches to solving the problems and improve your problem-solving skills. These solutions offer a roadmap to better understanding and allow you to correct any misconceptions you may have encountered during your practice.

The materials are structured to guide you step-by-step through the learning process, providing both clarity and confidence as you progress. With a combination of visual aids and detailed explanations, this section supports learners in tackling more complex challenges with ease and precision.

Mathematical Problem-Solving Solutions for Initial Lessons

This section provides a comprehensive collection of solutions for the first set of problems in the material. By offering clear, step-by-step instructions, it allows learners to reflect on their approaches and refine their understanding. The solutions focus on explaining the reasoning behind each answer, ensuring that every learner can follow the process from start to finish and improve their problem-solving skills.

Step-by-Step Problem Breakdown

Each solution is accompanied by a detailed explanation that highlights important steps in solving the problems. This breakdown helps clarify the logical process and demonstrates how to apply learned concepts effectively. Emphasis is placed on understanding the ‘why’ behind each step, ensuring that learners gain more than just the correct solution.

Improving Understanding Through Practice

Using this section as a reference, students can test their own solutions against the provided explanations. Consistent practice allows for better retention of material and a deeper grasp of mathematical principles. Mistakes are a natural part of the learning process, and revisiting complex problems with new insights can lead to a more complete understanding.

Overview of Core Connections Course 3

This section introduces the initial topics of the educational material, designed to enhance mathematical understanding and provide students with the skills necessary to tackle complex problems. The focus is on building a solid foundation through gradual progression and thoughtful exploration of new concepts. As the lessons advance, students will be guided to develop both conceptual understanding and practical problem-solving abilities.

The structure of the material is organized to encourage active learning and engagement. Each topic is carefully designed to challenge learners and foster critical thinking. Key objectives include:

• Strengthening problem-solving techniques
• Developing a deeper understanding of mathematical principles
• Enhancing reasoning and logical thinking abilities
• Improving accuracy and efficiency in solving complex exercises

Throughout the lessons, emphasis is placed on helping learners apply theoretical knowledge in practical situations, reinforcing the idea that mathematics is not just about memorizing formulas but understanding their applications in real-world contexts.

Key Concepts Covered in Chapter 1

The first section of the material introduces several foundational ideas that are critical for developing a strong mathematical base. These concepts are essential for solving a wide range of problems and are meant to prepare learners for more advanced topics. The focus is on ensuring that students understand the core principles before progressing to more complex exercises.

Essential Mathematical Skills

This section lays the groundwork for various essential skills that will be used throughout the study. Key topics include:

• Basic operations and their applications
• Understanding variables and expressions
• Identifying patterns and relationships
• Interpreting mathematical problems accurately

Problem-Solving Strategies

In addition to mastering the basic skills, learners are introduced to a range of strategies for approaching different types of problems. These include:

• Breaking down complex problems into simpler parts
• Using logical reasoning to guide solutions
• Recognizing patterns to predict outcomes
• Checking work for accuracy and consistency

By mastering these foundational concepts, students are better equipped to tackle challenges and deepen their understanding of mathematics.

Understanding the Answer Key Structure

The provided solutions are organized in a way that offers clarity and easy access to important details. Each solution is presented step by step, breaking down the problem-solving process into manageable parts. This structure helps students not only find the correct answers but also understand the reasoning behind each step, enhancing their overall comprehension.

Logical Flow is a key feature of the structure, ensuring that each solution follows a clear and sequential order. By following this methodical approach, learners can see how each stage builds on the previous one, allowing them to grasp the connections between different concepts.

Additionally, explanations are concise yet thorough, making complex ideas more accessible. This format helps learners reflect on their own methods and compare them with the suggested approach, leading to a deeper understanding of the material.

Importance of Practice Problems in Chapter 1

Engaging with practice exercises is essential for reinforcing the concepts introduced in the initial lessons. These problems provide learners with the opportunity to apply newly acquired knowledge and test their understanding in real-world contexts. By practicing regularly, students can identify areas that need improvement and solidify their grasp of fundamental principles.

Key benefits of completing practice exercises include:

• Improved problem-solving skills through hands-on experience
• Increased familiarity with different types of mathematical problems
• Greater confidence in tackling more complex challenges
• Ability to recognize patterns and apply strategies more effectively

By consistently working through practice problems, learners not only improve their skills but also develop the ability to think critically and approach problems systematically. This process ensures a deeper understanding of the material and better prepares students for future lessons.

Tips for Using the Answer Key Effectively

Utilizing the provided solutions correctly can significantly enhance your learning experience. Rather than simply looking for the final result, it is important to carefully follow the reasoning and steps outlined in each explanation. This approach helps you not only check your work but also understand the logic behind the problem-solving process.

Here are some tips for making the most of the solutions:

Tip	Explanation
Review Before Checking	Attempt the problem on your own first. Use the solution only after you’ve made your best effort.
Understand Each Step	Instead of just copying the solution, take time to understand why each step is taken.
Compare Your Method	Look at how your approach matches the solution. If different, analyze the reasons behind the difference.
Revisit Mistakes	When you get an answer wrong, focus on why the mistake was made. This helps avoid similar errors in the future.

By applying these strategies, you can use the solutions not only as a reference for correctness but as a tool for deeper understanding and improvement.

Common Mistakes in Chapter 1 Exercises

As learners work through the initial problems, certain mistakes tend to appear frequently. These errors can hinder progress and lead to misunderstandings of the fundamental concepts. Recognizing and addressing these common mistakes is crucial for improving performance and deepening one’s understanding of the material.

Frequent Errors in Problem Solving

Here are some of the most common mistakes made during the exercises:

• Misinterpreting the problem statement, leading to incorrect assumptions.
• Forgetting to apply the proper order of operations when solving equations.
• Overlooking small details, such as missing parentheses or signs, that significantly alter the outcome.
• Rushing through steps without double-checking calculations.
• Incorrectly simplifying expressions or terms, leading to wrong conclusions.

How to Avoid These Errors

To minimize these mistakes, consider the following strategies:

• Read each problem carefully and ensure a full understanding before starting.
• Break problems into smaller, manageable parts to reduce errors in calculations.
• Always double-check each step to make sure nothing is overlooked.
• Use a systematic approach to avoid skipping important steps.

By being aware of these common mistakes and using strategies to avoid them, learners can greatly improve their accuracy and overall understanding of the material.

How to Review Answers for Better Understanding

Reviewing your solutions carefully is essential for truly grasping the material and improving your problem-solving abilities. It’s not enough to simply check if your answers are correct; the process of reviewing should focus on understanding why a solution works, identifying any mistakes, and learning from them. This reflective approach helps reinforce your learning and enhances your overall comprehension of the subject.

Here are some effective methods for reviewing your solutions:

Step	What to Focus On
1. Analyze the Steps	Carefully review each step of the solution. Understand the reasoning behind it and how it leads to the next step.
2. Compare Approaches	If your method differs from the provided solution, compare the two approaches to understand the differences and similarities.
3. Identify Mistakes	If you made an error, pinpoint where you went wrong. Was it a misinterpretation of the problem or a calculation mistake?
4. Reinforce Understanding	Revisit concepts or steps you found confusing, and try to work through similar problems to solidify your understanding.

By systematically reviewing your solutions, you can deepen your understanding, avoid repeating mistakes, and build a more robust foundation for tackling future problems.

Connecting Chapter 1 to the Larger Course

The first set of exercises lays the foundation for many of the concepts that will be explored in greater detail throughout the entire program. The skills and knowledge acquired here will serve as building blocks for more complex ideas and challenges that will be encountered in later sections. Understanding how these initial lessons fit into the bigger picture is key to maintaining a clear learning path and building confidence in your abilities.

Building a Strong Foundation

The early material focuses on basic principles that are integral to mastering more advanced topics. By mastering these fundamental concepts, learners can approach later lessons with greater ease and a deeper understanding of the material. This progression ensures that each new idea connects logically to the ones learned before, creating a coherent and structured learning experience.

Linking New Topics to Prior Knowledge

As you move forward, you will find that the exercises in later sections often revisit concepts introduced here, but with added complexity. The ability to recall and apply these early lessons is crucial for success in future topics. Continuously reflecting on how earlier material relates to more advanced topics helps strengthen retention and comprehension.

Helpful Resources for Chapter 1 Learning

To enhance your understanding of the material covered in the first section, a variety of resources are available to provide additional support and clarify concepts. Whether through online tutorials, practice platforms, or supplementary guides, these tools can deepen your grasp of key topics and help you overcome challenges. Utilizing these resources effectively will ensure that you build a strong foundation for tackling more advanced material.

Online Tutorials and Video Lessons

Many online platforms offer video tutorials that break down complex topics into easy-to-understand explanations. These videos often include visual demonstrations of problem-solving methods and strategies, which can make difficult concepts more accessible. Platforms like Khan Academy and Coursera provide free content tailored to various learning levels, offering additional perspectives on the topics you’re studying.

Practice Tools and Exercises

In addition to textbook problems, using interactive practice tools can help reinforce learning. Websites like Wolfram Alpha and Desmos allow you to experiment with mathematical concepts, test solutions, and visualize problems in real-time. These tools are especially useful for practicing problem-solving techniques and verifying your work.

Detailed Step-by-Step Solutions in the Key

One of the most valuable aspects of solving problems is understanding the process behind the solution. The detailed, step-by-step breakdowns provided for each problem guide learners through each stage of the solution, explaining the reasoning behind every step. This structured approach not only helps confirm the correctness of answers but also strengthens the overall problem-solving skills needed to approach future exercises with confidence.

By following these comprehensive instructions, learners can gain insight into common methods, identify possible areas of confusion, and reinforce their understanding of key concepts. The goal is to offer clear explanations that demystify each step, helping learners to not only replicate the process but also to understand the logic driving it. This clarity is essential for building a strong foundation that will support more advanced challenges down the road.

Visualizing Problems for Easier Understanding

One of the most effective strategies for mastering complex concepts is to visualize the problems you’re trying to solve. Creating diagrams, charts, or other visual representations of the material can simplify abstract ideas and help make them more tangible. By transforming word problems or equations into visual formats, learners can see relationships, patterns, and connections more clearly, which aids in the comprehension and retention of key information.

Using Diagrams and Graphs

For many mathematical and logical problems, drawing a diagram or graph can help clarify the steps involved. Whether it’s plotting points on a graph or sketching out a geometric shape, visuals make it easier to understand the spatial or quantitative relationships between different elements of a problem.

Flowcharts and Step-by-Step Visual Guides

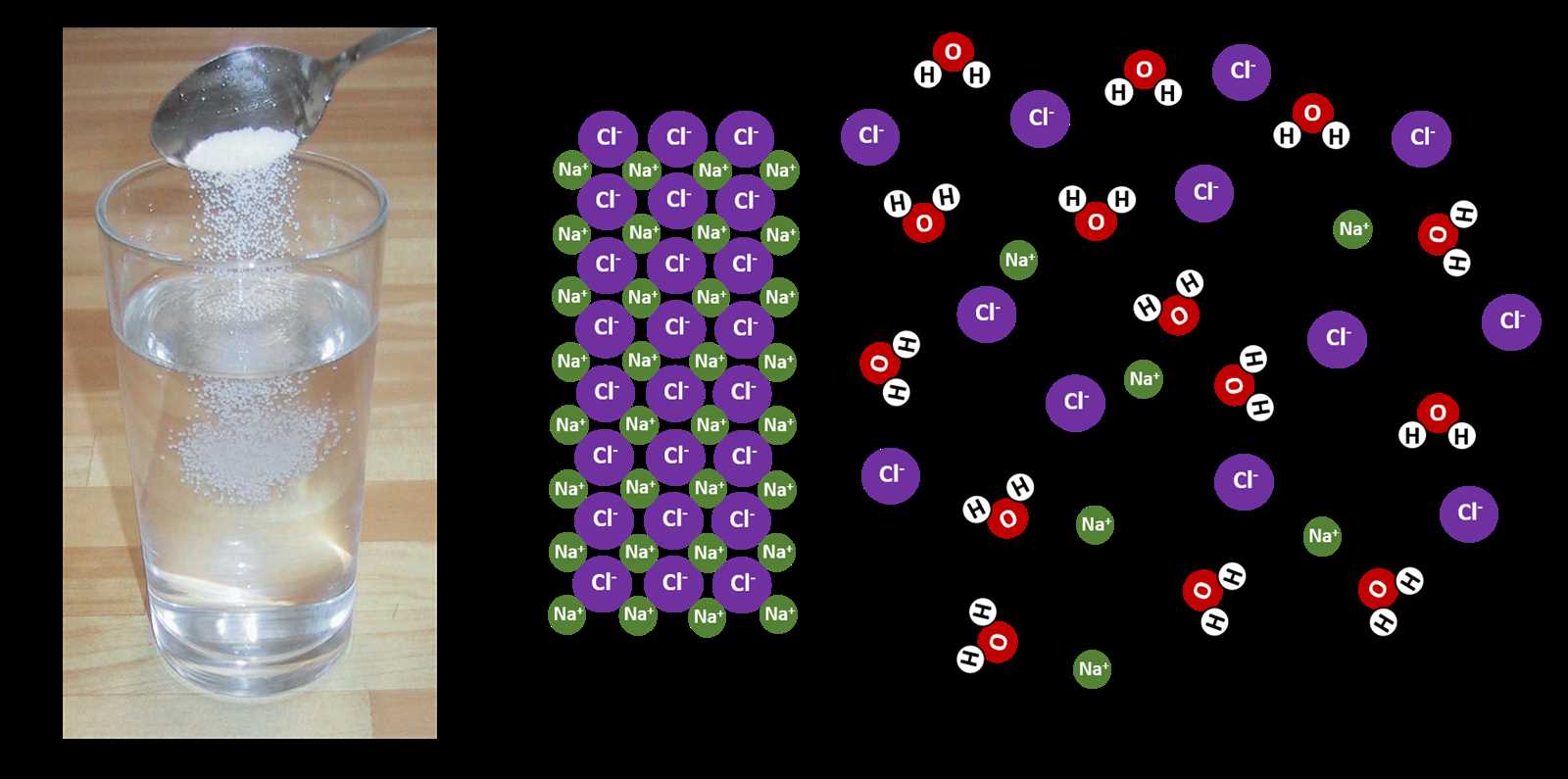
Another useful tool is creating flowcharts or step-by-step guides to outline the process for solving a particular problem. These visual aids break down the solution into smaller, more manageable parts, providing a clear roadmap for how to approach the task. By following these visual cues, learners can follow the logic of the solution more easily and avoid feeling overwhelmed by complex instructions.

Guidance for Difficult Problems

When faced with challenging problems, it’s important to approach them systematically rather than becoming overwhelmed. Breaking down complex tasks into smaller, more manageable steps is key to making progress. By following a structured problem-solving approach and utilizing various strategies, learners can navigate even the most difficult exercises with greater ease.

Here are some useful tips to help you tackle tough problems:

• Read the problem carefully: Ensure you understand all the given information before proceeding. Rephrase the problem in your own words to ensure clarity.
• Identify key concepts: Look for any concepts or formulas that may apply to the problem. Recognizing patterns and relationships can often lead to faster solutions.
• Break it down: Divide the problem into smaller steps or subproblems. Solve each part individually before attempting to solve the entire problem.
• Use visual aids: Drawing diagrams, charts, or graphs can provide clarity and help visualize the problem’s structure, making it easier to identify the next step.
• Review similar problems: Refer to examples and exercises that are similar in structure to the one you’re solving. Seeing how others were solved can provide valuable insights.

By following these strategies and remaining persistent, you can effectively solve even the most difficult problems and build a deeper understanding of the material.

How Chapter 1 Sets the Foundation for Success

The initial section of any learning material plays a crucial role in setting up the entire framework for understanding and mastery. By introducing essential concepts and fundamental techniques, it prepares learners for more advanced topics. The skills and strategies learned in this section provide the building blocks necessary for success in the subsequent lessons and exercises. Without a solid understanding of these key ideas, progress can be slow and challenging, making it difficult to build on future concepts effectively.

Establishing Fundamental Concepts

The first set of lessons introduces the basic principles that will be applied throughout the entire learning process. Mastering these foundational concepts helps ensure that learners are ready to tackle more complex problems later on. Whether it’s basic problem-solving methods, equations, or strategies, the earlier content is designed to give learners the tools they need to succeed in subsequent material.

Creating a Structured Approach to Learning

By guiding learners through logical steps and providing structured exercises, this section helps develop a methodical approach to tackling challenges. Students learn how to break down problems into smaller, manageable parts, creating a sense of organization and clarity. This organized approach is essential for effectively navigating more complex topics and exercises as the learning journey progresses.

Interactive Learning Tips for Course 3

Engaging with the material actively is essential to grasping new concepts effectively. Interactive learning techniques foster a deeper understanding and retention of information, especially when complex problems are involved. By using different methods to explore the content, students can enhance their learning experience and make abstract ideas more tangible. Below are some strategies for making the most out of this type of material.

Engage with Real-World Problems

One of the most effective ways to deepen understanding is by applying learned concepts to real-world situations. This not only makes the material more relevant but also helps reinforce key skills by putting them into practice. For example, when working through mathematical concepts or strategies, students can try solving problems that mimic real-life scenarios to see how these techniques work outside of the classroom.

Collaborate with Peers for Group Learning

Collaborative learning can be particularly effective in helping to solidify understanding. Working with classmates on problem-solving activities and discussing various solutions helps expose different approaches and provides the opportunity to learn from others. Group learning encourages critical thinking, and by explaining concepts to one another, students can reinforce their own understanding.

Utilize Interactive Tools and Resources

Many digital platforms offer interactive exercises and tools that allow students to explore topics in a hands-on way. Whether through simulations, quizzes, or games, these tools provide immediate feedback and make the learning process more engaging. Using interactive resources encourages students to experiment, make mistakes, and learn from them in a low-pressure environment.

Practice Regularly with Instant Feedback

Regular practice is key to mastering new material. When combined with immediate feedback, it helps students identify areas where they may be struggling and adjust their approach accordingly. Many interactive exercises offer instant results, allowing students to see their mistakes and learn from them without delay.

Track Progress and Set Goals

Keeping track of progress and setting specific learning goals can help motivate students throughout their studies. Interactive tools often come with tracking features, allowing learners to monitor their improvement over time. Setting small, achievable goals helps break down the material into manageable steps and gives students a sense of accomplishment as they work through each one.

Interactive Tip	Benefit
Real-world problem solving	Improves practical application of concepts
Group learning	Fosters critical thinking and collaboration
Interactive tools	Encourages engagement and instant feedback
Regular practice with feedback	Helps identify weak points and reinforce skills
Track progress and set goals	Motivates and organizes the learning process

Benefits of Self-Checking with the Solution Guide

Self-assessment plays a crucial role in the learning process, offering students the opportunity to independently verify their understanding and correct mistakes before seeking external help. This practice helps strengthen problem-solving skills and encourages active engagement with the material. By comparing one’s own work with a provided solution guide, learners can not only identify errors but also recognize areas for improvement and reinforce their learning.

One of the primary advantages of self-checking is the immediate feedback it provides. When learners have access to solutions, they can quickly determine whether their answers are correct or if adjustments are needed. This instant feedback helps build confidence, allowing students to feel more assured in their abilities while also highlighting the areas that require further practice.

Additionally, self-checking promotes critical thinking. By evaluating their own work, students are forced to reflect on the methods and approaches they used to reach their conclusions. This encourages deeper analysis of problem-solving strategies and the development of more efficient techniques. Over time, self-checking fosters a sense of ownership over the learning process, empowering students to take charge of their education and make informed decisions about how to improve.

Another benefit of self-checking is the ability to track progress. Regularly comparing answers with solutions enables students to observe their growth over time. This sense of progress can be highly motivating, as learners can visibly see improvements and areas where they continue to struggle. Recognizing these patterns allows students to focus their efforts on specific topics or types of problems that require more attention.

Ultimately, the process of self-checking with a solution guide encourages students to take an active role in their education. It cultivates an environment of independent learning, helping individuals develop the skills and habits necessary for long-term academic success.

Next Steps After Completing the First Section

Once the initial section has been completed, it’s important to consolidate the knowledge gained and prepare for the next stages of learning. Understanding the concepts covered thus far is crucial, as each new topic will build on the foundation laid in earlier lessons. Reflecting on what has been learned and applying it to various problem-solving situations is an effective way to strengthen comprehension and retention.

Review Key Concepts and Identify Weak Areas

Start by revisiting the key ideas and techniques covered in the first section. If any particular concepts or problems proved challenging, focus on those areas to ensure full understanding. Reworking problems and practicing with additional examples can help reinforce the material and clarify any lingering doubts.

Move on to the Next Set of Problems

After reviewing, continue with the next set of exercises. Moving forward helps keep the momentum of learning going and prevents stagnation. The new material will likely expand on the skills acquired so far, so approaching the next exercises with confidence will help apply the knowledge in a broader context. As always, continue using the solution guide to verify answers and correct mistakes as they arise.

By taking these steps, learners can ensure a strong foundation for the upcoming sections and remain engaged in the learning process. Continuous practice and review are essential for mastering new skills and achieving long-term academic success.