Core Connections Course 3 Chapter 9 Answer Key

The material in this section focuses on key mathematical concepts that are essential for understanding complex problem-solving strategies. As you progress, you will encounter exercises designed to challenge your critical thinking and test your grasp of advanced topics. Success in this section requires both careful analysis and consistent practice to strengthen your skills.
Mastering the exercises will provide valuable insights into various mathematical techniques. With a structured approach, each problem builds upon the previous one, allowing you to gradually enhance your ability to approach increasingly difficult tasks. The solutions presented offer clarity and reinforce the learning objectives, guiding you toward achieving a deeper understanding of the subject matter.
To make the most of this section, it is important to tackle each problem systematically and review any mistakes made during the process. This will not only help to solidify your knowledge but also prepare you for future challenges in related areas. With the right strategies and persistence, you will improve your problem-solving capabilities and develop the necessary skills for mastering the subject.
Core Connections Course 3 Overview
This section introduces fundamental principles aimed at enhancing problem-solving abilities and strengthening logical reasoning. The material is structured to build a deep understanding of essential concepts, gradually progressing in difficulty. As learners move forward, they will encounter various types of exercises that challenge both their comprehension and application skills.
The goal is to develop a solid foundation in mathematical reasoning, preparing students for more advanced topics. By working through the material, individuals are encouraged to explore different approaches to problem-solving while reinforcing their knowledge through practice. Each exercise is designed not only to test understanding but also to develop critical thinking skills necessary for real-world applications.
Adopting a systematic approach to the content ensures that learners gain a comprehensive understanding of the subject. Through consistent engagement and review, the concepts become more intuitive, enabling the learner to apply them confidently in future studies. With focus and dedication, the challenges within this section provide the necessary tools for mastering complex topics and improving problem-solving strategies.
Key Concepts in Chapter 9
This section focuses on a variety of essential principles that provide the foundation for understanding complex problem-solving strategies. The material is designed to enhance your ability to think critically and apply mathematical methods effectively. By engaging with the content, learners will refine their analytical skills and become more confident in approaching various types of problems.
Important Topics Covered

- Understanding variable relationships and their applications
- Working with algebraic expressions and equations
- Exploring geometric properties and their connections to algebra
- Solving multi-step problems using logical reasoning
Approaching Problem Solving
Mastering the concepts in this section requires a clear understanding of how different principles interact. Breaking down problems into smaller, manageable steps helps in identifying key relationships and applying the right techniques. Practice with real-world examples reinforces these strategies, making it easier to solve more complex tasks in the future.
By the end of this section, students should have a stronger grasp of the fundamental methods needed for tackling a wide range of mathematical challenges. Consistent practice and review will help ensure a thorough understanding and enable the application of these concepts in various contexts.
How to Approach Course 3 Exercises
Successfully tackling the exercises in this section requires a strategic approach that emphasizes understanding the underlying concepts and applying them effectively. Rather than rushing through problems, take the time to break each one down into smaller steps. This will help in identifying the key elements and determine the most appropriate methods for solving them.
Step-by-Step Problem Solving
- Read the problem carefully: Fully understand what is being asked before attempting to solve it.
- Identify relevant information: Highlight key data and equations that are crucial for solving the problem.
- Break the problem into parts: Work through each part of the exercise step by step, ensuring no detail is overlooked.
- Check your work: After finding a solution, review each step to verify accuracy and logic.
Building Problem-Solving Skills
As you engage with these exercises, it’s essential to focus on developing problem-solving techniques that go beyond memorization. Critical thinking is key, as is learning how to apply concepts in different contexts. With consistent practice, you’ll become more efficient in your approach, eventually leading to quicker and more accurate solutions.
Understanding Core Connections Problems
The problems presented in this section are designed to challenge your ability to think critically and apply mathematical concepts in a variety of scenarios. By examining these exercises closely, you can identify patterns and relationships that will help you solve them efficiently. The key to mastering these problems lies in breaking them down into smaller, manageable components and understanding the logic behind each step.
Analyzing Problem Structure
- Identify the main objective: Understand what is being asked and determine the required outcome.
- Look for relationships: Pay attention to how different elements of the problem are connected and how they can be used to find a solution.
- Focus on key information: Identify and isolate the most relevant details needed to solve the problem.
Developing Problem-Solving Techniques
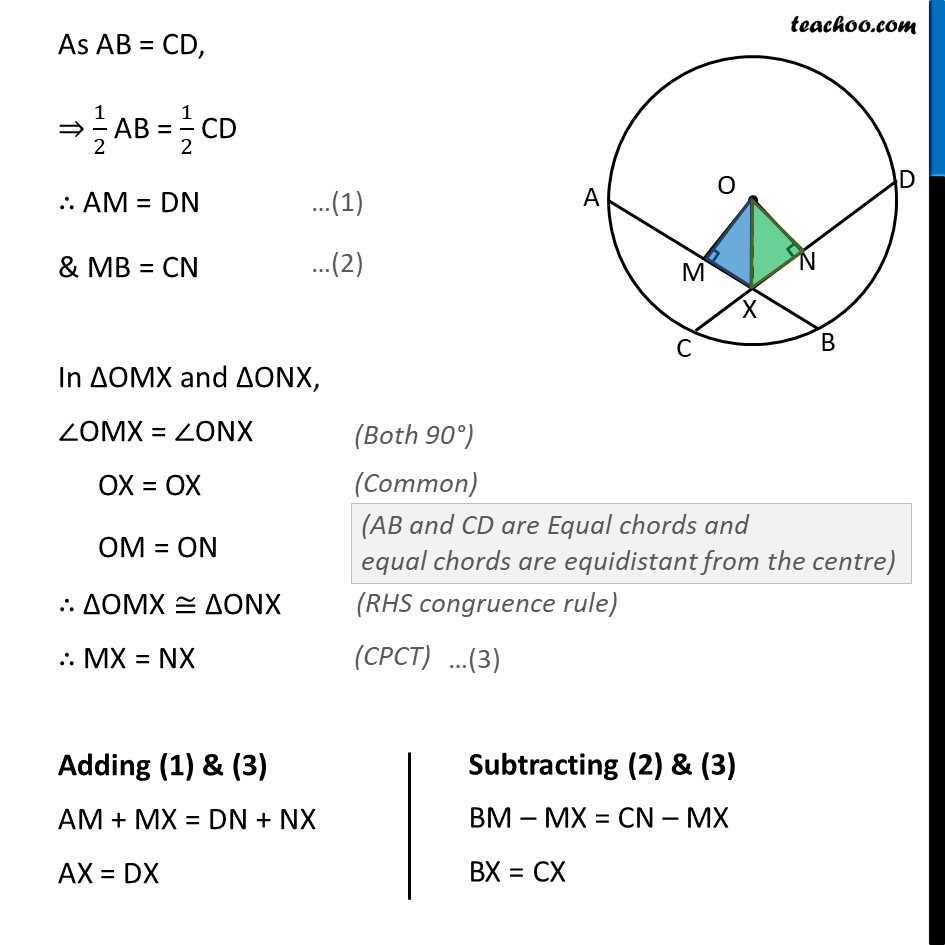
Approaching these problems with a clear and structured method is essential. Logical reasoning and critical thinking will allow you to evaluate different approaches and select the best strategy for each task. With practice, you will become more adept at recognizing common patterns and applying the right techniques to reach accurate solutions.
Tips for Solving Chapter 9 Questions
Successfully solving the problems in this section requires both a methodical approach and a clear understanding of the underlying concepts. To excel, it’s important to not just know how to perform calculations, but to also grasp the logic behind each problem. Applying effective strategies will help you navigate through the more challenging tasks with greater confidence and accuracy.
Effective Problem-Solving Strategies
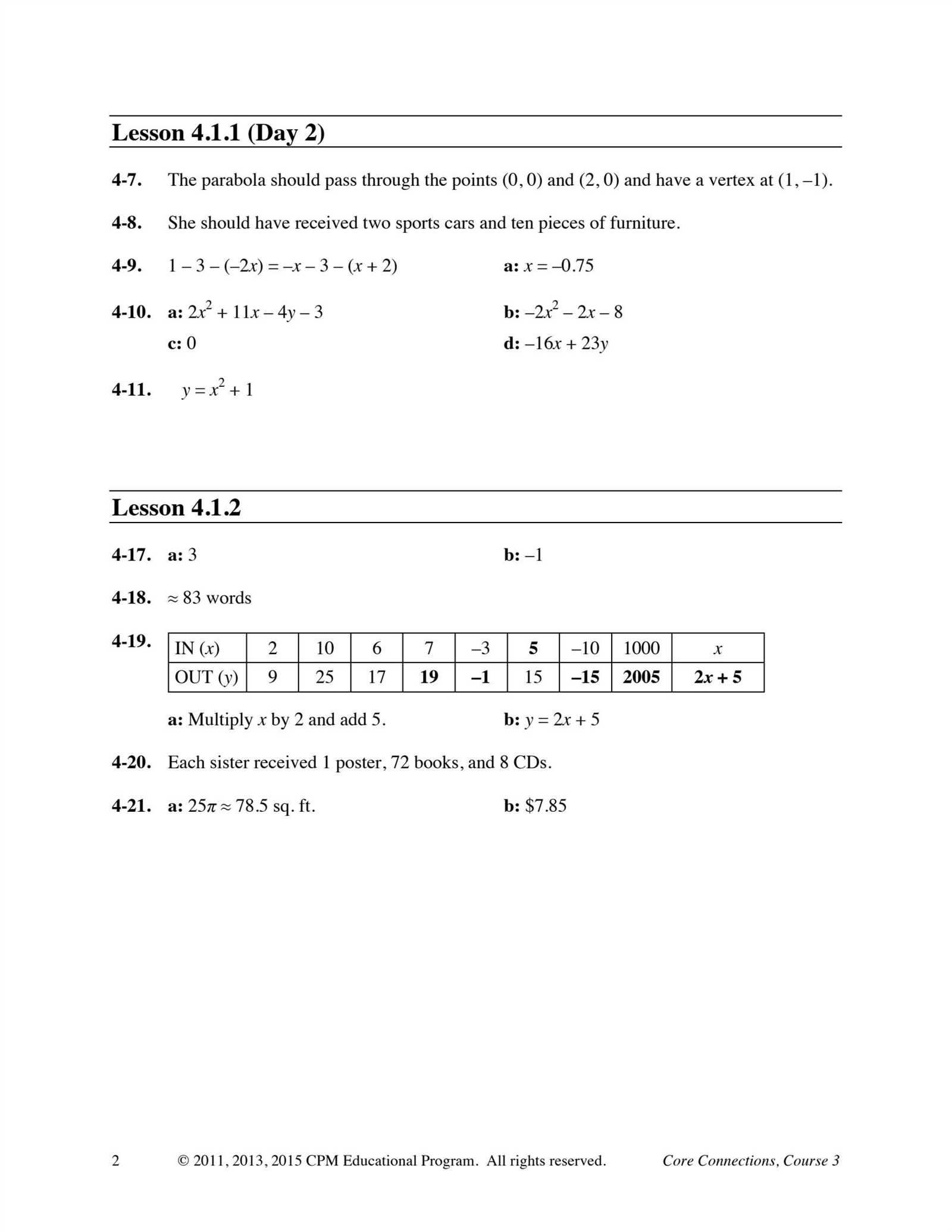
- Break it down: Divide each question into smaller, more manageable parts to simplify the process.
- Work step by step: Focus on completing one step at a time and ensure each calculation or operation is correct before moving on.
- Check for patterns: Look for recurring elements or relationships between variables that can help solve the problem more efficiently.
- Use diagrams: Visual representations can often clarify complex concepts, making it easier to identify the right approach.
Maximizing Accuracy
While working through the problems, attention to detail is critical. Double-check your calculations and verify each step to ensure that no mistakes are made along the way. With consistent practice and a focused mindset, you’ll develop the skills needed to solve even the most complex questions with ease.
Step-by-Step Problem Solving Guide
To effectively tackle problems in this section, following a structured, step-by-step approach is essential. This method helps break down complex tasks into smaller, more manageable parts, ensuring that each aspect is handled properly. By methodically working through each stage, you can enhance your problem-solving skills and reduce the likelihood of errors.
Step 1: Understand the Problem
- Read carefully: Make sure you fully comprehend what the problem is asking before you start working on a solution.
- Identify key information: Highlight the essential data and relationships needed to solve the problem.
Step 2: Develop a Plan
- Choose an approach: Based on the information you’ve gathered, decide on the best method to solve the problem.
- Break it down: Divide the solution process into smaller steps, ensuring you approach each one systematically.
Step 3: Execute and Review
- Perform calculations: Complete the necessary operations, keeping track of each step.
- Double-check your work: After reaching a solution, review each step to ensure accuracy and logic.
By following these steps, you will improve your ability to approach each problem with a clear strategy, ultimately enhancing both speed and accuracy in finding solutions.
Common Mistakes in Course 3
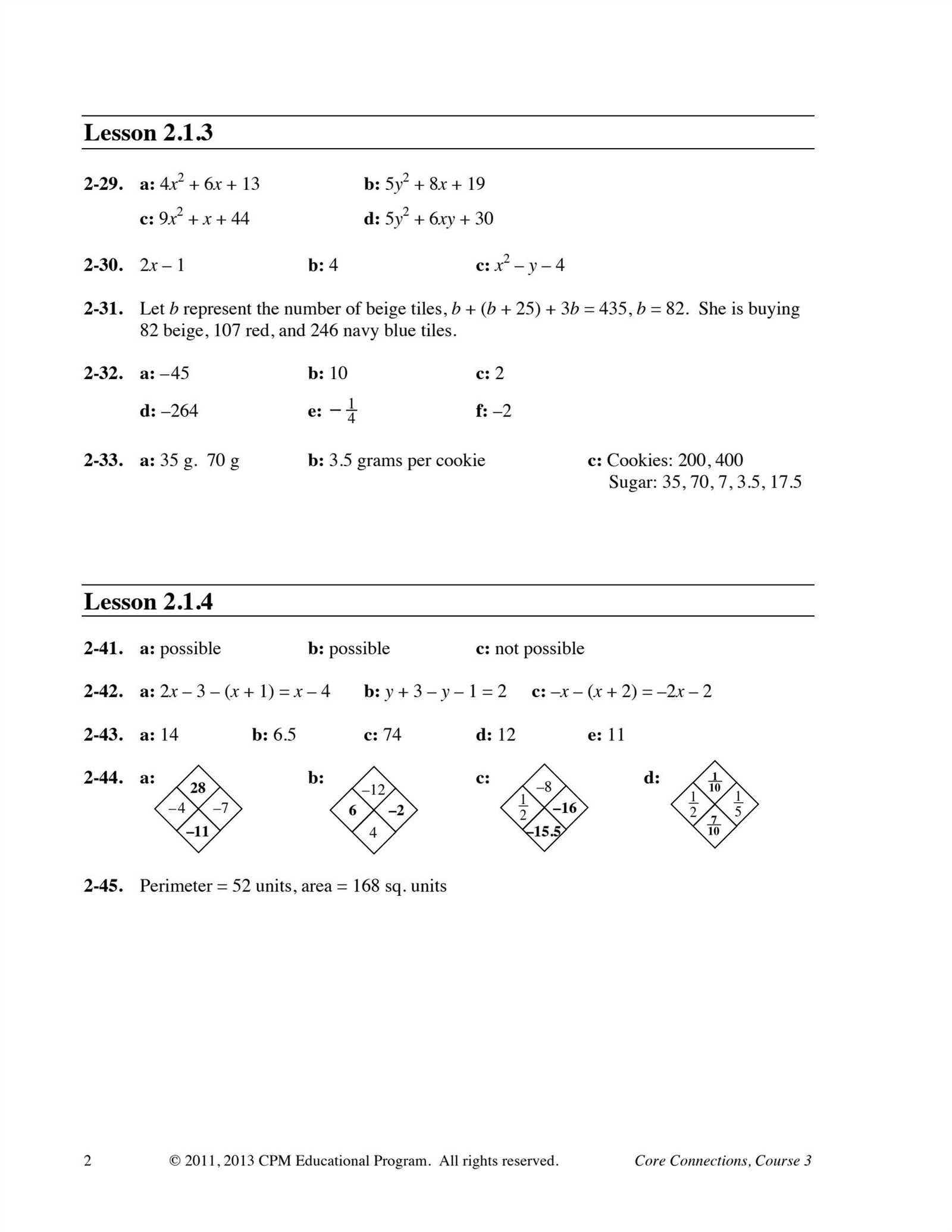
While working through complex mathematical problems, it’s easy to make certain mistakes that can lead to incorrect solutions. These errors often stem from misunderstanding key concepts, rushing through steps, or overlooking critical details. Identifying and addressing these common pitfalls can help you improve your problem-solving accuracy and efficiency.
Frequent Errors
- Skipping important steps: Rushing through calculations without carefully following each necessary step can result in mistakes.
- Misinterpreting the question: Not fully understanding what is being asked can lead to applying the wrong methods.
- Overlooking signs and symbols: Neglecting negative signs, exponents, or parentheses can lead to significant errors in calculations.
- Forgetting to check work: Failing to review each step often leads to undetected mistakes, even when the initial answer seems correct.
How to Avoid These Mistakes
- Slow down: Take the time to carefully read each question and perform calculations step by step.
- Double-check your work: Always verify the accuracy of your calculations before finalizing your solution.
- Practice regularly: The more you practice, the more familiar you become with common problem types and solutions, reducing the likelihood of errors.
By being mindful of these common mistakes and applying the right strategies to avoid them, you can enhance your understanding and improve your performance in mathematical problem-solving.
Strategies for Mastering Core Connections
To excel in solving mathematical problems, it’s essential to develop a comprehensive understanding of the concepts and techniques that will help you approach each task with confidence. Mastery comes through consistent practice, applying the right strategies, and continuously building upon your skills. By focusing on key areas and refining your approach, you can achieve better results and tackle even the most challenging exercises with ease.
Key Strategies for Success
| Strategy | Description |
|---|---|
| Understand the Basics | Ensure a strong grasp of foundational concepts before moving on to more complex problems. |
| Practice Consistently | Regularly solve problems to reinforce learning and become more familiar with various problem types. |
| Stay Organized | Break down each problem into smaller, more manageable steps to avoid confusion and mistakes. |
| Review and Reflect | After solving a problem, go over your solution to ensure accuracy and understand any errors. |
| Seek Help When Needed | Don’t hesitate to ask for assistance from peers, instructors, or online resources if you encounter difficulties. |
By following these strategies, you can build the skills needed to approach mathematical problems with confidence, ultimately mastering the techniques required for success.
Effective Time Management for Chapter 9
Managing your time effectively is crucial when working through complex problems in any section. By allocating appropriate time to each task and planning ahead, you can reduce stress and increase your productivity. A clear strategy allows you to stay focused, avoid procrastination, and ensure that you complete each exercise accurately within a reasonable timeframe.
Prioritizing Tasks
- Assess the difficulty: Start with problems that seem more manageable, saving the more challenging ones for later when you’re warmed up and focused.
- Break down large tasks: Divide difficult problems into smaller steps, so they become easier to handle and less overwhelming.
- Set clear goals: Define what you aim to achieve in each study session to stay focused and track progress.
Staying on Track

- Use a timer: Set a specific amount of time for each exercise to avoid spending too much time on any one task.
- Avoid distractions: Eliminate interruptions by finding a quiet space and turning off notifications to maintain full concentration.
- Review regularly: Take short breaks to refresh your mind, and review your work to ensure that you are staying on course.
By applying these time management strategies, you will be able to approach each task with a clear mind, complete problems efficiently, and feel confident in your progress.
Using the Answer Key Effectively
While reviewing solutions can be a helpful way to confirm your understanding, it’s important to use it as a tool for learning rather than just for checking if your answer is correct. An answer guide can be a valuable resource, but the key to mastery is in how you engage with it. Instead of simply looking up solutions when you’re stuck, use the guide to explore the reasoning behind each step and to learn the methods that lead to the correct result.
To make the most out of this resource, follow these strategies:
- Check after attempting: Try solving the problem on your own first, then refer to the guide to see if you’ve followed the right approach.
- Understand the process: Study the steps provided in the solution and try to grasp the logic behind each one. This deepens your understanding and helps in solving similar problems in the future.
- Identify mistakes: If your solution is incorrect, use the guide to pinpoint where you went wrong and focus on that specific part to avoid repeating the error.
- Practice with variations: Modify the problem slightly and solve it again using the same process. This ensures you grasp the concept and aren’t relying solely on memorization.
By following these strategies, you will not only improve your accuracy but also enhance your problem-solving skills for future challenges.
How to Check Your Work
Reviewing your solutions carefully is an essential part of mastering any subject. Ensuring the accuracy of your results involves more than just looking at the final answer–it’s about verifying each step and understanding how you reached the conclusion. This process helps prevent mistakes, solidifies your understanding, and improves your problem-solving techniques for future tasks.
Here are effective ways to double-check your work:
- Revisit the problem: Before reviewing your solution, re-read the original problem to make sure you fully understand what was asked.
- Review your steps: Go through each step of your process and ensure that you followed the correct methods. Look for any skipped steps or miscalculations.
- Check the units: If applicable, verify that the units in your solution match the units in the problem statement.
- Cross-check with similar problems: Compare your solution with examples you’ve already solved. If your method and reasoning match, you’re more likely on the right track.
- Verify calculations: Double-check any arithmetic, algebraic manipulations, or logical steps for mistakes.
By incorporating these practices into your routine, you can significantly reduce errors, strengthen your comprehension, and become more efficient at solving problems.
Common Pitfalls in Answering Problems
When tackling problems, it’s easy to fall into certain traps that can lead to incorrect solutions. These common mistakes often stem from rushing through the task, misinterpreting the problem, or overlooking important details. Identifying and avoiding these pitfalls is key to improving your accuracy and efficiency when solving complex exercises.
Here are some frequent errors to watch out for:
| Pitfall | Description |
|---|---|
| Misreading the question | Overlooking key information or failing to understand the problem’s requirements can lead to incorrect approaches or answers. |
| Skipping steps | Rushing through calculations without showing all necessary steps may result in missing important details or making errors. |
| Overcomplicating solutions | Attempting overly complex methods when simpler solutions exist can cause confusion and lead to mistakes. |
| Not reviewing work | Failing to check your work thoroughly can allow small mistakes to go unnoticed, especially in multi-step problems. |
| Neglecting units | For problems involving measurements or calculations, ignoring units can lead to incorrect answers or mismatched results. |
By staying mindful of these common pitfalls and practicing careful attention to detail, you can improve your problem-solving skills and reduce the likelihood of making these errors in the future.
Additional Resources for Effective Learning
Enhancing your understanding of complex topics often requires more than just textbook exercises. Utilizing supplementary materials can provide deeper insights, offer alternative explanations, and reinforce key concepts. Exploring additional resources can help clarify difficult ideas and offer fresh perspectives on problem-solving techniques.
Online Platforms and Tutorials
There are numerous educational websites that offer video tutorials, interactive lessons, and forums for discussion. These platforms often break down concepts step-by-step, making them easier to understand. Some popular platforms include:
- Khan Academy: Offers in-depth explanations and practice problems across various subjects.
- Coursera: Provides online courses from universities and colleges around the world.
- EdX: Another online learning platform offering university-level courses and materials.
Study Groups and Peer Assistance
Collaborating with others can also be an effective way to learn. Join study groups where you can discuss challenging topics, exchange strategies, and solve problems together. Peer feedback often helps in identifying gaps in knowledge and improves overall comprehension.
By leveraging these external resources, you can reinforce your learning and improve your problem-solving abilities. Make sure to explore multiple sources to get a well-rounded understanding of the material.
Study Techniques for Chapter 9
Mastering complex concepts requires a focused approach to studying. To effectively learn the material in this section, it’s important to apply specific strategies that promote deep understanding and retention. Different techniques can cater to various learning styles, ensuring that you grasp key ideas and can apply them with confidence.
Here are some methods to help you prepare effectively:
- Active Recall: Instead of passively rereading notes, try to actively recall information without looking at your materials. This strengthens memory retention and helps identify areas that need more attention.
- Practice Problems: Solving related problems repeatedly allows you to reinforce learned concepts and apply them in various contexts. Focus on both simple and challenging problems to build confidence.
- Chunking Information: Break down complex ideas into smaller, manageable parts. This helps in understanding the material step by step and makes it easier to remember.
- Teach Someone Else: Explaining concepts to a peer or study partner forces you to clarify your own understanding and can uncover any gaps in your knowledge.
- Use Visual Aids: Diagrams, charts, and mind maps can simplify complex relationships between concepts, making them easier to understand and recall during assessments.
By incorporating these techniques into your study routine, you’ll be better equipped to tackle the material in this section and achieve a stronger grasp of the concepts presented. Practice consistency and review regularly to maximize your learning experience.
Real-Life Applications of Course 3
The concepts covered in this section have significant practical value in everyday life and various professional fields. Understanding these principles not only prepares you for exams but also equips you with skills that are applicable in real-world situations. From problem-solving in business to applying mathematical reasoning in engineering, the knowledge gained can be invaluable in both academic and career pursuits.
Here are some real-life applications where the material from this section can be useful:
- Business and Economics: Many concepts relate directly to financial analysis, such as understanding trends, calculating profits, and optimizing resources for maximum efficiency.
- Engineering: Whether it’s designing structures, coding software, or optimizing systems, the ability to break down complex problems into smaller, solvable parts is crucial in engineering fields.
- Data Science: The ability to analyze patterns, make predictions, and evaluate data trends are skills that are fundamental in the field of data science and statistical analysis.
- Healthcare: Mathematical models are often used to predict disease outbreaks, calculate dosages, and analyze patient data for better treatment plans and outcomes.
- Personal Finance: Learning how to manage finances, invest wisely, and track expenses involves using mathematical concepts that you will encounter in this section.
By seeing how these ideas can be applied in various fields, you can appreciate the relevance and importance of mastering them, not just for academic success but for real-world problem-solving as well.
Improving Problem-Solving Skills
Enhancing your ability to solve problems effectively is an essential skill that can be applied to many aspects of life. Whether you’re tackling mathematical questions, making decisions at work, or addressing personal challenges, a systematic approach to problem-solving can help you reach solutions more efficiently. Developing strong problem-solving skills involves understanding the problem, breaking it down into manageable steps, and applying logical reasoning to find the best solution.
Here are several strategies to improve your problem-solving abilities:
- Understand the Problem: Take the time to thoroughly read and understand what is being asked. Clarify any uncertainties before jumping to solutions.
- Break It Down: Divide complex problems into smaller, more manageable parts. Solving each part step-by-step makes the overall task easier.
- Think Critically: Look at the problem from different angles. Ask yourself questions like “What do I know?” and “What needs to be figured out?”
- Use Visual Aids: Diagrams, charts, or lists can help visualize the problem and organize your thoughts. Sometimes, a visual representation makes the solution clearer.
- Practice Regularly: Like any skill, problem-solving improves with practice. Challenge yourself with different types of problems to strengthen your abilities.
By continuously practicing these methods and applying them in various situations, you can sharpen your problem-solving skills and become more effective at finding solutions in both academic and real-world contexts.