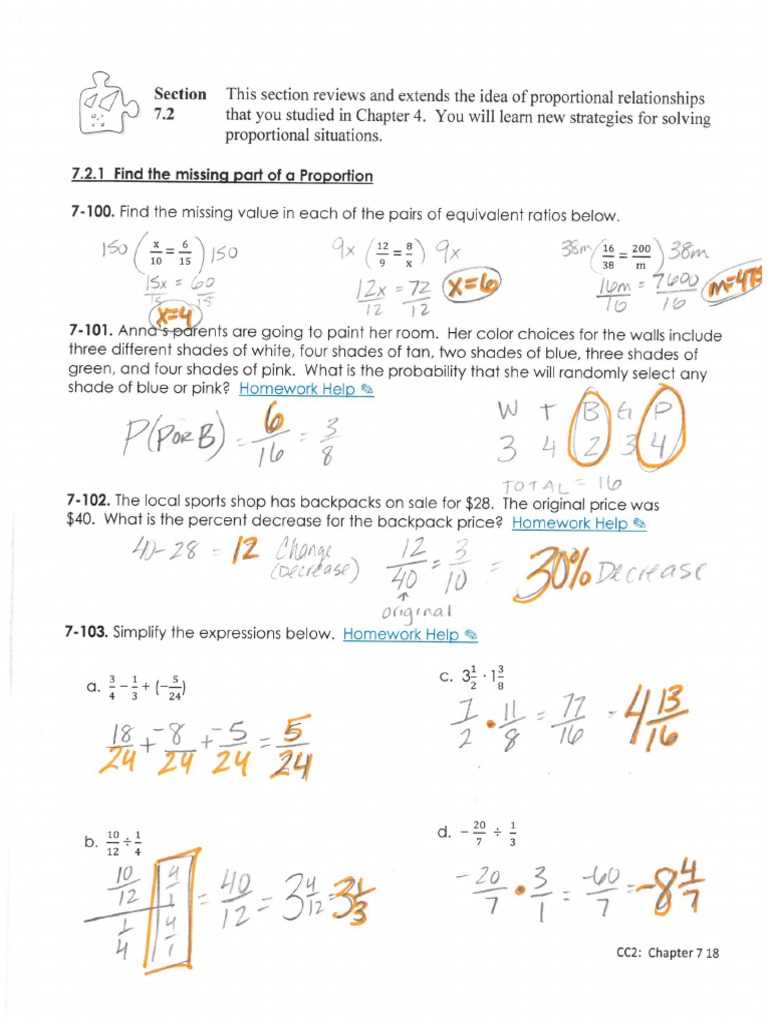
Course 2 Chapter 2 Percent Answer Key

In this section, we explore the fundamental principles behind calculating portions, ratios, and other related concepts. Understanding these mathematical ideas is essential for solving various real-life problems, from finance to daily measurements. By building a strong foundation, you’ll be able to tackle more advanced topics with confidence.

Effective problem-solving requires clear strategies, and in this guide, you’ll find practical examples that break down complex challenges into manageable steps. Whether it’s finding a part of a whole, determining increases or decreases, or converting between forms, this resource will help you navigate each calculation smoothly.

Through a variety of exercises, you’ll gain the skills needed to approach these mathematical tasks with ease and accuracy. The solutions provided offer insight into how to apply the core techniques to different scenarios, ensuring you’re well-prepared for both academic and practical applications.

Course 2 Chapter 2 Percent Answer Key

This section provides detailed solutions to the various problems related to calculating portions and ratios. By breaking down each problem step by step, you’ll gain a clearer understanding of how to solve similar challenges. The solutions offered here will guide you through the process, ensuring that you grasp the key concepts and apply them confidently in practice.

Understanding Key Concepts

Before diving into the solutions, it’s important to understand the basic principles behind the problems. In many cases, you will be asked to find a fraction of a whole or determine how one quantity relates to another. This could involve calculating increases or decreases, or converting between percentages and fractions.

Step-by-Step Solutions

The following table shows how each problem can be solved with the appropriate methods. The calculations are explained clearly, allowing you to follow the logic and apply the same steps to similar tasks.

Problem	Solution	Explanation
Find 25% of 200	50	Multiply 200 by 0.25 (200 * 0.25 = 50)
Increase 150 by 20%	180	Multiply 150 by 1.20 (150 * 1.20 = 180)
What is 30% of 500?	150	Multiply 500 by 0.30 (500 * 0.30 = 150)

By following these examples, you can develop a solid understanding of how to handle different types of problems involving portions, increases, and other related concepts. Practicing these methods will allow you to approach more complex problems with confidence.

Understanding Percent Calculations

Grasping the concept of calculating portions and ratios is crucial for solving a wide range of mathematical problems. Whether determining the share of a total amount or calculating changes, these skills are essential for both academic tasks and real-life applications. In this section, we’ll break down the process to make it easier to understand and apply in various contexts.

Key Concepts of Ratio Calculations

To solve problems involving proportions, you need to understand the relationship between a part and the whole. This typically involves the following principles:

• Fractions: Expressing a part of a whole as a fraction.
• Conversions: Changing between different forms, like from a fraction to a decimal or from a decimal to a percentage.
• Proportions: Comparing two parts relative to each other.

Common Methods for Solving Problems

There are several strategies you can use to solve these types of problems effectively. Some of the most common methods include:

1. Direct Calculation: Multiply the total amount by the fraction or decimal equivalent.
2. Percentage Increase/Decrease: Apply the percentage increase or decrease to the original number.
3. Proportional Relationships: Use ratios to compare two quantities and find the missing value.

By mastering these methods, you’ll be able to handle a variety of problems involving portions, comparisons, and changes in quantity. Whether it’s for financial calculations, statistical analysis, or other practical tasks, these skills will serve you well in many situations.

Step-by-Step Solutions for Percent Problems

Breaking down complex problems into simple steps makes them easier to understand and solve. By following a clear method, you can tackle various calculations involving parts of a total, increases, and reductions. This section will guide you through solving typical problems with a systematic approach that you can apply to similar situations.

Below is a table demonstrating how to solve some common problems using a step-by-step method. Each example is explained clearly to ensure you can follow the logic and replicate it for other calculations.

Problem	Steps	Final Solution
Find 30% of 250	1. Convert 30% to a decimal (0.30) 2. Multiply 250 by 0.30	75
Increase 400 by 15%	1. Convert 15% to a decimal (0.15) 2. Multiply 400 by 1.15	460
What is 25% of 1200?	1. Convert 25% to a decimal (0.25) 2. Multiply 1200 by 0.25	300

By following the steps outlined in the table, you’ll be able to solve similar problems with ease. The process involves first converting the percentage to a decimal and then applying simple multiplication to find the result. Practice these steps, and you’ll become more comfortable handling different types of calculations involving portions and changes in value.

Common Percent Mistakes to Avoid

When solving problems related to portions, fractions of a whole, and relative comparisons, it’s easy to make mistakes that can lead to incorrect results. Recognizing and avoiding common errors will help ensure your calculations are accurate. In this section, we’ll highlight frequent mistakes and provide tips on how to avoid them in your calculations.

Misinterpreting the Percentage

A common mistake is misinterpreting the percentage and how it relates to the total amount. For instance, confusing “50% of 200” with “50% more than 200” can lead to a significant error in calculation. Always ensure you understand whether the percentage is meant to represent a portion of the total or an increase/decrease relative to it.

Incorrect Decimal Conversion

Another frequent error occurs when converting percentages to decimals. Remember, to convert a percentage to a decimal, divide by 100. For example, 25% becomes 0.25, not 2.5. Misplacing the decimal point can cause incorrect results, especially in larger calculations.

By staying aware of these common mistakes, you’ll be able to handle percentage-based problems with more confidence and accuracy. Always double-check your conversions and understand the context of the problem before proceeding with your calculations.

Tips for Mastering Percent Concepts

Mastering the concept of calculating proportions, ratios, and relative values can significantly enhance your problem-solving skills. By focusing on key strategies and practicing consistently, you can build a solid understanding and tackle these challenges with confidence. Here are some practical tips to help you become proficient in these calculations.

Practice with Real-Life Examples

One effective way to grasp these concepts is by applying them to everyday situations. Try calculating discounts, sales tax, or changes in prices when shopping. Real-life examples provide context and make abstract concepts more tangible and easier to understand.

Break Down Complex Problems

When faced with a challenging problem, break it down into smaller, manageable steps. Start by identifying the total value, then figure out the proportion or change. Simplifying the problem into smaller parts reduces the chance of mistakes and helps you focus on one task at a time.

By following these tips and practicing regularly, you’ll gain the confidence to handle more advanced problems and apply these skills in practical scenarios. Developing a strong understanding of how to work with ratios and comparisons will serve you well in both academic and real-world applications.

How to Convert Decimals to Percentages

Converting decimals to percentages is a straightforward process that can be easily mastered with a few simple steps. This is an essential skill, as percentages are often used in various contexts, including finance, statistics, and everyday calculations. Below is a clear explanation of how to convert decimal values into percentage form.

Steps for Conversion

To change a decimal to a percentage, follow these basic steps:

1. Multiply the decimal by 100: This step shifts the decimal point two places to the right.
2. Add the percent sign (%): After multiplying, place the percentage symbol to show that the value represents a percentage.

Examples of Conversion

Here are some examples to demonstrate the conversion process:

• 0.45 becomes 45% (0.45 * 100 = 45)
• 0.03 becomes 3% (0.03 * 100 = 3)
• 1.25 becomes 125% (1.25 * 100 = 125)

By following these steps, you can easily convert any decimal to a percentage. With practice, this skill will become second nature, enabling you to handle a wide variety of problems involving proportions and comparisons.

Solving Word Problems Involving Percentages

Word problems often require translating real-life situations into mathematical expressions. These problems commonly involve calculating portions, changes in value, or comparing amounts. By breaking down the problem into smaller, manageable steps, you can apply mathematical concepts to find the solution efficiently. This section will guide you through the process of solving word problems related to relative values and quantities.

Identifying Key Information

The first step in solving any word problem is to carefully read the problem and identify the essential details. Look for the total amount, the percentage or ratio being discussed, and the question being asked. Once you have this information, you can begin to structure the problem mathematically.

Setting Up the Equation

After identifying the key information, the next step is to set up an equation. Here’s how you can approach this:

• Identify the total amount: This is often the starting point in most problems.
• Convert the percentage: If the problem gives you a percentage, convert it into a decimal by dividing by 100.
• Set up the equation: Multiply the total amount by the decimal value or use the proportional relationship.

Once the equation is set up, solve for the unknown value. Carefully follow the steps, and you will have your solution. Practicing with different types of word problems will help you gain confidence and improve your problem-solving skills.

Understanding Percent Increase and Decrease

When values change over time or due to varying factors, it is important to understand how much they have increased or decreased in relation to their original amount. This concept is essential for calculating changes in prices, salaries, populations, and many other situations. Learning how to determine the rate of change, whether an increase or a decrease, helps you evaluate the extent of these shifts effectively.

Calculating Percent Increase

To calculate a percentage increase, follow these steps:

1. Find the difference: Subtract the original value from the new value.
2. Divide by the original value: Take the difference and divide it by the initial value.
3. Multiply by 100: To convert the result to a percentage, multiply by 100.

Calculating Percent Decrease

The method for calculating a decrease is very similar to the increase. Here’s how you can do it:

1. Find the difference: Subtract the new value from the original value.
2. Divide by the original value: Divide the difference by the initial value.
3. Multiply by 100: To express the result as a percentage, multiply by 100.

Understanding these calculations allows you to assess how much an amount has grown or shrunk, which is useful in a wide range of practical situations, from budgeting to investment analysis.

Practical Examples of Percent Applications

Understanding how to work with relative values and ratios is essential for solving everyday problems. These concepts are used across various fields, from finance to shopping, and can help you make informed decisions based on real-world data. Below are a few practical examples where these skills are commonly applied.

Discounts and Sales

One of the most common applications is calculating discounts during sales. Retailers often offer a percentage off the original price, and knowing how to calculate this can save you money.

• Example: A $50 item with a 20% discount costs $40 after the discount (50 – (50 * 0.20)).
• Example: A $200 product with a 15% discount is reduced to $170 (200 – (200 * 0.15)).

Interest Rates and Loans

Another common use of relative value calculations is in understanding interest rates, which are often expressed as a percentage of the principal amount.

• Example: A $1,000 loan with a 5% annual interest rate will accrue $50 in interest after one year (1000 * 0.05).
• Example: If you invest $2,000 at an interest rate of 3%, the annual interest earned will be $60 (2000 * 0.03).

Tax Calculations

Sales tax is usually calculated as a percentage of the total price. Understanding how to calculate tax is important when making purchases.

• Example: A $100 purchase with a 7% sales tax will have an additional $7 in tax (100 * 0.07), making the total cost $107.
• Example: A $200 meal bill with an 8% tax will add $16 to the total (200 * 0.08), for a total of $216.

By mastering these applications, you can make more informed decisions when shopping, investing, or managing finances, ultimately saving you time and money in the long run.

How to Find Percentages of Numbers

Finding a portion of a number based on a given rate is a fundamental skill used in many daily activities. Whether you’re calculating sales tax, tips, or determining discounts, knowing how to compute the fraction of a total amount is essential. This process can be broken down into simple steps to help you quickly solve problems involving relative quantities.

Step-by-Step Guide

To calculate a specific portion of a total value, follow these steps:

• Step 1: Convert the percentage to a decimal. To do this, divide the percentage by 100.
• Step 2: Multiply the total amount by the decimal value.
• Step 3: The result is the portion of the total that corresponds to the percentage.

Example Calculations

Let’s go through a couple of examples to better understand how this works:

• Example 1: To find 20% of 150, first convert 20% to 0.20. Then, multiply 150 by 0.20: 150 * 0.20 = 30. So, 20% of 150 is 30.
• Example 2: To find 5% of 200, convert 5% to 0.05. Then, multiply 200 by 0.05: 200 * 0.05 = 10. So, 5% of 200 is 10.

By using this method, you can easily find any percentage of a number, making it a valuable tool for many real-life calculations.

Interactive Tools for Learning Percentages

Learning to work with relative values can be challenging, but using interactive tools can make the process much easier and more engaging. These resources allow learners to visualize concepts, practice calculations, and gain a deeper understanding of how to work with proportions and ratios. From online calculators to engaging games, interactive tools offer a hands-on approach to mastering these skills.

Many websites and apps offer step-by-step tutorials, quizzes, and practice exercises that guide learners through different scenarios. These platforms often include immediate feedback, helping to identify areas of improvement and reinforce understanding. Whether you’re a beginner or looking to refine your skills, interactive resources can make the learning process more effective and enjoyable.

By taking advantage of these tools, students and learners can improve their ability to calculate, solve problems, and apply their knowledge to real-world situations with greater confidence.

Visual Aids for Percent Understanding

Using visual aids is a powerful way to enhance comprehension when dealing with relative quantities and fractions of a whole. Graphs, charts, and diagrams help to illustrate abstract concepts, making them easier to grasp. These tools provide learners with a clearer picture of how values change in relation to each other, and can significantly improve understanding and retention.

For example, pie charts are often used to show how a portion relates to the total, while bar graphs can compare different values or show increases and decreases. Visual aids can also include number lines, fraction strips, and color-coded diagrams that break down complex calculations into manageable steps. By presenting information in a visual format, learners can better visualize the connections between numbers, improving both their understanding and their ability to solve related problems.

Incorporating these visual tools into practice can help build a stronger foundation for solving problems involving ratios, comparisons, and proportional reasoning, ultimately making the learning experience more accessible and engaging.

Why Percentages Are Important in Math

Understanding relative values and ratios is a fundamental aspect of mathematics, as it allows individuals to analyze and compare different quantities effectively. These concepts are essential for making sense of various real-world scenarios, from calculating discounts to interpreting data and making financial decisions. By grasping how one value relates to another, students can enhance their problem-solving skills and apply mathematical reasoning in diverse contexts.

Real-Life Applications

The ability to work with proportions is invaluable in many areas, such as economics, statistics, and everyday financial calculations. For example, when shopping, people use this knowledge to determine savings during sales, calculate tips, or understand tax rates. Similarly, understanding changes in values over time, such as growth rates or depreciation, relies heavily on this concept.

Mathematical Significance

In math, understanding ratios and their percentage forms simplifies many complex problems. These concepts help streamline equations and allow for a more efficient approach to solving problems, whether it be for schoolwork or practical use in other subjects like science or engineering. With a strong grasp of percentages, students can enhance their ability to think analytically and tackle more advanced topics confidently.

By learning to work with percentages, individuals not only improve their academic performance but also gain valuable skills for their daily life and professional endeavors.

Common Percent Formulas You Should Know

Being familiar with key mathematical expressions is essential for solving problems related to ratios, comparisons, and relative quantities. Understanding how to apply these formulas allows you to approach problems with more clarity and efficiency. In this section, we’ll explore the most commonly used equations that involve percentages and how they can be applied to various situations.

Basic Percentage Formula

The basic formula for calculating a percentage is:

Percentage = (Part ÷ Whole) × 100

This formula allows you to determine what percentage a part represents of the whole. For example, if you have 50 apples out of 200 total, you can find what percentage 50 represents of 200 by using this formula. In this case, the calculation would be: (50 ÷ 200) × 100 = 25%. This simple equation is one of the building blocks for more complex calculations.

Finding the Part from the Percentage

If you know the percentage and the whole amount, you can calculate the part by rearranging the formula:

Part = (Percentage × Whole) ÷ 100

For example, if you need to find 30% of 150, you would use the formula as follows: (30 × 150) ÷ 100 = 45. This is useful when you are given a specific percentage and need to calculate the actual portion it represents.

Mastering these basic formulas is crucial for solving a wide range of mathematical problems involving percentages, and they form the foundation for more advanced applications in various fields.

Ways to Practice Percent Calculations

Mastering the concept of relative quantities and their calculations requires consistent practice and application in various scenarios. By working through different methods and exercises, you can strengthen your understanding and enhance your ability to perform these calculations efficiently. In this section, we will explore effective ways to practice and improve your skills in calculating percentages.

Using Online Tools and Calculators

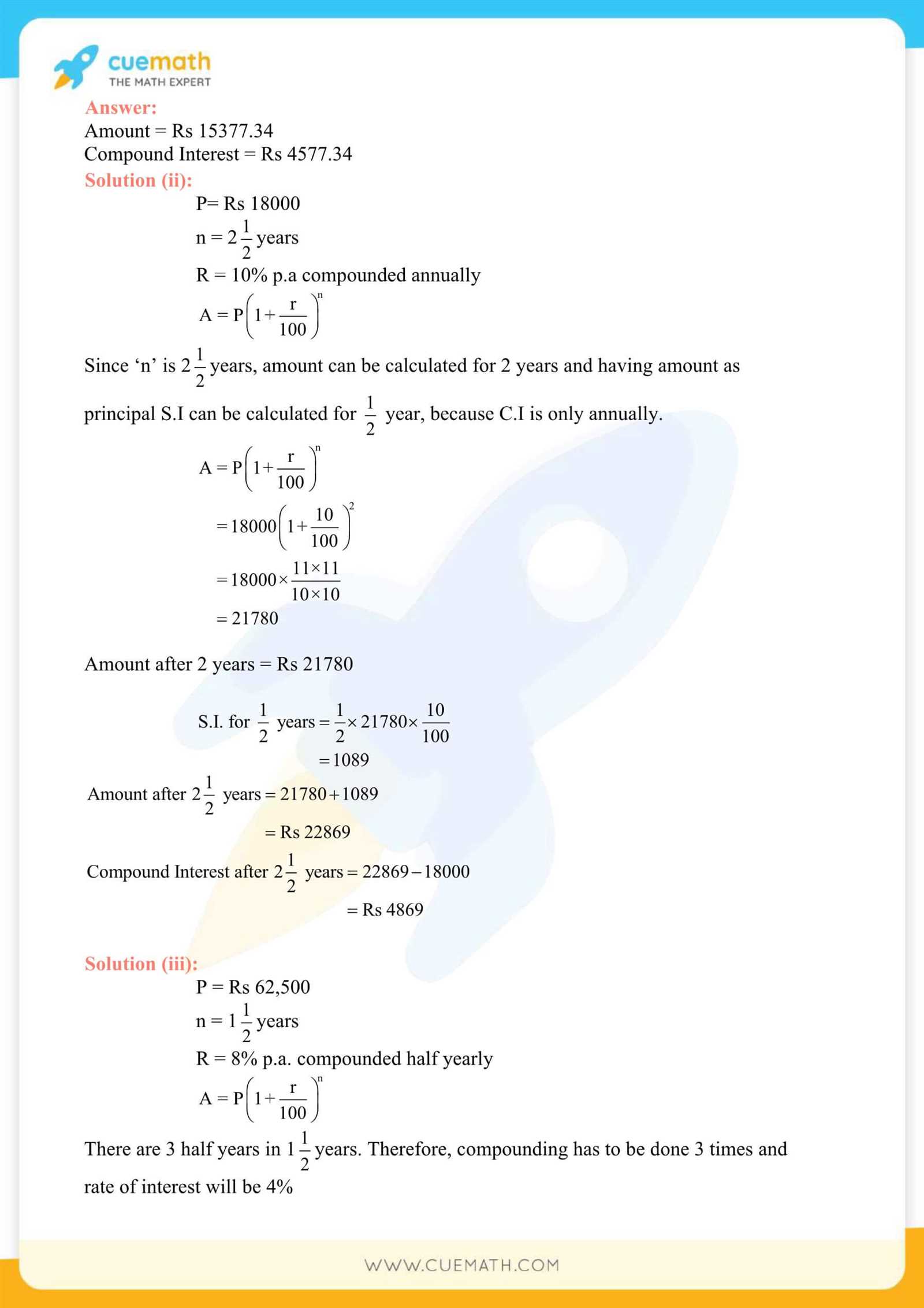
One of the easiest ways to practice is by using interactive tools and online calculators. Many websites provide percentage calculators that allow you to input different values and see immediate results. This can help you better understand the relationships between different numbers and visualize how percentages change when values are modified. Additionally, some tools offer exercises and quizzes, giving you instant feedback to track your progress.

Working with Real-Life Scenarios

Applying percentage calculations to everyday situations is an excellent way to reinforce your learning. Try calculating discounts when shopping, determining tax rates on purchases, or even figuring out the percentage increase in your savings over time. These real-life examples help you see the practical application of the concept and develop a deeper understanding of its relevance.

By practicing regularly using both digital tools and real-life examples, you’ll not only improve your speed and accuracy but also gain confidence in using percentage calculations in any situation.

How Percentages Relate to Fractions and Decimals

Understanding the relationship between different numerical forms is essential when it comes to mastering basic arithmetic concepts. Percentages, fractions, and decimals are often used interchangeably in calculations, and knowing how to convert between these forms can simplify many math problems. Each representation conveys the same information, but in different formats, making it crucial to understand how they are connected and how to work with them effectively.

Percentages can be thought of as a specific way to express a fraction or decimal in relation to 100. For example, 50% is the same as the fraction 1/2 or the decimal 0.5. This makes it easier to compare values across different mathematical expressions. To convert between these forms, you simply need to multiply or divide by powers of 10, depending on whether you are moving between fractions, decimals, or percentages.

Converting Between Fractions, Decimals, and Percentages

To convert a fraction to a percentage, multiply the fraction by 100. For instance, if you have the fraction 3/4, you would calculate:

• 3 ÷ 4 = 0.75
• 0.75 × 100 = 75%

Similarly, to convert a decimal to a percentage, you multiply by 100. For example, 0.25 is equal to 25%. On the other hand, to convert a percentage back to a fraction or decimal, you divide by 100. For instance, 40% equals 40 ÷ 100 = 0.4 or 2/5 as a fraction.

Practical Uses of These Conversions

Being able to switch between percentages, fractions, and decimals is a valuable skill, especially when working with financial calculations, measurements, or even in scientific contexts. Understanding how these forms relate to one another allows for greater flexibility in solving problems and makes it easier to interpret different types of data.