​

Mathematical problem-solving can often appear overwhelming, especially when dealing with abstract concepts. However, breaking down complex questions into manageable steps can lead to clarity and confidence in reaching the correct results. This section focuses on offering detailed solutions to a variety of mathematical challenges, guiding learners through each phase of the process.

In this context, we aim to provide practical methods for addressing common problems encountered during study. By following systematic approaches and applying the appropriate principles, you will be able to verify your results and improve your problem-solving skills. Consistency and practice play a crucial role in mastering the material and ensuring accuracy in your work.

With the right strategies in place, it’s possible to identify patterns and relationships that simplify even the most complicated tasks. Through examples and clear explanations, we will help you build a deeper understanding of the subject, supporting you in achieving both short-term success and long-term mastery.

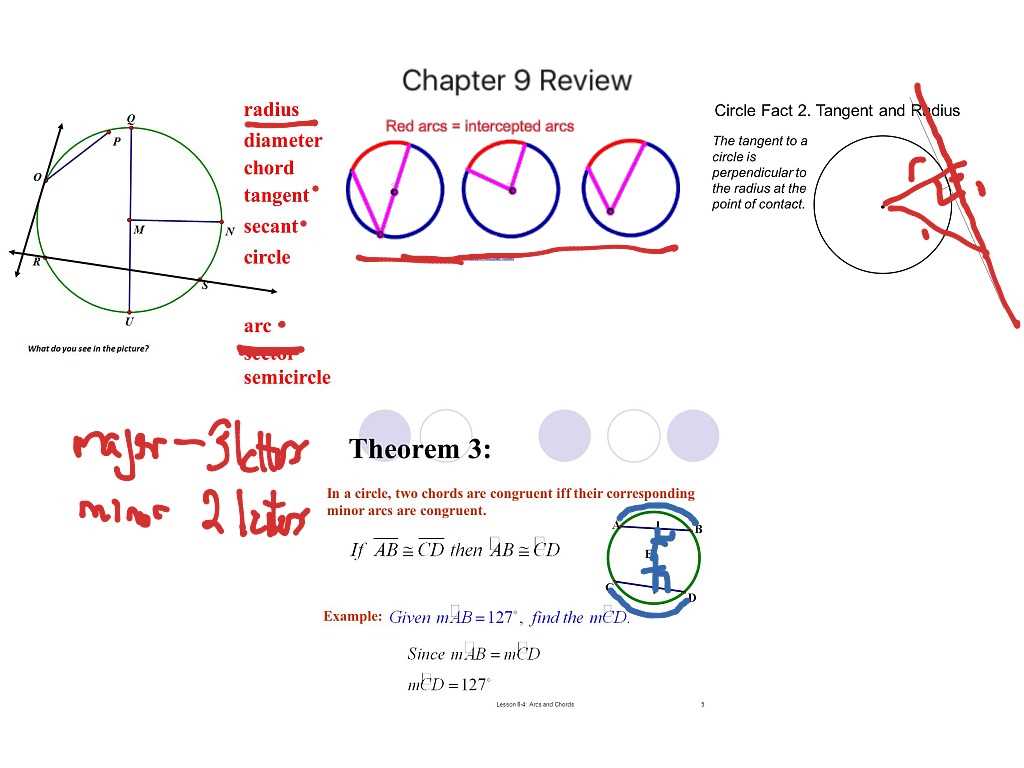
Key Concepts in Geometry Connections

Mathematics often requires the ability to recognize relationships and patterns between different elements. Understanding these fundamental principles is essential for solving complex problems effectively. In this section, we explore the core concepts that serve as the foundation for tackling various types of problems in this field. By grasping these ideas, students can approach each challenge with clarity and precision.

Understanding Relationships Between Shapes

One of the fundamental aspects of this discipline is understanding how different shapes interact with each other. Recognizing properties such as symmetry, parallelism, and proportionality allows for the development of logical strategies when solving problems. Key principles such as congruence and similarity are frequently used to establish these relationships and simplify solutions.

The Role of Logical Reasoning

Applying logical reasoning is crucial when connecting various elements of a problem. The use of theorems and proofs helps to validate assumptions and demonstrate the truth of statements within the context of the problem. This process is essential for ensuring that each solution is built on a solid, verifiable foundation.

Understanding the Answer Key Structure

The structure of a solution guide is designed to offer clarity and logical progression when reviewing results. By breaking down each problem into its components, learners can better understand how conclusions are reached and verify their own work. This section focuses on how solution frameworks are organized and how they assist in reinforcing key concepts while providing thorough explanations for each step.

Step-by-Step Breakdown

A well-organized solution typically follows a clear, step-by-step approach, where each action is explained in detail. This allows the student to trace the path from the problem’s beginning to its final resolution. By outlining intermediate steps, the structure ensures that the process is not only correct but also comprehensible, reinforcing the learning experience.

Explanation of Methods and Theorems

Each solution guide often includes references to relevant methods or theorems used to arrive at the final answer. These explanations are essential for providing a deeper understanding of the mathematical principles at play. By clarifying the rationale behind each technique, students gain insight into how to apply similar methods in different situations, improving their problem-solving skills.

Overview of Geometry Problem Types

Mathematical challenges come in various forms, each requiring different approaches to solve. These problems can vary greatly in their complexity and the strategies needed to find solutions. Understanding the different types of problems allows students to better prepare and apply the appropriate techniques. Below is an overview of common problem types encountered in this field.

Types of Problems

Mathematical problems can typically be categorized into the following types:

• Shape Properties: Problems that focus on the characteristics of various figures, such as area, perimeter, or volume calculations.
• Angle Relationships: Challenges that involve determining the relationships between different angles, such as complementary, supplementary, and vertical angles.
• Coordinate Problems: Problems that require analyzing points on a grid and finding relationships between coordinates, distances, and slopes.
• Transformations: Problems that deal with shifting, rotating, or reflecting shapes on a plane.

Common Problem Formats

In addition to different categories, problems also appear in various formats, including:

1. Proof-based: Problems that require logical reasoning and application of theorems to prove certain properties or relationships.
2. Calculation-based: Problems that involve performing specific numerical calculations to determine unknown values.
3. Construction-based: Problems that require constructing geometric figures with specific properties using basic tools like a compass or ruler.

Solving Common CPM Geometry Problems

Solving mathematical challenges requires a solid understanding of fundamental concepts and a systematic approach. In this section, we focus on some of the most common types of problems encountered during study. By examining these problems step by step, we can identify effective strategies and techniques to arrive at accurate solutions efficiently.

Approaching Shape and Area Problems

One of the most frequent tasks involves calculating the area, perimeter, or volume of various figures. To solve these problems, it is crucial to recall the appropriate formulas and understand the properties of the shapes involved. For example, when working with circles, knowing the radius and applying the formula for area (πr²) can help determine the desired value. Similarly, understanding the properties of polygons enables students to break down complex shapes into manageable parts, simplifying the calculation process.

Solving Angle Relationships

Another common problem involves determining the relationships between different angles. To solve these types of questions, it’s important to recall key theorems, such as the sum of angles in a triangle or the properties of parallel lines and transversals. By carefully applying these rules, students can systematically solve for unknown angles, ensuring that their results align with the logical structure of the problem.

Step-by-Step Guide to Solutions

Breaking down problems into manageable steps is key to finding accurate solutions. This guide offers a structured approach to solving common mathematical challenges. By following each step methodically, students can approach each question with confidence and clarity, ensuring that all elements of the problem are addressed in the correct sequence.

Step 1: Understand the Problem

Before attempting to solve a problem, it’s essential to carefully read and understand the question. Identify the known values, what needs to be determined, and any relationships that might be involved. Taking a moment to visualize the problem or sketch a diagram can often provide valuable insight into the solution approach.

Step 2: Apply Relevant Concepts and Formulas

Once the problem is fully understood, the next step is to apply the appropriate mathematical principles. This could involve recalling specific theorems, formulas, or techniques that relate to the problem at hand. Whether working with shapes, angles, or measurements, applying the correct formulas ensures that the solution path stays on track.

Understanding the Role of Diagrams

Diagrams play a crucial role in solving mathematical problems, as they provide a visual representation of the situation at hand. By translating abstract concepts into concrete images, diagrams help to clarify relationships between different elements, making it easier to identify patterns and understand the problem’s structure. Properly interpreting and creating diagrams is essential for arriving at accurate solutions.

Why Diagrams Matter

Visual aids can simplify complex problems and provide a clearer understanding of key concepts. Below are some of the ways diagrams assist in problem-solving:

• Clarifying Relationships: Diagrams allow students to visually grasp how different elements are related, such as the connection between angles, lines, and shapes.
• Breaking Down Complex Problems: A well-constructed diagram can break a problem into smaller, more manageable parts, making it easier to focus on one aspect at a time.
• Enhancing Memory and Understanding: Visual representations can improve memory retention and understanding, as they often make abstract concepts more tangible.

Creating and Interpreting Diagrams

When working with diagrams, it is important to focus on accuracy and clarity. Here are a few guidelines for creating and interpreting diagrams effectively:

1. Label Key Elements: Labeling important points, lines, and angles helps to avoid confusion and ensures that the diagram aligns with the given problem.
2. Identify Symmetry and Patterns: Recognizing symmetrical properties or geometric patterns can provide shortcuts to solving the problem more efficiently.
3. Double-Check Proportions: Ensure that measurements, angles, and distances in the diagram are proportional to the problem’s conditions to avoid errors.

How to Approach Complex Geometry Questions

Tackling complex problems requires a clear strategy and a structured approach. When faced with a difficult challenge, it’s essential to break it down into smaller, more manageable parts. By identifying key elements, understanding the relationships between them, and applying appropriate methods, the solution becomes more achievable. In this section, we will explore effective strategies for handling challenging problems.

Step-by-Step Breakdown

Approaching complex problems systematically helps to avoid confusion and ensures that all aspects of the problem are addressed. Here’s a methodical approach:

• Read the Problem Carefully: Begin by understanding the problem’s conditions. Identify what is given and what needs to be found.
• Draw a Diagram: Visualizing the problem can often provide insight into the relationships between different components. Sketching the figures helps to clarify any unclear aspects.
• Identify Known and Unknown Values: List the given values and mark the unknowns. This helps you to focus on the information that will lead to the solution.

Advanced Problem-Solving Techniques

For more intricate problems, additional techniques are necessary to simplify the process. Some approaches include:

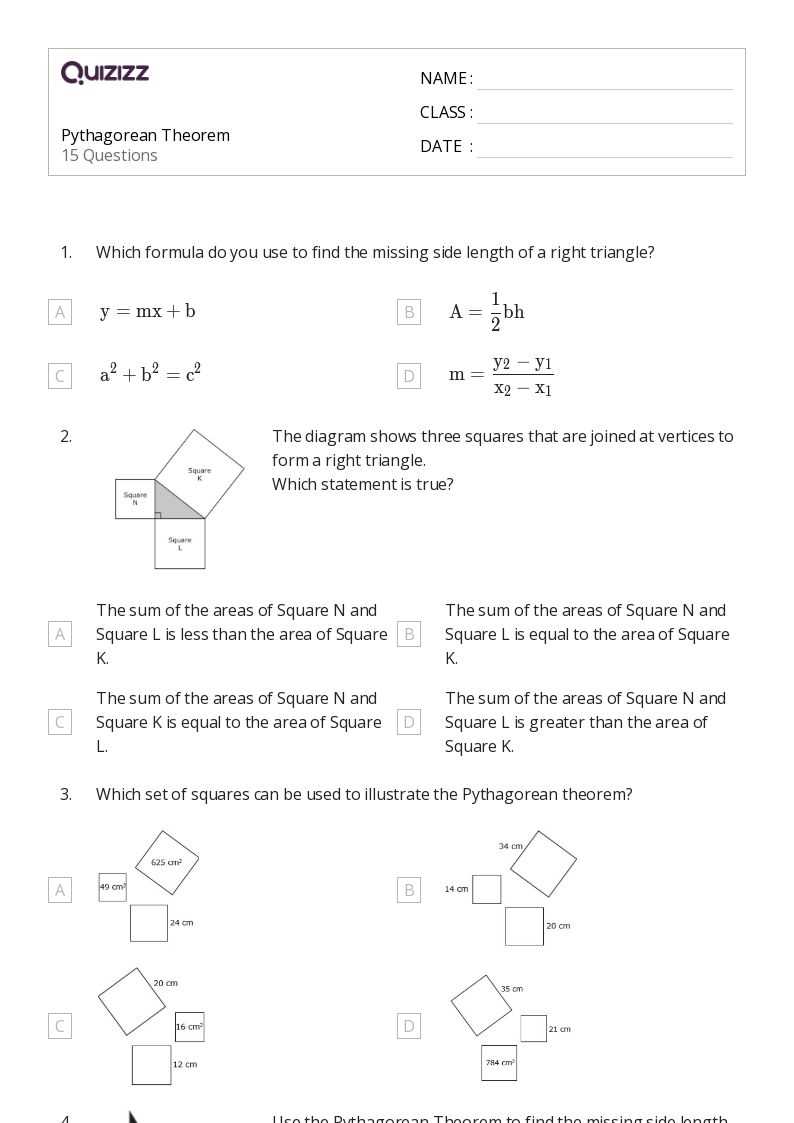
1. Apply Theorems and Properties: Utilize relevant mathematical theorems, such as the Pythagorean theorem or angle relationships, to establish connections between different parts of the problem.
2. Break Down the Problem into Sub-Problems: Divide the problem into smaller, solvable pieces. Solving each smaller problem separately can lead to an overall solution.
3. Check for Symmetry or Patterns: Identifying symmetry or repeating patterns can help reduce the complexity of the problem and lead to quicker solutions.

Geometry Theorems for Problem Solving

Theorems and established mathematical principles play a pivotal role in solving complex problems. These rules provide a structured framework for reasoning through challenges and finding logical solutions. By understanding and applying key theorems, students can efficiently approach problems, simplify difficult tasks, and validate their results. This section covers essential theorems that are frequently used in problem-solving scenarios.

Fundamental Theorems and Their Applications

Several foundational theorems serve as building blocks for solving a wide range of problems. Here are some commonly used principles:

• The Pythagorean Theorem: Used to find the relationship between the sides of a right triangle. The formula a² + b² = c² is instrumental in determining the length of an unknown side.
• Triangle Sum Theorem: This theorem states that the sum of the interior angles of any triangle is always 180°. It is essential for solving angle-related problems in triangles.
• Parallel Lines and Transversals: When a transversal crosses two parallel lines, several angle relationships, such as alternate interior angles and corresponding angles, can be used to find unknown values.

Advanced Theorems for Complex Problems

For more advanced scenarios, additional theorems are often needed to simplify the process:

• Angle Bisector Theorem: This theorem states that the angle bisector divides the opposite side into two segments that are proportional to the adjacent sides. It is particularly useful for problems involving triangles and proportional relationships.
• Congruence and Similarity Theorems: Theorems such as the Side-Angle-Side (SAS) and Angle-Angle (AA) are critical for proving that two shapes are congruent or similar, which can help in solving problems involving geometric transformations and measurements.

Using Formulas Effectively in Geometry

Formulas are essential tools that provide a structured way to solve mathematical problems involving shapes, angles, and measurements. Mastering the use of formulas can significantly streamline the problem-solving process, allowing for quick and accurate solutions. However, knowing when and how to apply these formulas is just as important as understanding them. In this section, we will explore effective strategies for using formulas in various scenarios.

First, it’s crucial to memorize key formulas and understand their underlying concepts. Recognizing which formula applies to which situation is often the first step in solving a problem. For example, knowing the formula for the area of a circle (πr²) or the perimeter of a rectangle (2l + 2w) can quickly lead to the correct solution if the right values are identified.

Additionally, it’s important to break down complex problems into simpler components, which can then be solved using relevant formulas. This method helps in isolating unknowns and applying the appropriate equations step by step. For example, when working with composite shapes, one might need to divide the figure into smaller parts, solve for each part, and then combine the results.

Finally, double-checking units and converting between them is a key aspect of using formulas effectively. Many problems involve measurements in different units, and applying a formula with incorrect units can lead to errors. Converting measurements to consistent units before applying formulas ensures that the calculations are accurate and meaningful.

Identifying Common Mistakes in Geometry

When solving mathematical problems, it’s easy to overlook details that can lead to errors. Recognizing and understanding these common mistakes can help improve accuracy and efficiency in solving complex tasks. Often, errors occur due to misinterpretation of the problem, incorrect application of formulas, or simple calculation mistakes. By identifying these pitfalls, students can refine their problem-solving techniques and avoid repeating the same mistakes.

One frequent issue is not properly analyzing the given information. Many problems require a clear understanding of the relationships between different elements, such as angles, lines, or shapes. Misreading the problem can lead to using the wrong approach or applying irrelevant formulas. To avoid this, always take time to carefully examine the problem and highlight key information before jumping to the solution.

Another common mistake is misapplying formulas. For instance, using the wrong formula for a specific shape or geometric concept can result in incorrect results. It’s essential to understand the conditions under which each formula applies. Sometimes, students use a formula without checking whether it fits the problem’s requirements, which can lead to errors. To prevent this, always ensure that you have the correct formula before proceeding with the calculations.

Overlooking Units and Conversions is another area where mistakes are often made. Whether it’s working with areas, volumes, or angles, failing to convert units properly can lead to significant errors. Always check that the units are consistent throughout the problem and make any necessary conversions before applying a formula. Inconsistent units can distort results and make the final solution inaccurate.

Finally, careless calculations can lead to minor but critical errors. Simple arithmetic mistakes, such as adding or multiplying incorrectly, are often overlooked in the heat of solving more complex problems. Double-checking every calculation is an effective way to minimize these kinds of errors and ensure the final answer is correct.

Understanding Coordinate Geometry Connections

In mathematics, the study of points, lines, and shapes on a plane is closely linked to the concept of coordinates. By using a coordinate system, we can describe geometric objects and solve problems that involve distances, slopes, and areas. Understanding how these elements interact is crucial for navigating more advanced concepts and developing problem-solving skills. In this section, we will explore how coordinate-based methods are applied to analyze relationships and solve various mathematical challenges.

One of the fundamental concepts in this area is the distance formula, which allows us to calculate the distance between two points on a plane. By knowing the coordinates of both points, the formula helps find how far apart they are. This is essential in problems involving the length of segments, as well as when determining whether points lie on the same line or curve.

Another key concept is the slope, which measures the steepness of a line. The slope formula calculates the ratio of vertical change to horizontal change between two points on a line. This is particularly useful for determining whether two lines are parallel, perpendicular, or intersecting. By understanding how slope works, one can also derive the equation of a line in slope-intercept form, which is crucial in solving problems related to linear equations and graphs.

Lastly, the midpoint formula helps find the exact middle point between two given points. This is important in problems where symmetry or balance is required, such as in bisecting a segment or finding the center of a shape. By applying these coordinate-based techniques, students can better understand how geometric concepts translate into algebraic expressions, strengthening their ability to solve complex problems.

Visualizing Geometry Problems with Graphs

Graphical representations are powerful tools in solving mathematical problems. By plotting shapes, lines, and points on a grid, one can transform abstract concepts into visual forms that are easier to understand and manipulate. This approach helps to break down complex problems and allows for a clearer understanding of relationships between elements. In this section, we will explore how graphs can be used to visualize and solve various mathematical challenges.

One common method for visualizing problems is by plotting points on a coordinate plane. This makes it easier to analyze distances, slopes, and intersections between lines. When dealing with equations of lines or curves, graphing them allows for a visual understanding of their behavior, helping to identify patterns and solutions that might not be immediately obvious through algebraic methods alone.

• Identifying Intersections: By graphing lines or curves, one can easily see where they intersect. This is particularly helpful when solving systems of equations or finding the point of intersection of geometric shapes.
• Understanding Shapes: Graphs help to clearly illustrate the properties of different shapes, such as triangles, quadrilaterals, and circles. By plotting the vertices or key points, it becomes easier to calculate area, perimeter, and other properties.
• Analyzing Symmetry: Visualizing symmetry is made simple by graphing. By plotting key points and lines of symmetry, one can quickly see whether a shape is symmetrical about a particular axis or point.

In addition to basic shapes, graphs can also be used to represent transformations such as rotations, translations, and reflections. By applying these transformations to points or shapes on the graph, students can better understand how the figure moves and how its properties change.

Overall, graphing is an invaluable technique for visualizing mathematical problems. It allows for a deeper understanding of geometric concepts and provides a powerful way to check the accuracy of solutions through visual confirmation.

Working with Angles in Geometry Connections

Angles are a fundamental concept in mathematics, playing a crucial role in understanding the relationships between lines, shapes, and figures. By mastering how to calculate, classify, and apply angles, students can solve various problems involving measurement, construction, and transformations. This section will explore the different types of angles and how to effectively work with them in various mathematical contexts.

There are several key types of angles that one must be familiar with:

• Acute Angle: An angle that measures less than 90 degrees. These are often found in triangles and other polygons, contributing to the overall shape and size of the figure.
• Right Angle: An angle that measures exactly 90 degrees. Right angles are crucial in creating perpendicular lines and understanding orthogonal relationships in shapes.
• Obtuse Angle: An angle greater than 90 degrees but less than 180 degrees. Obtuse angles often appear in various polygons, such as obtuse triangles, and impact their internal angle sum.
• Reflex Angle: An angle that is greater than 180 degrees but less than 360 degrees. Reflex angles are important when working with circular arcs or when measuring angles around a central point.

Understanding how to calculate angles is essential for solving problems. One common approach is using the angle sum property, which states that the sum of the interior angles of a polygon is related to the number of sides. For example, the sum of the interior angles of a triangle is always 180 degrees, and the sum of the interior angles of a quadrilateral is always 360 degrees.

Another important technique is angle bisectors, which divide an angle into two equal parts. This concept is particularly useful in constructions and when dealing with symmetry in geometric shapes.

In addition to these methods, understanding complementary and supplementary angles is critical. Two angles are complementary if their sum is 90 degrees and supplementary if their sum is 180 degrees. Identifying and using these relationships can simplify problem-solving, especially in complex geometric configurations.

Finally, when working with angles, it’s essential to remember the vertical angle theorem, which states that when two lines intersect, the opposite angles are equal. This property helps in solving for unknown angles and provides insight into the structure of intersecting lines.

Strategies for Answer Key Verification

Verifying the correctness of solutions is an essential step in problem-solving. It involves checking whether the computed results align with the expected outcomes, ensuring accuracy and reliability in the final answer. This process is important not only for confirming that the right approach has been used but also for building confidence in one’s work. In this section, we will explore several effective strategies to verify solutions and ensure consistency in the results.

One of the most reliable methods for verification is performing the calculations in reverse. This allows you to check the original process by undoing each step, ensuring that no mistakes were made. For example, when solving equations, you can substitute your final solution back into the equation to verify its validity.

Method	Explanation	Example
Reverse Substitution	Substitute the solution back into the original problem to confirm its accuracy.	For x = 5, substitute into the equation 2x + 3 = 13: 2(5) + 3 = 13. Since both sides match, the solution is verified.
Estimate and Compare	Use estimation techniques to compare the results with approximate values. If the estimated result is close to the computed one, the solution is likely correct.	If the answer is a large number, estimate the result based on the scale of the problem and compare it to the final answer.
Cross-Check with Multiple Methods	Solving the problem using different methods and comparing results can reveal any discrepancies.	Solve a problem algebraically, then check the result with a graphical or numerical approach to confirm consistency.

Another effective strategy is to verify the consistency of the results by checking intermediate steps. By confirming that the intermediate calculations are correct, you can be more confident in the final outcome. This method ensures that small errors are caught before they affect the overall solution.

Finally, reviewing the problem’s structure and ensuring all conditions are met can help identify any overlooked assumptions or errors. This might include ensuring the correct interpretation of the problem’s requirements, checking for units and conversions, or making sure the correct formulas were applied.

By employing these verification strategies, you can greatly improve the reliability of your solutions and avoid potential mistakes in the problem-solving process.

How to Check Your Solutions

Ensuring the correctness of your results is a crucial part of solving any problem. This involves reviewing your work to make sure that each step of the process was followed accurately and that the final outcome is reliable. Checking solutions is not just about verifying numbers, but also about confirming that the methods and principles used align with the problem’s requirements.

One effective way to check your solutions is by reworking the problem using a different method or approach. This helps to confirm the validity of your results by providing an alternative perspective. For example, if you used algebraic methods to solve a problem, try solving it graphically or using another mathematical tool to cross-check your result.

Common Techniques for Solution Verification

Verification Method	Description	Example
Recheck All Calculations	Go through each calculation step again to ensure no arithmetic errors occurred.	If your solution involves adding or multiplying numbers, verify that each operation was done correctly.
Substitute Back into the Problem	Substitute the final result back into the original problem to see if it holds true.	For a system of equations, substitute the values of variables into both equations to check for consistency.
Estimate and Compare	Estimate the result using a simpler method or approximation and compare it to your calculated answer.	If your final result is a large number, check if the order of magnitude seems reasonable.

Cross-Checking with Diagrams

If your solution involves spatial reasoning or shapes, drawing a diagram can help you visually check if your calculations align with the problem’s conditions. Ensure that all the points, lines, and angles are represented correctly. Checking the proportions and relationships between different elements in your diagram can often reveal mistakes that may not be immediately obvious in your numerical calculations.

By using these strategies to check your work, you can significantly reduce the likelihood of errors and strengthen your understanding of the concepts involved in solving the problem.

Importance of Practice in Mastery

Achieving proficiency in any subject, particularly in fields involving logical reasoning and spatial relationships, requires consistent practice. Mastering complex concepts is not simply about understanding the theories but being able to apply them repeatedly in various situations. The more problems you solve, the more familiar you become with the patterns and strategies necessary to find solutions efficiently.

Repetition not only strengthens your understanding but also improves problem-solving speed and accuracy. With each attempt, you begin to recognize nuances in questions, develop shortcuts for common problem types, and gain confidence in your ability to solve more challenging scenarios. Practice also helps in identifying common mistakes and learning how to avoid them in the future.

Key Benefits of Continuous Practice

Benefit	Description
Improved Understanding	Regular practice deepens your grasp of fundamental concepts and their applications, making complex topics more accessible.
Enhanced Problem-Solving Skills	The more problems you encounter, the more strategies you develop for solving similar questions more effectively.
Boosted Confidence	With enough practice, you develop the confidence to tackle even the most difficult problems with ease.
Faster Solutions	As you practice, you become quicker at identifying key information and using it to form solutions, which is especially useful during timed assessments.

By committing to a regular practice schedule, you can refine your problem-solving abilities and make solving even the most complex problems feel intuitive. The effort put into consistent practice pays off in the long run, turning difficult tasks into manageable challenges.

Final Thoughts on Solution Guides

When it comes to solving complex problems, having a reference that outlines correct solutions can be an invaluable resource. These guides not only provide the correct answers but also show the process behind reaching them, helping learners understand the reasoning involved. Whether you are reviewing your work or troubleshooting mistakes, a well-organized solution guide is crucial for reinforcing concepts and improving your problem-solving skills.

However, it’s important to remember that these guides should be used as a tool for learning, not as a crutch. While they can offer clarification and verification, true mastery comes from engaging with the problems independently. By attempting to solve problems first on your own and then consulting the solutions, you are reinforcing your understanding and developing the critical thinking skills necessary to apply concepts in diverse scenarios.

Using Solution Guides Effectively

• Review Step-by-Step: Instead of jumping straight to the final result, take time to follow the steps outlined in the guide. This will help you understand the logic behind each decision.
• Identify Mistakes: Use the guide to spot any errors you made in your approach, allowing you to learn from them and avoid repeating them in the future.
• Reinforce Concepts: After reviewing the solution, try solving similar problems without looking at the guide. This will test your understanding and ensure you’re applying the concepts correctly.

In conclusion, while solution guides provide helpful support, they are most effective when used as part of a balanced approach to learning. The key is to use them wisely–only after attempting problems on your own, to ensure that you are not simply memorizing answers but developing a deeper comprehension of the material.