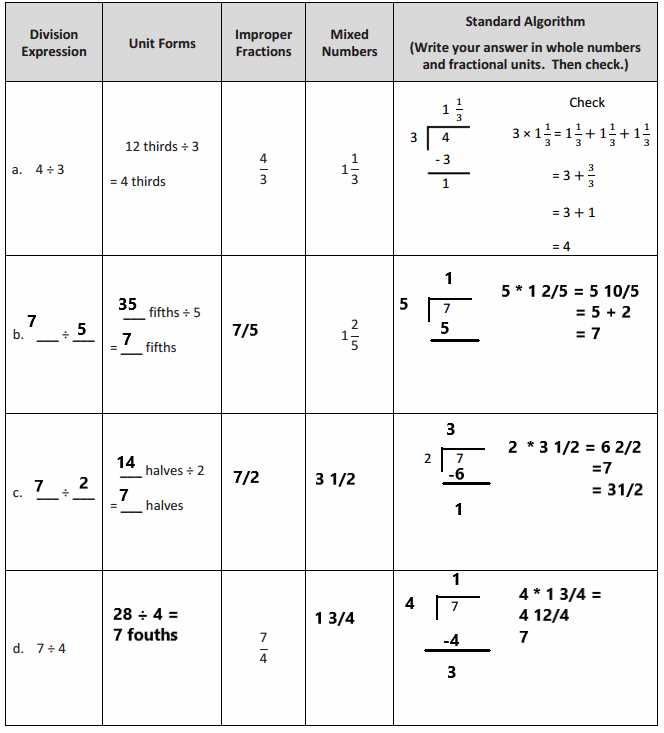
In the fifth level of learning, students are introduced to various complex concepts that challenge their analytical and problem-solving abilities. This section is designed to help learners grasp the essential skills needed to solve a wide range of exercises efficiently. By focusing on key topics, learners are encouraged to approach problems from different angles, ensuring they can tackle any challenge with confidence.
Effective problem-solving requires more than just knowing how to compute answers; it involves understanding the logic behind each question and the methods used to reach a solution. This approach helps students develop critical thinking skills that are applicable beyond just their studies. In this guide, we’ll explore how learners can use provided solutions to better understand the process and improve their skills.
By practicing different types of exercises and reviewing detailed solutions, students gain the confidence to handle similar problems in the future. Through consistent practice and a strong grasp of the core principles, anyone can excel in this challenging area of study. Whether it’s fractions, geometry, or other topics, the key is persistence and the willingness to learn from mistakes.
Engageny Math Grade 5 Module 4 Overview
This section focuses on developing students’ skills in key mathematical concepts that lay the foundation for more advanced topics. It challenges learners with a variety of exercises aimed at enhancing their problem-solving abilities and understanding of essential principles. Throughout this part of the curriculum, students are introduced to complex reasoning techniques that help build their confidence and proficiency.
Core Concepts Covered
The main focus is on strengthening comprehension of fractions, decimals, and basic geometry. Students learn how to manipulate fractions in various contexts, working with addition, subtraction, multiplication, and division. Emphasis is also placed on understanding the relationships between numbers and geometric figures, preparing learners for future mathematical challenges.
Developing Critical Skills
Beyond just solving problems, this section teaches students how to approach each question methodically. By learning to break down complex scenarios into manageable steps, students develop a systematic approach to solving any type of problem. This process not only improves mathematical accuracy but also fosters a deeper understanding of the subject matter.
Understanding the Key Concepts of Module 4
In this section, students are introduced to a series of concepts that build upon foundational knowledge and prepare them for more complex problem-solving. The focus is on strengthening comprehension of numerical relationships and enhancing the ability to approach a variety of challenges logically and systematically. The goal is to ensure that students understand not only the methods but also the reasoning behind each solution.
Fractions and Decimals
One of the primary areas covered in this section is the manipulation of fractions and decimals. Learners work on converting between the two, adding and subtracting fractions with unlike denominators, and understanding how decimals fit into everyday calculations. By gaining proficiency in these areas, students will find it easier to apply these concepts in more advanced topics.
Understanding Geometry
Another important concept explored is basic geometry, which includes learning about shapes, their properties, and how to calculate their areas and perimeters. The objective is to develop spatial awareness and the ability to visualize and solve real-world problems related to geometry. These concepts are crucial for understanding higher-level mathematics.
Understanding the Key Concepts of Module 4
In this section, students are introduced to a series of concepts that build upon foundational knowledge and prepare them for more complex problem-solving. The focus is on strengthening comprehension of numerical relationships and enhancing the ability to approach a variety of challenges logically and systematically. The goal is to ensure that students understand not only the methods but also the reasoning behind each solution.
Fractions and Decimals
One of the primary areas covered in this section is the manipulation of fractions and decimals. Learners work on converting between the two, adding and subtracting fractions with unlike denominators, and understanding how decimals fit into everyday calculations. By gaining proficiency in these areas, students will find it easier to apply these concepts in more advanced topics.
Understanding Geometry
Another important concept explored is basic geometry, which includes learning about shapes, their properties, and how to calculate their areas and perimeters. The objective is to develop spatial awareness and the ability to visualize and solve real-world problems related to geometry. These concepts are crucial for understanding higher-level mathematics.
Common Challenges in Grade 5 Math

At this level, students often encounter several difficulties as they deal with more complex mathematical concepts. While some topics may seem straightforward, they require a deeper understanding of the relationships between numbers, shapes, and operations. It’s important to recognize these challenges early and develop strategies to overcome them effectively.
Typical Struggles Faced by Students
Several common issues arise when students work through problems in this subject. These challenges are often related to the transition from simple calculations to more abstract concepts, which require both understanding and application. The following areas are where learners tend to face the most difficulty:
- Understanding Fractions: Many students struggle with fractions, especially when it comes to comparing, adding, or subtracting them with different denominators.
- Decimal Conversions: Converting between fractions and decimals can be confusing, and students often have difficulty mastering the rules for rounding or estimating decimal values.
- Word Problems: Applying mathematical operations to solve real-world problems is a common hurdle. Many students find it challenging to identify which steps to take and which operations to use.
Overcoming These Difficulties
While these challenges are common, there are proven methods to help students build confidence and improve their skills. Regular practice, clear explanations, and interactive tools can greatly enhance understanding and reduce frustration. Providing step-by-step guidance and encouraging critical thinking can help students approach problems with a positive mindset and avoid confusion.
Step-by-Step Guide to Answering Questions
When tackling problems, following a clear and organized approach is essential for achieving accuracy and understanding. This section provides a methodical breakdown of how to approach each query, ensuring all necessary steps are covered and that the solutions are both effective and well-supported. Each stage involves critical thinking, organization, and careful review to ensure the process is both thorough and efficient.
Understanding the Question
Before attempting any solution, take a moment to comprehend what is being asked. Break down the problem into smaller, manageable parts, and identify key information that will guide you to the correct answer.
- Read the problem carefully to fully grasp its meaning.
- Highlight or underline important details such as numbers, operations, or key phrases.
- Identify what the problem is asking for (e.g., a total, a comparison, a pattern).
Organizing Your Approach

Once the question is clear, create a plan for solving it. This helps in maintaining focus and ensures each necessary step is completed in the correct order.
- Write down any formulas, equations, or concepts that are relevant to the problem.
- Outline a strategy or method that fits the question’s structure.
- Ensure you have the necessary tools or resources to help solve the problem.
Executing the Solution
Now, implement the plan you’ve created. Work through the problem step by step, taking care to show all calculations and reasoning.
- Perform each calculation or operation methodically.
- Check each step to avoid small errors that could lead to incorrect results.
- Keep track of your progress, ensuring every detail aligns with the original question.
Review and Verify
After reaching a solution, go back and verify that everything makes sense. Review the steps taken to ensure that the approach was correct and the final answer is logical.
- Double-check all calculations and logic.
- Ensure that the final answer addresses what was asked in the problem.
- If possible, rework the problem in a different way to verify consistency.
Common Mistakes to Avoid in Module 4
When working through problems, it’s easy to make errors that can derail the entire process. Understanding and avoiding these common pitfalls can greatly improve accuracy and efficiency. Below are some of the most frequent mistakes that learners encounter, along with tips on how to prevent them.
- Misinterpreting the Problem: Failing to fully understand the question is a frequent mistake. Always take time to read and comprehend what is being asked before proceeding. Highlight key information and ensure you grasp the context before applying any methods.
- Skipping Steps: Rushing through steps can lead to careless mistakes. Make sure to follow a logical sequence, showing all work and reasoning. Each step should be clearly outlined to ensure nothing is overlooked.
- Incorrect Application of Concepts: Sometimes, the wrong formula or method is used due to a misunderstanding of the problem’s structure. Always ensure that the right technique is applied for each specific type of problem.
- Calculation Errors: Small mistakes in arithmetic can add up quickly. Double-check calculations to avoid simple errors that can compromise the result. Use tools like a calculator if needed for accuracy.
- Not Checking Work: Failing to review solutions before finalizing them can lead to missed errors. Always go back over the steps, recheck calculations, and ensure the solution makes sense within the context of the problem.
Key Strategies for Solving Fractions

Working with fractions can seem challenging, but with the right strategies, it becomes much easier to understand and solve problems. By following a structured approach and applying the correct techniques, you can simplify complex fraction problems and reach accurate solutions more efficiently.
Understanding the Problem
Before jumping into solving fraction problems, it’s important to first comprehend what is being asked. Take a moment to identify the types of operations involved and the relationship between the numbers.
- Read the problem carefully and identify if you are adding, subtracting, multiplying, or dividing fractions.
- Look for any common denominators or factors that could simplify the process.
- Ensure you know whether the fractions are proper, improper, or mixed numbers, as this affects the solution method.
Step-by-Step Methods
Each operation with fractions requires a specific approach. Applying the right method for each type of operation ensures that you can work through problems correctly and efficiently.
- Adding and Subtracting Fractions: Ensure the denominators are the same. If they are not, find the least common denominator (LCD) and adjust the fractions accordingly. Once the fractions have the same denominator, you can add or subtract the numerators.
- Multiplying Fractions: Multiply the numerators together and the denominators together. Simplify the result by finding the greatest common divisor (GCD) of the numerator and denominator, if possible.
- Dividing Fractions: To divide by a fraction, multiply by its reciprocal (the fraction flipped upside down). Follow the same steps as multiplication: multiply the numerators and the denominators.
Checking and Simplifying the Result
After solving the problem, it’s essential to check your work and simplify the fraction to its lowest terms. This ensures the solution is as simple and accurate as possible.
- Review your calculations for accuracy and consistency.
- Simplify the fraction by dividing both the numerator and denominator by their greatest common divisor.
- If applicable, convert improper fractions into mixed numbers for clarity.
Working with Decimals in Module 4
Decimals play an important role in many mathematical operations and real-life applications. Understanding how to work with decimal numbers is crucial for solving a variety of problems. In this section, we will explore essential techniques for performing calculations with decimals and how to handle them effectively in different scenarios.
Adding and Subtracting Decimals
When adding or subtracting decimal numbers, alignment of the decimal points is key. This ensures that the numbers are correctly placed in each column and no digits are skipped during the operation.
| Example | Step-by-Step Process |
|---|---|
| 3.56 + 2.1 |
|
| 5.75 – 2.25 |
|
Multiplying and Dividing Decimals
For multiplication, you multiply as if the numbers are whole numbers, then place the decimal point in the product based on the total number of decimal places in the factors. For division, adjust the divisor to be a whole number by shifting the decimal point, and do the same with the dividend.
| Example | Step-by-Step Process |
|---|---|
| 1.25 × 3.2 |
|
| 6.4 ÷ 0.8 |
|
Understanding Geometry in Module 4
Geometry involves the study of shapes, sizes, and the properties of space. Understanding these concepts is essential for solving problems related to measurement, angles, and spatial relationships. This section covers fundamental geometric ideas and provides techniques for identifying and working with various shapes, understanding their properties, and solving related problems effectively.
Recognizing and Classifying Shapes
The first step in understanding geometry is to recognize different types of shapes and classify them according to their properties. Each shape has specific characteristics that define it, such as the number of sides, angles, and symmetry.
- Triangles: Classified by the lengths of their sides (equilateral, isosceles, scalene) or by their angles (acute, right, obtuse).
- Quadrilaterals: Includes squares, rectangles, parallelograms, rhombuses, and trapezoids, each with distinct properties like parallel sides or equal angles.
- Circles: Defined by a central point and all points equidistant from that point, with properties like radius, diameter, and circumference.
Measuring and Calculating Properties
Once the shapes are identified, understanding how to measure their properties is essential. This involves calculating areas, perimeters, and angles. By applying formulas, one can determine the size and extent of a shape in various contexts.
- Perimeter: The total length of the boundary of a shape. For example, the perimeter of a rectangle is calculated by adding the lengths of all four sides.
- Area: The space contained within a shape. For a rectangle, this is calculated by multiplying its length by its width.
- Angles: Angles are formed where two sides meet. They can be measured using a protractor, and their sum in any triangle is always 180 degrees.
How to Review for Module 4 Assessments
Preparing for an assessment requires more than just memorizing information; it involves reviewing key concepts, practicing problem-solving techniques, and strengthening areas of weakness. A structured approach to studying can help you understand the material more deeply and approach the test with confidence. This section outlines strategies to help you effectively review for upcoming evaluations.
- Organize the Material: Begin by reviewing your notes, textbooks, and any resources provided during lessons. Organize the content into topics or themes, focusing on the key concepts that will likely appear on the assessment.
- Review Examples and Practice Problems: Go through example problems, paying close attention to the steps involved in solving them. Then, attempt similar problems on your own. This helps reinforce your understanding of the process and prepares you for the types of questions that may appear.
- Focus on Difficult Areas: Identify the topics that are most challenging for you and dedicate extra time to those. This might involve revisiting specific concepts or seeking help from a teacher or peer for clarification.
Effective Study Techniques
- Active Recall: Instead of passively reading through notes, actively test yourself on key concepts. Try to recall important facts, formulas, and methods without looking at your notes. This strengthens memory retention and understanding.
- Use Flashcards: Create flashcards for important terms, formulas, or concepts. These can be used for quick, repeated review and are great for reinforcing knowledge in small, digestible chunks.
- Group Study Sessions: If possible, study with classmates or peers. Explaining concepts to others can deepen your own understanding and help clarify difficult areas.
Reviewing Test-Taking Strategies
- Time Management: Practice solving problems under timed conditions. This will help you manage your time effectively during the actual assessment and reduce anxiety.
- Stay Calm and Focused: On the day of the test, ensure you’re well-rested and calm. Take deep breaths if you feel anxious and focus on each problem one at a time.
- Read Instructions Carefully: Before answering any questions, make sure you fully understand the instructions. Rushing through can lead to mistakes or misinterpretations.
Importance of Practice in Grade 5 Math
Regular practice is essential for mastering new concepts and reinforcing what has already been learned. By consistently working through exercises and problems, students can develop a deeper understanding and improve their problem-solving skills. The more a student practices, the more confident they become in applying mathematical concepts in different scenarios, ensuring long-term retention and success.
Practice helps in building accuracy, speed, and a solid foundation for future learning. It enables students to identify patterns, refine their techniques, and better understand the connections between different topics. Additionally, frequent practice allows for a more thorough assessment of areas that may need further attention or improvement.
Interactive Tools for Math Learning
Engaging with interactive tools can significantly enhance the learning experience, making complex concepts easier to understand and apply. These resources allow students to actively participate in their learning journey, providing instant feedback and visual representations of abstract ideas. By utilizing technology, learners can gain a deeper understanding through hands-on exploration and problem-solving exercises.
Benefits of Interactive Learning Tools
- Immediate Feedback: Interactive platforms often provide real-time feedback, helping students identify and correct mistakes as they go.
- Visual Learning: Many tools use visual aids, like graphs and diagrams, to illustrate abstract concepts, making them more accessible and easier to grasp.
- Increased Engagement: The interactive nature of these tools encourages active participation, keeping students motivated and focused on the task at hand.
- Adaptability: Many platforms adjust the difficulty of tasks based on the learner’s progress, providing personalized challenges that match their skill level.
Examples of Interactive Tools
- Online Simulations: These tools allow students to manipulate variables and see real-time changes, helping them visualize the effect of different factors on mathematical outcomes.
- Interactive Quizzes: Digital quizzes with instant feedback help reinforce key concepts and test students’ understanding of specific topics.
- Virtual Manipulatives: These digital objects, like virtual blocks or number lines, allow students to explore mathematical ideas in a hands-on way, enhancing their understanding of abstract concepts.
- Math Games: Educational games turn learning into a fun and competitive activity, motivating students to practice essential skills while enjoying the process.
How Module 4 Prepares for Future Learning
The content covered in this section serves as a crucial stepping stone for more advanced concepts. By mastering the foundational skills and knowledge presented, students build the necessary tools to approach increasingly complex problems. The lessons learned here not only provide a solid grasp of key ideas but also lay the groundwork for continued success in future topics.
This stage of learning focuses on reinforcing critical thinking, problem-solving abilities, and the application of various techniques that will be essential in more challenging scenarios. By developing a deep understanding of these concepts, students can easily adapt to new and more intricate topics in future studies, fostering a more seamless learning progression.
Using the Answer Key Effectively
Answer guides are valuable resources that help clarify the correct approach to solving problems. However, to gain the most benefit, it is important to use them as a tool for learning rather than simply as a means of checking solutions. By carefully analyzing the provided solutions and understanding the reasoning behind each step, students can enhance their comprehension and improve their problem-solving skills.
Rather than relying solely on the final answer, focus on how each problem is solved. Break down the steps involved and ensure that the methods used align with what was taught. If a mistake is made, compare the approach to the correct solution and identify where things went off track. This reflective process helps reinforce key concepts and ensures a deeper understanding of the material.
Exploring Word Problem Solving Strategies
Word problems can often seem overwhelming because they require translating real-world scenarios into mathematical operations. However, with the right strategies, students can break down complex problems into manageable steps. By approaching each problem methodically, learners can improve their ability to identify relevant information and apply the appropriate techniques to find a solution.
A key strategy is to carefully read the problem multiple times to ensure that all details are understood. Highlighting key information, such as quantities and relationships, helps to identify what needs to be solved. Once the information is clear, students can translate the problem into mathematical terms, deciding which operations are necessary to arrive at the correct answer. Practicing these steps regularly improves both accuracy and confidence when tackling word problems.
How to Teach Module 4 Concepts at Home
Teaching complex topics at home can be both rewarding and challenging. With the right approach, parents can support their children’s learning by creating an engaging and structured environment. Helping students understand fundamental concepts and reinforcing those lessons through practice will strengthen their ability to grasp new ideas. This guide provides strategies for effectively teaching key ideas at home.
1. Break Down Concepts into Manageable Steps
Start by introducing one concept at a time and breaking it into smaller, digestible parts. This allows your child to focus on mastering one skill before moving on to the next. Use simple examples and gradually increase complexity as their understanding improves. Reinforce each concept with practical exercises to ensure the material is fully understood before progressing.
2. Use Real-Life Examples
Connecting abstract ideas to everyday situations helps make learning more relatable. For example, you can use shopping scenarios to explain addition, subtraction, or fractions, or measure ingredients while cooking to demonstrate concepts like units of measurement and volume. This hands-on approach can make the material feel more relevant and engaging.
Additionally, regular practice is key. Set aside consistent time for review and exercises, ensuring that your child feels comfortable with each concept before introducing new material. With patience and persistence, learning at home can be a positive and productive experience for both the student and teacher.
Mastering the Module 4 Skills
Achieving mastery in any subject requires a clear understanding of foundational skills and consistent practice. In this section, students will build essential abilities that are critical for success in more advanced topics. By focusing on key techniques and applying them regularly, learners will develop confidence and proficiency. The goal is to fully grasp the skills presented and use them as a solid foundation for future academic challenges.
1. Reinforce Key Concepts Through Repetition

To master any skill, repetition is key. By consistently practicing exercises and revisiting concepts, students can strengthen their understanding. It’s important to vary the types of problems encountered to ensure that learners can apply their knowledge in different contexts. This will not only solidify their grasp of each concept but also prepare them for more complex problem-solving in the future.
2. Focus on Real-World Applications
Understanding how the skills learned can be applied outside of the classroom can enhance comprehension. Encourage students to solve practical problems that are relevant to their everyday lives. Whether it’s budgeting, planning, or measuring, using skills in real-world scenarios helps solidify their value and makes learning more meaningful.
Mastery comes with time and consistent effort. By continuously challenging themselves and seeking new ways to apply their skills, students will find that their confidence and competence grow, paving the way for success in future academic endeavors.