Grade 3 Math Module 5 Answer Key and Solutions

In this section, we provide a collection of resources designed to help students navigate through key topics and concepts at an essential learning stage. By offering structured solutions, we aim to guide learners and support their understanding of critical skills needed for further educational development.

Detailed explanations are included to enhance comprehension and boost confidence in tackling complex exercises. This resource serves as a valuable tool for both students and parents who wish to ensure a solid foundation in important academic areas.

Whether you are reviewing material at home or assisting with in-class exercises, this guide simplifies challenging tasks and provides clear, step-by-step instructions. With careful practice and the right approach, learners can master the topics and build stronger academic abilities moving forward.

Grade 3 Math Module 5 Overview

This section focuses on essential topics that students encounter during their third-year learning journey. It covers important concepts that build the foundation for advanced problem-solving skills. The content is designed to help learners understand patterns, relationships, and critical thinking strategies that will serve them well in future studies.

The material is structured to gradually introduce new skills, reinforcing previously learned topics while challenging students to explore more complex problems. With clear explanations and practice examples, this guide ensures learners stay on track and gain confidence in their abilities.

Topic	Description
Understanding Numbers	Students explore number relationships and develop strategies for working with larger quantities.
Word Problems	Complex situations requiring application of learned concepts in real-world scenarios.
Patterns and Sequences	Identifying and creating patterns to strengthen problem-solving techniques.
Measurement	Introduction to measurement tools and techniques for understanding size and quantity.
Operations	Focus on multiplication and division, with an emphasis on practical problem-solving strategies.

This overview helps to provide a clear roadmap for what students will learn and practice, ensuring a smooth transition into more complex topics in future studies. By mastering these core concepts, learners will have the necessary skills to tackle more advanced exercises with confidence.

Understanding Key Concepts in Module 5

This section covers fundamental ideas that students must grasp to succeed in more advanced topics. The concepts introduced here serve as building blocks, helping learners establish a strong foundation for solving problems with ease. Understanding these key areas is essential for progressing through later stages of learning.

The following concepts are central to this section:

• Number Relationships: Students explore how numbers relate to each other and how they can be manipulated in various contexts.
• Patterns and Sequences: Recognizing recurring structures and learning how to extend them builds critical thinking skills.
• Operations with Larger Numbers: Focusing on addition, subtraction, multiplication, and division helps solidify essential arithmetic skills.
• Problem-Solving Strategies: Learners develop strategies for tackling complex, real-world problems, helping them apply their knowledge practically.
• Measurement Techniques: Students become familiar with using different units and tools to measure and compare objects effectively.

By mastering these concepts, students will not only strengthen their mathematical reasoning but also improve their ability to approach unfamiliar problems with confidence. The progression of these skills is key to their success in both current and future studies.

How to Use the Answer Key Effectively

To maximize the benefits of review materials, it’s important to approach solutions with a focused and thoughtful mindset. The right techniques can help students better understand where they went wrong and how they can improve their skills for future tasks. This section outlines strategies for using the solutions guide in the most productive way.

Here are some tips for using the solutions guide effectively:

• Check Step-by-Step: Review each step of the solution carefully. This helps you understand the process, not just the final result.
• Identify Mistakes: If your result differs from the provided solution, examine where you made an error. This process encourages learning from mistakes.
• Don’t Rush: Take time to read through explanations, even if you get the correct answer. Understanding the reasoning behind each step is key to mastering the concept.
• Use it as a Learning Tool: Treat the solution guide as a resource for learning rather than just a way to check your work. Reflect on why the method works and how it applies to other problems.
• Practice Similar Problems: Once you’ve reviewed the solution, attempt similar exercises to reinforce your understanding.

By following these practices, students can ensure they are gaining a deeper understanding of the material, which will help them succeed in more advanced tasks. The goal is not just to get the right answer but to develop the problem-solving skills that will be valuable throughout their studies.

Common Challenges in Module 5

Throughout this section, learners often face a variety of obstacles that can make certain topics more difficult to grasp. These challenges, while common, can be overcome with the right strategies and practice. Understanding these potential struggles is the first step in finding effective solutions and improving performance.

Some of the most frequent difficulties include:

• Understanding Complex Word Problems: Many students find it challenging to translate word problems into solvable equations. These require careful reading and a step-by-step approach.
• Multiplying and Dividing Larger Numbers: As numbers grow in size, operations become more complex. Students may struggle to keep track of calculations and perform them accurately.
• Recognizing Patterns: Identifying and extending patterns can be difficult, especially when the structure is not immediately obvious or requires multiple steps.
• Measurement Conversions: Understanding how to convert between different units of measurement can confuse students, as they often need to apply different techniques for different types of quantities.
• Applying Problem-Solving Strategies: Learners may find it hard to know which strategies to use in different situations, especially when the problem-solving method is new or unfamiliar.

By being aware of these challenges, students can adopt specific strategies to tackle each one. With consistent practice and guidance, these obstacles can be turned into valuable learning opportunities, helping students strengthen their skills and build confidence.

Step-by-Step Solutions for Each Problem

Breaking down complex tasks into smaller, manageable steps is a crucial strategy for understanding and solving problems effectively. By following a clear, step-by-step approach, students can grasp the logic behind each solution and apply it to similar challenges. This section provides a structured way to solve problems, ensuring clarity and comprehension at every stage.

Each problem is presented with a detailed process that walks through the necessary steps to arrive at the correct solution. By following these steps, learners can develop the skills to independently solve similar problems in the future.

Problem	Steps to Solve
Example 1: Addition of Large Numbers	Identify the numbers to be added. Align the digits based on place value. Start with the ones column, carrying over as needed. Proceed to the tens, hundreds, and higher place values. Write the final sum at the bottom.
Example 2: Subtraction Word Problem	Read the problem carefully to understand what is being asked. Write down the numbers involved in the subtraction. Perform the subtraction, borrowing if necessary. Check the result to ensure accuracy.
Example 3: Multiplication of Two-Digit Numbers	Write the numbers in a vertical format. Multiply each digit of the bottom number by the top number. Align the partial products correctly. Add the partial products together to find the final result.
Example 4: Division with Remainders	Divide the dividend by the divisor. Record the quotient and the remainder. If necessary, convert the remainder into a fraction or decimal.

By following these structured steps, students can systematically tackle each problem with confidence. These solutions not only provide the correct answers but also foster a deeper understanding of the underlying processes, which will aid in solving more complex problems in the future.

Tips for Improving Math Skills

Building strong problem-solving abilities requires practice, patience, and the right strategies. To enhance performance and increase confidence in handling various tasks, it’s essential to incorporate specific techniques and routines. By applying these practical tips, learners can sharpen their skills and make steady progress in their studies.

Practice Regularly

Consistent practice is one of the most effective ways to improve skills in any area. Regularly working through exercises not only reinforces concepts but also helps develop faster and more accurate responses. Here are some useful tips for creating a practice routine:

Tip	Description
Start with Simple Problems	Begin by solving easier problems to build confidence and reinforce basic concepts before moving to more challenging tasks.
Break Down Complex Problems	Split difficult problems into smaller, manageable steps to prevent feeling overwhelmed and to ensure accuracy at each stage.
Track Your Progress	Keep a record of completed exercises to monitor improvement and identify areas that need more attention.

Understand the Process

Rather than simply memorizing formulas or procedures, it’s important to understand the reasoning behind each step. This deeper comprehension fosters a stronger grasp of the material and makes it easier to apply concepts in different contexts. Consider these methods:

Tip	Description
Ask Why	Question why certain steps are taken or why a specific formula is used. Understanding the logic behind each decision enhances overall learning.
Work with Visual Aids	Using diagrams, charts, or number lines can make abstract concepts more tangible and easier to comprehend.
Discuss with Others	Explaining solutions to someone else or asking questions in study groups helps reinforce knowledge and expose gaps in understanding.

By applying these strategies and remaining consistent, learners can see noticeable improvements in their problem-solving abilities. The key is to focus on mastering the process, not just reaching the right answer. With dedication, success will follow naturally.

Interactive Resources for Students

Engaging with interactive tools can significantly enhance learning experiences. These resources offer a dynamic way to practice, reinforce concepts, and receive immediate feedback. By incorporating technology and hands-on activities, students are able to learn at their own pace, making the learning process both enjoyable and effective.

There are various types of interactive resources that can support students in mastering different concepts:

• Educational Games: These games turn learning into an enjoyable experience while helping students practice key skills. Games that focus on problem-solving and logical thinking can strengthen understanding of core concepts.
• Online Practice Tests: Virtual quizzes and practice tests offer instant feedback, allowing students to identify areas that need improvement while gaining more confidence in their abilities.
• Interactive Simulations: These tools allow students to manipulate variables and observe outcomes, making abstract concepts more tangible and easier to understand.
• Video Tutorials: Step-by-step video guides provide visual and verbal explanations, helping students see and hear how problems are solved, which can be especially helpful for visual learners.
• Interactive Worksheets: Digital worksheets often come with interactive features like drag-and-drop exercises, clickable answers, and instant feedback, allowing students to practice skills in an engaging way.

Using these tools can help break down difficult topics, making them more accessible and engaging. By exploring these interactive resources, students can reinforce their understanding and take an active role in their own learning process.

How to Review Answers with Your Child

Reviewing solutions with your child is an essential part of the learning process. It provides an opportunity to reinforce concepts, address misunderstandings, and build problem-solving skills. A positive and supportive approach during this time can make a big difference in your child’s confidence and overall progress.

Here are some steps to guide you through the process of reviewing solutions with your child:

1. Encourage Independent Thinking: Before giving the correct solutions, ask your child to explain how they arrived at their answers. This helps you identify where they may have made mistakes and encourages critical thinking.
2. Focus on the Process, Not Just the Result: It’s important to highlight the steps they took to solve a problem, not just the final answer. This reinforces the understanding of how to approach similar problems in the future.
3. Discuss Mistakes Positively: Mistakes are a natural part of learning. When errors are made, turn them into teaching moments. Gently explain where they went wrong and guide them through the correct approach.
4. Use Visual Aids: Diagrams, number lines, or visual representations can help clarify concepts and make abstract ideas easier to understand. Encourage your child to draw out problems to better visualize the solutions.
5. Provide Reinforcement: After reviewing the mistakes, go over similar problems together to ensure your child understands the concepts. Repeat practice is often key to mastering new skills.
6. Be Patient and Supportive: Learning takes time, and every child progresses at their own pace. Offer encouragement, celebrate small victories, and maintain a calm and positive attitude throughout the review session.

By creating a collaborative and supportive environment, you can help your child become more confident and capable in solving problems. Regular review sessions also provide a chance to build stronger bonds while promoting academic growth.

Exploring the Importance of Word Problems

Word problems are a key element in developing problem-solving skills. These types of exercises challenge learners to translate real-life situations into mathematical terms, helping them apply abstract concepts to everyday scenarios. By engaging with word problems, students learn to think critically, break down complex tasks, and practice reasoning in a structured way.

Bridging Theory with Practical Application

One of the main benefits of working with word problems is the opportunity to connect theoretical knowledge with practical use. Rather than simply solving equations, students must understand the context and use their skills to make sense of the problem at hand. This real-world application builds a deeper understanding of the concepts, making them more relevant and meaningful.

Developing Critical Thinking and Analytical Skills

Word problems require more than just following formulas–they demand careful analysis and strategic thinking. To find a solution, students must assess the given information, determine what is needed, and decide on the best approach. This process helps develop logical thinking and improves their ability to tackle complex situations. Here are some reasons why word problems are important:

• Encourage Analytical Thinking: Students need to identify key details and eliminate irrelevant information to find the correct solution.
• Improve Decision-Making: By working through different strategies to solve a problem, learners develop the ability to make informed choices in challenging situations.
• Build Real-World Problem-Solving Skills: Word problems simulate situations students might encounter in real life, helping them prepare for future challenges.
• Enhance Communication Skills: Explaining how to approach and solve a word problem strengthens both written and verbal communication, as students must clearly express their thought process.

Overall, word problems play a critical role in fostering well-rounded problem-solvers. They encourage students to think creatively, approach problems from multiple angles, and develop a deeper understanding of how mathematical concepts apply to the world around them.

Guidelines for Teachers Using the Answer Key

As an educator, using resources effectively is crucial for helping students grasp important concepts. While review materials and solutions can provide valuable insight, it’s essential for teachers to approach them with a thoughtful strategy. These resources should be used to guide instruction, provide targeted feedback, and support student learning without simply giving away solutions.

Approach with Care and Precision

When utilizing solution guides, it’s important to follow these principles:

• Encourage Independent Thinking: Before showing the correct responses, give students time to solve problems on their own. Use the resource to verify their reasoning and provide extra support where needed.
• Focus on Process, Not Just Results: The goal is to teach students the steps required to reach a solution, not just the correct answer. Walk them through the method used to solve each problem, emphasizing the reasoning behind each step.
• Personalize Feedback: Every student may encounter unique challenges. Use the guide to address specific misconceptions or errors, offering tailored explanations that meet their needs.

Building Confidence and Understanding

Solution guides should be used as a teaching tool to build confidence in students. Here are some strategies to maximize their effectiveness:

• Provide Positive Reinforcement: When students arrive at the correct solution, praise their efforts. Recognition of correct steps helps build confidence and motivates students to keep practicing.
• Identify Patterns in Mistakes: If students consistently make similar mistakes, the solution guide can help pinpoint areas of misunderstanding. Use this information to adjust future lessons.
• Use Group Discussions: Encourage students to explain their thought processes to peers. This fosters collaborative learning and allows students to learn from one another.

Incorporating solution resources in a balanced way can enhance teaching, create opportunities for deeper learning, and foster a more supportive classroom environment.

Helping Students Master Core Topics

To truly succeed, students need a strong foundation in fundamental concepts. Mastering key ideas early on helps them build the skills necessary for more advanced learning. However, this process requires a balanced approach, combining practice, reinforcement, and application in meaningful contexts. It’s essential for educators to support students in a way that fosters understanding and long-term retention.

Here are some effective strategies to help students master core topics:

• Provide Clear Explanations: Start by breaking down complex topics into smaller, manageable pieces. Simplify explanations and use examples that are relatable to the students’ everyday experiences.
• Encourage Active Participation: Engage students through interactive activities that allow them to apply what they’ve learned. Hands-on exercises, group discussions, and problem-solving sessions help reinforce concepts and make learning more dynamic.
• Promote Consistent Practice: Repetition is key to mastering new skills. Provide students with a variety of exercises that gradually increase in difficulty to help them solidify their understanding and build confidence.

In addition to these strategies, it’s important to regularly assess progress and adjust teaching methods accordingly. Students learn at different rates, so identifying areas where they may struggle allows for timely intervention. By focusing on these core topics and providing a structured learning environment, educators can ensure that students develop the necessary skills to succeed in the future.

Parent Strategies for Supporting Learning

Parents play a crucial role in supporting their child’s educational journey. By creating a positive and nurturing learning environment at home, they can help their children develop the skills and confidence they need to succeed. In addition to providing emotional support, parents can use various strategies to actively engage in their child’s learning process.

Creating a Productive Study Environment

A well-organized, quiet, and distraction-free space is essential for focused learning. Here are some practical tips for parents to create the ideal study area:

• Designate a Specific Space: Choose a quiet corner or room free from distractions, like TV or noise, where the child can focus on tasks.
• Ensure Proper Lighting: Make sure the study area is well-lit to reduce eye strain and encourage concentration.
• Provide Necessary Materials: Keep all required tools, such as pencils, paper, erasers, and calculators, within easy reach to minimize interruptions.

Effective Communication and Encouragement

Regular communication between parents and children about their academic progress can make a significant difference. Here are some ways parents can enhance their involvement:

• Set Realistic Expectations: Encourage progress, not perfection. Set achievable goals and celebrate milestones to boost the child’s confidence.
• Ask Questions: Engaging in open conversations about schoolwork helps parents understand their child’s challenges and strengths. Ask about what they learned each day and if they encountered any difficulties.
• Offer Positive Reinforcement: Praise efforts, not just results. Positive reinforcement motivates children to keep working hard and builds their self-esteem.

By staying involved and providing consistent support, parents can help foster a love of learning in their children, encouraging them to overcome challenges and take pride in their educational achievements.

What to Do If Answers Are Incorrect

Encountering mistakes during learning is a natural part of the educational process. It’s important to approach incorrect responses not as failures, but as opportunities for growth and improvement. Understanding where mistakes occur allows students to identify gaps in their understanding and refine their problem-solving strategies. With the right approach, these setbacks can be valuable learning experiences.

Review the Problem Step by Step

When the result doesn’t match the expected outcome, it’s essential to go back and review each step carefully. Often, the error lies in a small misstep or a misunderstanding of the instructions. Here’s how to identify and correct mistakes:

• Check for Misinterpretation: Re-read the question or task to ensure there was no misunderstanding about what was being asked.
• Reevaluate Each Step: Walk through the process again, ensuring that each step was followed correctly. Look for any skipped steps or incorrect calculations.
• Identify Patterns of Mistakes: If similar errors occur repeatedly, focus on the specific area where the issue lies and practice those concepts more thoroughly.

Seek Assistance and Additional Practice

If the mistakes continue, it may be helpful to ask for guidance. Teachers, tutors, or even parents can provide valuable insights into where things went wrong and suggest strategies for improvement. Here are some ways to move forward:

• Ask for Clarification: Don’t hesitate to seek help from someone who can explain the concept in a different way. A fresh perspective can make a difference in understanding.
• Practice Similar Problems: Additional practice can solidify concepts and provide confidence in the material. Completing more exercises helps reinforce the correct methods.
• Stay Positive: Mistakes are part of learning, and it’s important to maintain a positive attitude. Focus on the progress made and keep practicing for improvement.

By approaching mistakes thoughtfully and seeking help when needed, students can build resilience and improve their skills over time. Mistakes are a crucial part of the learning journey, and each one is a step toward mastery.

Key Learning Objectives for Module 5

In this section, students focus on understanding fundamental concepts that will help them solve problems more effectively. The primary objectives are designed to guide learners through the essential skills and techniques they need to grasp. Achieving these goals ensures a solid foundation for more advanced topics and strengthens problem-solving abilities.

Understanding Core Concepts

Students are introduced to several core ideas that are crucial for their overall development in this subject. The following are some of the key concepts that they will master:

• Building Fluency with Basic Operations: Students practice addition, subtraction, multiplication, and division with increasing complexity.
• Applying Logical Thinking: Problem-solving exercises focus on developing critical thinking and logical reasoning skills.
• Recognizing Patterns: Learners identify patterns in numbers and operations to make predictions and solve problems efficiently.

Practical Application of Skills

In addition to mastering theoretical concepts, students are encouraged to apply what they’ve learned to real-life scenarios. This approach helps them see the relevance of what they are studying and enhances their understanding. The objectives in this area include:

• Word Problems: Students tackle real-world problems that require them to apply their knowledge of basic operations and reasoning.
• Problem-Solving Strategies: Encouraging students to break down complex problems into smaller, manageable steps helps improve their overall approach to challenges.
• Visual Representation: Students use charts, graphs, and number lines to represent problems visually, making abstract concepts more concrete.

By the end of the unit, students will have a clear understanding of these key objectives, allowing them to tackle increasingly complex tasks with confidence and skill.

Understanding Common Math Errors

When students encounter challenges while solving problems, it’s important to identify and address the most frequent mistakes. Recognizing these errors can provide valuable insight into a student’s thought process and help in guiding them toward the correct approach. By understanding the underlying causes of these missteps, educators and parents can offer targeted support to improve accuracy and confidence.

Here are some of the most common errors that students often make:

• Misreading the Problem: Students may overlook key details in a question, leading to incorrect calculations or inappropriate methods being applied.
• Forgetting to Follow Order of Operations: In more complex problems, students sometimes ignore the order in which operations should be performed, leading to wrong results.
• Making Simple Calculation Mistakes: Often, basic arithmetic errors occur due to carelessness or rushing through problems without double-checking the work.
• Incorrectly Applying Formulas: When formulas are involved, students might misunderstand the components or apply them incorrectly, causing errors in the final solution.
• Overlooking Units: Especially in word problems involving measurement, students may forget to include or convert units, resulting in an incomplete or inaccurate answer.

By understanding and addressing these common mistakes, students can develop stronger problem-solving habits and become more proficient in their work. With practice, they will learn to avoid these errors and build a deeper understanding of the concepts being taught.

Benefits of Self-Checking Work in Math

Self-checking is a vital skill for students, allowing them to review their own work and catch mistakes before finalizing answers. This practice not only enhances accuracy but also fosters independence and builds confidence in problem-solving abilities. By regularly self-checking their work, students gain a deeper understanding of the material and develop critical thinking skills that are essential for long-term success.

Here are some key benefits of self-checking:

• Improved Accuracy: By reviewing their solutions, students are more likely to identify errors and correct them, leading to more accurate results.
• Enhanced Understanding: The process of checking work forces students to reconsider their approach and reinforces their grasp of the concepts.
• Increased Confidence: When students consistently verify their own answers, they feel more confident in their ability to solve problems on their own.
• Development of Critical Thinking: Self-checking encourages students to think critically about their methods and reasoning, helping them refine their problem-solving strategies.
• Better Time Management: Learning to quickly identify and correct mistakes can save time in the long run, allowing students to focus on learning rather than correcting major errors.

Incorporating self-checking into regular practice not only leads to higher-quality work but also cultivates habits that contribute to greater success in future learning experiences.

Preparing for Tests with Module 5

Effective test preparation is essential for ensuring students are confident and well-equipped to demonstrate their understanding. The process involves revisiting key concepts, practicing problems, and identifying areas that require further attention. By breaking down the content into manageable sections and focusing on the most challenging topics, students can approach their exams with clarity and assurance.

Key Strategies for Test Preparation

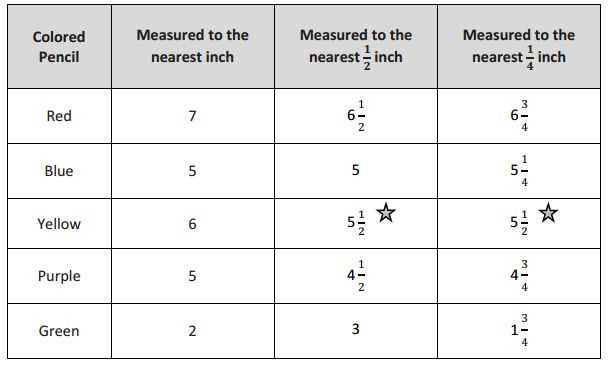
There are several strategies that can help students prepare efficiently:

• Review the Material: Go over all the topics covered, paying special attention to areas that were particularly challenging or complex.
• Practice Regularly: Complete exercises and practice problems to reinforce skills and ensure familiarity with various types of questions.
• Focus on Weak Areas: Identify areas where mistakes were made previously and dedicate extra time to mastering those concepts.
• Take Mock Tests: Simulate the test environment by taking practice tests under timed conditions to build test-taking stamina and reduce anxiety.
• Use Study Aids: Utilize study resources such as worksheets, review books, or online platforms to get additional practice and explanations.

Maintaining a Positive Mindset

Test preparation is not just about working through problems–it’s also about mental preparation. Encouraging students to stay positive, manage their stress, and approach the test with a clear mind can significantly impact their performance. Remind them that preparation is key and that each challenge is an opportunity to learn and grow.

With the right approach, students can feel ready to tackle the exam and show their full potential, turning preparation into a successful experience.