Math U See Algebra 1 Answer Key Guide

In the world of mathematics, accurate solutions are crucial to mastering complex concepts. Having a reliable reference can make all the difference in building confidence and understanding. This guide offers a comprehensive resource to assist in verifying and correcting your calculations, ensuring a deeper grasp of the material.

Whether you are a student tackling exercises independently or a tutor supporting a learner, a structured guide to solutions can enhance the learning process. By comparing your work with detailed explanations, you gain valuable insight into problem-solving techniques and strategies.

Utilizing a step-by-step approach helps identify areas for improvement and accelerates progress. With this resource, you can strengthen your skills and boost your confidence, ultimately leading to greater success in mathematics.

Math U See Algebra 1 Answer Key Overview

This section provides a detailed look at the solution guide for one of the most widely used educational programs. Designed to support learners in their mathematical journey, this resource is invaluable for both self-study and tutoring purposes. It serves as a structured reference for verifying calculations and understanding the steps behind problem-solving techniques.

With an organized approach, the guide enables students to track their progress and identify areas needing further review. Each solution is presented clearly, offering step-by-step explanations that highlight essential strategies for tackling each type of problem. This not only reinforces key concepts but also fosters a deeper understanding of mathematical principles.

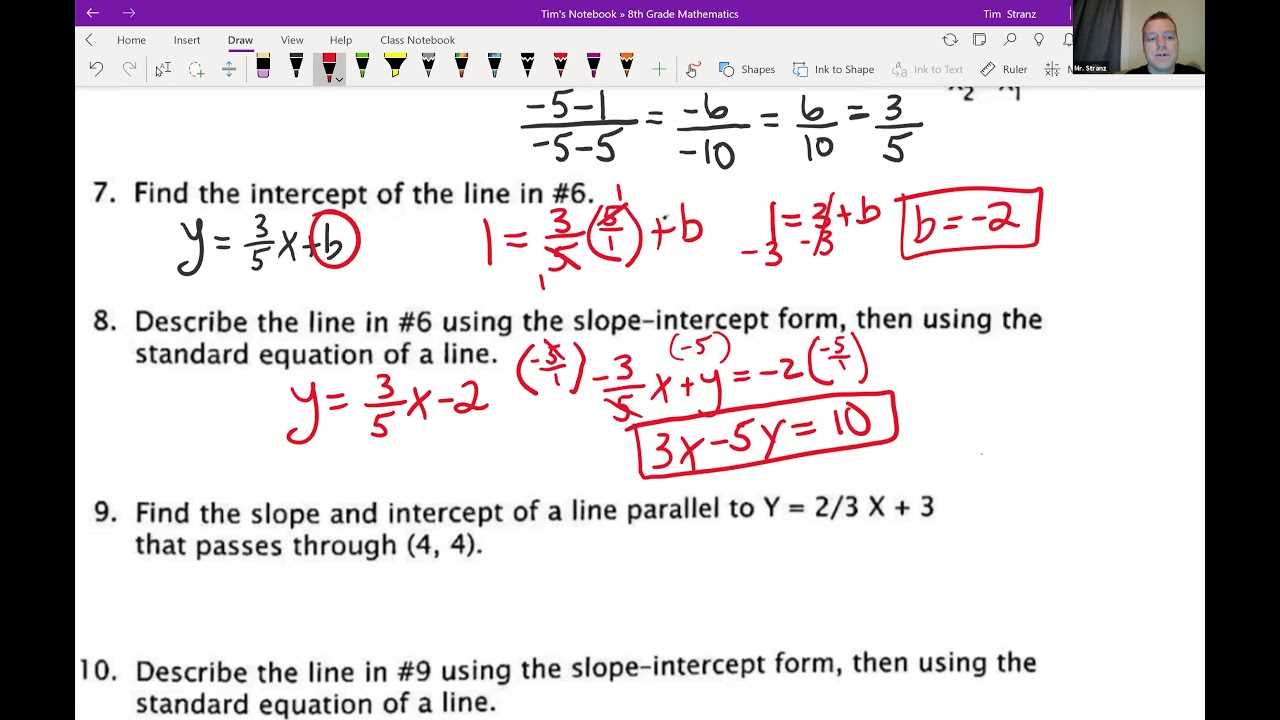
Structure of the Solution Guide

The guide is divided into sections based on the topics covered in the program. Each section includes a variety of practice problems followed by detailed solutions. This format allows learners to engage with the material actively, ensuring they gain both the practice and the feedback necessary for success.

Benefits of Using the Solution Guide

Using a comprehensive solution guide like this one offers multiple advantages. Students can work through exercises at their own pace while having access to clear, accurate solutions for reference. This helps build confidence and mastery, as students are encouraged to solve problems independently before checking their work.

Topic	Number of Problems	Solution Availability
Linear Equations	20	Full Solutions
Quadratic Equations	15	Step-by-Step
Polynomials	18	Detailed Explanations
Exponents	12	Complete Breakdown

Understanding the Curriculum Structure

This educational program is designed with a clear, methodical framework that ensures steady progress for learners. Each unit builds on previous concepts, allowing students to develop their skills in a logical, incremental way. The structure is intended to provide a balanced approach to learning, combining both theory and practice.

The content is divided into several core areas, each focusing on a specific aspect of mathematical reasoning. Starting with foundational concepts, the curriculum gradually introduces more complex topics, giving students ample opportunity to master each stage before moving on. By the end of the course, learners will have a solid understanding of the key principles needed for advanced study in mathematics.

Why Choose Math U See for Algebra?

This program offers a unique approach to mastering foundational mathematical concepts, making it an excellent choice for learners at various stages of their education. By focusing on clarity and step-by-step development, it ensures that students can tackle complex problems with confidence. Whether you’re a beginner or need additional practice, the program’s structured method provides the support needed to succeed.

Key Benefits of the Program

• Progressive Learning: The curriculum is designed to build on previous lessons, allowing learners to gradually develop their skills.
• Clear Explanations: Each topic is explained in a simple and understandable way, making even the most challenging subjects more approachable.
• Hands-On Practice: Regular exercises allow students to apply what they’ve learned and gain practical experience.
• Comprehensive Support: Detailed solutions and guidance help reinforce concepts and provide clarity when needed.

Why This Approach Works

The program’s focus on mastery rather than speed means that students are encouraged to fully understand each concept before moving on to the next. This method leads to a deeper grasp of mathematical principles and fosters long-term retention. Additionally, the visual and hands-on elements incorporated into lessons cater to different learning styles, making the content accessible to a wider range of students.

How to Use the Answer Key Effectively

Utilizing a solution guide can greatly enhance your learning process if approached correctly. Rather than simply copying the answers, it’s important to understand the reasoning behind each step. This method helps you identify and correct any mistakes, strengthening your problem-solving skills and deepening your comprehension of the material.

Start by attempting the problems on your own before consulting the guide. This ensures that you actively engage with the material and identify areas where you might need help. Once you check your work, carefully review the provided solutions and compare them with your own approach.

Focus on the process, not just the result. Pay attention to how each problem is solved, looking for patterns or strategies that can be applied to future exercises. If a particular step or method is unclear, take the time to research it or seek additional resources. This approach allows you to learn from your mistakes and avoid repeating them in the future.

Incorporating this practice into your study routine not only improves your accuracy but also boosts confidence in tackling more complex tasks. Remember, the goal is mastery, and using the solution guide as a tool for self-assessment is key to achieving that success.

Key Topics Covered in Algebra 1

This educational program covers a range of essential concepts that lay the foundation for more advanced mathematical studies. Each topic is designed to build a deeper understanding of mathematical operations and their real-world applications. The lessons progress from basic principles to more complex problem-solving techniques, ensuring students are well-prepared for further studies.

Core Concepts and Skills

• Equations and Inequalities: Understanding how to solve and manipulate linear equations and inequalities is fundamental to mathematical reasoning.
• Functions: Learning about different types of functions and their properties, including how to graph and analyze them, is a key component.
• Polynomials: Identifying, simplifying, and factoring polynomial expressions is a crucial skill in solving more complex equations.
• Rational Expressions: Simplifying and performing operations with rational expressions is covered in detail to prepare for future studies in calculus.
• Exponents and Radicals: Understanding the laws of exponents and working with square roots and other radical expressions are essential for solving more advanced problems.

Additional Important Topics

• Systems of Equations: Methods for solving systems of linear equations, such as substitution and elimination, are introduced and practiced.
• Quadratic Equations: Students learn to solve quadratic equations using various techniques, including factoring, completing the square, and the quadratic formula.
• Sequences and Series: The course introduces arithmetic and geometric sequences, focusing on finding terms and solving related problems.
• Graphing Techniques: Techniques for graphing equations and interpreting the results are covered, including plotting linear and quadratic graphs.

Benefits of Having an Answer Key

Having a solution guide is an essential tool for reinforcing learning and ensuring accuracy in mathematical work. It provides immediate feedback, helping learners identify mistakes and correct them promptly. This support not only boosts confidence but also accelerates understanding of concepts by offering a clear path to the correct solution.

One of the key advantages of having access to a solution reference is the ability to independently check one’s work. Instead of relying on external help, students can verify their solutions, which promotes self-sufficiency and builds critical thinking skills. Moreover, this immediate feedback loop enables quicker learning and mastery of more complex problems.

Another benefit is the opportunity for review and reinforcement. By analyzing the detailed steps provided in the guide, students can better understand the reasoning behind each solution. This helps deepen their comprehension and ensures they don’t just memorize answers but understand the methods behind them. Such understanding is crucial for applying these concepts in different contexts and future studies.

How to Check Your Algebra Solutions

Verifying your work is a crucial step in the learning process. It helps ensure that your solutions are accurate and that you fully understand the methods used to reach them. Checking your solutions also allows you to spot any errors early, which is key to improving your problem-solving skills over time.

Steps to Verify Your Work

Start by reviewing the problem carefully to make sure you have interpreted it correctly. Double-check the values and terms involved, ensuring that there are no mistakes in how you’ve set up the equation. After that, go through your steps methodically, retracing each calculation. Compare your process with the correct solutions to see if you missed any steps or applied the wrong methods.

Common Techniques for Verifying Solutions

• Substitution: Plug your solution back into the original equation to see if it satisfies the conditions.
• Graphing: For equations involving variables, graphing can be a helpful way to visually verify the accuracy of your solution.
• Cross-checking with Different Methods: Solve the problem using a different technique (e.g., factoring vs. quadratic formula) to see if you arrive at the same result.

By following these steps and using these techniques, you can ensure your solutions are correct, reinforcing your understanding of the material and building confidence in your problem-solving abilities.

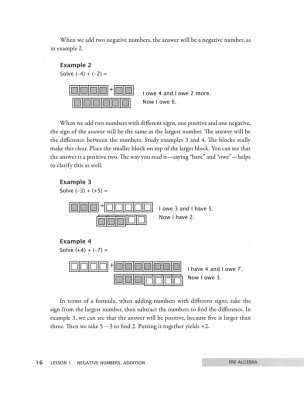
Common Mistakes and How to Avoid Them

When working through mathematical problems, it’s easy to make errors, especially when dealing with complex steps or unfamiliar concepts. Identifying and understanding these common mistakes is essential for improving accuracy and building stronger problem-solving skills. By recognizing these pitfalls, you can take proactive steps to avoid them and ensure more reliable results.

Frequent Errors and How to Prevent Them

• Sign Mistakes: One of the most common mistakes is misplacing or ignoring negative signs. Always double-check your signs, especially when adding or subtracting terms with different signs. A good practice is to highlight negative numbers to avoid confusion.
• Misapplying Operations: Sometimes, it’s easy to forget to apply certain operations, like distributing a number across parentheses. Review each step carefully, ensuring that all operations are properly applied. Write out the steps in full to avoid skipping any parts.
• Skipping Steps: In an effort to work quickly, it’s tempting to skip steps or skip reviewing intermediate results. Make sure to follow each step methodically and verify your calculations as you go. Rushing can lead to unnecessary mistakes.
• Incorrect Factoring: Factoring can be tricky, especially when dealing with polynomials. Ensure you check your factors by expanding them back out to verify their correctness.

How to Avoid These Mistakes

• Take Your Time: Don’t rush through the problems. Allow yourself time to understand each step and recheck your work for any inconsistencies.
• Break Down Complex Problems: If a problem seems overwhelming, break it into smaller, more manageable parts. Tackle each part systematically and reassemble the solution step by step.
• Use Visual Aids: Sometimes drawing out equations or graphs can help you spot mistakes and visualize solutions more clearly.

By staying attentive to these common pitfalls and implementing strategies to avoid them, you can greatly improve your accuracy and mastery of the material.

Improving Algebra Skills with Answer Keys

Using a solution guide is an effective way to enhance your mathematical abilities. By reviewing solutions, students can pinpoint errors, understand the reasoning behind each step, and gradually improve their problem-solving techniques. The key to effective learning is not just finding the correct answer but understanding the process involved in arriving at it.

Analyzing Solutions for Better Understanding

When you compare your work to the provided solutions, take the time to carefully analyze each step. Focus on how the solution is structured, and note the different methods used to simplify or solve the problem. This deeper review helps in recognizing patterns and strategies that can be applied to similar problems in the future. It’s important to focus on understanding the logic behind the solution, rather than simply memorizing the outcome.

Building Confidence Through Practice

Frequent practice combined with solution references enables you to identify areas of improvement. By regularly working through problems and checking your results, you can build confidence in your skills. Repeatedly solving problems while using the guide to verify your work helps reinforce concepts and improve retention, making it easier to tackle more complex challenges over time.

How to Interpret the Solutions Correctly

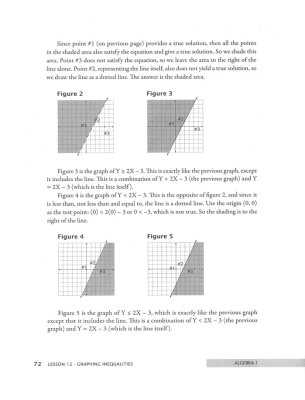
Understanding the provided solutions is an essential part of mastering any subject. Simply looking at the final result does not always offer a complete picture. It’s important to focus on the process behind the solution to truly grasp the concepts being applied. Interpreting each step correctly allows you to see the logic behind the solution and apply similar techniques in future problems.

When analyzing solutions, it’s crucial to follow each step carefully. Pay attention to how equations are manipulated, how operations are performed, and how intermediate steps lead to the final result. This process ensures that you’re not just memorizing answers, but truly understanding the methodology behind them.

Key Elements to Consider

Step	Explanation
Starting Point	Identify the initial setup of the equation and the given values. Ensure you understand what is being asked.
Operations	Note the operations performed at each stage (addition, subtraction, multiplication, etc.) and why they are necessary.
Final Result	Once the solution is reached, confirm that it satisfies the original problem. Revisit any calculations that seem unclear.

By breaking down each step and analyzing it thoroughly, you gain a deeper understanding of the approach and can better apply these techniques to new problems.

Practical Tips for Algebra 1 Success

Achieving success in any mathematical subject requires a combination of understanding core principles, practicing regularly, and developing problem-solving strategies. To excel, it’s crucial to approach each topic methodically, ensuring that foundational concepts are mastered before moving on to more complex problems. With the right techniques, anyone can improve their skills and gain confidence in their abilities.

Building a Strong Foundation

Start by mastering the basic concepts. Whether it’s understanding variables, operations, or the rules of equations, having a solid grasp of the fundamentals is essential. Revisit earlier lessons if necessary and make sure you understand each component before proceeding. This foundational knowledge will help you solve more challenging problems with greater ease.

Consistent Practice and Review

Regular practice is key to reinforcing what you learn. Set aside time each day to work through problems, ensuring that you are consistently applying the techniques you’ve learned. After completing exercises, always review your work–check your solutions, identify any mistakes, and understand why those errors occurred. This process of continuous improvement will help you retain knowledge and enhance your problem-solving abilities.

The Role of Practice in Mastery

To truly master any subject, consistent practice is essential. Repeatedly applying the concepts learned allows you to internalize methods and strategies, turning theoretical knowledge into practical skills. By regularly working through problems, you build the confidence and competence needed to tackle more difficult tasks with ease.

Reinforcing Knowledge Through Repetition

Every time you solve a problem, you are reinforcing your understanding of the core principles involved. It’s not just about memorizing formulas or techniques; it’s about applying them in various contexts until they become second nature. This continuous exposure helps solidify your skills, making complex problems more approachable.

Building Confidence and Problem-Solving Ability

Through regular practice, you become more comfortable with problem-solving. As you encounter different types of challenges, you develop strategies for approaching them. With each successful solution, your confidence grows, making it easier to take on more advanced material. The more you practice, the more intuitive the process becomes.

How to Tackle Complex Algebra Problems

Confronting difficult problems can often feel overwhelming, but breaking them down into manageable parts is key to finding a solution. By approaching each challenge methodically, you can simplify even the most complicated tasks. With the right strategies and mindset, solving tough problems becomes a step-by-step process that leads to success.

Start with a Clear Understanding of the Problem

Before jumping into calculations, take a moment to fully understand what the problem is asking. Identify the known and unknown variables, and ensure that you comprehend the relationships between them. A clear grasp of the question sets the foundation for a logical approach and prevents unnecessary mistakes.

Break the Problem into Smaller Steps

Instead of trying to solve the entire problem at once, break it down into smaller, more manageable parts. Tackle each piece one at a time, applying the appropriate techniques to simplify the task. This method not only makes the process less daunting but also helps maintain focus and clarity as you work toward the final solution.

By practicing this systematic approach, you can steadily build the confidence and problem-solving skills needed to handle increasingly challenging questions. The more you practice, the more natural it becomes to apply these techniques in a variety of contexts.

What to Do After Using the Answer Key

After reviewing the solutions provided, it’s important to take a few steps to ensure that the learning process continues effectively. Simply checking answers isn’t enough for true mastery. Reflecting on the work, identifying mistakes, and reinforcing understanding will help solidify your knowledge and improve problem-solving skills in the future.

Review Your Work Carefully

Before moving forward, take the time to carefully compare each solution with your own work. Look for discrepancies and try to understand why the answer you obtained differs from the provided one. This reflection process is crucial in pinpointing any misunderstandings and correcting them.

Identify and Learn from Mistakes

• Pinpoint where you made an error and understand the reason behind it.
• Revisit the concept or method you struggled with to reinforce your understanding.
• Consider alternative approaches or strategies to ensure better results next time.

Once mistakes are identified, it’s important to correct them and practice similar problems to reinforce new insights. This method helps in preventing the same errors from occurring again and builds stronger problem-solving habits.

Practice More Problems to Reinforce Understanding

To truly grasp the material, it’s essential to apply what you’ve learned. Practice additional problems that are similar but not identical to those you have already solved. This will help strengthen your skills and ensure that the knowledge stays fresh, allowing for better retention and greater mastery.

Using the Answer Key for Self-Assessment

Using a solution guide can be an effective tool for self-assessment. It allows learners to evaluate their understanding of concepts, track progress, and identify areas that need improvement. By checking the results of exercises or problems, individuals can gain insights into their strengths and weaknesses, helping to direct their study efforts more efficiently.

Reflect on Your Problem-Solving Process

Before looking at the solutions, take some time to evaluate your approach to solving the problems. Consider how you approached each step and whether there were moments where you felt uncertain or needed to make assumptions. This self-reflection can provide valuable feedback on the methods you are using, and can highlight areas where your understanding may need reinforcement.

Track Progress and Identify Patterns

• Compare your results over time to track improvements or recurring challenges.
• Notice any patterns in the types of problems you struggle with the most.
• Assess whether you are becoming more efficient in your problem-solving approach.

Using the guide regularly for self-assessment helps build a better understanding of personal strengths and areas for growth. With each assessment, learners can develop more effective strategies for improvement, leading to stronger skills in the subject overall.

How to Prepare for Algebra 1 Tests

Preparing for a test in a subject that involves problem-solving and logical reasoning requires a strategic approach. It’s important to review key concepts, practice different types of problems, and ensure that you understand both the theory and application of the material. Effective preparation will help build confidence and improve performance when it comes time for the assessment.

Review Core Concepts

Start by revisiting the main principles and formulas that are essential to the subject. Focus on:

• Identifying key rules and operations that form the foundation of the material.
• Reviewing any examples discussed in class or textbook exercises.
• Rewriting formulas and creating a reference sheet for quick access.

Practice Consistently

Repetition is crucial for reinforcing understanding and improving accuracy under pressure. Use a variety of practice methods, such as:

• Working through practice problems that test different aspects of the topic.
• Attempting previous test papers or exercises from your study materials.
• Exploring additional online resources or problem sets to broaden your exposure to different types of questions.

Focus on Weak Areas

Identify the areas where you struggle the most and dedicate extra time to improving them. Common strategies include:

• Breaking down complex problems into smaller, manageable steps.
• Seeking help from a tutor or peers to clarify misunderstandings.
• Using visual aids or diagrams to better grasp difficult concepts.

With focused review, consistent practice, and targeted improvement, you can enhance your preparedness and boost your chances of success on the test.

Enhancing Your Math Study Routine

Developing an effective study routine is essential for mastering any subject that involves logical reasoning and problem-solving. A structured and consistent approach to learning can greatly improve your understanding of key concepts and your ability to apply them accurately. By refining your study habits, you can boost retention, reduce stress, and increase overall performance.

Set Clear Goals

Establish specific, measurable, and time-bound objectives for each study session. Having clear goals helps you stay focused and organized. These might include:

• Completing a set number of practice problems.
• Understanding a particular concept or solving a type of problem.
• Reviewing and reinforcing learned material from previous lessons.

Active Learning Techniques

Instead of passively reading through notes or textbooks, engage with the material actively. Active learning helps reinforce understanding and makes complex topics easier to grasp. Some techniques to try include:

• Solving problems on your own before checking solutions.
• Explaining concepts to others or teaching them out loud.
• Using flashcards or quizzes to test your knowledge and memory.

Create a Distraction-Free Environment

Minimize distractions by setting up a dedicated study space. An optimal environment can help you focus and increase productivity. Consider the following:

• Choose a quiet location with minimal interruptions.
• Turn off unnecessary devices and notifications.
• Organize your study materials and have everything you need at hand.

Review and Reflect Regularly

Make it a habit to regularly review what you’ve learned. This reinforces your understanding and helps identify areas that may need further attention. Reflect on your progress and adjust your study approach if necessary. Regular revision will keep your knowledge fresh and build long-term retention.

By following these strategies, you can optimize your study sessions, reduce the time spent struggling with difficult topics, and achieve greater success in your studies.