Mathnation Geometry Answer Key and Solutions Guide

When tackling mathematical exercises, having access to reliable resources is crucial for understanding and verifying your work. With the right set of tools, you can easily break down complex calculations and find effective solutions. This section is dedicated to providing a deeper understanding of how you can approach various problems in the field of mathematics.

By using well-structured guides and resources, learners can gain a better grasp of essential concepts and improve their problem-solving skills. The goal is to equip you with the knowledge to solve challenges with confidence, ensuring that you can tackle assignments and tests with ease.

Helpful resources provide step-by-step explanations, allowing individuals to check their work and learn from any mistakes. These materials are designed to reinforce understanding and support continuous improvement, especially for those looking to master mathematical principles.

Mathnation Geometry Answer Key Overview

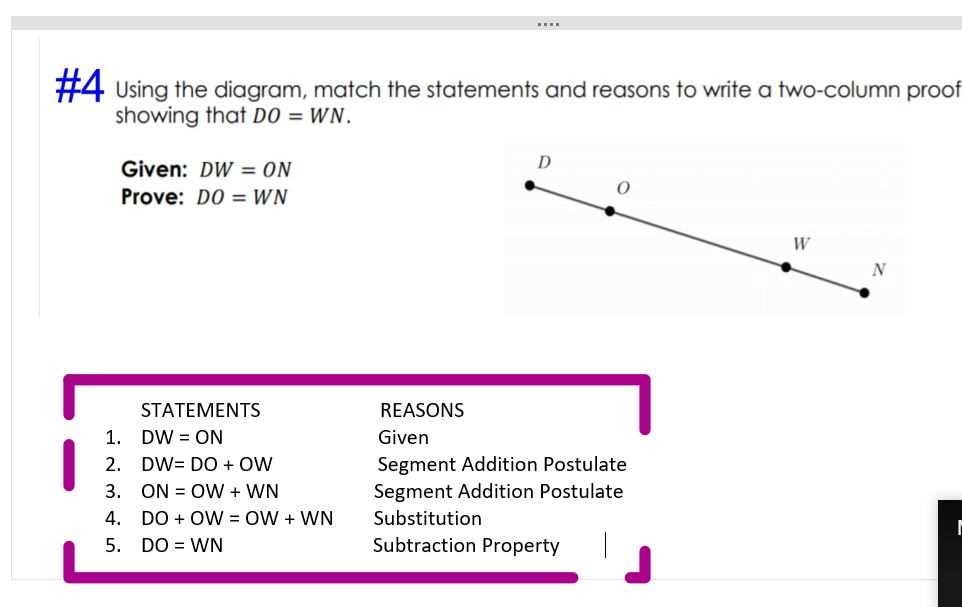
In any field of study, having access to reliable resources for reviewing and verifying your work is essential for achieving mastery. For learners facing mathematical challenges, structured solutions play a key role in understanding complex problems and refining techniques. This section highlights how these valuable tools can help clarify concepts and reinforce learning.

With a clear and systematic approach, students can easily find detailed explanations for a wide range of exercises. By referencing the step-by-step solutions provided, learners gain the ability to identify errors, correct misunderstandings, and develop stronger problem-solving strategies. The ultimate goal is to foster self-confidence and a deeper understanding of mathematical principles.

These resources are designed to be both accessible and comprehensive, offering solutions to the most commonly encountered issues. Whether used for independent study or as a supplement to lessons, they serve as a guide to navigate the complexities of mathematical challenges with ease and precision.

How to Access the Answer Key

Accessing reliable solutions for your mathematical exercises is essential for reviewing and improving your understanding. To ensure you have the correct resources at hand, there are several straightforward methods to find these solutions online and offline. Here are some effective ways to gain access to the resources you need:

• Visit trusted educational websites that offer free or paid resources.
• Sign up for platforms that provide detailed guides for various mathematical topics.
• Use library subscriptions to academic resources and databases for textbook solutions.
• Check for digital study tools and apps that include built-in solution guides.
• Join online forums or student communities that share solutions and tips.

Once you have located a reliable source, make sure to review the solutions carefully. It’s important not only to check your results but also to study the process involved in each solution. This way, you can strengthen your understanding and apply similar techniques to other exercises.

Step-by-Step Guide to Solutions

Having a clear, organized approach to solving complex problems is essential for mastering any subject. A step-by-step guide allows you to break down each task into manageable parts, making it easier to understand the process and arrive at the correct results. This method not only helps in finding the right answers but also in reinforcing the underlying concepts that will aid future problem-solving.

Each solution typically follows a logical sequence, starting with identifying the key elements of the problem. From there, the appropriate steps are outlined in a way that ensures clarity and progression. By following this structured approach, learners can gradually build their skills, improve accuracy, and gain confidence in tackling more advanced challenges.

It’s important to take your time with each step, review your work, and ensure that each part of the solution aligns with the overall objective. This practice will help you develop a deeper understanding and improve your ability to solve similar problems in the future.

Understanding Geometry Concepts Easily

Mastering mathematical principles can sometimes feel overwhelming, but with the right approach, even the most complex concepts can be broken down into simpler, more understandable pieces. By focusing on the core ideas and building a strong foundation, students can improve their comprehension and problem-solving skills. The key to success is understanding the “why” behind each concept, rather than just memorizing formulas or steps.

Simplifying Complex Problems

When faced with challenging tasks, it’s helpful to take a step back and identify the basic elements involved. Often, breaking a problem into smaller, more digestible parts makes it easier to see patterns and relationships. This method helps in approaching each question systematically and finding the most efficient path to a solution.

Practical Tips for Learning

One effective way to understand concepts is to apply them to real-life situations. Using tangible examples helps reinforce abstract ideas, making them more relatable. Additionally, practicing regularly and reviewing common problems can further deepen understanding, allowing you to grasp more complex theories with ease.

Common Geometry Problems Explained

When working with mathematical exercises, certain challenges frequently arise. These problems, while appearing complex, often share common patterns that can be solved using similar strategies. By recognizing these recurring issues and understanding their solutions, learners can improve their ability to tackle new, more difficult questions with confidence.

Identifying Basic Shapes and Properties

One of the most fundamental aspects of solving mathematical problems is understanding the properties of shapes. Identifying key features such as angles, sides, and symmetry is crucial for approaching many exercises. Whether it’s calculating the area of a rectangle or determining the perimeter of a triangle, knowing how to apply these properties is essential.

Working with Measurements and Formulas

Another common challenge is dealing with measurements and applying the correct formulas. Misunderstanding how to calculate distances, areas, or volumes can lead to incorrect results. However, once the correct formula is identified, solving the problem becomes much simpler.

Problem Type	Formula	Explanation
Area of a Rectangle	Area = Length × Width	Multiply the length by the width to find the area.
Perimeter of a Triangle	Perimeter = Side 1 + Side 2 + Side 3	Add the lengths of all three sides to get the perimeter.
Circumference of a Circle	Circumference = 2 × π × Radius	Multiply the radius by 2π to find the circumference.

Benefits of Using the Solution Guide

Utilizing a reliable set of solutions offers numerous advantages when studying complex problems. These resources not only help verify results but also provide a deeper understanding of the steps involved in solving each exercise. By following a structured approach to learning, students can strengthen their skills and gain confidence in their abilities.

One key benefit is the ability to identify mistakes and learn from them. Having access to detailed explanations allows learners to see where they went wrong, making it easier to correct their approach in future tasks. This feedback loop fosters continuous improvement and mastery over time.

Moreover, these guides often present multiple methods for solving the same problem, giving students the flexibility to explore different strategies and find the one that works best for them. This versatility enhances critical thinking and problem-solving abilities, providing a more comprehensive understanding of the subject matter.

How to Verify Your Solutions

Ensuring the accuracy of your results is a crucial step in the learning process. Verification helps confirm that the approach you used is correct and that your final solution is reliable. There are several methods to double-check your work, ensuring you fully understand the process and minimize errors.

First, it’s essential to review the steps you took to arrive at the solution. Going through each stage again can reveal any mistakes or overlooked details. Additionally, comparing your result to known benchmarks or using alternative methods to solve the problem can help validate the correctness of your solution.

Utilize available resources to cross-check your work. Comparing your solution with reference materials or guides that provide step-by-step explanations can confirm whether your method aligns with the correct process. If discrepancies are found, take the time to analyze the differences and refine your approach.

Another effective way to verify solutions is by applying your result to practical scenarios. If your answer holds up under real-world conditions or in different contexts, it serves as a strong indicator that your solution is accurate and reliable.

Tips for Problem Solving in Mathematics

Approaching mathematical problems can be a daunting task, but with the right techniques, it becomes much more manageable. Developing a solid strategy for problem solving not only helps you reach the correct solutions but also builds a deeper understanding of the principles behind each task. Below are several tips to enhance your problem-solving skills and make tackling complex questions easier.

Key Strategies for Success

• Break down the problem: Start by identifying the key elements and breaking the problem into smaller parts. This makes it easier to focus on individual steps without feeling overwhelmed.
• Visualize the problem: Drawing diagrams or sketches can often reveal relationships between different components, helping you better understand how the pieces fit together.
• Work methodically: Take a structured approach by solving the problem step by step. Avoid skipping steps or making assumptions, as this can lead to mistakes.
• Check your work: After completing the problem, review your steps and results to ensure consistency and accuracy. Revisiting your solution can often help identify errors or areas for improvement.

Enhancing Your Problem-Solving Skills

• Practice regularly: The more problems you solve, the more familiar you will become with common techniques and patterns. Continuous practice builds confidence and efficiency.
• Learn from mistakes: Don’t be discouraged by errors. Analyzing mistakes helps you understand where you went wrong and how to avoid similar issues in the future.
• Seek alternative solutions: There is often more than one way to approach a problem. Experiment with different methods to find the most efficient or intuitive solution.

Maximizing Learning with Solution Guides

Utilizing solution guides effectively can significantly enhance your learning experience. These resources not only provide quick access to correct answers but also serve as valuable tools for reinforcing concepts, improving problem-solving skills, and identifying areas that need further study. By using these guides strategically, you can deepen your understanding and accelerate your progress.

To make the most out of these resources, it’s important to focus on more than just verifying your results. The real value lies in analyzing the steps leading to the final solution and understanding the reasoning behind each step. This process helps to build a more solid foundation for tackling future challenges.

Effective Strategies for Using Solution Guides

• Study the process: Instead of simply checking answers, review each step in the solution to understand the logic behind it. This will help you learn how to approach similar problems in the future.
• Identify patterns: Look for recurring methods or techniques in different solutions. Recognizing these patterns will make it easier to apply them when solving new problems.
• Compare your work: After solving a problem, compare your approach with the guide to identify areas where you might have made a mistake or could improve your technique.
• Use as a learning tool: Treat solution guides as a learning aid rather than a shortcut. Use them to reinforce your understanding and gain insight into better problem-solving strategies.

Common Pitfalls to Avoid

• Over-reliance on solutions: While solution guides are helpful, relying too heavily on them can hinder your ability to think critically and solve problems independently.
• Skipping the review: Merely checking the answers without analyzing the steps can prevent you from fully understanding the material and learning from your mistakes.
• Not practicing enough: Just using solution guides without practicing similar problems can lead to surface-level understanding. Consistent practice is key to mastering any topic.

Solution Guide for Mathematics Practice

Having access to a solution guide while practicing mathematics can greatly enhance your learning process. These resources are not only useful for confirming the accuracy of your work but also provide insight into the methods used to reach the final solution. By reviewing each step in detail, learners can improve their problem-solving strategies and deepen their understanding of the concepts being studied.

When used effectively, a solution guide helps reinforce key principles, making it easier to recognize patterns and apply the right techniques to different types of problems. It also serves as a useful tool for self-assessment, allowing students to identify areas where they may need more practice or additional support.

One of the key advantages of having access to such guides is the ability to work at your own pace. You can attempt problems independently and refer to the guide only when necessary, which promotes a balance of independence and support in your learning journey.

Additionally, these guides often offer multiple approaches to solving a single problem, which can be particularly beneficial when you’re looking to explore different strategies or deepen your understanding of a topic.

Key Features of Solution Guides

A well-designed solution guide offers more than just the final results; it provides a comprehensive learning tool that helps students understand how to approach and solve problems effectively. These resources are valuable for both independent study and guided learning, offering insights into the processes that lead to accurate outcomes. Understanding the features of a good solution guide can significantly enhance the learning experience.

Typically, solution guides include step-by-step instructions, explanations of different problem-solving techniques, and sometimes alternative methods for approaching the same problem. They help students develop a deeper understanding of the material by providing context for each step, which is essential for mastering the subject matter.

Key features to look for in a solution guide include:

Feature	Description
Step-by-step solutions	Guides should break down each problem into manageable steps, allowing students to follow along easily and understand how the solution is developed.
Clear explanations	Each step should be explained in simple terms, with a focus on why specific methods are used, helping to reinforce the underlying concepts.
Visual aids	Illustrations, diagrams, or graphs can provide clarity, particularly for problems that involve shapes, measurements, or visual representations.
Multiple approaches	Some guides offer alternative methods for solving the same problem, giving students the opportunity to explore different strategies and deepen their understanding.
Practice problems	Many guides include additional problems for practice, which help solidify the concepts and techniques learned in the main problems.

By utilizing these features, a solution guide becomes more than just a reference for checking answers–it becomes a tool for active learning and problem-solving development.

Using Solution Guides to Improve Grades

Solution guides can be powerful tools for enhancing academic performance. By helping students understand their mistakes and learn the correct methods, these resources provide an opportunity for growth and improvement. When used effectively, solution guides offer more than just the right answers–they help students build the skills and confidence necessary to excel in their studies.

To maximize the benefit of these resources, students should approach them strategically. Instead of just verifying their results, they should focus on understanding the reasoning behind each solution step. This process helps strengthen critical thinking skills and promotes a deeper understanding of the material, ultimately leading to improved performance on future assessments.

How to Use Solution Guides to Boost Grades:

• Review step-by-step: Carefully examine each step in the solution, paying close attention to how the problem is approached and solved. This helps reinforce your understanding and ensures you can replicate the method on your own.
• Learn from mistakes: When you compare your work to the solution guide, take note of any errors you made. Understanding why your approach was incorrect and how to correct it is key to improving your performance.
• Practice consistently: Use the guide to identify areas where you’re struggling, and then focus on practicing similar problems until you’re comfortable with the techniques and strategies.
• Focus on concepts: Rather than memorizing answers, focus on grasping the underlying concepts. This will enable you to solve a wide variety of problems with ease and confidence.

By incorporating these strategies into your study routine, you can significantly improve your academic performance and achieve a deeper understanding of the subject matter.

Real-Life Applications of Mathematics

Mathematical principles are not confined to the classroom–they have a wide range of practical applications in our daily lives. From architecture to technology, these concepts are foundational to numerous fields, helping to solve complex problems and drive innovation. Understanding how these principles are applied in real-world scenarios can deepen one’s appreciation for the subject and demonstrate its relevance beyond theoretical exercises.

Everyday tasks such as measuring space, designing structures, or even navigating with a map rely on mathematical ideas. Professionals across various industries use these skills to optimize designs, create accurate models, and solve practical challenges. The following are just a few areas where mathematical concepts play a crucial role:

• Architecture and Construction: Accurate measurements, angles, and proportions are essential for building stable structures. Engineers and architects use mathematical principles to design everything from bridges to skyscrapers.
• Computer Graphics and Animation: Artists and programmers use math to create realistic computer-generated images, simulate movements, and render three-dimensional objects in films and video games.
• Navigation and Mapping: GPS systems and map-making require calculations involving distances, angles, and scales to provide accurate directions and locations.
• Medical Imaging: Doctors and technicians use mathematical algorithms to analyze images from MRIs, CT scans, and X-rays, helping diagnose diseases and plan treatments.
• Robotics: Engineers use math to program robots for tasks such as assembly, surgery, or exploring hazardous environments, ensuring precision and efficiency.

By recognizing the importance of these applications, students can see how learning mathematical concepts equips them with the tools to solve real-world challenges and make informed decisions in diverse fields.

How Mathematical Solutions Relate to Tests

Mathematical problem-solving is a crucial aspect of tests, especially when it comes to assessing students’ understanding of concepts. The process of arriving at the correct solution is often as important as the final result. Tests are designed to evaluate not just the ability to recall formulas but also the capacity to apply these formulas in different scenarios. Understanding how solutions are structured and how they relate to the problems presented can significantly impact test performance.

Tests typically consist of problems that require applying learned concepts and methods to achieve a correct result. The solutions provided for these problems serve as a guide, helping students evaluate their understanding of the material. When students compare their approach to the solutions, they can identify areas of strength and weaknesses, gaining valuable insights into their progress.

Understanding Test Structure:

Problem Type	Solution Approach	Test Relevance
Calculation-Based	Step-by-step numeric operations	Tests the ability to follow correct procedures and obtain accurate results
Conceptual Reasoning	Applying formulas or theorems	Assesses understanding of core principles and the ability to apply them effectively
Application Questions	Solving real-world problems	Evaluates how well concepts can be applied to practical scenarios

By recognizing how test problems align with real-world solutions and understanding the application of various methods, students can enhance their test-taking strategies. Reviewing the process of solving problems not only boosts confidence but also provides deeper insights into the subjects being tested, ultimately leading to better performance on assessments.

Common Mistakes in Mathematical Problems

When working through mathematical problems, it’s easy to overlook small details or make errors that can lead to incorrect solutions. These mistakes often arise from misunderstandings of the core concepts, rushed calculations, or misapplication of formulas. Recognizing common pitfalls can help improve accuracy and ensure a more thorough understanding of the material.

Frequent Errors to Avoid

• Misunderstanding Formulas: Using the wrong formula for a specific problem or misapplying a formula can lead to inaccurate results. It’s crucial to understand the context in which a formula is used.
• Calculation Errors: Simple mistakes in arithmetic or algebra, such as adding or multiplying incorrectly, can throw off the entire solution.
• Incorrect Assumptions: Sometimes, assuming information not given in the problem, like the shape or size of a figure, can result in incorrect conclusions.
• Overlooking Units: Forgetting to include or convert units can significantly affect the accuracy of a solution, especially in measurement-based problems.
• Not Drawing Diagrams: Skipping the step of sketching a diagram can lead to confusion about relationships between different elements in a problem, making it harder to apply the right methods.

Tips to Prevent Mistakes

1. Double-Check Calculations: Always review your arithmetic and ensure that each step is correct before moving on to the next one.
2. Read the Problem Carefully: Pay attention to the wording and the details provided in the problem to avoid making assumptions.
3. Practice with Different Types of Problems: The more varied your practice, the better you will become at recognizing and solving problems accurately.
4. Use Visual Aids: Drawing diagrams or visualizing the problem can help clarify relationships and avoid misunderstandings.

By identifying these common mistakes and applying strategies to avoid them, you can improve your problem-solving skills and approach mathematical challenges with greater confidence.

How to Study Using the Solution Guide

Studying effectively involves more than just memorizing facts–it requires understanding the underlying concepts and applying them to various problems. A solution guide can be a valuable tool in this process, providing step-by-step instructions that help clarify challenging topics and reinforce learning. By using the guide strategically, students can improve their problem-solving abilities and deepen their comprehension.

Start with the Basics: Begin by reviewing the fundamental concepts and formulas related to the problems you are working on. The guide can serve as a reference to ensure that you are applying the correct principles. It’s important to understand why each step is taken, rather than just following the instructions.

Work Through Examples: Go through sample problems in the guide, and try solving them on your own first. After attempting the problem, check the solution to identify where you might have gone wrong or how you could have approached it differently. This allows you to learn from your mistakes and reinforce your skills.

Use the Guide as a Tool for Review: The guide is not only for solving problems but can also be an excellent review resource. When preparing for tests, use it to practice a variety of problems, focusing on areas where you feel less confident. Repetition of concepts will help solidify your knowledge.

Identify Common Patterns: As you work through different problems, look for patterns in the steps and solutions. Understanding these patterns can help you tackle similar problems more efficiently and give you a clearer sense of how different concepts connect.

Ask Questions: If a solution is unclear or if you’re struggling to understand a particular step, take time to ask questions. Use additional resources, such as textbooks, online forums, or tutors, to clarify concepts that seem confusing. The more you understand the reasoning behind each solution, the more effective your studying will be.

By following these strategies and actively engaging with the solution guide, you can enhance your understanding of challenging topics and develop stronger problem-solving skills. Over time, you’ll build confidence and a deeper appreciation for the subject matter.

Mastering Concepts with the Solution Guide

Achieving mastery in any subject requires consistent practice, deep understanding, and the ability to apply knowledge to a wide range of problems. A well-structured solution guide can help students reach this level of proficiency by breaking down complex concepts into manageable steps and offering clear explanations. With regular use, students can significantly improve their problem-solving skills and gain confidence in their abilities.

Step-by-Step Approach: Begin by focusing on understanding the basic principles before diving into more complex problems. The solution guide offers a structured approach to tackling each problem, allowing you to build a solid foundation before progressing to more advanced topics. As you gain confidence, you’ll be able to apply these foundational principles to new and unfamiliar challenges.

Practice Regularly: Mastery comes with repetition. The more problems you solve, the more familiar you become with the methods and techniques needed to reach the correct solution. By regularly working through exercises in the guide, you reinforce your understanding and start to recognize patterns and strategies that work best for you.

Analyze Mistakes: Mistakes are an inevitable part of learning, and they offer invaluable insights. When you encounter an error, take the time to analyze what went wrong and why. This reflective process helps you to learn from your mistakes, refine your understanding, and ultimately improve your problem-solving abilities.

Challenge Yourself: Once you’ve mastered the basics, challenge yourself with more difficult problems. The solution guide can help you take on advanced topics by providing detailed explanations and suggesting ways to approach complex questions. By pushing your limits, you build critical thinking skills and further deepen your understanding.

By consistently working through the material, analyzing your mistakes, and tackling progressively harder problems, you can master the subject and achieve long-term success. The key to mastering any topic is persistence, and using a structured guide as a resource allows you to progress with confidence and clarity.