Pre Algebra with Pizzazz Answer Key for Page 79
Mathematics can be challenging, especially when faced with new concepts and problem-solving techniques. To make learning easier, it’s important to have access to resources that guide you through each step. These tools help clarify complex problems and provide solutions that not only correct mistakes but also explain the underlying principles. By working through examples and understanding the reasoning behind each solution, learners can gain a deeper grasp of mathematical concepts.
In this article, we will explore helpful methods for solving exercises, focusing on a specific set of practice problems that are designed to strengthen core skills. These problems offer valuable opportunities to practice and refine techniques. We will also highlight the importance of understanding the process of finding solutions, allowing students to build confidence as they work through each challenge.
By breaking down each task carefully, you can gain a solid foundation in key mathematical ideas. Whether you’re struggling with a specific problem or aiming to improve your overall understanding, the approach we will take ensures that you can tackle similar questions with ease in the future. Let’s delve into the details and uncover the best strategies for mastering these essential skills.
Understanding Core Math Concepts
Grasping the fundamental principles of mathematics is essential for solving complex problems. A solid foundation allows students to approach various types of exercises with confidence, recognizing patterns and applying appropriate strategies. Whether working with numbers, equations, or geometric shapes, the goal is to build the ability to think logically and solve problems systematically.
Key concepts such as operations, fractions, and integers form the building blocks of more advanced topics. By mastering these basic ideas, learners can tackle more complicated tasks, gradually developing their problem-solving skills. Understanding the relationships between different mathematical elements is crucial for progress, as it ensures that students can break down challenges into manageable steps.
Practical applications of these concepts in real-life scenarios further reinforce the value of mathematical understanding. From budgeting to analyzing patterns, math provides tools for making informed decisions and solving everyday problems. Therefore, a clear grasp of these core concepts will not only support academic success but also equip individuals with valuable life skills.
How to Use Solutions Effectively
When tackling mathematical exercises, having access to solutions can be a valuable tool for understanding the steps involved in reaching the correct result. However, it’s important to approach these resources strategically to maximize their effectiveness. Simply looking at the final answer without analyzing the process can hinder learning. The key is to use solutions as a guide for better comprehension and improvement.
Here are some tips on how to make the most of these resources:
- Check your work after solving: Once you’ve completed a problem, compare your method and result with the provided solutions. This will help you identify mistakes and learn the correct approach.
- Analyze the steps: Pay close attention to how the solution is structured. Break down each step to understand the reasoning behind it. This will strengthen your problem-solving skills.
- Learn from mistakes: If your solution differs from the provided one, don’t just correct the error. Try to understand why the mistake occurred and how to avoid it in the future.
- Use as a reference: When stuck, refer to the solutions for hints, but try not to rely on them too early. This will help you build your own problem-solving abilities.
- Review similar problems: Once you’ve understood the solution, practice similar exercises to reinforce the method and ensure you can apply it independently.
By following these strategies, you can transform solutions into a powerful learning tool, improving both your understanding and confidence. The goal is not just to get the right answer but to master the process of reaching it. This approach will ultimately enhance your skills and prepare you for more challenging tasks ahead.
Step-by-Step Solutions for Exercises on Page 79
Solving mathematical problems step by step allows for a deeper understanding of the methods and processes involved. By breaking down each task into manageable parts, learners can systematically approach challenges and ensure they grasp the underlying principles. In this section, we will walk through the solutions for a set of exercises, explaining the logic and steps required to reach the correct outcome.
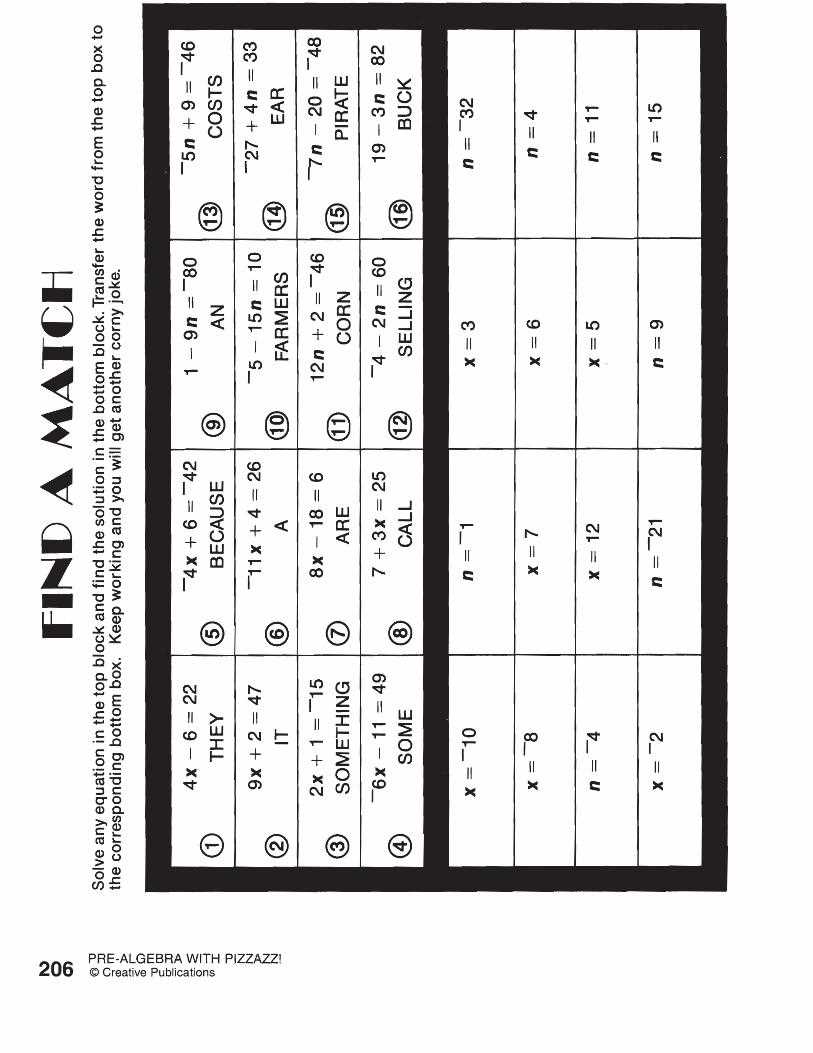
Problem 1: Solving Simple Equations
Let’s begin by solving a basic equation involving integers. The goal is to isolate the variable on one side and simplify the expression.
| Step | Action | Result |
|---|---|---|
| 1 | Start with the equation: 3x + 5 = 14 | 3x + 5 = 14 |
| 2 | Subtract 5 from both sides: 3x = 9 | 3x = 9 |
| 3 | Divide both sides by 3: x = 3 | x = 3 |
Problem 2: Working with Fractions
In the next example, we will simplify a fraction and apply the appropriate mathematical operations to find the solution.
| Step | Action | Result |
|---|---|---|
| 1 | Start with the fraction: 4/6 + 2/3 | 4/6 + 2/3 |
| 2 | Find a common denominator: 4/6 + 4/6 | 8/6 |
| 3 | Simplify the fraction: 8/6 = 4/3 | 4/3 |
By following these steps, you can see how the process works for each type of problem. With consistent practice, these techniques will help you approach even more complex exercises with greater ease and confidence.
Mastering Basic Math Topics Quickly
Learning foundational math concepts efficiently requires a focused approach and regular practice. By understanding key principles and applying them consistently, you can accelerate your mastery and build confidence in solving problems. The key to quick learning is not just memorizing formulas but truly understanding how and why certain methods work. This allows for greater flexibility when facing new challenges.
One effective way to speed up the learning process is to break down complex topics into smaller, more manageable pieces. Start by mastering simple tasks and gradually increase the difficulty level. This approach not only makes learning less overwhelming but also helps to reinforce concepts over time. Regularly revisiting past material will ensure that the foundation remains strong, while you build on it with more advanced techniques.
Another useful strategy is to focus on understanding the “why” behind each method. Instead of relying solely on memorization, try to comprehend the reasoning behind each step of a solution. This deeper understanding will allow you to apply your knowledge more effectively across different types of problems. Furthermore, practicing regularly and seeking out challenges that push your limits will help you progress quickly.
Key Strategies for Solving Math Problems
Solving math challenges requires a systematic approach that combines logic, organization, and a clear understanding of the methods involved. By adopting effective strategies, learners can tackle even the most complex tasks with confidence. These strategies help break down problems into manageable steps, making it easier to find the solution and gain a deeper understanding of the process.
1. Understand the Problem First
Before jumping into solving any problem, take the time to carefully read and understand the question. Identify what is being asked, the information provided, and any constraints or relationships between the elements in the problem. This step ensures that you approach the solution in the right direction.
2. Break Down the Problem into Smaller Steps
Large problems can seem overwhelming, but by dividing them into smaller, more manageable steps, you can simplify the process. Start by focusing on one part of the problem at a time. This method helps to avoid confusion and keeps you on track. It also allows for easier error-checking as you go along.
In addition, always double-check your calculations after each step to avoid small mistakes that can lead to incorrect answers. Consistent practice with these strategies will not only improve your problem-solving skills but also increase your confidence in handling new and more challenging questions.
Common Mistakes to Avoid in Math
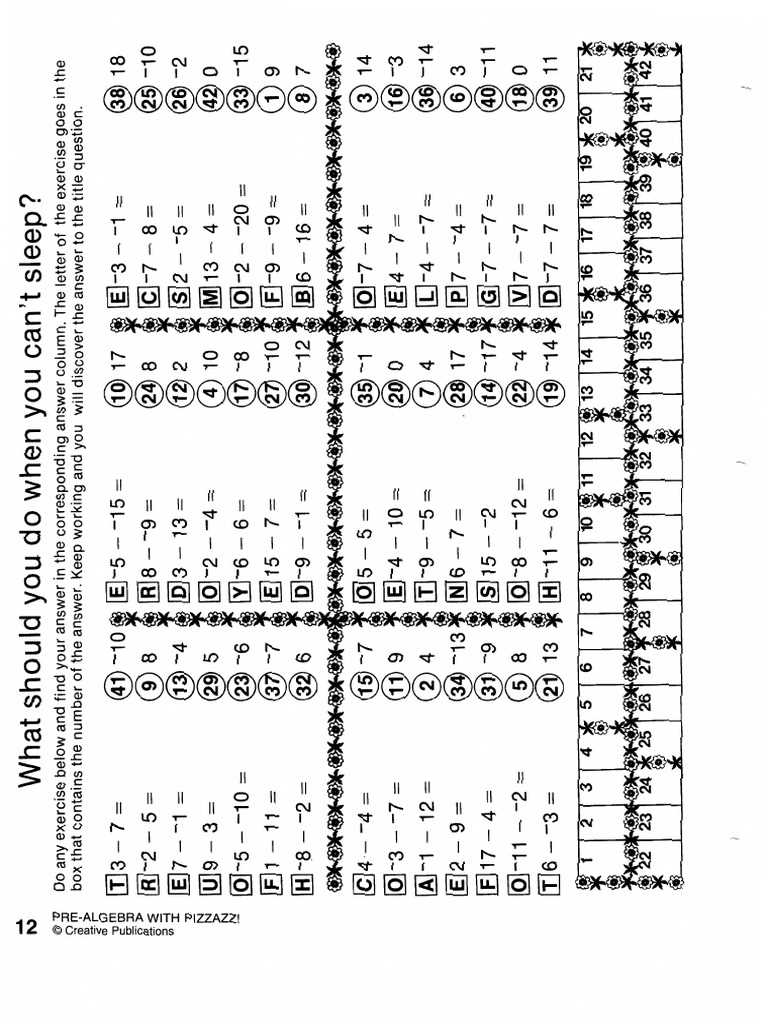
When solving mathematical problems, it’s easy to make small errors that can lead to incorrect results. These mistakes often stem from misunderstandings of the concepts, overlooking key steps, or rushing through calculations. By being aware of the most common pitfalls, learners can avoid them and improve their problem-solving accuracy. It’s important to recognize these mistakes early to prevent them from becoming habits.
One frequent mistake is misapplying mathematical operations. For example, failing to correctly follow the order of operations can lead to inaccurate answers. Always remember to perform tasks like multiplication and division before addition and subtraction unless parentheses indicate otherwise. Another common issue is ignoring negative signs, especially when simplifying expressions or solving equations. Misplacing or overlooking a negative sign can change the entire solution.
Additionally, many students make the mistake of failing to check their work. Once you’ve completed a problem, it’s essential to go back and verify each step to ensure no errors were made during the process. This habit not only catches mistakes but also reinforces the correct methods for future problems.
Why Solution Guides Are Important
Having access to solution guides is an essential part of the learning process in mathematics. These resources provide students with a clear path to understanding how to solve various types of problems. They not only offer the correct results but also break down the steps needed to reach those results, helping learners gain insight into the methods behind the solutions.
One of the primary benefits of using solution guides is that they allow students to check their work and identify where they may have gone wrong. This immediate feedback is invaluable for recognizing mistakes and understanding how to correct them. It also offers an opportunity for students to refine their techniques and solidify their grasp on key concepts.
Additionally, these guides support independent learning by enabling students to work through problems at their own pace. Rather than relying solely on teachers or tutors for assistance, learners can consult the solutions to better understand the process and apply it to similar problems. Over time, this builds confidence and enhances overall problem-solving abilities.
Tips for Studying Basic Math
Effective studying in mathematics goes beyond simply completing exercises. It requires a structured approach that builds a deep understanding of the concepts and ensures consistent improvement. By adopting the right study habits, students can enhance their ability to solve problems and retain critical information. Below are some strategies that can help you master essential mathematical skills quickly and efficiently.
1. Practice Regularly
Mathematics is a subject that requires consistent practice. The more you work on problems, the more familiar you become with different methods and techniques. Set aside time each day to work through exercises, focusing on both easy and challenging problems. This will help reinforce concepts and build confidence in your abilities.
2. Understand, Don’t Memorize

Rather than memorizing formulas and procedures, focus on understanding the reasoning behind them. This deeper comprehension allows you to apply your knowledge to a wider range of problems. When you truly grasp how and why certain methods work, you’re better equipped to solve complex challenges.
Additionally, review previous lessons regularly to ensure you retain what you’ve learned. Concepts in math often build upon each other, so maintaining a solid foundation is crucial for tackling more advanced material later on.
Breaking Down Complex Math Problems
Complex mathematical problems can often feel overwhelming, but breaking them down into smaller, more manageable steps makes them much easier to solve. Instead of attempting to tackle the entire problem at once, focus on one part at a time. By simplifying each section, you can understand the logic behind each step and work your way toward the solution more confidently.
1. Identify Key Information
The first step in solving a complex problem is to identify the most important elements. Look for numbers, variables, and relationships between them. Understanding what is being asked and what information you already have will help guide the entire process. It’s crucial not to get distracted by irrelevant details–focus only on what’s necessary to solve the problem.
2. Break the Problem into Steps
Once you’ve identified the key information, start breaking the problem down into smaller steps. Begin with simple operations or expressions and gradually build up to the more complex parts. This allows you to approach the problem in stages, ensuring that you don’t overlook any critical components. Each step should feel like a smaller puzzle piece that fits into the larger picture, helping you stay organized and focused throughout the process.
By simplifying the problem in this way, you’re more likely to identify patterns or common techniques that can be applied across similar problems. As you gain more experience, these strategies will help you solve even more challenging problems with ease.
How to Approach Word Problems
Word problems can often seem tricky because they require translating real-world situations into mathematical equations. However, with a systematic approach, they can become much easier to handle. The key is to break down the information provided, identify the relationships between variables, and translate the narrative into solvable steps. Here’s how you can approach them effectively:
- Read the problem carefully: Begin by reading the problem through once or twice to ensure you understand what’s being asked. Pay close attention to the details, such as numbers, units, and keywords that indicate operations (e.g., “total,” “difference,” “product”).
- Identify the unknowns: Determine what you need to find. These are usually the variables you’ll solve for. Label them clearly, whether it’s “x,” “y,” or another symbol.
- Extract relevant information: Highlight or underline the key facts. This could include quantities, rates, or conditions given in the problem. Discard irrelevant details that won’t help in solving the equation.
- Translate into a mathematical equation: Convert the problem into an equation or set of equations based on the relationships between the numbers. Use addition, subtraction, multiplication, or division, as needed.
- Solve the equation: Follow the standard steps for solving the equation, using proper operations and checking your work as you go.
- Review and interpret your answer: Once you have a solution, revisit the original problem to ensure your answer makes sense in context. Verify units and ensure that the result answers the specific question asked.
By following these steps methodically, you can approach any word problem with confidence and accuracy. The more you practice this process, the more intuitive it will become, making it easier to solve problems quickly and correctly.
Improving Your Math Skills with Practice
One of the most effective ways to strengthen your math skills is through consistent practice. The more you work on problems, the more familiar you become with different techniques, patterns, and methods. Practicing regularly helps reinforce your understanding of core concepts and improves your ability to apply them in various scenarios. It also boosts confidence, making you more comfortable with challenging problems.
To track your progress and identify areas for improvement, it’s helpful to practice a variety of problem types. Below is a table that outlines how different levels of practice can help improve different aspects of mathematical problem-solving:
| Practice Level | Focus Area | Benefit |
|---|---|---|
| Basic Exercises | Simple calculations and operations | Builds foundational skills and reinforces key concepts |
| Intermediate Problems | Word problems, multi-step equations | Enhances problem-solving strategies and logical thinking |
| Advanced Challenges | Complex equations, abstract concepts | Develops critical thinking and prepares for more difficult topics |
Regular practice at varying levels not only helps you improve your technical skills but also strengthens your ability to approach new problems with confidence. Each type of problem you encounter builds upon your previous knowledge, creating a solid foundation that supports more advanced work.
Understanding Math Word Problems
Math word problems can often be tricky because they require you to translate real-world scenarios into mathematical expressions. The key to solving these problems is to break down the information provided, identify the relationships between different quantities, and then create a plan to find the solution. By approaching word problems systematically, you can make sense of the text and convert it into something solvable.
Start by reading the problem carefully and identifying the important details, such as numbers, units of measurement, and key phrases that indicate operations (e.g., “sum,” “difference,” “product”). Once you’ve gathered the information, think about what you are being asked to find and what equations or operations are needed to solve the problem. This step-by-step approach ensures that nothing is overlooked and helps avoid mistakes in the solution process.
Another helpful technique is to visualize the problem whenever possible. Drawing a diagram, chart, or table can make abstract concepts more tangible and easier to manipulate. This visual representation can clarify relationships between values, making it easier to understand how to apply the right operations. Over time, as you practice more, this method will become second nature and improve your ability to solve complex problems quickly and accurately.
Unlocking the Secrets of Mathematical Equations
Mathematical equations can appear daunting at first, but once you understand the underlying principles, solving them becomes much more manageable. At their core, equations represent relationships between variables and constants, and unlocking these relationships is key to finding the solution. The process of solving equations involves isolating the unknowns, applying operations systematically, and using logic to find the correct answer.
1. Recognizing Patterns in Equations
The first step in tackling any equation is recognizing the patterns that govern its structure. Most equations follow a set of rules or operations that, when understood, make the problem easier to solve. Whether it’s balancing both sides of the equation, using inverse operations, or simplifying expressions, spotting these patterns helps guide your approach. Over time, these patterns become intuitive, making it easier to solve more complex equations.
2. Using Step-by-Step Methods
Breaking the problem down into smaller, more manageable steps is one of the most effective ways to solve equations. Start by isolating the variable, performing operations on both sides, and simplifying the expression as you go. For example, if the equation involves addition or subtraction, reverse those operations first to eliminate constants from the variable side. Then, move on to multiplication or division if necessary. By working through each operation carefully, you can solve even the most complicated equations with confidence.
Patience and consistency are essential when solving equations. Each equation is a puzzle, and with practice, you’ll become faster and more accurate at identifying the best strategies for solving them.
The Role of Solution Guides in Learning

Solution guides play a crucial role in the learning process by providing students with immediate feedback on their work. These resources help learners verify their answers, understand the steps involved in solving a problem, and identify any mistakes they may have made. Rather than simply offering the correct solution, they serve as a tool for deeper understanding and self-correction, enabling students to learn from their errors and refine their problem-solving techniques.
One of the key benefits of using solution guides is that they encourage independent learning. Students can check their work without always needing assistance from a teacher or tutor. This allows them to take ownership of their learning process, develop critical thinking skills, and become more confident in their abilities. When used effectively, solution guides promote active learning and help students develop the problem-solving skills needed for success in future topics.
Additionally, these guides are essential for reinforcing concepts that students may struggle with. By working through the steps outlined in the solutions, learners can see the logic behind each operation and gain a clearer understanding of the underlying principles. Over time, this repeated exposure strengthens their grasp on key concepts, making it easier to tackle increasingly complex problems.
How Solution Guides Can Boost Confidence

Solution guides are powerful tools that help students build confidence in their problem-solving abilities. By providing immediate feedback, these guides allow learners to check their work, identify areas of improvement, and gain assurance in their skills. When students can independently verify their answers and understand the reasoning behind them, they feel more capable and motivated to tackle more complex problems.
- Instant Feedback: A solution guide offers quick verification of answers, helping students see where they’ve succeeded or where they need to adjust. This instant feedback fosters a sense of accomplishment and helps reduce the anxiety that often accompanies learning new concepts.
- Clarifying Mistakes: Mistakes are a natural part of learning, but they can be discouraging. By showing the correct steps and solutions, guides help students understand where they went wrong and how to correct their errors, turning mistakes into valuable learning opportunities.
- Building Independent Learning Habits: The ability to check and adjust their own work empowers students to become more self-reliant. As they become more proficient in using guides, they develop the confidence to work through problems independently, making them better prepared for future challenges.
Over time, regular use of solution guides builds a strong foundation of trust in one’s own abilities. As students repeatedly see that their understanding is improving, they develop a positive mindset, knowing that with practice and persistence, they can master any concept. This growing confidence makes tackling new and harder problems seem less intimidating and more achievable.