Webassign Calculus 3 Answer Key and Solutions Guide

In the world of higher education, tackling complex mathematical challenges can be daunting. However, with the right resources and strategies, students can not only understand the core concepts but also excel in their coursework. A vital tool in this learning journey is having access to reliable resources that provide thorough solutions to intricate exercises. These solutions help to break down each problem step by step, enabling students to grasp difficult material more effectively.

By utilizing solutions for advanced math assignments, students can verify their approaches and refine their problem-solving techniques. Whether it’s working through intricate integrals or solving multi-variable equations, having access to the right answers can serve as both a learning tool and a guide. With careful study of the provided solutions, learners can gain deeper insights and strengthen their grasp of the subject.

Focusing on the details of each solution allows students to see where they might have made errors in their reasoning and how to correct them. In turn, this practice leads to better performance in exams and assignments, equipping them with the necessary skills to succeed in future mathematical challenges.

Webassign Calculus 3 Answer Key Overview

In the study of higher mathematics, having access to comprehensive problem-solving guides is essential for mastering complex topics. These resources not only provide solutions but also explain the methodology behind each step. Understanding the process involved in solving intricate exercises can significantly enhance a student’s comprehension and ability to approach similar challenges in the future. This section will give an overview of how detailed solutions can support learning and improve performance in advanced math courses.

How Solution Guides Support Learning

Solution resources serve multiple purposes. They help clarify difficult concepts, reinforce learning, and offer a structured approach to solving problems. Key aspects of these resources include:

• Breaking down complex problems into manageable steps
• Providing clear explanations for each solution
• Helping identify common mistakes and offering corrective strategies
• Serving as a reference for similar exercises

Benefits of Using Solution Guides

Accessing detailed solutions for advanced math assignments can have a profound impact on academic success. Among the main advantages are:

1. Improved understanding of problem-solving strategies
2. Increased confidence in tackling similar problems independently
3. Better time management during tests and assignments
4. Enhanced critical thinking and analytical skills

By using these resources effectively, students can not only improve their grades but also develop a deeper appreciation for the subject matter. The key is to understand the reasoning behind each solution, which lays a solid foundation for future success in more advanced topics.

Understanding Platform for Advanced Math Courses

The digital learning environment for higher-level mathematics offers a variety of tools designed to support students in mastering complex topics. These platforms provide interactive exercises, immediate feedback, and access to detailed solutions that help students refine their problem-solving skills. Understanding how to navigate and utilize these resources effectively can significantly enhance a student’s ability to succeed in challenging courses and assignments.

Features of the Learning Platform

The platform is designed to streamline the learning process by offering a user-friendly interface and a range of features. Key aspects include:

• Interactive problem sets with instant feedback
• Access to step-by-step solutions for practice problems
• Trackable progress and performance analytics
• Collaboration tools for student-instructor interaction

Maximizing the Platform’s Potential

To get the most out of the platform, it’s important to approach it with a strategy that emphasizes understanding rather than just completing tasks. Here are some tips for effective use:

1. Start by reviewing the feedback provided for each problem
2. Break down complex problems into smaller, manageable steps
3. Make use of available resources, such as video tutorials and sample problems
4. Consistently track progress to identify areas for improvement

By actively engaging with the platform, students can build a solid foundation in their subject, improve their understanding of key concepts, and better prepare for exams and assignments.

How to Access Answer Resources for Advanced Math Assignments

Accessing detailed solutions for complex mathematical exercises can be crucial for mastering advanced topics. Many educational platforms offer these resources as part of their interactive learning tools. Understanding how to locate and effectively use these solutions can enhance your problem-solving skills and provide deeper insights into difficult material. In this section, we will guide you through the process of finding these resources and utilizing them for academic success.

Steps to Access the Solutions

To access the solutions for your assignments, follow these simple steps:

1. Log into your account on the learning platform.
2. Navigate to the course or assignment section.
3. Look for the specific exercise or chapter you need help with.
4. Click on the provided link or tab for solutions or resources.
5. Review the step-by-step breakdown to understand the solution process.

Solution Availability by Topic

Different topics may have varying levels of solution availability depending on the course. Below is a general overview of how solutions are typically structured:

Topic	Availability	Resource Type
Integration	Full solutions available	Step-by-step walkthrough
Multivariable Functions	Partial solutions available	Hints and guidance
Differential Equations	Full solutions available	Text and video explanations

By following the steps above, you can easily access the solutions and use them to improve your understanding of the material. Additionally, utilizing various types of resources–such as hints, full solutions, and video guides–can provide a more comprehensive approach to mastering the concepts.

Step-by-Step Guide for Advanced Math Problems

When tackling complex mathematical exercises, breaking them down into smaller, more manageable steps is essential for understanding the underlying concepts. A structured approach not only helps clarify each stage of the problem-solving process but also improves accuracy and efficiency. This guide provides a clear outline for solving challenging problems by following a methodical process from start to finish.

1. Analyze the Problem

The first step in solving any advanced problem is to carefully read and understand the question. Pay attention to key information and identify the type of problem you’re dealing with. Consider the following:

• What is the problem asking you to find?
• What information is provided, and what do you need to calculate?
• Are there any specific constraints or conditions given?

2. Set Up the Problem

Once you understand the problem, begin to organize the information and choose the appropriate method for solving it. This may include:

• Identifying the relevant formulas or concepts
• Determining the variables and constants involved
• Choosing the right approach (e.g., integration, differentiation, etc.)

3. Solve the Problem

Now, apply the selected method to begin solving the problem step by step. Be sure to:

• Perform calculations carefully and systematically
• Show all intermediate steps to avoid mistakes
• Check for any simplifications that can be made along the way

4. Review Your Solution

After solving the problem, take a moment to review your work and ensure that your solution is correct. Double-check:

• All calculations
• That you’ve answered the question as asked
• The units and format of the final answer

By following this structured approach, you can effectively tackle even the most challenging exercises, improving both your understanding and problem-solving abilities in the process.

Common Mistakes in Advanced Math Assignments

When working through challenging math exercises, it is easy to make small errors that can lead to incorrect solutions. Identifying and understanding these common mistakes is crucial for improving accuracy and avoiding frustration. In this section, we will highlight frequent pitfalls that students encounter and offer tips for overcoming them.

Common Mistakes and How to Avoid Them

Many of the errors that occur in advanced math problems are due to misunderstandings of key concepts or oversights in the problem-solving process. The following table outlines some typical mistakes and strategies to avoid them:

Common Mistake	Explanation	How to Avoid It
Skipping Steps in Calculation	Rushing through the process can lead to missed operations or incorrect simplifications.	Write out each step clearly to track your work and catch mistakes early.
Misapplying Formulas	Using the wrong formula or applying it incorrectly can lead to wrong answers.	Ensure you understand which formulas apply to the specific problem and verify each step.
Not Checking for Units	Overlooking the proper units in calculations can cause confusion or incorrect solutions.	Always double-check that the units match and are consistent throughout your solution.
Ignoring Signs in Equations	Signs can easily be missed, especially when working with multiple terms.	Be vigilant with signs, especially when expanding or factoring expressions.
Incorrectly Interpreting the Problem	Misunderstanding the question can result in solving the wrong type of problem.	Read the problem carefully and identify exactly what is being asked before beginning.

By being aware of these common errors and following best practices for problem-solving, students can improve both their accuracy and confidence when working through challenging exercises.

Using Platform Tools to Improve Your Math Skills

Enhancing your problem-solving abilities and understanding complex mathematical concepts requires consistent practice and the right resources. A robust online platform can offer a variety of tools designed to support your learning journey. By utilizing interactive exercises, immediate feedback, and comprehensive explanations, students can significantly strengthen their skills in tackling difficult topics.

The platform’s structure allows for a more personalized approach to learning, offering step-by-step guidance and the opportunity to revisit problems multiple times. Using these tools effectively can help you grasp advanced concepts faster and build a solid foundation for future challenges. Whether you are reviewing previous assignments or working on new exercises, you can take advantage of tailored support to improve your overall performance.

Benefits of Using the Platform

By actively engaging with the resources available on the platform, you can:

• Receive immediate feedback on your solutions, helping you identify areas for improvement.
• Reinforce concepts by practicing problems in a variety of formats and difficulty levels.
• Track your progress to ensure that you are mastering the material before moving on to more complex topics.
• Utilize available explanations and examples to understand the process behind solving each problem.

Maximizing the Platform’s Features

To get the most out of the platform, consider the following strategies:

• Start with simpler problems to build confidence and gradually work up to more challenging exercises.
• Use the hints and step-by-step breakdowns to guide your understanding when you get stuck.
• Review your mistakes and try similar problems to reinforce your understanding and avoid repeating errors.

By dedicating time and effort to using these tools, you can significantly enhance your ability to solve complex mathematical problems and achieve greater success in your studies.

Best Practices for Solving Advanced Math Problems

To successfully navigate complex mathematical tasks, following structured methods and strategies is crucial. Adopting best practices not only improves the accuracy of your solutions but also enhances your problem-solving skills over time. By staying organized, checking your work, and using available resources effectively, you can significantly boost your performance and understanding.

Key Strategies for Success

To maximize your effectiveness when working through math problems, consider incorporating the following best practices:

• Understand the Problem First: Carefully read and analyze the question before jumping into calculations. Identifying the goal and gathering all necessary information is essential.
• Break Down the Problem: Divide the problem into smaller, manageable parts. Solve each section step by step to avoid feeling overwhelmed and ensure that no part of the problem is skipped.
• Show Your Work: Write out every step clearly, even if they seem simple. This helps prevent errors and makes it easier to spot mistakes if they arise.
• Double-Check Your Calculations: Always review your work before submitting. It’s easy to overlook small mistakes, especially with complex equations, so taking a few moments to verify your answers can save time in the long run.
• Use Available Tools: Take full advantage of hints, explanations, and examples to guide your process when needed. These resources can provide valuable insights and help clarify tricky steps.

How to Effectively Use Feedback

Feedback is an essential part of the learning process. After receiving feedback on your solutions, use it as an opportunity to improve:

• Review Mistakes Thoroughly: Understand where you went wrong and why. This can help prevent similar errors in the future and strengthen your grasp on the material.
• Reattempt Problems: Try solving the same problems again after reviewing feedback. This repetition will reinforce the correct methods and help you internalize the material.
• Ask for Clarification: If any part of the feedback is unclear, don’t hesitate to seek clarification from a teacher, tutor, or peer.

By following these best practices, you can ensure that you not only complete your tasks efficiently but also deepen your understanding of advanced topics and become more confident in solving difficult mathematical problems.

Solutions for Complex Integral Problems

Solving intricate integrals that involve multiple variables or complex functions can be daunting. However, mastering the methods required to tackle these problems is crucial for progressing in advanced mathematical studies. By breaking down the process and understanding the underlying concepts, students can improve their approach to solving complex integrals and ensure more accurate results.

Common Techniques for Solving Complex Integrals

When working with integrals that involve complex functions, there are several essential techniques to keep in mind:

• Substitution Method: A common technique used to simplify integrals, especially when dealing with composite functions. Carefully identify the right substitution to transform the integral into a simpler form.
• Integration by Parts: This method is useful when the integral consists of the product of two functions. It involves applying the integration by parts formula to break down the problem.
• Contour Integration: A powerful technique used for evaluating integrals in the complex plane, particularly when dealing with singularities or multi-variable functions.
• Partial Fractions Decomposition: Used when dealing with rational functions, this method helps break down complex rational expressions into simpler fractions that are easier to integrate.
• Residue Theorem: A key tool in evaluating complex integrals, especially in contour integration. This theorem helps calculate integrals by focusing on the residues of singularities within a closed contour.

Step-by-Step Approach for Solving Complex Integrals

To solve complex integrals effectively, it’s important to follow a systematic approach:

• Step 1: Identify the type of integral – Analyze the integral to determine which technique is most appropriate for solving it.
• Step 2: Simplify the integrand – If possible, use substitutions or break down the expression to make it easier to handle.
• Step 3: Apply the chosen method – Implement the technique that best fits the problem, whether it’s substitution, parts, or contour integration.
• Step 4: Evaluate the integral – Solve the integral step by step, ensuring to track any constants or variables as you work through the problem.
• Step 5: Verify the solution – Always check your final result against the conditions of the problem, ensuring all aspects have been addressed.

By following these steps and understanding the key techniques, students can develop a more systematic and efficient approach to solving complex integrals, leading to a deeper understanding of multi-variable functions and integration methods.

Mastering Multivariable Functions

Understanding and solving problems related to functions with more than one variable can be challenging but rewarding. These types of functions are foundational in many advanced mathematical and engineering disciplines. By practicing the various techniques for handling multivariable functions, students can build a strong understanding and improve their problem-solving skills.

Key Concepts for Mastering Multivariable Functions

To effectively work with functions involving multiple variables, consider focusing on these essential concepts:

• Partial Derivatives: A fundamental tool for analyzing how a multivariable function changes with respect to one variable at a time while holding others constant.
• Gradient and Directional Derivatives: Understanding how the gradient vector points in the direction of the steepest ascent can help you determine the rate of change in any direction.
• Multiple Integrals: Used to find quantities such as area, volume, and mass when dealing with two or more variables. Mastering double and triple integrals is essential in this context.
• Level Curves and Surfaces: Visualizing these functions can provide a deeper insight into their behavior, helping to identify important features such as maxima, minima, and saddle points.
• Optimization Techniques: Learning how to find local maxima and minima of functions with several variables is crucial for solving real-world problems in fields such as physics, economics, and engineering.

Effective Strategies for Solving Problems

To improve your ability to solve multivariable function problems, adopt these strategies:

• Step-by-Step Approach: Start by carefully analyzing the problem. Identify which variables are involved and the relationships between them before proceeding with calculations.
• Graphical Representation: Whenever possible, use graphs and level curves to visualize the function. This can provide insight into its behavior and help guide the solution process.
• Use Symmetry: Many problems involving multivariable functions have symmetrical properties. Identifying these can simplify calculations and make it easier to solve the problem.
• Check Units and Conditions: Always ensure that your solution makes sense in the context of the problem. Verify your units and check if the conditions given in the problem are met.

By focusing on these strategies and regularly practicing different types of multivariable problems, you will develop a more intuitive understanding of these complex functions and enhance your overall mathematical skills.

Tips for Solving Differential Equations

Solving differential equations can be a challenging yet essential skill in advanced mathematics and applied sciences. These equations model a variety of real-world phenomena, from physics to engineering and economics. Mastering the techniques for solving these problems requires both understanding the theory and practicing the methods that simplify complex equations.

To effectively tackle differential equations, it is important to focus on key strategies that allow you to identify appropriate methods and make the solution process more manageable. The following tips can guide you in solving both ordinary and partial differential equations.

Key Strategies for Effective Problem Solving

• Classify the Equation: Determine the type of equation you are working with, such as linear, separable, or exact. Recognizing the form early on helps you choose the right solution method.
• Use Substitution Techniques: In many cases, substituting variables or using transformations can simplify the equation. Common methods include substituting for specific terms or employing trigonometric identities.
• Check for Integrating Factors: For linear equations, look for an integrating factor that will make the equation easier to solve. This often simplifies the integration process, turning a non-exact equation into an exact one.
• Understand Boundary Conditions: Always consider the given boundary or initial conditions, as these will guide you toward finding a particular solution, rather than a general one.
• Practice With Numerical Methods: While analytic solutions are ideal, some equations may not have a simple closed-form solution. In such cases, numerical methods like Euler’s method or Runge-Kutta can provide approximate solutions.

Common Mistakes to Avoid

• Forgetting to Check Domain Restrictions: Many differential equations have domain restrictions that must be considered when finding the solution. Ignoring these can lead to incorrect or incomplete results.
• Misapplying Solution Methods: Applying the wrong method for the equation type can lead to unnecessary complexity or incorrect answers. Always verify the method you choose is suitable for the problem at hand.
• Skipping Intermediate Steps: Rushing through the solution process or skipping intermediate steps may cause you to miss important details, especially when working with complex functions or conditions.

By focusing on these strategies and avoiding common mistakes, you can significantly improve your ability to solve differential equations and gain a deeper understanding of their applications in various fields.

How to Navigate Through the Platform’s Interface

Mastering the navigation of an online learning platform is essential for efficiently managing assignments, accessing resources, and staying on top of coursework. The interface of such platforms is designed to provide easy access to all necessary tools, but it can seem overwhelming at first. Understanding how to navigate it will save time and reduce frustration.

Understanding the Dashboard

The dashboard serves as your starting point, providing an overview of all active assignments, deadlines, and progress. Familiarizing yourself with this area is the first step toward seamless use of the platform. Key features of the dashboard typically include:

• Upcoming Deadlines: A section displaying assignments with their due dates, so you can plan your study time effectively.
• Recent Activity: A feed of your recent work, including completed assignments and new tasks assigned by your instructor.
• Resource Links: Quick access to course materials such as textbooks, practice exercises, and additional reading resources.

Assignment Navigation and Submission

Once you’re familiar with the dashboard, the next step is to learn how to navigate individual assignments. This includes understanding how to start, complete, and submit tasks efficiently:

• Accessing Assignments: Click on the assigned task to open the problem set or exercise. You’ll be taken directly to the question page where you can input your answers.
• Tracking Progress: Many platforms allow you to track your progress throughout an assignment, showing which questions have been answered and which are still pending.
• Submitting Solutions: Once you’ve completed a task, ensure that you submit it before the deadline. Double-check your answers and confirm that everything is saved correctly before submitting.

Helpful Tools and Features

Several built-in tools can assist you in completing assignments more effectively:

• Hints and Feedback: Some platforms offer hints or immediate feedback after each question to guide your learning. Take advantage of these tools to enhance your understanding of the material.
• Progress Tracking: A progress bar or completion percentage can help you visualize how much of the assignment is left to do and keep you on track.
• Help and Support: If you encounter any issues with the interface or an assignment, most platforms have a support section or FAQs that can assist you.

Important Navigation Tips

To maximize your efficiency while using the platform, consider these additional tips:

• Use Keyboard Shortcuts: Some platforms offer keyboard shortcuts for quick navigation, such as jumping to the next question or saving your progress.
• Stay Organized: Keep track of due dates and assignments by using a digital or physical planner, and set reminders to stay ahead.
• Review Before Submitting: Always review your responses and ensure all tasks are marked as complete before final submission.

With a clear understanding of the platform’s interface and navigation tools, you’ll be able to manage your coursework more effectively and ensure a smoother experience throughout the semester.

Understanding Limits and Continuity in Advanced Mathematics

In higher-level mathematical analysis, the concepts of limits and continuity form the foundation for understanding how functions behave near specific points or over entire domains. These ideas are critical in exploring more complex functions and understanding the structure of solutions to problems. A deep comprehension of these principles enables students to approach and solve problems involving multi-variable functions, integrals, and more advanced topics.

Limits: The Core Concept

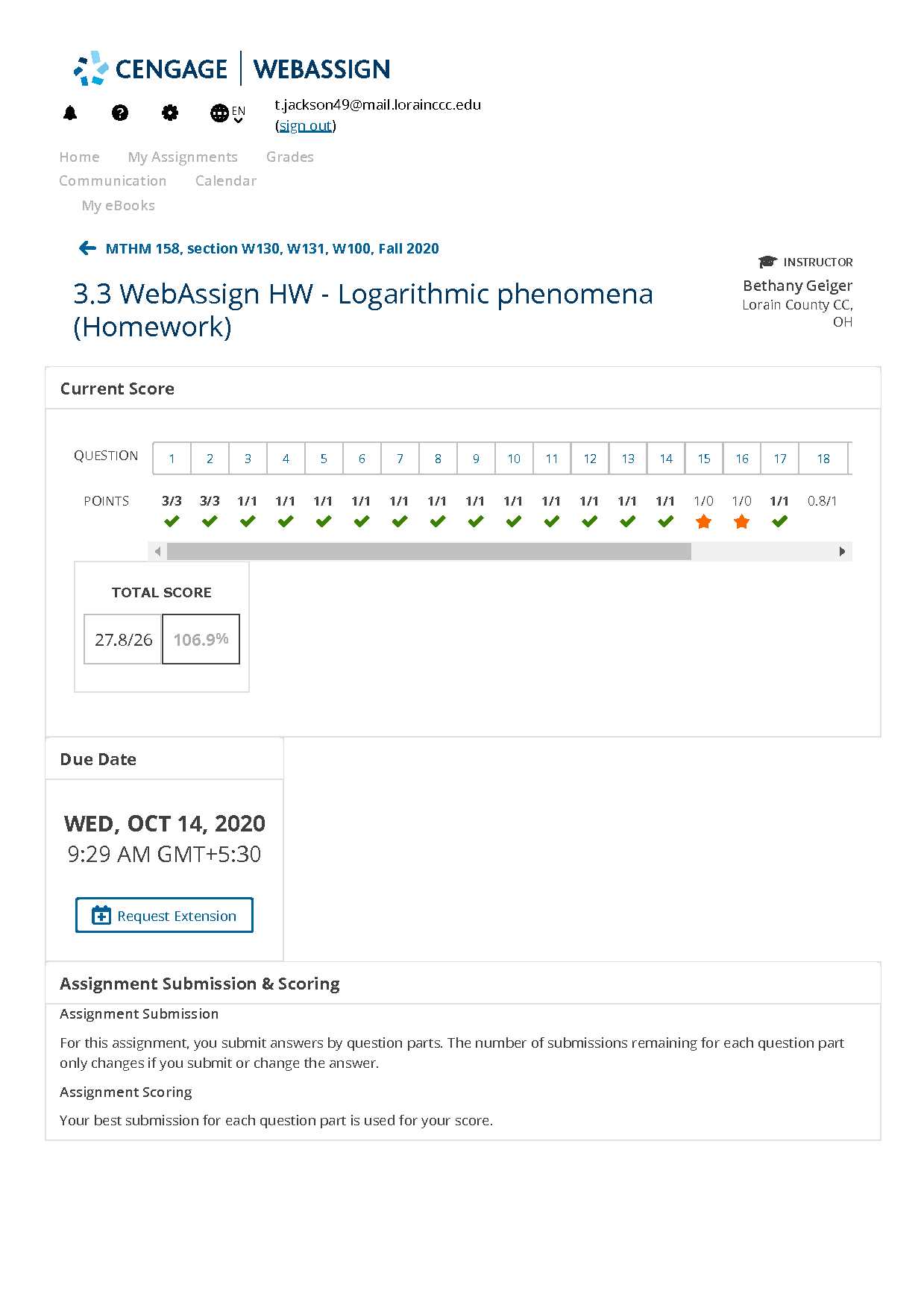
At the heart of many mathematical problems is the concept of a limit, which describes how a function behaves as it approaches a specific point. This idea is essential in determining the behavior of a function at points where it may not be directly defined. Limits help to formalize ideas of approaching values and allow us to explore the behavior of functions even when direct evaluation is impossible.

• Finite Limits: These describe situations where the function approaches a finite value as the input approaches a specific point.
• Infinite Limits: These occur when the function’s output increases or decreases without bound as the input approaches a certain value.
• One-Sided Limits: A one-sided limit examines the behavior of a function as it approaches a point from only one direction (either from the left or right).

Continuity: The Connection Between Limits and Functions

Continuity describes the behavior of a function in terms of its smoothness and the absence of sudden jumps or breaks. A function is said to be continuous at a point if the limit at that point exists, is finite, and equals the function’s value at that point. Understanding continuity is key to ensuring that a function behaves predictably over its domain.

• Continuous Functions: A function is continuous if, at every point within its domain, the limit as the input approaches that point is equal to the function’s value.
• Discontinuities: Discontinuities occur when a function fails to be continuous at one or more points. These may include removable discontinuities, jump discontinuities, or infinite discontinuities.
• Types of Continuity: Functions can be classified as continuous or discontinuous depending on how they behave across intervals of their domain.

By mastering the principles of limits and continuity, students can better understand the behavior of more complex mathematical structures, leading to deeper insights in higher-level courses and applications.

How to Use the Platform for Practice Sets

Online learning platforms offer a great way to practice and refine your problem-solving skills through various exercises. These practice sets are designed to help students tackle different types of problems, improve their understanding of complex topics, and reinforce key concepts through repetition. By engaging with these exercises, learners can solidify their knowledge and enhance their problem-solving abilities.

To make the most out of these practice sets, it’s important to approach them strategically and utilize all available resources. Here are some effective strategies to maximize the benefits:

• Start with the Basics: Begin by working through simpler problems to establish a strong foundation before moving on to more challenging ones.
• Track Your Progress: Many platforms provide tools to track your progress, which can help you identify areas where improvement is needed. Regularly review your mistakes and focus on those areas.
• Utilize Hints and Solutions: If you’re stuck on a problem, use the provided hints or review the solutions to understand the approach. These resources can guide you to the correct method without giving away the complete answer.
• Practice Consistently: Set aside time each day or week to work through practice problems. Consistent practice is key to mastering difficult concepts.

By following these tips and leveraging the full range of available exercises, you can deepen your understanding of the material and improve your overall performance in the subject.

Key Strategies for Homework Success

Effective completion of assignments requires a strategic approach that balances time management, problem-solving techniques, and understanding of the material. Students can significantly improve their performance by adopting the right methods and tools while working on their tasks. The following strategies are essential for mastering difficult topics and achieving success in assignments.

• Plan Ahead: Start your assignments early to avoid last-minute stress. Break them down into manageable sections, and allocate specific time blocks for each task.
• Understand the Concepts: Before diving into the exercises, ensure you have a solid grasp of the underlying theories. Review any notes, textbooks, or other resources to strengthen your understanding.
• Use Available Resources: Many platforms and textbooks offer helpful resources, including examples, hints, and step-by-step solutions. These can guide you when you encounter particularly challenging problems.
• Stay Organized: Keep track of deadlines, assignments, and your progress. Organize your work area and materials to minimize distractions and improve focus.
• Review and Revise: After completing a task, always review your work. Double-check your calculations, ensure your answers are correct, and refine your methods where needed.
• Seek Help When Needed: Don’t hesitate to ask for help from instructors, peers, or online forums when you’re struggling with a problem. Clarifying doubts early prevents confusion later on.

By incorporating these strategies into your study routine, you can approach your homework with greater confidence and efficiency, ultimately achieving better results and a deeper understanding of the material.

Reviewing Solution Methods for Advanced Math Problems

Understanding the steps involved in solving complex mathematical problems is essential for students aiming to master advanced topics. There are several methods and techniques that help simplify and solve intricate problems efficiently. By reviewing and practicing these methods, learners can improve their problem-solving skills and gain deeper insights into the subject matter.

Method 1: Breaking Down Complex Problems

One of the most effective strategies is breaking a complicated problem into smaller, more manageable parts. This approach helps you focus on each section individually, making it easier to identify patterns and relationships between different variables. Start by carefully reading the problem and identifying the key components. Then, solve each smaller problem step by step, combining the solutions as you go.

Method 2: Applying Formulas and Theorems

Many problems can be solved by applying well-known formulas and theorems. Understanding when and how to use these formulas is critical for achieving correct results. For example, concepts such as derivatives, integrals, and multivariable functions each have their own set of rules that simplify the process. Make sure you memorize these key formulas and practice using them in various contexts.

Key Tips:

• Practice regularly: Consistent practice reinforces the application of formulas and helps solidify your understanding.
• Know when to simplify: In many cases, simplifying expressions before applying a formula can make the solution process much easier.

Method 3: Using Visual Aids and Graphs

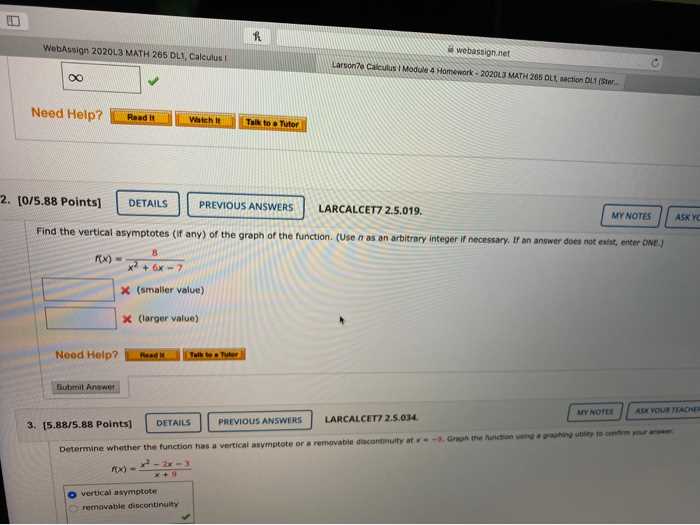
Sometimes, visualizing a problem can make it much easier to solve. Graphs, charts, and diagrams can help illustrate relationships and allow you to see the problem from a different perspective. When dealing with multivariable functions or integrals, visual representations often provide valuable insights that help clarify the solution process.

By reviewing these methods and integrating them into your study routine, you can improve your ability to solve complex problems more effectively and with greater confidence.

Getting Help with Harder Math Problems

As you tackle more challenging mathematical problems, it is normal to encounter roadblocks that can make progress difficult. Fortunately, there are several resources and strategies available that can guide you through these tough problems. Seeking help from different avenues can offer fresh perspectives and solutions that may not have been apparent at first.

Utilizing Online Resources

In today’s digital age, the internet offers a wealth of tools and platforms designed to help students overcome difficult problems. Websites that focus on math education provide step-by-step explanations, video tutorials, and interactive problem solvers. These resources can break down complex problems into understandable components, helping you approach the problem from various angles.

Resource Type	Benefits
Video Tutorials	Visual explanations that break down difficult topics into easy-to-follow steps.
Interactive Solvers	Automated tools that offer immediate feedback and step-by-step solutions.
Online Forums	Community-driven help with diverse problem-solving approaches and discussions.

Seeking Help from Professors and Peers

Another valuable source of support is your professor or classmates. Professors can provide office hours or one-on-one assistance, explaining difficult concepts in more depth. Additionally, study groups with peers allow for collaborative problem solving, where you can share insights and learn new techniques from others. Many students find that discussing problems with classmates helps clarify their own understanding.

By leveraging both online tools and human resources, you can strengthen your ability to solve even the most difficult problems. Combining these strategies with your own practice will help build a deeper understanding and improve your problem-solving skills over time.

Using Solutions to Enhance Your Learning

Having access to completed solutions can significantly improve your understanding of complex mathematical problems. By reviewing how problems are solved step by step, you can identify key strategies, uncover common patterns, and reinforce your own problem-solving skills. Solutions provide a roadmap, showing you not only the correct result but also the methods used to reach it.

When you use solutions as a learning tool, it’s important to focus on understanding the reasoning behind each step rather than just memorizing the final answer. This process helps build a deeper conceptual understanding and allows you to apply similar techniques to different problems in the future.

Here are a few strategies for using solutions effectively:

• Compare Your Work: After attempting a problem, compare your solution with the provided one. Look for any differences in approach and analyze why one method may be more efficient than another.
• Identify Common Techniques: Many problems in advanced math require similar techniques. By studying the solutions, you can familiarize yourself with these methods and use them in future exercises.
• Focus on Problem Structure: Pay attention to how the problem is broken down. Understanding how to organize and approach a problem is just as important as getting the right answer.

By incorporating solutions into your study routine, you can enhance your comprehension and develop the skills necessary to tackle more advanced topics with confidence.